Question

The circuit shown in the figure is in steady state. Find the potential difference across the capacitor.

(A) $V$
(B) $\dfrac{V}{2}$
(C) $\dfrac{V}{3}$
(D) $\dfrac{{2V}}{3}$

Answer 1

Hint Neglect the branch that has a capacitor attached with. Then, find the current in the remaining circuit. Then, select any one loop including the branch with capacitor and apply Kirchhoff’s Voltage Law.

Step by Step Solution

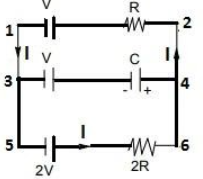
 When the circuit is in steady state, then, the branch having capacitor can be neglected or omitted. Then, the equivalent circuit becomes –



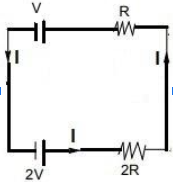
Then, find the equivalent current in the circuit by using Kirchhoff's Voltage Law for finding the current which states that, “the voltage across a loop is equal to the sum of every voltage drop is equal to zero in the same loop”.
Therefore, in the above circuit, applying Kirchhoff’s Law, we get –
$
   - V + 2V - IR - 2IR = 0 \\
  2V - V = I\left( {R + 2R} \right) \\
 $
By transposition, finding the value of current –
$
  I = \dfrac{{2V - V}}{{R + 2R}} \\
  I = \dfrac{V}{{3R}} \\
 $
Now, considering the below circuit –

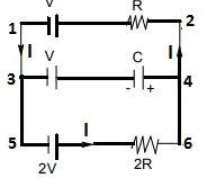
In the loop 43564, let the voltage across the capacitor be ${V_c}$. So, applying the Kirchhoff’s Law, we get –
$
   - {V_c} - V + 2V - 2IR = 0 \\
  {V_c} = - V + 2V - 2IR \cdots \left( 1 \right) \\
 $
We have already got the value of current, $I = \dfrac{V}{{3R}}$. So, putting this value in the equation, we get –
$
  {V_c} = - V + 2V - 2 \times \dfrac{V}{{3R}}2R \\
  {V_c} = V - \dfrac{{2V}}{3} \\
  {V_c} = \dfrac{V}{3} \\
 $
Hence, the value of voltage across the capacitor is $\dfrac{V}{3}$.

Therefore, the correct option is (C).

Note The Kirchhoff’s Laws helps us to calculate the electrical resistance of a complex network or impedance of Alternating Current (AC). It also helps us in calculating the current flow in different loops or streams of the circuit. There are following two Kirchhoff’s Laws
Kirchhoff’s Current Law It states that the total current inside the junction is equal to the sum of current outside the junction.
If current inside the junction is ${I_i}$ and current outside the junction is ${I_o}$. Then, according to the Kirchhoff’s Current Law –
$
  {I_i} = {I_o} \\
   \Rightarrow {I_i} - {I_o} = 0 \\
 $
And the other Kirchhoff’s Law is Kirchhoff’s Voltage Law.