Question

The angle of elevation of the top of a tree of height 18 meters is \[30^\circ \] when measured from a point $P$ in the plane of its base. The distance of the base of the tree from $P$ is
A. 6 m
B. $6\sqrt 3 $m
C. 19 m
D. $18\sqrt 3 $m

Answer 1

Hint: The given scenario can be represented by a figure. Draw the diagram of the given problem statement for a better understanding of the situation. Use the formula $\sin \theta = \dfrac{{{\text{Opposite}}}}{{{\text{Hypotenuse}}}}$ and $\tan \theta = \dfrac{{{\text{Perpendicular}}}}{{{\text{Base}}}}$ to find the value of the distance between the tree and the point $P$.

Complete step by step solution
Let us assume the distance between the tree and the point $P$ be \[x\] meters. It is given in the question that the angle of elevation of the top of the tree from the point $P$ is \[30^\circ \]. We can therefore draw the diagram representing the scenario.



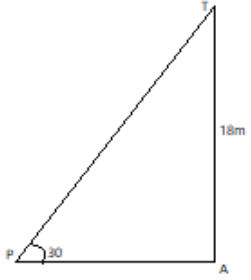
Here the line TA represents the tree height of 18 meters and the point $P$ is the point in the plane of the tree's base.

We know that in a right angled triangle the $\tan \theta = \dfrac{{{\text{Perpendicular}}}}{{{\text{Base}}}}$

In the triangle\[TPA\],
\[\tan {30^ \circ } = \dfrac{{TA}}{{PA}}\]
Substituting the value 18 for $TA$ and \[x\] for $PA$ in the equation, we get
\[\tan {30^ \circ } = \dfrac{{18}}{x}\]
Solving the above equation for \[x\]
$
  \dfrac{1}{{\sqrt 3 }} = \dfrac{{18}}{x} \\
  x = 18\sqrt 3 \\
$
Thus the horizontal distance of the base of the tree from $P$ is \[18\sqrt 3 \]m.
Therefore the option D is the correct answer.

Note: In a right angled triangle, the $\tan \theta $ is the equal to $\dfrac{{{\text{Perpendicular}}}}{{{\text{Base}}}}$, where perpendicular is the side opposite to the angle $\theta $, and $\sin \theta $ is the equal to $\dfrac{{{\text{Perpendicular}}}}{{{\text{Hypotenuse}}}}$, where perpendicular is the side opposite to the angle $\theta $.