Question

Same current $i = 2\;{\text{A}}$ is flowing in a wireframe figure. The frame is a combination of two equilateral triangles ACD and CDE of side $1\;{\text{m}}$. It is placed in a uniform magnetic field $B = 4\;{\text{T}}$ acting perpendicular to the plane of frame. The magnitude of the magnetic force acting on the frame is

(A) $24\;{\text{N}}$
(B) zero
(C) $16\;{\text{N}}$
(D) .z.

Answer 1

Hint: The above problem can be solved by using the concept of the magnetic field. The magnetic field is the region in which other objects experience the effect of the magnetic field intensity. The magnetic field varies according to the current and distance of the conductor.

Complete step by step answer
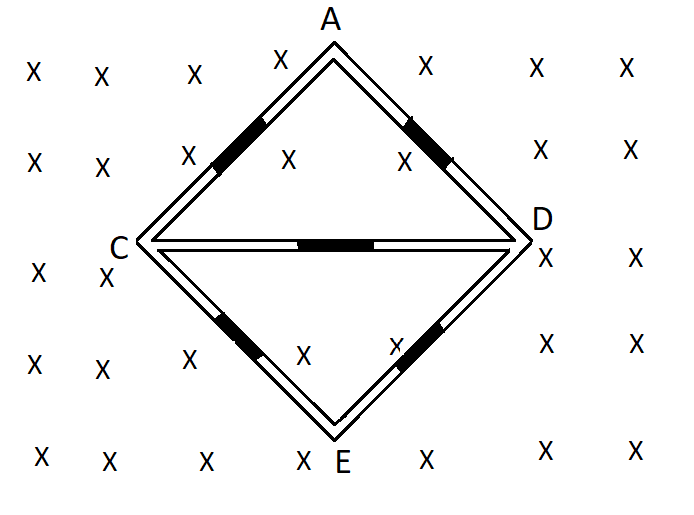
Given: The current in the wireframe is $i = 2\;{\text{A}}$, the magnetic field around the wireframe is $B = 4\;{\text{T}}$, the side of the wireframe is $l = 1\;{\text{m}}$, the angle of the wireframe with plane is $\theta = 90^\circ $.
The given wireframe is symmetrical so the forces in all the members will be equally distributed. The force in the triangle ACD, triangle CDE and the diagonal CD is the same.
The formula to calculate the net force acting on the frame is given as,
$F = 3Bil\sin \theta $
Substitute $2\;{\text{A}}$for $i$, $1\;{\text{m}}$ for $l$, $4\;{\text{T}}$ for B and $90^\circ $ for $\theta $ in the above expression to find net force acting on the frame.
$F = 3\left( {4\;{\text{T}}} \right)\left( {2\;{\text{A}}} \right)\left( {1\;{\text{m}}} \right)\left( {\sin 90^\circ } \right)$
$F = 24\;{\text{N}}$

Thus, the magnitude of the magnetic force acting on the frame is $24\;{\text{N}}$and the option (A) is the correct answer.

NoteThe magnetic force in the vector form is given as $\vec F = i\left( {\vec l \times \vec B} \right)$. Always substitute the angle of the object with the plane of the magnetic field. The force in all the members of the wireframe is uniform because of the uniform magnetic field around the wireframe.