Self Inductance of a Solenoid - Important Concept and Derivation

Self-inductance is a form of electromagnetic inductance. It can be defined as the property of a current-carrying coil that resists or opposes the change of the current flowing through it. It can also be defined as the induction of a voltage in any current-carrying wire if the current in the wire changes. This happens due to the magnetic field created by the changing current. It induces a voltage in the same circuit, so it can be said that the voltage is self-induced. 

The self-induced voltage or emf always resists the change in current. Hence, if the current is increasing, it will resist the rise of the current and similarly, when the current is decreasing, it will resist the fall of the current. This means that the direction of induced emf is opposite to the applied voltage if the current is increasing. Similarly, the direction of the induced emf would be the same as that of the applied voltage if the current is falling. 

It should be noted that this property of the coil exists only for changing currents, i.e alternating current or AC. This property does not exist for direct or steady current. The self-inductance is measured in Henry which is a SI unit having dimensions ML²T^-2I^-2.

⇒ Don't Miss Out: Get Your Free JEE Main Rank Predictor 2024 Instantly! 🚀

What is an Inductance Coil?

The inductor is a term that is used to describe a circuit that possesses the property of inductance. A coil of wire is one of the most common inductors and so in circuit diagrams, a coil of a wire is used as a symbol for an inductive component.  

The alternating current that runs through any coil creates a magnetic field in and around the coil. This is because the current increases or decreases. The magnetic field created due to the alternating current forms concentric loops around the wire and then they join together to form larger loops. When the current increases in one loop, the surrounding magnetic field expands, and it will cut across some or all the neighboring loops of wires. This induces a voltage in the loops. In this way, a voltage is induced in the coil when the current is changing. 

Given below is a diagram depicting the fields in an inductor coil. This is the principle behind the classic self-inductance of a coil experiment.

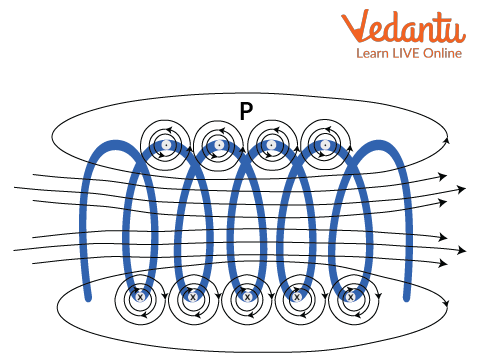
Self-inductance Derivation

It is evident from the diagram that the number of turns in the coil will affect the amount of voltage that is induced. Thus, the rate of change of magnetic flux will also affect the induced emf. This is captured precisely in Faraday’s law. Faraday’s law states that the induced emf is directly proportional to the rate of the change of magnetic flux.

Moreover, Lenz’s law states that the induced current has a direction such that its magnetic field opposes the change in the magnetic field which had previously induced the current. 

This means that the induced emf will be given as,

$V_{L}=-N\left(\dfrac{\mathrm{d} \varphi}{\mathrm{d} t}\right)$

Here, VL is the induced voltage, N is the number of turns in the coil and $\dfrac{\mathrm{d} \varphi}{\mathrm{d} t}$ is the rate of change of magnetic flux. The negative sign is due to Lenz’s law.

Since the magnetic field in a current-carrying wire is directly proportional to the current, the flux created by this particular field will also be proportional to the current. So,

$\varphi\propto I$

Here, I is the current and $\phi$ is the magnetic flux. The above expression can also be written as,

$\varphi=LI$

L is the constant of proportionality and is known as ‘self-inductance’. For a coil having N turns, the flux can be written as,

$N\varphi=LI$

Substituting this expression for flux in Faraday’s law expression will give an alternate expression for the induced emf which is,

$V_L=-L\dfrac{dI}{dt}$

To calculate the magnitude of self-inductance, the negative sign can be ignored. Hence, the formula of self-inductance will be,

$L=\dfrac{|\varphi|}{\left|\dfrac{dI}{dt}\right|}$

This is also known as the ‘coefficient of self-inductance formula’. If one asks to state the expression for the self-inductance of a coil, this is the expression. 

Self-inductance of a Solenoid

Let us take a solenoid having N turns with a length l and a cross-section area A and let current I flow through it. There will be a magnetic field at any given point in the solenoid, so let us represent it by B. The magnetic flux per turn will then be equal to the product of B and the area of each turn. 

We know that for a solenoid,

$B=\dfrac{\mu_{0} N I}{l}$

$\mu_{0}$ is the permeability of free space.

So, the magnetic flux per turn will be given by the product of B and the area of each turn which will be, $\dfrac{\mu_{0} N I}{l}A$.

The total magnetic flux will be given by the product of flux present in each turn and the number of turns. 

$\begin{align} \varphi=\dfrac{\mu_{0} N I}{l} \cdot N \\ \varphi=\dfrac{\mu_{0}{N^{2} I}}{l}\dots(1) \end{align}$ 

Now we know the relation between the self-inductance L of a coil and the flux which is given as, $\varphi=LI$.

Combining this equation and (1), we get

$L=\dfrac{\mu N^{2} A}{l}$

This is the self-inductance of a solenoid.

Mechanical Equivalent of Self-inductance

When there's a change in electric current in a circuit, a force called self-induced electromotive force (emf) acts against this change. 

When you have a circuit with self-inductance, the self-induced voltage, or back voltage, tries to resist any changes in the current. Think of self-inductance like inertia in mechanics – it's the electrical version of mass. So, when you're setting up the current in the circuit, you have to do some work against this back voltage (VL). The energy used in this process is stored as magnetic potential energy and can be calculated using the formula:

$W = \dfrac{1}{2} LI^2$

This formula tells you how much energy it takes to create a current (I) in the inductor. It's similar to the formula for the kinetic energy of a moving object $(mv^2/2)$, where L (inductance) plays the role of mass, opposing both the increase and decrease of current in the circuit.

Mutual Inductance

This occurs when one coil in a circuit resists changes in its current because of the presence of another coil. If there's a material with relative permeability μr around, the mutual inductance can be calculated using the formula $M = \mu r \mu_0 n_1n_2 \pi {r^{2}}_1$ l. Here, n1 and n2 are the numbers of turns of the two coils per unit length, and r1 is the radius of the inner coil, while l is the length of the coil.

Difference between Mutual Inductance and Self Inductance

Uses of Self-Inductance

1. Storing Energy: Inductors are like energy storage units that hold electrical energy in a magnetic field.

2. In Different Devices: They're used in things like tuning circuits, sensors, and motors to make them work.

3. Transforming Energy: Inductors are also part of transformers, which change electrical energy from one form to another.

4. Filtering and Choking: They help in filters and chokes to control the flow of electrical signals.

Limitations of Inductors

• Current Limits: Inductors can only handle a certain amount of electric flow before they get too hot.

• Manufacturing Challenges: Making perfect inductors is tough because of extra effects and size issues, unlike capacitors which are easier to make.

• Magnetic Interference: Inductors can mess with nearby parts of a circuit because of their magnetic fields.

Solved Problems

1. How is the self-inductance of an inductor given?

a) L = N⁄S

b) L = 1/S

c) L = NΦ/I,

d) L = N^2

Answer: c)

To find the self-inductance (L) of an inductor, use the formula:

L = NΦ/I,

Where,

L is the self-inductance of the inductor (measured in henries, H)

N is the number of turns of wire in the coil

Φ is the magnetic flux through the coil (measured in webers, Wb)

I is the current flowing through the coil (measured in amperes, A)

Explanation:

Self-inductance: It's a property of a coil that opposes changes in the current flowing through it. It arises due to the coil's ability to induce an electromotive force (EMF) within itself when the current through it changes.

Formula: The formula L = NΦ/I captures this relationship. It states that the self-inductance of a coil is directly proportional to the number of turns of wire, the magnetic flux through the coil, and inversely proportional to the current flowing through it.

Interpretation:

• More turns of wire (N) increase the magnetic field and flux, leading to greater self-inductance.

• A stronger magnetic flux (Φ) increases the induced EMF, resulting in higher self-inductance.

• A higher current (I) generates a stronger magnetic field, but it also reduces the relative impact of the induced EMF, leading to lower self-inductance.

2. What factor affects the self-inductance of an inductor?

a) Permeability

b) Permittivity

c) Planck’s constant

d) Rydberg constant

Answer: a)

Self-inductance depends on several factors, including:

• Number of turns (N): The more turns the coil has, the stronger the magnetic field it produces and the greater the self-inductance.

• Cross-sectional area (A): A larger cross-sectional area allows for more magnetic flux to pass through the coil, increasing self-inductance.

• Length of the coil (l): A longer coil has a longer path for the magnetic flux to travel, leading to higher self-inductance.

• Permeability of the core material (μ): The permeability of the material inside the coil determines how easily magnetic fields can be induced. Higher permeability materials, like iron, increase self-inductance.

• Shape of the coil: While not as significant as the other factors, the shape of the coil can also affect self-inductance. For example, a long, thin solenoid will have higher self-inductance than a short, thick coil.

Note: Self-inductance depends on the number of turns, cross-sectional area, length, permeability of the core material, and to a lesser extent, the shape of the coil.

3. What is the typical phase angle for an RL circuit?

Typical Phase Angle for an RL Circuit:

• General Range: The phase angle in an RL circuit typically lies between -90° and 0°.

• Negative Value: It's always negative, indicating that the current lags behind the voltage.

• Determining Factors:

• Values of resistance (R) and inductance (L): Larger L leads to greater lag and a phase angle closer to -90°.

• Frequency (f) of the applied AC voltage: Higher frequencies increase the phase difference.

4. What is φ in terms of voltage?

a) $\phi = \cos^{-1} V/VR$

b) $\phi = \cos^{-1} V \times VR$

c) $\phi = \cos^{-1} VR/V$

d) $\phi = \tan^{-1} V/VR$

Answer: c)

• Φ (phi) represents the phase angle between voltage and current in an AC circuit.

• VR is the real voltage across the load, while V is the total voltage applied to the circuit.

• The cosine of the phase angle (cos(Φ)) can be calculated using the ratio of real voltage to total voltage: cos(Φ) = VR / V.

Therefore, to find the phase angle Φ itself, we need to take the arccosine (cos^-1) of the above ratio: Φ = $cos^-1$(VR / V).

5. What does sin ϕ represent in the impedance triangle?

a) XL/R

b) XL/Z

c) R/Z

d) Z/R

Answer: b)

• Impedance (Z): It's the total opposition to current flow in an AC circuit, combining both resistance (R) and reactance (X).

• Resistance (R): It's the opposition to current flow due to energy dissipation as heat.

• Reactance (X): It's the opposition to current flow due to energy storage in inductors (XL) or capacitors (XC).

Conclusion

Self-inductance is a kind of electromagnetic induction and it is a property by which a current-carrying conductor opposes any change in the current that flows through it. This property gives rise to an induced emf which opposes the change in the current through the conductor. A coil of wire is usually used as an inductor and so the symbol of inductive components in a circuit diagram is a coil of wire. By using Faraday’s law and Lenz’s law, the self-inductance of a coil is calculated to be, $L=\dfrac{|\varphi|}{\left|\dfrac{\mathrm{d} I }{ \mathrm{d} t}\right|}$. 

Thus, the self-inductance of a solenoid is, $L=\dfrac{\mu_{0} N^{2} A}{l}$.