




Newton and Dyne - Definition With Solved Examples
When we talk about the units of measurement for any physical, electrical, or any other quantity, we can differentiate between the metric systems used based on the SI or International System of Units) and CGS (Centimetre-Gram-Second) units. The SI unit is known to be the easiest one to use and is used for all scientific work. We have seven fundamental quantities that are the basis for SI units. These include time, length, mass, temperature, amount of substance, electric current, and luminous intensity.
For example, Force. A force is nothing but a push or pull on your body to alter its state. The standard unit for force in the SI metric system is Newton (N), where in the CGS metric system, the unit is dyne. We can measure in Newton or dynes, but the relationship between the two is critical and must be known. Additionally, Newton and dyne are not the only units of force. In this article, we will understand the relationship between the two units NEwton and Dyne in detail.
What is Force?
Force is defined as the pull or push that is applied to an object to change its state. The force applied can change the motion of an object. A force can be applied to either stop an object’s motion or change the motion of an object.
Force is the product of mass (m) and acceleration (a). Mathematically, force is expressed as $F=m\times a$, where
F is the force acting on an object of mass m, and m is the mass of an object, whereas a is the acceleration.
Refer to the image given below:

Pictorial representation of Force
Force is a vector quantity. Thus it has a magnitude and direction. Newton and dyne are not the only units of force. Besides Newton and Dyne, there are also some other units of force. Some of these include: Poundal and pound, gram force, ton-force, ounce force, and so on.
What is Newton?
The unit of force in the SI metric system is called Newton. It is denoted by the letter N. So how do we define 1 Newton?
1 Newton is defined as the force required to move an object with a mass of 1 kilogram (kg) and moving with an acceleration of 1 $\dfrac{metre}{second^2}$ ( 1 $\dfrac{m}{s^2}$) in the direction of force (F).
$1 N=1 \mathrm{~kg} \cdot 1 \dfrac{\mathrm{m}}{\mathrm{s}^{2}}=1 \cdot \dfrac{\mathrm{kg} \cdot \mathrm{m}}{\mathrm{s}^{2}}$
The image below shows a mass of 1kg accelerating with 1 $\dfrac{m}{s^2}$ when 1N force is applied to it.
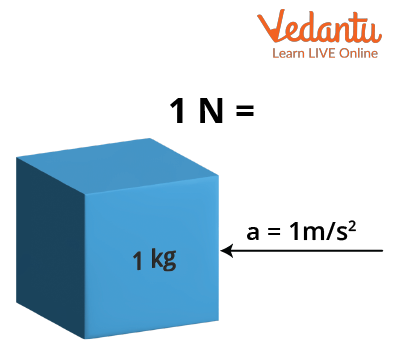
Pictorial representation of 1 newton
What is Dyne?
In CGS (or centimetre-gram-second), the length is measured in centimetres, mass in grams, and time in seconds. The CGS system is another variant of the metric system. Similarly, the unit of Force is dyne in the centimetre-gram-second or CGS metric system. So how do we define 1 dyne.
1 dyne is the force applied to a mass of 1 gram, increasing its acceleration by 1 cm/second 2 in the direction of the force applied.
Mathematically, 1 dyne can be denoted as $1 \text { dyne }=1 \mathrm{~g} \cdot 1 \dfrac{\mathrm{cm}}{\mathrm{s}^{2}}=1 \dfrac{\mathrm{g} \cdot \mathrm{cm}}{\mathrm{s}^{2}}$
What are Dyne and Newton?
Dyne: Dyne is the unit of force in the CGS unit system. 1 dyne is equal to 1 g.cm/s2. When the object of one gram mass is accelerated at the rate of one centimetre per second squared, then the force applied on the object is one dyne.
Newton: Newton is the unit of force in the SI unit system. 1 newton is equal to 1kg.m/s2. When the object of one-kilogram mass is accelerated at the rate of one metre per second squared, then the applied force is one newton.
Relation Between Newton and Dyne
Now that we have discussed Newton and dyne, let us understand the relation between the two. So how can we convert 1 newton into dyne. Both units are in different metric systems thus, we can use conversion to depict one in terms of another.
Now we know that
$1 \mathrm{~N}=1 \mathrm{~kg} \cdot 1 \dfrac{\mathrm{m}}{\mathrm{s}^{2}}=1 \cdot \dfrac{\mathrm{kg} \cdot \mathrm{m}}{\mathrm{s}^{2}}$
$1\cdot\dfrac{\mathrm{kg} \cdot \mathrm{m}}{\mathrm{s}^{2}}$
$\begin{align} &=1000 g \times 100 \dfrac{\mathrm{cm}}{\mathrm{s}^{2}} \\ &=1000 \times 100 \times \dfrac{g \cdot c m}{s^{2}} \\ &=10^{5} \times \dfrac{g \cdot c m}{s^{2}} \\ &=10^{5} \text { Dyne }\left(\because 1 \text { Dyne }=\dfrac{g \cdot c m}{s^{2}}\right) \end{align}$
Thus, 1N=105 dyne
We have the relationship of Newton in terms of dyne, therefore now we can easily
convert Dyne into Newton or vice versa.
$\therefore 1 \text { dyne }=0.00001 \text { Newton }(N)={10^{-5}}\,N$
Or
$ \text { Newton }(N)={10^{5}} \text { dyne }$
Conversion of a Newton and Dyne
Now, we know that both the units of forces are used according to the requirement. In order to solve the problems, we must understand the interaction between Newton and Dyne.
Both are recognised as units of force in their respective systems. So, let's begin with a definition: The force required to move an object with a mass of one kilogramme at an acceleration of one m/s2 is one newton. Mathematical notation for:
\[1N = 1Kg \times 1\dfrac{m}{{{s^2}}}\]
\[1N = 1000g \times 100\dfrac{{cm}}{{{s^2}}}\]
\[\begin{array}{l}1N = 1000 \times 100 \times g \times \dfrac{{cm}}{{{s^2}}}\\\end{array}\]
\[1N = {10^5}g\dfrac{{cm}}{{{s^2}}}\]
Therefore, the interrelation between Newton and Dyne is thus as follows:
1 Newton = 105 Dyne.
Using this relation, we can easily convert the value of force in CGS from SI or vice versa.
Solved Examples on Newton and Dyne
Example 1: A constant force is acting on a body of mass 6 kgs which changes its speed from 10 ms-1 to 50 ms-1 in 30 seconds. Calculate the magnitude of force being applied on the body in dyne?
Solution:
Given:
The mass of the body=m=6kg
Acceleration $= \dfrac{\text{Change in velocity}}{\text{Time}}$ or $\dfrac{\Delta v}{t}$
$\begin{align} &=\dfrac{50-10}{30} \\ &=1.33 \mathrm{~ms}^{-2} \end{align}$
Force acting on the body will be $F=m\times a$
$\begin{align} &=6 \times 1.33 \\ &=7.98 \mathrm{~N} \end{align}$
As we know that the SI unit of Force is Newton and the CGS unit of Force is dyne and as derived from the earlier sections, we have 1N=105 dyne
$\therefore 7.98 \mathrm{~N}=7,98,000 \text { dyne }\left(7.98 \times 10^{5}\right)$
Example 2. How many dynes are there in one gram? Also calculate the dynes for 10 grams of mass?
Solution: As one dyne equals 0.00102 grams. Therefore 1 gram will have 980.392 dynes.
$\begin{align} &1 \text { gram }=\dfrac{1}{0.00102} \\ &1 \text { gram }=980.392 \text { dynes } \end{align}$
From the above relation, we have 1 gram =980.392 dynes. Thus, for 10 grams we will have 9803.92 dynes which is equal to 0.098 Newtons (N).
Interesting Facts
The amount needed to accelerate 1 kilogramme of mass at a velocity of 1 metre per second squared was defined as the unit of force for the MKS system of units in Conférence Générale des Poids et Mesures (CGPM) Resolution 2 of 1946.
The 9th CGPM Resolution 7 in 1948 gave this force the moniker "newton." The current SI system of units is based on the MKS system, which was eventually adopted.
The Newton was adopted as the default unit of force in the International System of Units. The Newton is a tribute to Isaac Newton. Although its symbol starts with an uppercase letter (N), its full name is written using the same capitalization rules as a common word.
For instance, the word "newton" is capitalised at the beginning of a sentence.
Conclusion
The article presents a differentiation between SI and CGS metric systems. We often use the SI metric system as it is easier. A thorough understanding of the SI and CGS unit of force i,e Newton and dyne respectively are discussed. We know that conversions from one metric system to another come in handy when solving complex numerical problems. We have discussed the two variants of the metric system thoroughly in the above article.
Newton and dyne are discussed, and a relationship between the two has also been derived. Solved examples have also been added for a thorough understanding of the concept. It is a small topic with respect to the JEE main exam. However, as it is a part of gravitation, these small concepts must be thoroughly well-read by the learners.
FAQs on Relation Between Newton and Dyne for JEE
1. Where is dyne/cm and Dyne/cm2 used?
Dyne is a CGS unit of force. Dyne is the force applied to a body of 1 mass kg to increase its acceleration by 1ms-2. The dyne per centimetre or $\dfrac{Dyne}{cm}$ is a unit that is traditionally used to measure surface tension. For example, the surface tension for distilled water is 72 dynes/cm or 72 $\times$ 10-3 $\dfrac{N}{m}$. This unit of surface tension is measured at 25 degree Celsius or 77 degree Fahrenheit. $\dfrac{Dyne}{cm^2}$, on the other hand, is used to measure pressure associated with shear stress. 1 $\dfrac{Dyne}{cm}$ also equals 0.1 Pascal (Pa).
2. What is the difference between force and weight?
Weight is the force acting under the gravitational pull, whereas forces are the additional push or pull other than the gravity. Both are forces and are measured in Newton (N).
When a force is applied on a body of mass m, it causes the object to accelerate. Thus, we have the force equation as F = ma. If you accelerate a 4 kg mass at an acceleration of 4 $\dfrac{m}{s^2}$, then that will require a force of 16 N. For a free falling object, it is under the influence of gravity, so it accelerates at 9.81 $\dfrac{m}{s^2}$. This is because gravity applies a force of 9.81 N per kg.





























