




What is Wave?
In a medium, a disturbance propagating through it without any significant change in its form is known as a wave. A very simple example of a wave is ripples in water when you throw a stone in a calm lake. The deformation in the medium can be many things, like elastic deformation, or a varying pressure gradient, potential, and even temperature with distance and time. Based on their propagation through the medium, waves can be either transverse or longitudinal.
Transverse waves are waves in which the individual particle motion is perpendicular to the direction of propagation of the wave through the medium. In Longitudinal waves, the particle motion is in the same direction as the wave propagation. The continuous compression and rarefaction effect causes the wave to move and if the medium had zero resistance, then the wave would have propagated forever. But due to opposition like air resistance, the wave eventually dies out. Sound waves need a material medium for propagation while electromagnetic waves can travel through a vacuum.
Properties of Wave
In the language of Physics, waves can be mathematically, structurally, and theoretically explained by the following properties or features: -
Wavelength:- A wave structurally is made out of a crest and a trough in accordance with a reference line as shown in the figure below. Wavelength is the distance between two adjacent points, one from where the crest starts forming and the second one where the trough part ends. So, the wavelength is the length between one crest and one trough and is measured in metres.
Amplitude: - Amplitude is the maximum height of a crest. It is the maximum perpendicular displacement from the mean reference line to the top of the curve. The crest is the highest point of a wave and the trough is the lowest point but the magnitude of the displacement from the mean line in both gives the amplitude in metres.
Frequency: - The frequency of a wave is the number of vibrations, i.e., the number of crests and troughs passing through a reference point in a chosen amount of time. It has a unit of Hertz.
Time Period: - It is proportional to the reciprocal of frequency mathematically. A full-wave (one crest + one trough) propagating through a fixed point takes a certain amount of time which is known as the Time Period and is measured in seconds.
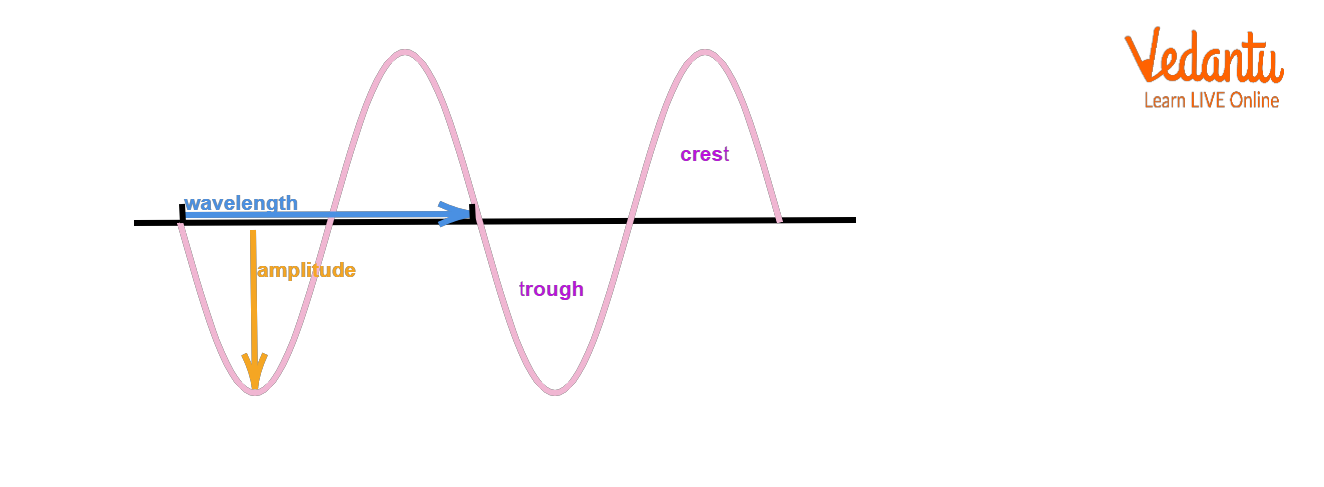
Wave Motion and Its Parameters
What is Simple Harmonic Motion?
SHM is a topic of massive interest in Physics and is immensely important while discussing waves and oscillations. The oscillation motion is to and for motion about a central point known as the mean position. The displacement of the particle which is oscillating on either side of the central point is the same. The best way to understand SHM is through a pendulum or a spring motion.
What is Progressive Harmonic Wave?
Let us understand the mechanism behind the ripple waves in the water when a stone is dropped in a calm lake. The stone that has been thrown has had some kinetic energy. This stone when it hits the water surface imparts part of its energy to the particles in water it is directly contacting. The same energy is being transferred to the other adjacent particles and it physically manifests as ripples or waves that we see which are nothing but excited water particles in motion. The vibrations travel from point to point through energy transfer. Now that we have a basic idea of how waves propagate, we can define a harmonic progressive wave.
Progressive harmonic waves are waves that progress continuously in a fixed direction with no change in their physical form. The wave performs simple harmonic motion about a fixed position without any change in amplitude, frequency, and time period while passing through it.
Equation of Progressive Harmonic Wave and Its Derivation
Let us derive the equation of a simple progressive harmonic wave in
Consider the figure below: -
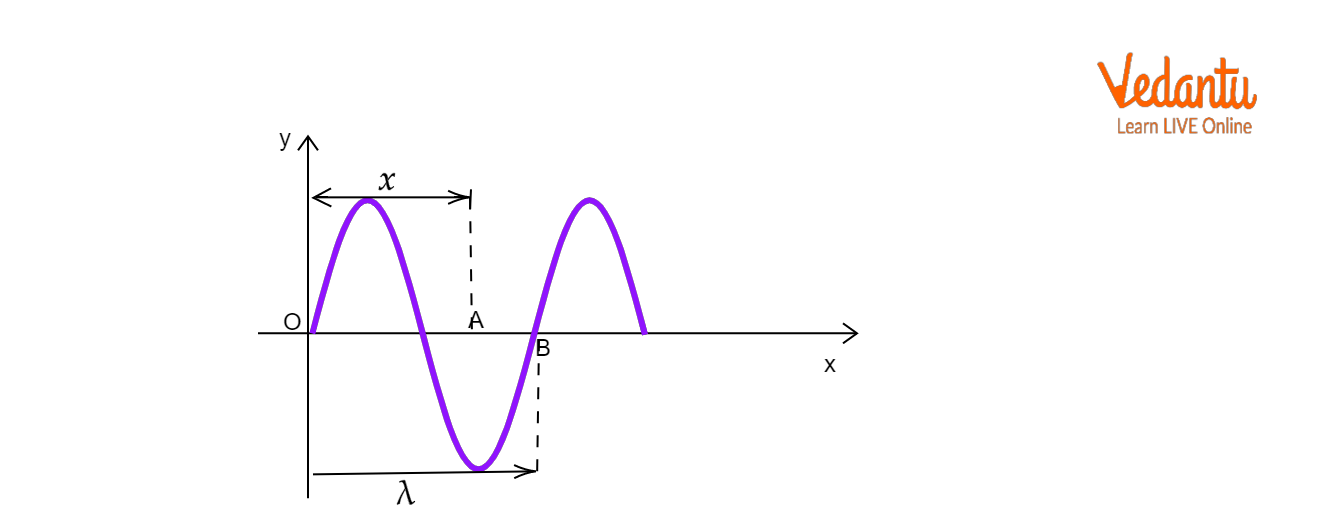
The Propagation of Progressive Harmonic Wave along Positive X- Direction.
The progressive harmonic wave is travelling along the positive
Here,
Now, consider the particle
Now, we already know that the path difference
Therefore,
Substituting the value of
This is the equation of the progressive harmonic wave in positive X-direction.
Exploring other forms now:
Since
Then, the equation of wave can be expressed as:
Since
Then the equation of wave can be expressed as:
The Main Characteristics of the Progressive Harmonic Wave
All the particles in this wave exhibit simple harmonic motion.
The amplitude of the particles does not change with time.
The frequency of the particles does not change with time.
There is transmittance of energy through the medium.
The Speed of a Progressive Harmonic Wave
The speed of a progressive harmonic wave can be determined from its displacement equation as follows: -
Now, the speed is given by distance divided by time. Here, the distance a wave covers is its wavelength. So,
Conclusions
A wave is a disturbance propagating through a medium with no significant change in its form. Waves are mathematically characterised by wavelength, frequency, amplitude, and time period. A progressive harmonic wave is a wave propagating in a fixed direction and exhibits simple harmonic motion. The equation of a simple progressive harmonic wave is






FAQs on Plane Progressive Harmonic Wave – JEE Important Topic
1. Explain the superposition of waves.
According to the principle of superposition, whenever two or more waves cross paths with each other at a certain point in space, then the total displacement at that point in space is equal to the summation of the displacements happening due to individual waves. The sign of the individual wave displacements may be negative or positive and thus has to be taken care of while performing the summation. If zero displacement happens, then the waves have collided destructively and if some finite value is obtained, then the waves have collided constructively.
2. What is simple harmonic motion? Give examples.
Simple harmonic motion is periodic in nature. The nature of motion is such that it is always about a fixed point and the acceleration is directed towards the fixed point. Numerically, the acceleration is proportional to the displacement of the oscillating point from that fixed point. Pendulum motion is a great example of simple harmonic motion. Also, the motion of a spring when a load is suspended is also an example.
















