




The Story of Motion in One Dimension
Motion in one dimension means that an object is travelling on a line or in a specific direction. While we are residing in a three-dimensional world filled with a lot of complexity, there is a need for knowledge to separate the complexity into simpler terms. This is why we need to learn about the simple one-dimensional motion which is basic for any other motions that exist. A car driving on a straight road in a particular direction is a one-dimensional motion example.
Before we dive into this topic, you might have already heard about these words in physics somewhere at some point in your life which are Displacement, Velocity, acceleration and time. These look simple but these are the foundation for numerous and infinite laws, formulae and properties of many quantities in physics. Time is a scalar quantity as it has only magnitude whereas velocity, displacement and acceleration have both magnitude and direction and they are addressed as vectors.
Let us go deeper into the concept of one-dimensional motion.
Displacement, Velocity and Acceleration
We will take a closer look at all three quantities displacement, velocity and acceleration separately, let us first start with displacement.
Displacement:
Displacement is a vector quantity and can be represented by $\overrightarrow{r}$. It can be described as the difference between the position of an object when it is travelling in a particular direction from one place to another in a specific amount of time. SI unit of displacement is metre(m). Let us assume that the two displacement vectors r1 and r2 as shown in the below image, the object is moving from the position 1 to the position 2 and here the distance from a reference point or the origin to the point 1 is taken as r1 and similarly the distance from the origin to the point 2 is taken as r2.
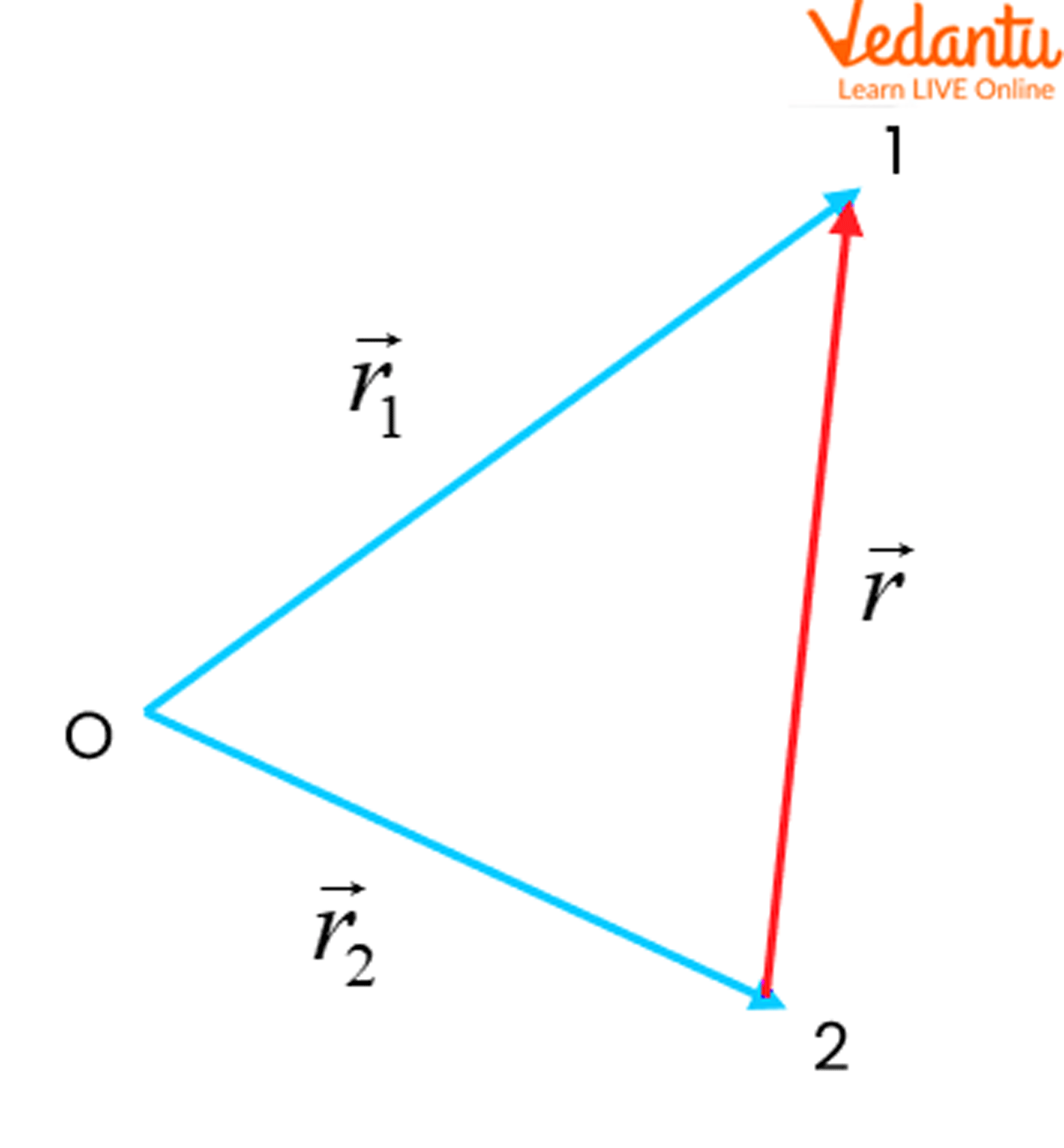
Here, the total displacement of an object $\vec r$ can be described as the subtraction of both vectors and this is represented as the change in the value of r, i.e;, $\Delta \vec r$
$\Delta \vec r=\vec r_2-\vec r_1$
Note: In uniform motion, the displacement would be a straight line plotted on a graph in between two points. Whilst in non-uniform motion, the displacement vectors don’t change constantly according to the time, so the graph would be non-uniform.
Velocity:
This is also a vector quantity that is having both magnitude and direction. Velocity is defined as the rate of change of displacement with time. The SI unit of speed or the velocity is $\dfrac{metre}{sec}~\left(\dfrac{m}{s}\right)$.
Let’s say the position of an object at time t1 is r1 while the position of an object at t2 is r2, then the velocity according to the definition can be written as below,
$v=\dfrac{r_{2}-r_{1}}{t_{2}-t_{1}}$
The numerator in this equation gives us the change in displacement and the denominator gives us the change in time.
In uniform motion, the velocity will be constant for the entire time interval. But, in non-uniform motion where the velocity would not remain constant, we have to find the average velocity over a given time interval instantaneously.
This is mathematically represented as,
$<v>=\dfrac{\Delta \vec{r}}{\Delta t}$
Where the numerator is the change in displacement to that particular instant and the denominator is the time interval of that instant.
Acceleration:
Acceleration is also a vector quantity and it is defined as the rate of change in velocity with respect to time. As velocity is changing with time, then it is a non-uniform motion. Therefore, the acceleration is represented in the equation below.
$\text { Acceleration }=\dfrac{\text { Change in velocity }}{\text { Time interval }}$
I.e,$\vec{a}=\dfrac{\Delta\vec{v}}{\Delta}=\dfrac{\vec{v}_{2}-\vec{v}_{1}}{t_{2}-t_{1}}$
This is the formula of acceleration where the numerator gives the change in velocity and the denominator is the change in time. SI unit of acceleration is m/s2.
Equations of Motion
To understand these equations of motion adequately, let us assume the initial velocity of a vehicle as u, the final velocity as v, the change in displacement as s and the acceleration as a which is a constant value for a uniform motion.
The First Equation of Motion:
As we know, the change in velocity is v - u and therefore the equation of acceleration is
$a=\dfrac{v-u}{t}$
at = v - u
Therefore we can write v as, v = u + at
This is the first equation of motion.
The Second Equation of Motion:
The change in displacement is s and now the average velocity can be written as
$<v>=\dfrac{s}{t}$
$S = <v>.t$ - - - - (a)
And here average velocity between the initial point of time say t0 and at a later point
of time say t, can be written as
$\langle v\rangle=\dfrac{v+u}{2}$
Submitting the value of v that we obtained in the first equation of motion, now we get,
$\begin{align} &<v>=\dfrac{u+a t+u}{2} \\ &<v>=\dfrac{2 u+a t}{2} \end{align}$
Therefore, the average velocity is given as
$<v>=u+\dfrac{1}{2}at$ - - - - - (b)
Substituting the value of average velocity from eq(b) in the eq(a)
$S=\left(u+\dfrac{1}{2}at\right)(t)$
Therefore here we go,
$S=u+\dfrac{1}{2}at^2$
This is the second equation of motion.
Third Equation of Motion:
We all know that distance travelled by a vehicle is calculated by multiplying the average velocity of the vehicle by time.
$v = u + at$
Therefore,$t=\dfrac{v-u}{a}$
Average velocity, $<v>=\dfrac{v+u}{2}$
Therefore putting these values in equation(a), we get
$s=\dfrac{v-u}{a}\cdot \dfrac{v+u}{2}$
I.e, 2as = v2 - u2
This is the third equation of motion.
Motion in one dimension formulas is discussed with proof and a proper explanation. Let us solve a problem using the equations of motion.
Example: A car is travelling with an initial velocity of 25$\dfrac{m}{s}$ and with an acceleration of 4$\dfrac{m}{s^2}$. Find the distance travelled by car within 20 seconds in a uniform motion.
Solution:
Here, Given that, initial velocity, u = 25$\dfrac{m}{s}$ and the acceleration, a = 4$\dfrac{m}{s^2}$. The second equation of motion can be used here to find the displacement of the car within t = 20 sec.
According to second equation of motion
$S=u t+\dfrac{1}{2} a t^{2}$
Substituting given values in above equation we get,
S = 25.20 + (0.5)(4)(20)2
S = 1100 m
Thus, the car will travel 1100 m in t = 5 sec.
Conclusion
The motion in one dimension brings out basic physical quantities like distance and time. The dimensions of many other physical quantities are derived from these fundamental quantities. For example, the dimension of the area is m2 where m is the basic quantity used. Not only the dimension of the area but there is also the infinite number of physical quantities that depend on these two quantities. We described what is one-dimensional motion, the dimension of speed, the dimension of velocity, the dimension of distance, the dimension of displacement and the one-dimensional motion examples in this article. And we discussed displacement, velocity and acceleration including the equations of motion in this article.
FAQs on Motion in One Dimension - Laws and Formula for JEE
1. What is the difference between speed and velocity?
As far as these two are concerned, we might think they are the same terms with the same properties. But that is not true. Speed is a scalar quantity and it has no direction. Velocity is a vector quantity and it has a direction. As velocity is a vector quantity, it can be negative or positive or even zero. But speed can only be positive and it can never be negative or become zero. Any vehicle travelling on a road can have different velocities but it can have only a constant speed.
2. What is the weightage of this topic in JEE?
The concept of Equations of motion and kinematics has a weightage of 7% in JEE and is widely related to some topics from the mechanics section in physics. About 2 to 3 questions can be asked based on the equations of motion that we have learnt in this article. Finding the velocity, displacement and acceleration of a vehicle, and finding the relative velocity of a vehicle concerning ground or concerning any other vehicle are the prominent questions that are asked from these topics in JEE.
3. Distinguish between displacement and distance?
Displacement is a vector quantity as it has both magnitude and direction. But distance is a scalar quantity as it does not possess any direction. Distance is the complete length between any two points but displacement is the shortest distance between those two points. We multiply time with speed to calculate the distance whereas we multiply time with velocity to estimate the displacement. As we can see, distance depends on the path followed by the object whereas the displacement only needs the initial and the final positions of the object.



























