Lateral Displacement of Light - Important Concepts for JEE

Light rays are waves that travel through a given non-opaque medium at a fixed speed. On encountering a press of a different refractive index, these rays tend to travel at a different rate than the original medium. On exiting the second medium, these rays eject themselves from the medium parallelly to the original direction, but with a slight displacement from the original ray. Therefore, to define lateral displacement of light, this behaviour of movement of light through separated refractive indices is termed as lateral displacement.

Refraction of Light

This phenomenon of light is based on the property of the wavefront. When a wave passes from one medium to another (of varying density), obliquely, various points of the wavefront move at different speeds in the second medium. 

Refraction is based on the inherent property of the medium, where the density of the material constitutes a parameter called the refractive index. For example, the refractive index of a vacuum is 1. The refractive index does not have a unit because it is related to the ratio of different media. 

Imagine a wave moving from one medium to another where it moves slower than in the first medium. When the wave strikes the glass slab at an angle from the normal, which is not 0, one side of the wavefront will contact the interface earlier than the other side. This discrepancy makes the wavefront bend in such a way that the entire wavefront enters the medium in unison. This phenomenon can be explained on the basis of the Huygens principle (which is outside the scope of this topic). This principle probes into the wave nature aspect of electromagnetic waves, i.e., light.  

Snell’s Law: This law states that for a given pair of media, the angles of incidence and refraction are proportional to their phase velocities. In turn, these phase velocities correlate to the refractive indices of the respective media.

Lateral Displacement of Light in Glass Slab

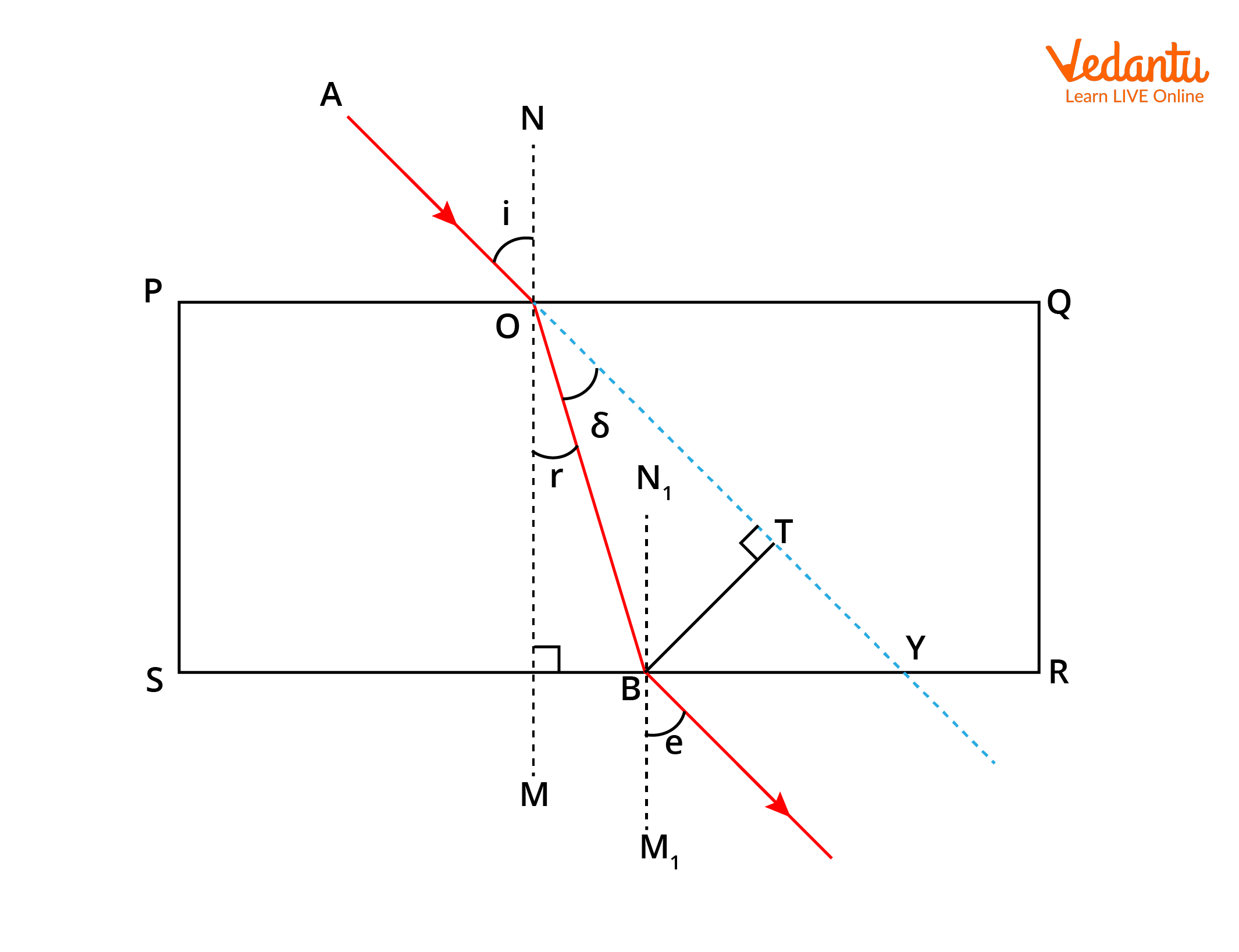
Lateral displacement of light in a glass slab

Lateral Displacement of Light Formula

To derive the formula for displacement, we use the lateral displacement of light through a glass slab. Our first task is to attempt to derive the displacement using known parameters of the system. The light ray strikes the second medium at an angle of ‘i ’ to normal. After refraction, the light ray travels at an angle of ‘r ’ to the normal.

Now the emergent ray, after travelling through the second medium, travels at an angle of ‘e.’ Originally, the light was travelling through a medium of refractive index ‘$\mu_1$’ and passing through the second medium of refractive index ‘$\mu_2$ ’. Let the thickness of the second medium be PS= t.

Now, using the Snell’s law of refraction, i.e.,

Also,

$ \begin{align} &\mu_1 \sin (i)=\mu_2 \sin (r) \\ &\mu_2 \sin (r)=\mu_1 \sin (e) \end{align}$

This tells us that the angle of incidence is the same as the angle of emergence, i.e., 

i = e.

Let's put a perpendicular from B to the original light path at a point T to create a right-angled triangle. In that triangle, 

$ \sin (i-r)=\dfrac{B T}{O B}$                       (1) 

Also, if we extend the normal NM to SR, we get,

$\sin (90-r)=\dfrac{P S}{O B}$  

which is the same as,

$\cos (r)=\dfrac{t}{O B}$       OR     $O B=t \sec (r)$

Here, as you can see, BT is the lateral displacement required. 

Using the above equation in (1), we get the lateral displacement BT as

$B T=t \cdot \sin (i-r) \cdot \sec (r)$

We obtained the formula for the lateral shift in light using the parameters provided originally. Using this formula, we can obtain the respective relations with the lateral shift.

Factors Affecting Lateral Displacement of Light

1. The thickness of the slab (t): The lateral shift is directly proportional to the thickness of the slab.

2. The angle of incidence (i): The lateral shift is directly proportional to the angle of incidence.

3. Refractive index of the glass slab ($\mu_2$): As the refractive index increases, the displacement also increases. 

Conclusion

Lateral displacement of light probes into a property of light as a wave. This is crucial in identifying the duality of light as a wave and a particle. The lateral displacement of light happens due to the refraction of light through the surface of the slab.  Using a few initial parameters, we can quickly obtain the lateral shift in the light path. The factors that affect the lateral displacement of light include thickness of the slab, the angle of incidence and the refractive index of the slab being used.