Kinetic Energy of Rotating Body and its Derivation | JEE

An Introduction to Kinetic Energy of a Rotating Body 

Kinetic energy is usually possessed by an object or a body that is in motion. The motion of the body could be rotational or linear. The energy of a rotating body is termed as rotational kinetic energy. The difference between linear kinetic energy and rotational kinetic energy is that the former is revolving with an angular velocity. The kinetic energy of the rotating body can also be expressed in terms of mass of inertia. 

Other factors that the rotational kinetic energy depends on are its angular velocity, the distance of point mass from the axis of rotation, its mass and translational velocity. The relation between translational and angular velocity is given as $v=\omega ~ r$. Let us further explore the concept of rotational kinetic energy. 

Where Does Rotational Kinetic Energy Come from? 

As the name suggests, rotational kinetic energy is the energy possessed by an object that is rotating or revolving. When a body is rotating, the linear velocity of every point on it is different. As linear velocity is a vector quantity therefore always is tangential to circular motion which is why it is changing direction. This leads to addition of a new quantity termed as the angular velocity. Thus, the linear or translational velocity can be related to the angular velocity as $v=\omega ~ r$. Thus, the rotational kinetic energy comes from the translational or linear velocity. 

Kinetic Energy of Rotating Body Formula

The kinetic energy of a rotating body is given as ,

$K_{r o t}=\dfrac{1}{2} I \omega^{2}$

Where I is the moment of inertia and $\omega$ is the angular velocity. 

For a translational motion the kinetic energy is given as,

$K_{\text {linear }}=\dfrac{1}{2} m v^{2}$

Where m is the mass of a body and v is the linear velocity. 

If we are to relate the kinetic energy of rotating body formula with that of kinetic energy of linear motion I and $\omega$ in the former is equivalent to m and v for the latter. The SI unit of rotational kinetic energy is Joules or J. This can be disintegrated as moment of inertia is measured in kgm² whereas angular velocity is measured in radians/second. Thus, the SI unit of kinetic energy of rotation is $\dfrac{kgm^2}{s^2}$ which is equal to Joules. In MKS system this can be denoted as $\left[{M}^1~{L}^2 ~ {T}^{-2}\right]$.

Derive an Expression for Kinetic Energy of Rotating Body

Let us derive an expression for kinetic energy of a rotating body. Assume a rotating body as shown below. 

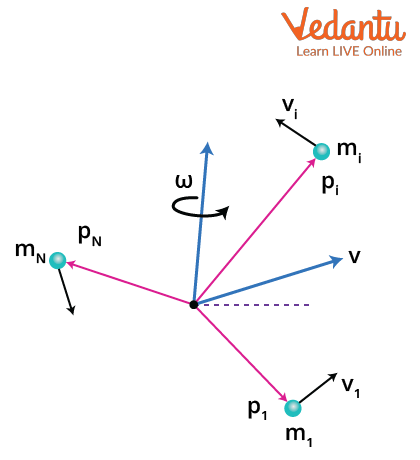
Body in Rotation

Suppose particle 1 rotates in a circle with radius r₁. Its linear velocity and kinetic energy thus can be given as will v₁=wr₁ and 

$\begin{align} &\dfrac{1}{2} m v^{2} \\ \\ &\Rightarrow \dfrac{1}{2} m_{1}\left(w r_{1}\right)^{2} \\ \\ &\Rightarrow \dfrac{1}{2} m_{1} \omega^{2} r_{1}^{2} \end{align}$

Similarly, the kinetic energy for particle 2 or (P₂) will be 

$\Rightarrow \dfrac{1}{2} m_{2} \omega^{2} r_{2}^{2}$

The sum of all the kinetic energy will be given as

$\begin{align} &K_{r o t}=\dfrac{1}{2} m_{1} \omega^{2} r_{1}^{2}+\dfrac{1}{2} m_{2} \omega^{2} r_{2}^{2} \\ \\ &\Rightarrow \dfrac{1}{2} \omega^{2}\left(m_{1} r_{1}^{2}+m_{2} r_{2}^{2}\right) \\ \\ &\Rightarrow \dfrac{1}{2} \sum{ }_{1}^{N}\left(m r^{2}\right) \omega^{2} \end{align}$

The $\sum_{1}^{N}\left(m r^{2}\right)$ is the moment of inertia I.

Thus, the kinetic energy of a rotating body is given as $K_{r o t}=\dfrac{1}{2} I \omega^{2}$

So how can we find the total kinetic energy of a rolling body? To find the rotational kinetic energy we first need to find the moment of inertia then the angular velocity and substitute in the above formula. 

So how do we find the total kinetic energy of a rolling body is given by the sum total of its translational and rotational motion. Mathematically, it can be denoted as $K_{\text {rolling }}=\dfrac{1}{2} m v^{2}+\dfrac{1}{2} I \omega^{2}$

Where v is the linear or translational velocity, m is the mass of the body and $\omega$ is the angular velocity.  It is also the total kinetic energy of a rolling body also denoted as K_rolling.

Additionally, it must also be known that when a cylinder is rolling on a horizontal surface without slipping the body has an equal amount of rotational kinetic energy as its translational kinetic energy. 

Solved Examples for Kinetic Energy of a Rotating Body

Example 1:  The wheel of a car with a moment of inertia 500 kg m² is revolving with an angular velocity of 12 rad/second. What is the kinetic energy of this rotating wheel

Solution: 

Given, I= 500 kg m2 and $\omega$=12 radians/second.

Substituting the values in the formula above $K_{r o t}=\dfrac{1}{2} I \omega^{2}$ we get the $\begin{align} &K E_{r o t}=\dfrac{1}{2} \times 500 \times(12)^{2} \\ &K E_{r o t}=36000 \text { or } 36 \text { kiloJoules } \end{align}$

Example 2: A merry-go-round is rotating with an angular velocity of 20 rev/minute with a kinetic energy of 28.8 kilojoules or 28000 joules. Calculate the value of its mass of inertia or I.

Solution: 

Given, 

$\begin{align} &K_{r o t}=28.8 \text { kilojoules } \\ &\omega=\dfrac{20 \text { revolutions }}{\text { min }} \text { or } \dfrac{20 \times 2 \times \pi}{60} \dfrac{\text { radians }}{\text { second }}=\dfrac{2.09 \text { radians }}{\mathrm{sec}} \end{align}$

Using the formula $K_{r o t}=\dfrac{1}{2} I \omega^{2}$ and substituting the values we get,

$ \begin{align} &K_{r o t}=\dfrac{1}{2} I \omega^{2} \\ \\ &28800=\dfrac{1}{2} I(2.09)^{2} \\ \\ &I=13,186 \dfrac{\mathrm{kg}}{\mathrm{m}^{2}} \end{align}$