Answer
64.8k+ views
Hint: Find the magnetic field due to the current-carrying wire. Use this magnetic field equation to find the induced emf between $EF$ . Using Ohm’s law, find the current in the loop and hence find the force required to maintain the constant speed.
Complete Step by step solution:
The equation for the magnetic field around the current-carrying wire at a distance $r$ is given by
$\Rightarrow$ $B=\dfrac{{{\mu }_{o}}I}{2\pi r}$
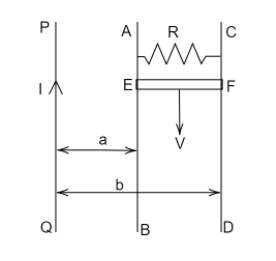
Emf induced by the current-carrying rod $PQ$ on the rod $EF$ is given by
$\Rightarrow$ $emf=\int\limits_{a}^{b}{BVdr}$
Substituting the already written equation for the magnetic field into the above equation, we get
$\Rightarrow$ $emf=\int\limits_{a}^{b}{\dfrac{{{\mu }_{o}}I}{2\pi r}Vdr}$
After integration and substituting the lower and upper limit into the integrated term, we get
$\Rightarrow$ $emf=\dfrac{{{\mu }_{o}}IV}{2\pi }\ln \left( \dfrac{b}{a} \right)$
By Ohm's law,
$I=\dfrac{V}{R}$
Therefore, using OHM’s law, we get the current due to induced emf as
$i=\dfrac{emf}{R}$
By substituting the derived equation for emf in the above equation for current, we get
$\Rightarrow$ $i=\dfrac{{{\mu }_{o}}IV}{2\pi R}\ln \left( \dfrac{b}{a} \right)$
Now, the force on the induced current-carrying rod due to the magnetic field produced due to the current flowing through the rod $PQ$ is given by
$\Rightarrow$ $F=\int\limits_{a}^{b}{Bidr}$
By substituting the equations for the magnetic field and the induced current, we get
$F=\int\limits_{a}^{b}{\dfrac{{{\mu }_{o}}I}{2\pi r}\dfrac{{{\mu }_{o}}IV}{2\pi R}\ln \left( \dfrac{b}{a} \right)dr}$
Integrating the above equation and substituting the upper and lower limits to the integrated equation gives us
$\Rightarrow$ $F=\dfrac{V}{R}{{\left( \dfrac{{{\mu }_{o}}I}{2\pi }\ln \left( \dfrac{b}{a} \right) \right)}^{2}}$
This is the force required to move the rod $EF$ at a constant velocity $V$ .
Note:
Force on a current-carrying conductor is given by
$F=BIL\sin \theta $
Here, $\theta $ is the angle subtended between the conductor and the direction of the magnetic field. In the given question, the angle between the conductor and the magnetic field is found to be ${{90}^{\circ }}$ . So, $\sin \theta $ becomes $1$ and that is the reason we don’t see the sine term in the equation.
Complete Step by step solution:
The equation for the magnetic field around the current-carrying wire at a distance $r$ is given by
$\Rightarrow$ $B=\dfrac{{{\mu }_{o}}I}{2\pi r}$
Emf induced by the current-carrying rod $PQ$ on the rod $EF$ is given by
$\Rightarrow$ $emf=\int\limits_{a}^{b}{BVdr}$
Substituting the already written equation for the magnetic field into the above equation, we get
$\Rightarrow$ $emf=\int\limits_{a}^{b}{\dfrac{{{\mu }_{o}}I}{2\pi r}Vdr}$
After integration and substituting the lower and upper limit into the integrated term, we get
$\Rightarrow$ $emf=\dfrac{{{\mu }_{o}}IV}{2\pi }\ln \left( \dfrac{b}{a} \right)$
By Ohm's law,
$I=\dfrac{V}{R}$
Therefore, using OHM’s law, we get the current due to induced emf as
$i=\dfrac{emf}{R}$
By substituting the derived equation for emf in the above equation for current, we get
$\Rightarrow$ $i=\dfrac{{{\mu }_{o}}IV}{2\pi R}\ln \left( \dfrac{b}{a} \right)$
Now, the force on the induced current-carrying rod due to the magnetic field produced due to the current flowing through the rod $PQ$ is given by
$\Rightarrow$ $F=\int\limits_{a}^{b}{Bidr}$
By substituting the equations for the magnetic field and the induced current, we get
$F=\int\limits_{a}^{b}{\dfrac{{{\mu }_{o}}I}{2\pi r}\dfrac{{{\mu }_{o}}IV}{2\pi R}\ln \left( \dfrac{b}{a} \right)dr}$
Integrating the above equation and substituting the upper and lower limits to the integrated equation gives us
$\Rightarrow$ $F=\dfrac{V}{R}{{\left( \dfrac{{{\mu }_{o}}I}{2\pi }\ln \left( \dfrac{b}{a} \right) \right)}^{2}}$
This is the force required to move the rod $EF$ at a constant velocity $V$ .
Note:
Force on a current-carrying conductor is given by
$F=BIL\sin \theta $
Here, $\theta $ is the angle subtended between the conductor and the direction of the magnetic field. In the given question, the angle between the conductor and the magnetic field is found to be ${{90}^{\circ }}$ . So, $\sin \theta $ becomes $1$ and that is the reason we don’t see the sine term in the equation.
Recently Updated Pages
Write a composition in approximately 450 500 words class 10 english JEE_Main

Arrange the sentences P Q R between S1 and S5 such class 10 english JEE_Main

What is the common property of the oxides CONO and class 10 chemistry JEE_Main

What happens when dilute hydrochloric acid is added class 10 chemistry JEE_Main

If four points A63B 35C4 2 and Dx3x are given in such class 10 maths JEE_Main

The area of square inscribed in a circle of diameter class 10 maths JEE_Main

Other Pages
A boat takes 2 hours to go 8 km and come back to a class 11 physics JEE_Main

Electric field due to uniformly charged sphere class 12 physics JEE_Main

In the ground state an element has 13 electrons in class 11 chemistry JEE_Main

According to classical free electron theory A There class 11 physics JEE_Main

Differentiate between homogeneous and heterogeneous class 12 chemistry JEE_Main

Excluding stoppages the speed of a bus is 54 kmph and class 11 maths JEE_Main