Question

In the part of a circuit shown in figure, the potential difference (${V_G} - {V_H}$) between points G and H will be:

A) $0V$
B) $15V$
C) $7V$
D) $3V$

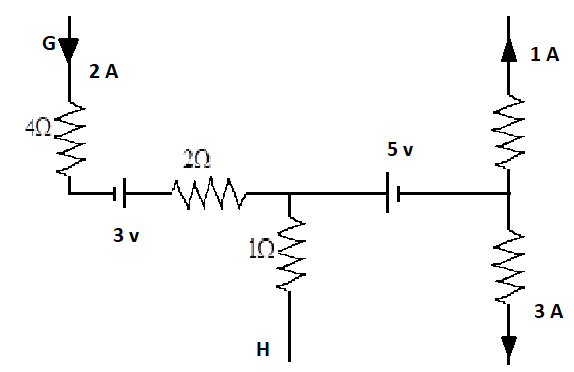
Answer 1

Hint: In order to solve this question, we have to remember all the concepts related to Kirchhoff’s current law, Kirchhoff’s voltage law and junction law. Kirchhoff’s current law is also known as Kirchhoff’s first law.

Complete step by step solution:
Kirchhoff’s current law (KCL) states that the total current or charge entering a node is equal to the charge leaving the node, as no charge is lost within the node.
Using Kirchhoff’s current law, we can have the current flowing through the $1\Omega $ resistor, which is $2A$ in the upward direction.

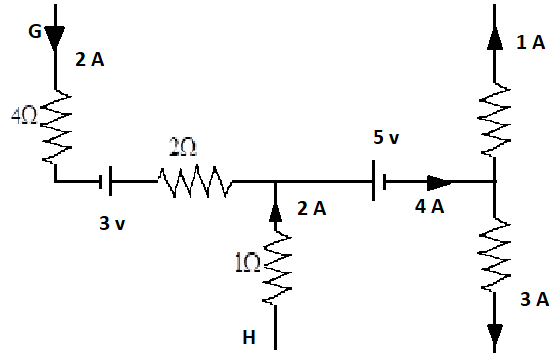
Now, On applying kirchhoff’s current law between points G and H, we have
${V_G} - 2 \times 4 + 3 - 2 \times 2 + 2 \times 1 - {V_H} = 0$
$ \Rightarrow {V_G} - {V_H} = 7V$
Therefore, by applying Krichhoff’s current law we get the potential difference (${V_G} - {V_H}$) between points G and H as $7V$.

Hence, the correct option is (C).

Additional Information: Kirchhoff’s circuit law has two laws:
Kirchhoff’s current law (KCL): This law states that the total current entering a node is equal to the current leaving the node. The algebraic sum of all the currents entering and leaving a node must be zero.
Kirchhoff’s voltage law: This law states that in a close loop, the total voltage in that close loop is equal to the sum of all the voltage drops in that same loop. This law deals with the conservation of energy.

Note: Always keep in mind that Kirchhoff’s current law deals with the conservation of current whereas Kirchhoff’s voltage law deals with the conservation of energy entering and leaving a node. Always write the answers in their SI units.