Question

In the above circuit the current in each resistance is :
a) 0 A
b) 1 A
c) 0.25 A
d) 0.5 A

Answer 1

Hint: The given circuit can be split using Kirchhoff’s law. Apply Kirchhoff’s law by splitting the circuit into 3 loops and identify the current at each resistance.

Complete step by step Solution:
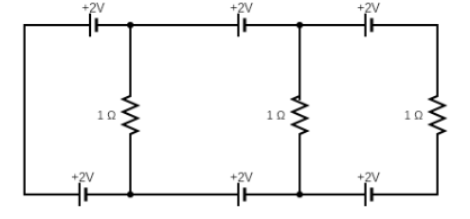
The given circuit can be split into 3 loops , loop 1 consisting of two 2V source and 1 ohm resistor and the second loop consisting of two 2V source and two 1 ohm resistors and the final loop similar as the second loop.
To identify the current at each loop we can use Kirchhoff’s Voltage rule and grounding method. At the grounding method, we can close the loop at one point where we can consider it as ground.
Now the flow of current takes place from the positive node of 2V source in the lower section to the negative node of the source in lower section.
Applying KCL at node 1,
Note: Current at loop1 is assumed as i1 , current at loop2 is assumed as i2 and current at loop3 is assumed as i3.
The current flowing in loop 1 is i1
\[2 - 2 + ({i_1} - {i_2}) \times 1 = 0\]
\[ \Rightarrow {i_1} = {i_2}\]
Applying KCL at node 2,
\[2 + (({i_1} - {i_2}) \times 1) - 2 + (({i_2} - {i_3}) \times 1) = 0\]
Since, i1=i2
\[2 - 2 + (({i_2} - {i_3}) \times 1) = 0\]
\[ \Rightarrow {i_2} = {i_3}\]
Applying KVL at loop 3,
\[2 + (({i_2} - {i_3}) \times 1) - 2 + ({i_3} \times 1) = 0\]
Since i2=i3
\[ \Rightarrow 2 - 2 + ({i_3} \times 1) = 0\]
\[ \Rightarrow {i_3} = 0\]
Therefore since \[{i_2} = {i_3}\], the value of \[{i_2} = 0\]
And since the value of \[{i_1} = {i_2}\]
The current value in loop 1 is also equal to 0.
Hence it is found that the value of current across all the resistors to be zero. Hence, option(a) is the right answer.

Note:
Kirchhoff’s Voltage law states that in any closed loop network, the total voltage that is circulating in the loop is equal to the algebraic sum of all voltage drops inside the loop.