Answer
64.8k+ views
Hint: When two longitudinal waves of same frequency travel through a medium in the opposite directions standing waves are produced.
Complete solution:
In an organ pipe column the sound behaves as a standing wave. The waves resonate harmonically.
There are two types of organ pipes;
Closed organ pipe: One end of the pipe is closed.
Open organ pipe: Both the ends are open.
In an air column filled with water, the conditions are the same as a closed organ pipe.
Hence, we can treat the situation as a closed organ pipe.
Now for standing waves in closed pipe the condition is given by;
$l = (2n + 1)\dfrac{\lambda }{4}$ (equation: 1)
Here l=length of air column, n= 0, 1, 2… $\lambda $=wavelength of the wave.
The diagram below will help you understand the situation
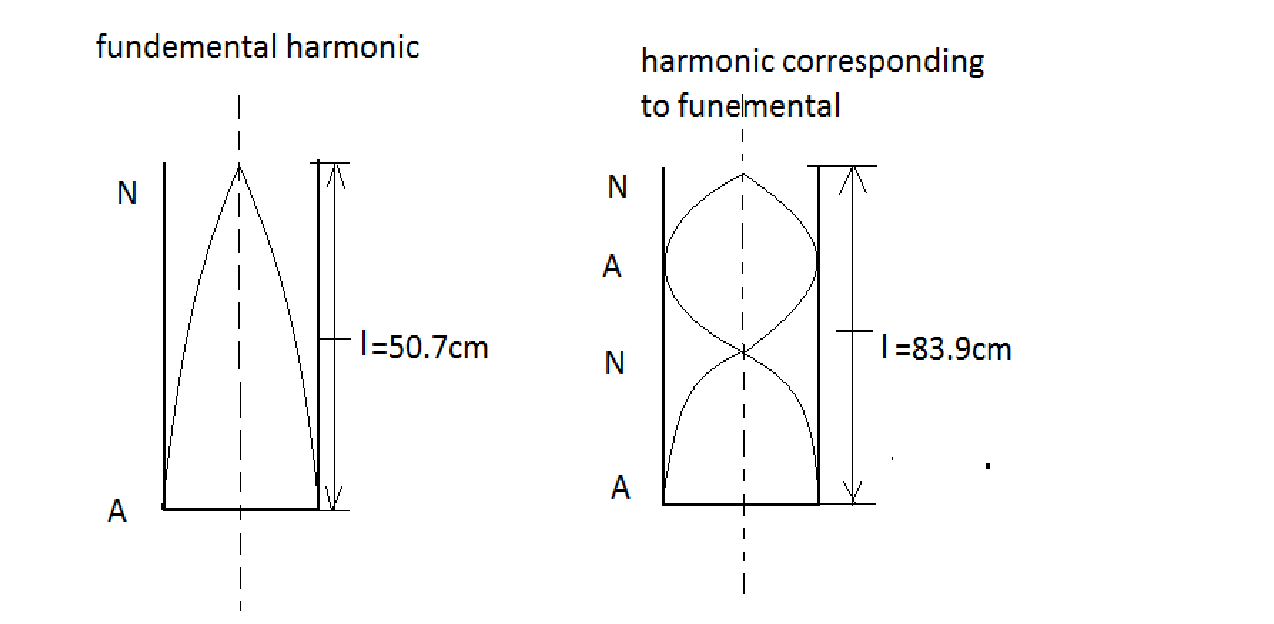
Now the above figure is just an example but not the actual case, as we do not know the number of harmonics.
Now, let us assume that the two successive harmonics at 50.7 and 83.9 cms be ${n_1}$ and ${n_2}$ respectively.
As the difference in length of two successive resonating air columns is half the wavelength of the wave,
$83.9 - 50.7 = \dfrac{\lambda }{2}$
Thus,$\lambda = 2 \times 33.2 = 66.4$
Hence, wavelength of sound is 66.4cm
Now as $\dfrac{\lambda }{4} = 16.6cm$, 50.7 is not the fundamental harmonic.
Also, as $\dfrac{{3\lambda }}{4} = 49.8 = e + 50.7cm$ (here, e is the end correction)
50.7 is the third harmonic and so 83.9 will be the fifth harmonic.
Now, $e = 49.8 - 50.7 = - 0.9cm$.
Hence the end correction is 0.9cm.
Now for the speed of sound $v = \lambda \upsilon $ ($\lambda $is the wavelength, $\upsilon $ is the frequency)
$v = 66.4 \times 500 = 33200cm{s^{ - 1}} = 332m{s^{ - 1}}$
Thus option A, option B and option C are correct.
Note:
(1) In the case of air columns, always consider some end correction.
(2) For organ pipe end correction may not be considered.
Complete solution:
In an organ pipe column the sound behaves as a standing wave. The waves resonate harmonically.
There are two types of organ pipes;
Closed organ pipe: One end of the pipe is closed.
Open organ pipe: Both the ends are open.
In an air column filled with water, the conditions are the same as a closed organ pipe.
Hence, we can treat the situation as a closed organ pipe.
Now for standing waves in closed pipe the condition is given by;
$l = (2n + 1)\dfrac{\lambda }{4}$ (equation: 1)
Here l=length of air column, n= 0, 1, 2… $\lambda $=wavelength of the wave.
The diagram below will help you understand the situation

Now the above figure is just an example but not the actual case, as we do not know the number of harmonics.
Now, let us assume that the two successive harmonics at 50.7 and 83.9 cms be ${n_1}$ and ${n_2}$ respectively.
As the difference in length of two successive resonating air columns is half the wavelength of the wave,
$83.9 - 50.7 = \dfrac{\lambda }{2}$
Thus,$\lambda = 2 \times 33.2 = 66.4$
Hence, wavelength of sound is 66.4cm
Now as $\dfrac{\lambda }{4} = 16.6cm$, 50.7 is not the fundamental harmonic.
Also, as $\dfrac{{3\lambda }}{4} = 49.8 = e + 50.7cm$ (here, e is the end correction)
50.7 is the third harmonic and so 83.9 will be the fifth harmonic.
Now, $e = 49.8 - 50.7 = - 0.9cm$.
Hence the end correction is 0.9cm.
Now for the speed of sound $v = \lambda \upsilon $ ($\lambda $is the wavelength, $\upsilon $ is the frequency)
$v = 66.4 \times 500 = 33200cm{s^{ - 1}} = 332m{s^{ - 1}}$
Thus option A, option B and option C are correct.
Note:
(1) In the case of air columns, always consider some end correction.
(2) For organ pipe end correction may not be considered.
Recently Updated Pages
Write a composition in approximately 450 500 words class 10 english JEE_Main

Arrange the sentences P Q R between S1 and S5 such class 10 english JEE_Main

What is the common property of the oxides CONO and class 10 chemistry JEE_Main

What happens when dilute hydrochloric acid is added class 10 chemistry JEE_Main

If four points A63B 35C4 2 and Dx3x are given in such class 10 maths JEE_Main

The area of square inscribed in a circle of diameter class 10 maths JEE_Main

Other Pages
In the ground state an element has 13 electrons in class 11 chemistry JEE_Main

Excluding stoppages the speed of a bus is 54 kmph and class 11 maths JEE_Main

Differentiate between homogeneous and heterogeneous class 12 chemistry JEE_Main

Electric field due to uniformly charged sphere class 12 physics JEE_Main

According to classical free electron theory A There class 11 physics JEE_Main

A boat takes 2 hours to go 8 km and come back to a class 11 physics JEE_Main



