Question

In a uniform electric field, the potential is 10V at the origin of coordinates, and 8V at each of the points (1,0,0), (0,1,0) and (0,0,1). The potential at the point (1, 1, 1) will be:
A) $0$
B) $4V$
C) $8V$
D) $10V$

Answer 1

Hint: The relation between electric field and potential has a negative sign which implies that the direction of electric field is in the direction of decreasing potential. No work done in moving a test charge over an equipotential surface.

Formula used:
We have a relation that is, relation between electric potential and electric field. that is,
$E = - \dfrac{{dV}}{{dr}}$ …........(1)

Complete step by step answer:

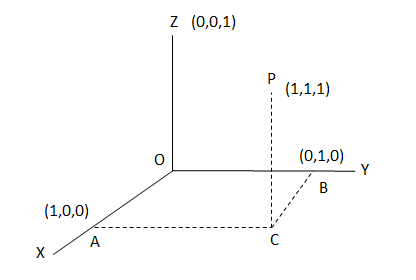
In a given question there are $x$, $y$, $z$ coordinate axes.
Let we have,$E = {E_x}\hat i + {E_y}\hat j + {E_z}\hat k$ along $x$, $y$ and $z$ axis respectively.
Similarly,$dr = dx\hat i + dy\hat j + dz\hat k$
From equation (1),
$dV = - E.dr$
Electric potential \[\;dV\] is scalar quantity therefore, representing in terms of scalar product or dot product.
Now substitute its values in the above equation we get,
$dV = - ({E_x}\hat i + {E_y}\hat j + {E_z}\hat k).(dx\hat i + dy\hat j + dz\hat k)$
After multiplication using the concept of dot product, that is,
According to this,$\hat i.\hat i = 1$
$\hat j.\hat j = 1$And $\hat k.\hat k = 1$
Also we have, \[\hat i.\hat j = \hat j.\hat k = \hat k.\hat i = 0\]
Then we get, $dV = - {E_x}dx - {E_y}dy - {E_z}dz$
After integration, we can write it as,
This can written as, ${V_{\left( {x,y,z} \right)}} = - {E_x}x - {E_y}y - {E_z}z + c$…………….(2)
Given, the potential is 10v at the origin of coordinates that is at ($0$, $0$, $0$)
Then, at origin, ${V_{\left( {0,0,0} \right)}} = 10V$
At origin equation (2) becomes, ${V_{\left( {0,0,0} \right)}} = - {E_x}x - {E_y}y - {E_z}z + c$
$10 = - {E_x} \times 0 - {E_y} \times 0 - {E_z} \times 0 + c$
$ \Rightarrow c = 10$
Now equation (2) becomes, substituting the value of c,
${V_{\left( {x,y,z} \right)}} = 10 - {E_x}x - {E_y}y - {E_z}z$
Given in the question, 8v at each of the points ($1$,$0$,$0$), ($0$,$1$,$0$) and ($0$,$0$,$1$) that is,
$\Rightarrow {V_{\left( {1,0,0} \right)}} = {V_{(0,1,0)}} = {V_{\left( {0,0,1} \right)}} = 8V$
From these values we can calculate the electric field. We know that, electric field is uniform
Then, ${V_{\left( {1,0,0} \right)}} = 10 - {E_x}x - {E_y}y - {E_z}z = 10 - {E_x} \times 1 - {E_y} \times 0 - {E_z} \times 0$
After simplification, we get $8 = 10 - {E_x}$
$\Rightarrow {E_x} = 2V$
Similarly, ${E_x} = {E_y} = {E_z} = 2V$ since the given electric field is uniform.
Now let us the potential at the point ($1$, $1$, $1$) will be,
$\Rightarrow {V_{\left( {x,y,z} \right)}} = 10 - {E_x}x - {E_y}y - {E_z}z$
On putting the values and we get
$\Rightarrow {V_{\left( {1,1,1} \right)}} = 10 - 2 \times 1 - 2 \times 1 - 2 \times 1$
On simplification we get
$ \Rightarrow {V_{\left( {1,1,1} \right)}} = 10 - 6$
Let us subtract we get
$\therefore {V_{\left( {1,1,1} \right)}} = 4V$

Thus, the potential at the point ($1$, $1$, $1$) will be $4V$.

Note: Any surface which has the same electric potential at every pint is called equipotential surface.
When a charged particle moves in an electric field, the field exerts a force that can do work on the particle. This work can always be expressed in terms of electrostatic potential energy.