Answer
359.1k+ views
Hint: Find the number of students who play both cricket and tennis by finding \[n\left( A\cup B \right)\]. From this the students who play neither cricket nor tennis can be formed by subtracting from total students.
Complete step-by-step answer:
Given the total number of students in a class = 60.
Let ‘A’ be the set of students who play cricket, which is 25 in number.
\[\therefore n\left( A \right)=25\]
Let ‘B’ be the set of students who play tennis, 20 in number.
\[\therefore n\left( B \right)=20\]
The number of students who play both cricket and tennis is 10.
\[\therefore n\left( A\cap B \right)=10\]
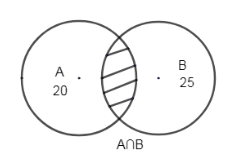
The shaded area shows \[A\cap B\].
The intersection of two sets A and B, consist of all elements that are both in A and B. The figure shows a Venn diagram representing the same.
Here, we are asked to find the number of students who don’t play cricket or tennis. Thus we need to find \[\left( A\cup B \right)\] and subtract it from the total number of students.
\[A\cup B\] is A union B, which means creating a new set containing every element from either of A and B.
The given Venn diagram represents \[A\cup B\].
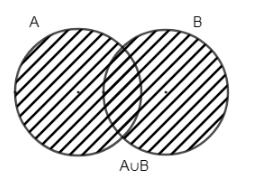
Hence, \[n\left( A\cup B \right)=n\left( A \right)+n\left( B \right)-n\left( A\cap B \right)\]
This formula can be directly derived from the above Venn diagram,
\[\therefore n\left( A\cup B \right)=25+20-10=35\].
Here, 35 students play at least one out of cricket or tennis out of 60 students in a class.
\[\therefore \]The number of students who play neither cricket nor tennis =
Total students – number of students who play at least one game
= Total students - \[n\left( A\cup B \right)\]
= 60 – 35 = 25
\[\therefore \]The number of students who play neither cricket nor tennis = 25.
Hence, option (d) is the correct answer.
Note: A Venn diagram is used to represent all possible relations of different sets. Here we used \[A\cap B\], which is the intersection of 2 sets to represent the common elements in both set A and B. And \[A\cup B\]represents the combined elements of set A and B.
Care should be taken not to confuse between \[A\cap B\] and \[A\cup B\].
Complete step-by-step answer:
Given the total number of students in a class = 60.
Let ‘A’ be the set of students who play cricket, which is 25 in number.
\[\therefore n\left( A \right)=25\]
Let ‘B’ be the set of students who play tennis, 20 in number.
\[\therefore n\left( B \right)=20\]
The number of students who play both cricket and tennis is 10.
\[\therefore n\left( A\cap B \right)=10\]

The shaded area shows \[A\cap B\].
The intersection of two sets A and B, consist of all elements that are both in A and B. The figure shows a Venn diagram representing the same.
Here, we are asked to find the number of students who don’t play cricket or tennis. Thus we need to find \[\left( A\cup B \right)\] and subtract it from the total number of students.
\[A\cup B\] is A union B, which means creating a new set containing every element from either of A and B.
The given Venn diagram represents \[A\cup B\].

Hence, \[n\left( A\cup B \right)=n\left( A \right)+n\left( B \right)-n\left( A\cap B \right)\]
This formula can be directly derived from the above Venn diagram,
\[\therefore n\left( A\cup B \right)=25+20-10=35\].
Here, 35 students play at least one out of cricket or tennis out of 60 students in a class.
\[\therefore \]The number of students who play neither cricket nor tennis =
Total students – number of students who play at least one game
= Total students - \[n\left( A\cup B \right)\]
= 60 – 35 = 25
\[\therefore \]The number of students who play neither cricket nor tennis = 25.
Hence, option (d) is the correct answer.
Note: A Venn diagram is used to represent all possible relations of different sets. Here we used \[A\cap B\], which is the intersection of 2 sets to represent the common elements in both set A and B. And \[A\cup B\]represents the combined elements of set A and B.
Care should be taken not to confuse between \[A\cap B\] and \[A\cup B\].
Recently Updated Pages
Write a composition in approximately 450 500 words class 10 english JEE_Main

Arrange the sentences P Q R between S1 and S5 such class 10 english JEE_Main

What is the common property of the oxides CONO and class 10 chemistry JEE_Main

What happens when dilute hydrochloric acid is added class 10 chemistry JEE_Main

If four points A63B 35C4 2 and Dx3x are given in such class 10 maths JEE_Main

The area of square inscribed in a circle of diameter class 10 maths JEE_Main



