Question

If the area of each plate is $A$ and separation between consecutive plates are $d$, then equivalent capacitance between $A$ and $B$, is

$\left( a \right)$ $\dfrac{{3{\varepsilon _0}A}}{{4d}}$
$\left( b \right)$ $\dfrac{{4{\varepsilon _0}A}}{{3d}}$
$\left( c \right)$ $\dfrac{{3{\varepsilon _0}A}}{d}$
$\left( d \right)$ $\dfrac{{2{\varepsilon _0}A}}{{3d}}$

Answer 1

Hint Since it is given that the separation between them is $d$ and also the terminal $B$ is connected between the second and third terminal end of capacitance. So except the first capacitor, all will be in the series. And then they will be in parallel. So solving both of them and we will get the equivalent capacitance.
Formula used:
So if the capacitance is in series,
$\dfrac{1}{c} = \dfrac{1}{{{c_1}}} + \dfrac{1}{{{c_2}}} + .....$
And similarly, if the capacitance is in parallel then
${c_p} = {c_1} + {c_2} + ......$

Complete Step by Step Solution So before solving these types of questions, first of all, we should make the circuit diagram and name them as said in the problem.

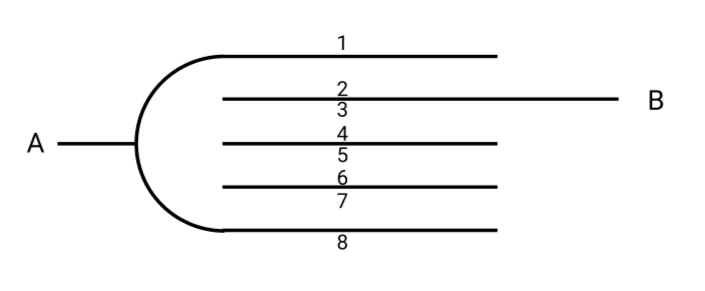
Now, when we make the circuit diagram of the above figure, it will look like this

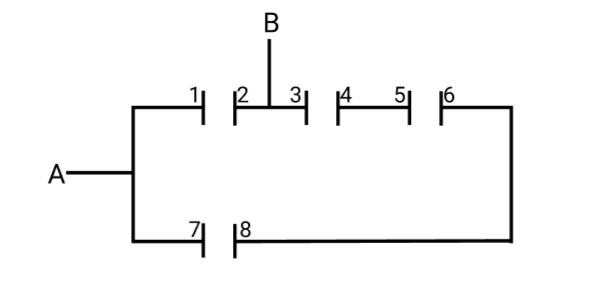
So from this figure, it is clear to us that the $B$terminal is connected between the second and third capacitance. So the capacitance made from the first and second will be parallel to the rest of the capacitance.
With the figure, it will be clearer to us.

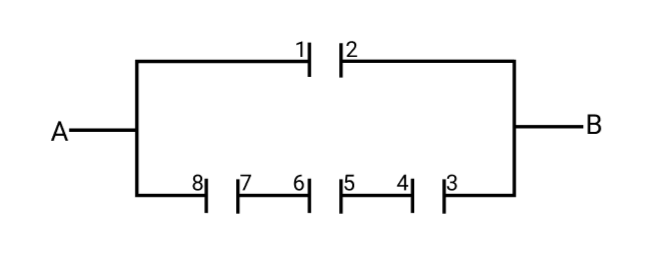
So now we will apply the formula for the simplification of series and parallel capacitance.
Firstly${c_{34}}$, ${c_{65}}$and${c_{87}}$all are in series.
So on applying the series formula,
We get
Since all have the same capacitance that is $c$
Therefore,
$ \Rightarrow \dfrac{1}{{{c_{series}}}} = \dfrac{1}{c} + \dfrac{1}{c} + \dfrac{1}{c}$
Now on adding and solving the above equation, we get
$ \Rightarrow {c_{series}} = \dfrac{c}{3}$
Now the ${c_{12}}$will come in parallel with ${c_{series}}$
Therefore, on solving it, we get
$ \Rightarrow {c_{eq}} = c + \dfrac{c}{3}$
On further simplifying this, we get
$ \Rightarrow {c_{eq}} = \dfrac{{4c}}{3}$
And as we know $c = \dfrac{{{\varepsilon _0}A}}{d}$
Therefore the above equation can be written as
$ \Rightarrow {c_{eq}} = \dfrac{4}{3}\dfrac{{{\varepsilon _0}A}}{d}$

Therefore the option $B$ is correct.

Note In the Series circuit there is only one path for electrons to flow. Only one current. In parallel circuits you have more than one path for electrons to flow through, thereby you have different currents that you want to use for different purposes. From the point of view of the voltage, in parallel circuits, it is the same voltage level for elements connected in parallel mode (remember that voltage is a level of electric potential, not a flow like the current).