Answer
64.8k+ views
Hint: The given equation takes the exact form of the progressive wave equation$y = A\sin (\omega t - kx)$. So, on comparing both the wave functions, find out the value of the propagation constant k. k is related to the wavelength by $k = \dfrac{{2\pi }}{\lambda }$ . Now on substituting the values calculate the wavelength of the wave.
Complete step-by-step answer
A mathematical description of the disturbance created by a wave is called a wave function.
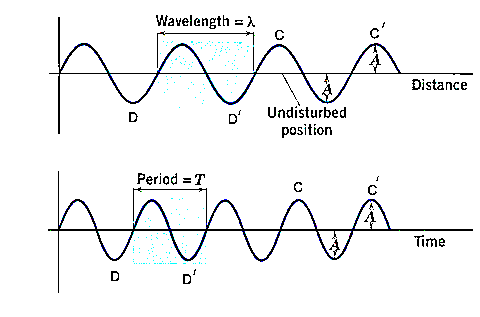
We know that, a progressive wave function is given by,
$y = A\sin (\omega t - kx)$
Where,
Y is the displacement; A is the amplitude; \[\omega \]is the angular frequency; x is the position; k is the propagation constant and t is the time.
$y = 0.0015\sin (62.4x + 316t)$
The given equation is similar to this wave function and therefore has corresponding values. On comparing with the progressive wave function we find out the value of the propagation constant also known as angular wave number.
$k = 62.8$
The number of wavelengths in the distance\[2\pi \]is called the wave number which is given by,
$k = \dfrac{{2\pi }}{\lambda }$
Substitute the value of the angular wave number.
$\lambda = \dfrac{{2 \times 3.14}}{{62.8}}$
$\lambda = 0.1unit$
Hence, the wavelength of the wave is 0.1 units and the correct option is C.
Note: If during the propagation of a progressive wave, the particles of the medium perform SHM about their mean position then the wave is known as a harmonic progressive wave. Various forms of progressive wave function.
$y = A\sin 2\pi \left( {\dfrac{t}{T} - \dfrac{x}{\lambda }} \right)$
$y = A\sin \dfrac{{2\pi }}{T}\left( {t - \dfrac{{xT}}{\lambda }} \right)$
$y = A\sin \dfrac{{2\pi }}{\lambda }\left( {vt - x} \right)$
$y = A\sin \omega \left( {t - \dfrac{x}{v}} \right)$
We can also write it in exponential form
$y = A\dfrac{{{e^{i\omega \left( {t - \dfrac{x}{v}} \right)}} - {e^{ - i\omega \left( {t - \dfrac{x}{v}} \right)}}}}{{2i}}$
Complete step-by-step answer
A mathematical description of the disturbance created by a wave is called a wave function.

We know that, a progressive wave function is given by,
$y = A\sin (\omega t - kx)$
Where,
Y is the displacement; A is the amplitude; \[\omega \]is the angular frequency; x is the position; k is the propagation constant and t is the time.
$y = 0.0015\sin (62.4x + 316t)$
The given equation is similar to this wave function and therefore has corresponding values. On comparing with the progressive wave function we find out the value of the propagation constant also known as angular wave number.
$k = 62.8$
The number of wavelengths in the distance\[2\pi \]is called the wave number which is given by,
$k = \dfrac{{2\pi }}{\lambda }$
Substitute the value of the angular wave number.
$\lambda = \dfrac{{2 \times 3.14}}{{62.8}}$
$\lambda = 0.1unit$
Hence, the wavelength of the wave is 0.1 units and the correct option is C.
Note: If during the propagation of a progressive wave, the particles of the medium perform SHM about their mean position then the wave is known as a harmonic progressive wave. Various forms of progressive wave function.
$y = A\sin 2\pi \left( {\dfrac{t}{T} - \dfrac{x}{\lambda }} \right)$
$y = A\sin \dfrac{{2\pi }}{T}\left( {t - \dfrac{{xT}}{\lambda }} \right)$
$y = A\sin \dfrac{{2\pi }}{\lambda }\left( {vt - x} \right)$
$y = A\sin \omega \left( {t - \dfrac{x}{v}} \right)$
We can also write it in exponential form
$y = A\dfrac{{{e^{i\omega \left( {t - \dfrac{x}{v}} \right)}} - {e^{ - i\omega \left( {t - \dfrac{x}{v}} \right)}}}}{{2i}}$
Recently Updated Pages
Write a composition in approximately 450 500 words class 10 english JEE_Main

Arrange the sentences P Q R between S1 and S5 such class 10 english JEE_Main

What is the common property of the oxides CONO and class 10 chemistry JEE_Main

What happens when dilute hydrochloric acid is added class 10 chemistry JEE_Main

If four points A63B 35C4 2 and Dx3x are given in such class 10 maths JEE_Main

The area of square inscribed in a circle of diameter class 10 maths JEE_Main

Other Pages
In the ground state an element has 13 electrons in class 11 chemistry JEE_Main

Excluding stoppages the speed of a bus is 54 kmph and class 11 maths JEE_Main

Differentiate between homogeneous and heterogeneous class 12 chemistry JEE_Main

Electric field due to uniformly charged sphere class 12 physics JEE_Main

According to classical free electron theory A There class 11 physics JEE_Main

A boat takes 2 hours to go 8 km and come back to a class 11 physics JEE_Main



