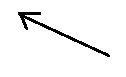
Answer
64.8k+ views
Hint: Since the magnitudes of the vectors are the same so we need to only consider their direction. In order to calculate the acceleration, the direction of the first vector must be reversed to represent the difference.
Complete step by step answer
The basic difference between scalars and vectors is that scalar quantities just represent the magnitude whereas the vector represents both the direction and magnitude of the quantity.
For example, mass of a body does not have any particular direction but it does have a magnitude to represent the quantity. These types of quantities are called scalar quantities. On the other hand, the force acting on a body can be represented by both direction and magnitude. When we push someone or something, we are exerting some amount of force in a particular direction. These quantities are called vectors.
Vectors are usually denoted by drawing an arrow just above the sign representing the quantity.
In this question we need to find the resultant of two vectors.
We are given the velocity vectors at time ${t_1}$ and ${t_2}$
Now, acceleration is defined as the rate of change of velocity
So, $\overrightarrow a = \dfrac{{\overrightarrow {{v_2}} - \overrightarrow {{v_1}} }}{{{t_2} - {t_1}}}$
Now, the resultant of vectors $\overrightarrow {{v_1}} $ and $\overrightarrow {{v_2}} $results in option A. Since we need to find their difference, we can assume that the direction of vector $\overrightarrow {{v_1}} $ is reversed.
If this direction is reversed then their resultant vector would be correctly represented by option C.
Therefore, the correct option is C.
Note: Unlike scalar quantities, vectors have two types of product. Dot and cross. Dot product tells us how much of two vectors are in the same direction whereas the cross product tells us how little the two vectors are in the same direction. Dot product of two vectors gives us a scalar quantity, while the cross product of two vectors gives us a vector quantity.
Complete step by step answer
The basic difference between scalars and vectors is that scalar quantities just represent the magnitude whereas the vector represents both the direction and magnitude of the quantity.
For example, mass of a body does not have any particular direction but it does have a magnitude to represent the quantity. These types of quantities are called scalar quantities. On the other hand, the force acting on a body can be represented by both direction and magnitude. When we push someone or something, we are exerting some amount of force in a particular direction. These quantities are called vectors.
Vectors are usually denoted by drawing an arrow just above the sign representing the quantity.
In this question we need to find the resultant of two vectors.
We are given the velocity vectors at time ${t_1}$ and ${t_2}$
Now, acceleration is defined as the rate of change of velocity
So, $\overrightarrow a = \dfrac{{\overrightarrow {{v_2}} - \overrightarrow {{v_1}} }}{{{t_2} - {t_1}}}$
Now, the resultant of vectors $\overrightarrow {{v_1}} $ and $\overrightarrow {{v_2}} $results in option A. Since we need to find their difference, we can assume that the direction of vector $\overrightarrow {{v_1}} $ is reversed.
If this direction is reversed then their resultant vector would be correctly represented by option C.
Therefore, the correct option is C.
Note: Unlike scalar quantities, vectors have two types of product. Dot and cross. Dot product tells us how much of two vectors are in the same direction whereas the cross product tells us how little the two vectors are in the same direction. Dot product of two vectors gives us a scalar quantity, while the cross product of two vectors gives us a vector quantity.
Recently Updated Pages
Write a composition in approximately 450 500 words class 10 english JEE_Main

Arrange the sentences P Q R between S1 and S5 such class 10 english JEE_Main

What is the common property of the oxides CONO and class 10 chemistry JEE_Main

What happens when dilute hydrochloric acid is added class 10 chemistry JEE_Main

If four points A63B 35C4 2 and Dx3x are given in such class 10 maths JEE_Main

The area of square inscribed in a circle of diameter class 10 maths JEE_Main

Other Pages
Excluding stoppages the speed of a bus is 54 kmph and class 11 maths JEE_Main

In the ground state an element has 13 electrons in class 11 chemistry JEE_Main

Electric field due to uniformly charged sphere class 12 physics JEE_Main

A boat takes 2 hours to go 8 km and come back to a class 11 physics JEE_Main

According to classical free electron theory A There class 11 physics JEE_Main

Differentiate between homogeneous and heterogeneous class 12 chemistry JEE_Main