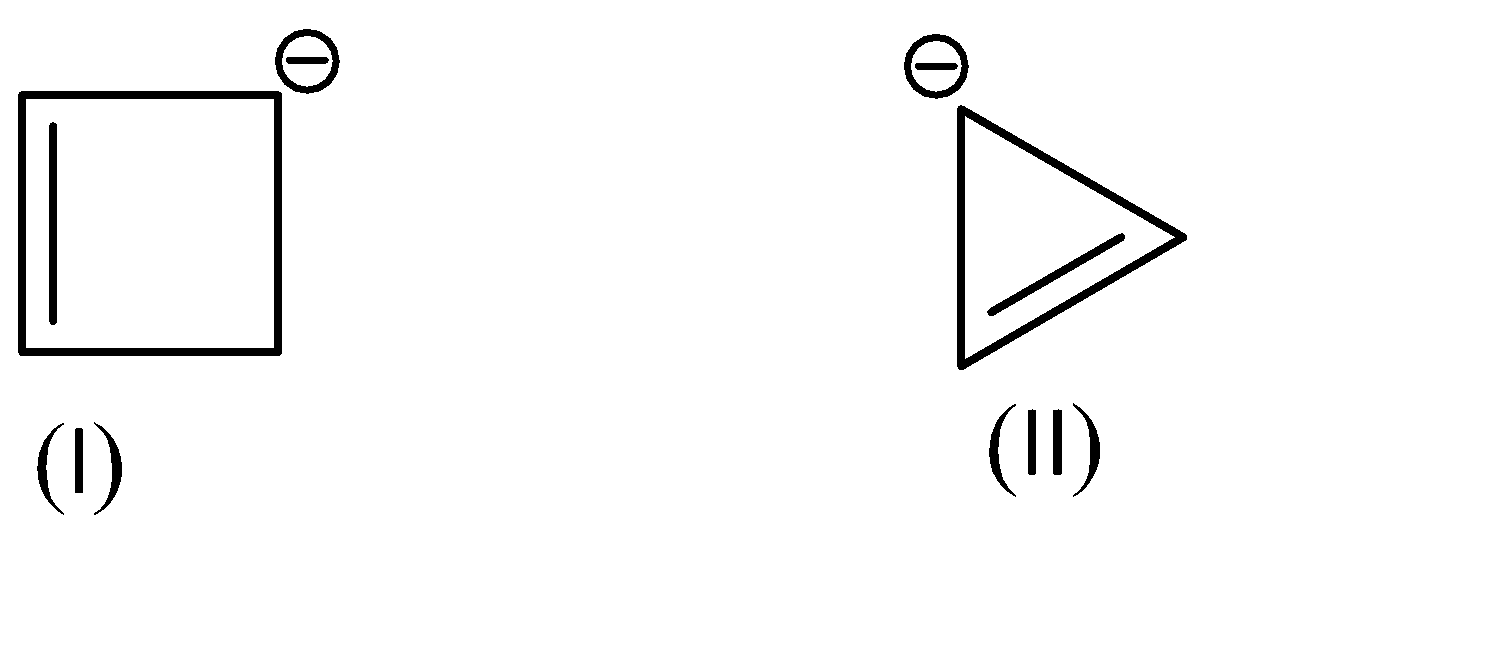Answer
64.8k+ views
Hint: Aromatic and Anti-aromatic compounds can be differentiated on the basis of Huckel's Rule.
Complete step by step answer:
We can identify the and classify compounds as either aromatic or anti-aromatic based on the following rules:
1.For a compound to be Aromatic, the following conditions must be satisfied
-The compound should be cyclic
-The molecular geometry of the compound should be planar
-The compound should exhibit Resonance
-The compound should obey Huckle’s rule, and hence should have (4n+2) \[\pi \]electrons, where ‘n’ denotes the number of subsequent energy levels and can be equal to any whole number, i.e. 0,1,2,3,…
2.And for the compound to be Anti-Aromatic, the following conditions must be satisfied
-The compound should be cyclic
-The molecular geometry of the compound should be planar
-The compound should exhibit Resonance
-The compound should obey Huckle’s rule, and hence should have (4n) \[\pi \]electrons
3.If a compound does not have a continuous ring of conjugated p orbitals in a planar conformation, then it is nonaromatic
One important question you must be having is how we identify \[\pi \]electrons. For that, remember the following points. \[\pi \]electrons lie in p orbitals and \[s{p^2}\]hybridized orbitals, each have 1 p orbital. In a completely conjugated molecule, each carbon atom is \[s{p^2}\]hybridized, and hence the electrons present in these p orbitals are \[\pi \]electrons.
As for the compounds given to us,

Compound (I) satisfies the following conditions:
1.It is a cyclic compound
2.Its molecular geometry is planar
3.But it does not exhibit resonance

Shifting of electrons would bring us back to the same structure.
On the other hand, Compound (II) satisfies the following conditions:
1.It is a cyclic compound
2.Its molecular geometry is planar
3.It does exhibit resonance
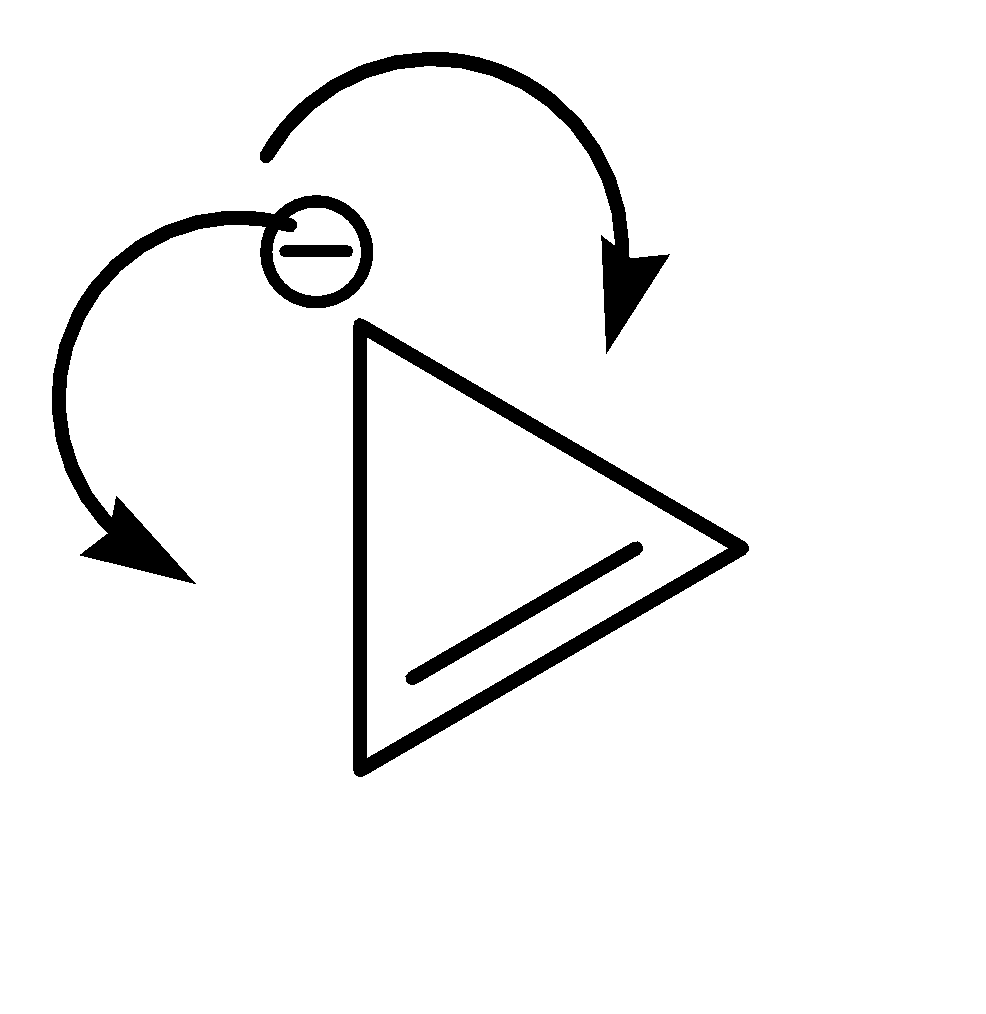
This compound exhibits resonance in both directions.
And it has 4\[\pi \]electrons, which satisfies Huckle’s rule with the value of n=1.
Hence, we can conclude that Compound (I) is Non-Aromatic and Compound (II) is Anti-Aromatic.
-We know that, the trend in the stability of Aromatic, Non-Aromatic and Anti Aromatic compounds is:
Aromatic > Non-Aromatic > Anti-Aromatic
Hence, Anti aromatic compounds are less stable than Non-aromatic Compounds. This means that the conjugate bases of Anti aromatic compounds are more stable than Non-aromatic Compounds. And we know that the increasing stability of the conjugate base increases the acidity of the compound.
Hence Anti aromatic compounds are less acidic than Non-aromatic Compounds.
\[p{K_a}\] is a method used to indicate the strength of an acid. The lower the value of \[p{K_a}\], higher is the strength of the acid.
Now, relating the above conclusions to the given compounds, we get,
\[p{K_a}\] of Compound(I) < \[p{K_a}\]of Compound (II)
Hence, both the reason and assertion are true and are interdependent.
Option A is the correct
Note:
You should not fret over finding the value of ‘n’ in Huckle’s rule because ‘n’ is not a characteristic property of any molecule. It is merely a constant which can be substituted with any natural number to satisfy the Huckel's Rule.
Complete step by step answer:
We can identify the and classify compounds as either aromatic or anti-aromatic based on the following rules:
1.For a compound to be Aromatic, the following conditions must be satisfied
-The compound should be cyclic
-The molecular geometry of the compound should be planar
-The compound should exhibit Resonance
-The compound should obey Huckle’s rule, and hence should have (4n+2) \[\pi \]electrons, where ‘n’ denotes the number of subsequent energy levels and can be equal to any whole number, i.e. 0,1,2,3,…
2.And for the compound to be Anti-Aromatic, the following conditions must be satisfied
-The compound should be cyclic
-The molecular geometry of the compound should be planar
-The compound should exhibit Resonance
-The compound should obey Huckle’s rule, and hence should have (4n) \[\pi \]electrons
3.If a compound does not have a continuous ring of conjugated p orbitals in a planar conformation, then it is nonaromatic
One important question you must be having is how we identify \[\pi \]electrons. For that, remember the following points. \[\pi \]electrons lie in p orbitals and \[s{p^2}\]hybridized orbitals, each have 1 p orbital. In a completely conjugated molecule, each carbon atom is \[s{p^2}\]hybridized, and hence the electrons present in these p orbitals are \[\pi \]electrons.
As for the compounds given to us,

Compound (I) satisfies the following conditions:
1.It is a cyclic compound
2.Its molecular geometry is planar
3.But it does not exhibit resonance

Shifting of electrons would bring us back to the same structure.
On the other hand, Compound (II) satisfies the following conditions:
1.It is a cyclic compound
2.Its molecular geometry is planar
3.It does exhibit resonance

This compound exhibits resonance in both directions.
And it has 4\[\pi \]electrons, which satisfies Huckle’s rule with the value of n=1.
Hence, we can conclude that Compound (I) is Non-Aromatic and Compound (II) is Anti-Aromatic.
-We know that, the trend in the stability of Aromatic, Non-Aromatic and Anti Aromatic compounds is:
Aromatic > Non-Aromatic > Anti-Aromatic
Hence, Anti aromatic compounds are less stable than Non-aromatic Compounds. This means that the conjugate bases of Anti aromatic compounds are more stable than Non-aromatic Compounds. And we know that the increasing stability of the conjugate base increases the acidity of the compound.
Hence Anti aromatic compounds are less acidic than Non-aromatic Compounds.
\[p{K_a}\] is a method used to indicate the strength of an acid. The lower the value of \[p{K_a}\], higher is the strength of the acid.
Now, relating the above conclusions to the given compounds, we get,
\[p{K_a}\] of Compound(I) < \[p{K_a}\]of Compound (II)
Hence, both the reason and assertion are true and are interdependent.
Option A is the correct
Note:
You should not fret over finding the value of ‘n’ in Huckle’s rule because ‘n’ is not a characteristic property of any molecule. It is merely a constant which can be substituted with any natural number to satisfy the Huckel's Rule.
Recently Updated Pages
Write a composition in approximately 450 500 words class 10 english JEE_Main

Arrange the sentences P Q R between S1 and S5 such class 10 english JEE_Main

What is the common property of the oxides CONO and class 10 chemistry JEE_Main

What happens when dilute hydrochloric acid is added class 10 chemistry JEE_Main

If four points A63B 35C4 2 and Dx3x are given in such class 10 maths JEE_Main

The area of square inscribed in a circle of diameter class 10 maths JEE_Main

Other Pages
A boat takes 2 hours to go 8 km and come back to a class 11 physics JEE_Main

In the ground state an element has 13 electrons in class 11 chemistry JEE_Main

Differentiate between homogeneous and heterogeneous class 12 chemistry JEE_Main

Electric field due to uniformly charged sphere class 12 physics JEE_Main

According to classical free electron theory A There class 11 physics JEE_Main

Excluding stoppages the speed of a bus is 54 kmph and class 11 maths JEE_Main