Answer
64.8k+ views
Hint We know Bernoulli equation relates the speed of the fluid at a point, the pressure at that point and the height of that point above the reference level. Bernoulli equation is given by:
$P + \rho gh + \dfrac{1}{2}\rho {v^2} = $ constant
Since height is the same for both A and B hence $\rho gh$ term can be neglected.
Complete Step by step solution
On applying Bernoulli equation separately for A and B we get,
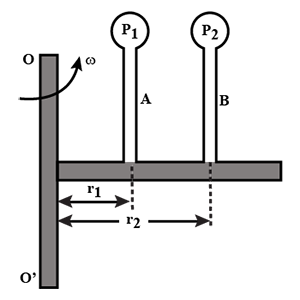
For A, ${p_1}$ is the reading shown in the barometer.
Hence, \[{p_1} + \rho gh + \dfrac{1}{2}\rho {v_1}^2 = \] constant…… (1)
For B, ${p_2}$ is the reading shown in the barometer.
Hence, \[{p_2} + \rho gh + \dfrac{1}{2}\rho v_2^2 = \] constant…… (2)
From equation (1) and (2) we get
\[
{p_1} + \rho gh + \dfrac{1}{2}\rho v_1^2 = {p_2} + \rho gh + \dfrac{1}{2}\rho v_2^2 \\
{p_1} + \dfrac{1}{2}\rho v_1^2 = {p_2} + \dfrac{1}{2}\rho v_2^2 \\
\]
Now we know that $v = r\omega $
Therefore, above equation becomes
\[
{p_1}\rho {({r_1}\omega )^2} = {p_2} + \dfrac{1}{2}\rho {({r_2}\omega )^2} \\
({p_2} - {p_1}) = \dfrac{1}{2}\rho ({r_1}^2 - {r_2}^2){\omega ^2} \\
\therefore {\omega ^2} = \dfrac{{2({p_2} - {p_1})}}{{\rho ({r_1}^2 - {r_2}^2)}} \\
\\
\]
$\omega = \sqrt {\dfrac{{2({p_2} - {p_1})}}{{\rho (r_1^2 - r_2^2)}}} $
Hence the required angular velocity is, $\omega = \sqrt {\dfrac{{2({p_2} - {p_1})}}{{\rho (r_1^2 - r_2^2)}}} $
Option (A) is correct.
Note Bernoulli equation is just the application of work-energy theorem in the case of fluid flow. In the Bernoulli equation we make a few assumptions like the fluid is ideal i.e. incompressible and nonviscous, it has constant density, both points lie on a streamline, flow is steady and there is no friction.
$P + \rho gh + \dfrac{1}{2}\rho {v^2} = $ constant
Since height is the same for both A and B hence $\rho gh$ term can be neglected.
Complete Step by step solution
On applying Bernoulli equation separately for A and B we get,
For A, ${p_1}$ is the reading shown in the barometer.
Hence, \[{p_1} + \rho gh + \dfrac{1}{2}\rho {v_1}^2 = \] constant…… (1)
For B, ${p_2}$ is the reading shown in the barometer.
Hence, \[{p_2} + \rho gh + \dfrac{1}{2}\rho v_2^2 = \] constant…… (2)
From equation (1) and (2) we get
\[
{p_1} + \rho gh + \dfrac{1}{2}\rho v_1^2 = {p_2} + \rho gh + \dfrac{1}{2}\rho v_2^2 \\
{p_1} + \dfrac{1}{2}\rho v_1^2 = {p_2} + \dfrac{1}{2}\rho v_2^2 \\
\]
Now we know that $v = r\omega $
Therefore, above equation becomes
\[
{p_1}\rho {({r_1}\omega )^2} = {p_2} + \dfrac{1}{2}\rho {({r_2}\omega )^2} \\
({p_2} - {p_1}) = \dfrac{1}{2}\rho ({r_1}^2 - {r_2}^2){\omega ^2} \\
\therefore {\omega ^2} = \dfrac{{2({p_2} - {p_1})}}{{\rho ({r_1}^2 - {r_2}^2)}} \\
\\
\]
$\omega = \sqrt {\dfrac{{2({p_2} - {p_1})}}{{\rho (r_1^2 - r_2^2)}}} $
Hence the required angular velocity is, $\omega = \sqrt {\dfrac{{2({p_2} - {p_1})}}{{\rho (r_1^2 - r_2^2)}}} $
Option (A) is correct.
Note Bernoulli equation is just the application of work-energy theorem in the case of fluid flow. In the Bernoulli equation we make a few assumptions like the fluid is ideal i.e. incompressible and nonviscous, it has constant density, both points lie on a streamline, flow is steady and there is no friction.
Recently Updated Pages
Write a composition in approximately 450 500 words class 10 english JEE_Main

Arrange the sentences P Q R between S1 and S5 such class 10 english JEE_Main

What is the common property of the oxides CONO and class 10 chemistry JEE_Main

What happens when dilute hydrochloric acid is added class 10 chemistry JEE_Main

If four points A63B 35C4 2 and Dx3x are given in such class 10 maths JEE_Main

The area of square inscribed in a circle of diameter class 10 maths JEE_Main

Other Pages
Excluding stoppages the speed of a bus is 54 kmph and class 11 maths JEE_Main

In the ground state an element has 13 electrons in class 11 chemistry JEE_Main

Electric field due to uniformly charged sphere class 12 physics JEE_Main

A boat takes 2 hours to go 8 km and come back to a class 11 physics JEE_Main

According to classical free electron theory A There class 11 physics JEE_Main

Differentiate between homogeneous and heterogeneous class 12 chemistry JEE_Main