Question

A thermodynamic process is shown in the figure. The pressure and volumes corresponding to some points in the figure are:
${{\text{P}}_{\text{A}}}=3\times {{10}^{4}}\text{Pa}$
$\mathrm{V}_{\mathrm{A}}=2 \times 10^{-3} \mathrm{m}^{-3}$
${{\text{P}}_{\text{B}}}=8\times {{10}^{4}}\text{Pa}$
$\mathrm{V}_{\mathrm{C}}=5 \times 10^{-3} \mathrm{m}^{-3}$
In the process A B, 600 J of heat is added to the system and in process B C, 200 J of heat is added to the system. The change in internal energy of the system in process AC would be

(A) 560 J
(B) 800 J
(C) 600 J
(D) 640 J

Answer 1

Hint: We know that thermodynamics is the branch of physics that deals with the relationships between heat and other forms of energy. In particular, it describes how thermal energy is converted to and from other forms of energy and how it affects matter. Traditionally, thermodynamics has stated three fundamental laws: the first law, the second law, and the third law. A more fundamental statement was later labelled the 'zeroth law'. The third law of thermodynamics states that a system's entropy approaches a constant value as the temperature approaches absolute zero. Entropy, the measure of a system's thermal energy per unit temperature that is unavailable for doing useful work. Because work is obtained from ordered molecular motion, the amount of entropy is also a measure of the molecular disorder, or randomness, of a system.

Complete step by step answer
We know that in physics and chemistry, the law of conservation of energy states that the total energy of an isolated system remains constant; it is said to be conserved over time. For instance, chemical energy is converted to kinetic energy when a stick of dynamite explodes. The principle of the conservation of energy refers to the idea that energy is not created or lost – it is only transformed from one form to another. In all changes some energy is always converted into forms (mainly low-grade heat) that cannot be used to make further changes. This law is very important because it is a very easy way to figure out important information about an object. For example, if you know an object's mass and initial height, you can find its initial potential energy, which is all the energy that it starts with.
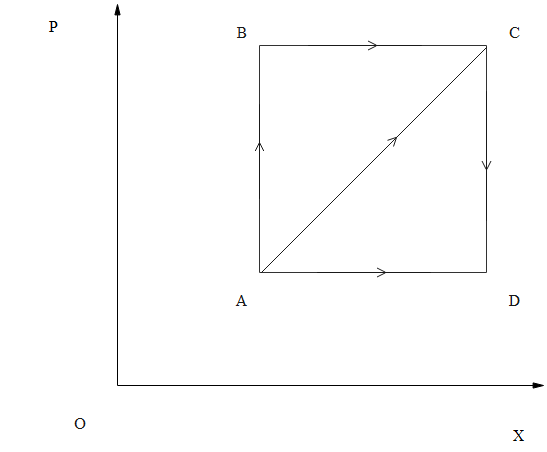
No work is done during the isochoric process $\mathrm{A} \rightarrow \mathrm{B}$.
Work done during isobaric process $\mathrm{B} \rightarrow \mathrm{C}=\mathrm{P}_{\mathrm{B}}\left(\mathrm{V}_{\mathrm{C}}-\mathrm{V}_{\mathrm{B}}\right)$
$=\mathrm{P}_{\mathrm{B}}\left(\mathrm{V}_{\mathrm{D}}-\mathrm{V}_{\mathrm{A}}\right)=240 \mathrm{J}$
From conservation of energy, $\mathrm{Q}=\mathrm{U}+\mathrm{W}$
$\Rightarrow \text{U}=\text{Q}-\text{W}=(600+200)\text{J}-240\text{J}=560\text{J}$

Hence the correct answer is option A.

Note: We know that an isobaric process is one in which a gas does work at constant pressure, while an isochoric process is one in which volume is kept constant. Because volume is constant in an isochoric process, no work is done. Because the volume change is zero in this case, the work done is zero. An example of the isobaric process includes the boiling of water to steam or the freezing of water to ice. In the process, a gas either expands or contracts to maintain constant pressure and hence the net amount of work is done by the system or on the system. An Isochoric process, also called a constant-volume process, an isovolumetric process, or an isometric process, is a thermodynamic process during which the volume of the closed system undergoing such a process remains constant.