Question

A smooth narrow tube in the form of an arc AB of a circle of centre O and radius $r$ is fixed so that A is vertically above O and OB is horizontal. Particles P of mass $m$ and Q of mass $2m$ with a light inextensible string of length $\left( {\pi r/2} \right)$ connecting them are placed inside the tube with P at A and Q at B and released from rest. Assuming the string remains taut during motion, find the speed of particles when P reaches B.

(A) $v = \sqrt {\dfrac{1}{3}\left( {1 + \pi } \right)gr} $
(B) $v = \sqrt {\dfrac{2}{3}\left( {1 + \pi } \right)gr} $
(C) $v = \sqrt {\dfrac{1}{3}\left( {2 + \pi } \right)gr} $
(D) $v = \sqrt {\dfrac{2}{3}\left( {2 + \pi } \right)gr} $

Answer 1

Hint: Potential energy is the energy by virtue of an object’s position relative to other objects and kinetic energy is the energy possessed by virtue of motion of a body. All surfaces of the narrow tube are smooth. As a result of this, the mechanical energy of the system will remain conserved.

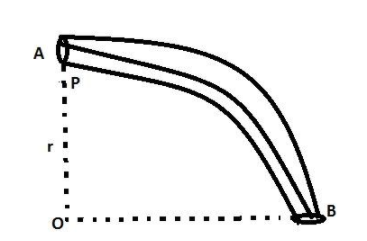
Complete Step by Step Solution: It has been given that a smooth narrow tube in the form of an arc AB of a circle of centre O and radius $r$ is fixed so that A is vertically above O and OB is horizontal. Particles P of mass $m$ and Q of mass $2m$ with a light inextensible string of length $\left( {\pi r/2} \right)$ connecting them are placed inside the tube with P at A and Q at B and released from rest.
All surfaces of the narrow tube are smooth. As a result of this, the mechanical energy of the system will remain conserved.
Mechanical energy is the total amount of kinetic energy and potential energy of an object that is used to do a specific work. Mechanical energy can also be defined as the energy of an element due to its position or motion or both. The potential energy of an object is due to its position and kinetic energy is due to its motion. The potential energy of an object is zero when it is in the movement and kinetic energy is zero when the object is in rest.
$\therefore $ Decrease in PE of both the blocks =increase in KE of both blocks.
When P reaches B it has lost height = $r$
and B will lose height = $\left( {\pi r/2} \right)$ where $\left( {\pi r/2} \right)$ is the length of the string.
The decrease in potential energy of the blocks is thus given by, $mgr + 2mg\left( {\pi r/2} \right)$.
The increase in kinetic energy is given by, $\dfrac{1}{2}\left( {m + 2m} \right){v^2}$.
Now we know, $mgr + 2mg\left( {\pi r/2} \right) = \dfrac{1}{2}\left( {m + 2m} \right){v^2}$
Thus, the velocity of particles when P reaches B is, ${v^2} = \dfrac{{2mgr\left( {1 + \pi } \right)}}{{3m}} = \dfrac{2}{3}\left( {1 + \pi } \right)gr$.
Taking root of the equation on both sides, we get,
$v = \sqrt {\dfrac{2}{3}\left( {1 + \pi } \right)gr} $.

Hence, the correct answer is Option B.

Note: All the forms of energy follow the law of conservation of energy. In brief, the law of conservation of energy states that, in a closed system, i.e., a system that is isolated from its surroundings, the total energy of the system is conserved.
So in an isolated system such as the universe, if there is a loss of energy in some part of it, there must be a gain of an equal amount of energy in some other part of the universe. Although this principle cannot be proved, there is no known example of a violation of the law of conservation of energy.