Question

A mt is going up. The total mass of the lift and the passenger is \[1500kg\]. The variation in the speed of the mt is as given in the graph. The tension in the rope pulling the lift at \[t = {11^{th}}\sec \] will be:

\[(A)17400N\]
\[(B)14700N\]
\[(C)12000N\]
$(D){\text{zero}}$

Answer 1

Hint: Calculate the acceleration of the lift using the graph as we know the acceleration is nothing but the change of the velocities with time. Draw a free body diagram and find the relation between the forces due to the tension of the rope and the motion of the lift. From the given values we will get the tension of the rope.

Formula used:
The acceleration, $a = \dfrac{{{v_2} - {v_1}}}{t}$
The equation we get from the acting forces on the lift, $mg = ma + T$
$m$ is the total mass of lift and the person, $g$ is the gravitational acceleration, and $T$ is the tension of the rope.

Complete step by step answer:
The lift is going up with a person, so the acceleration is working upward.
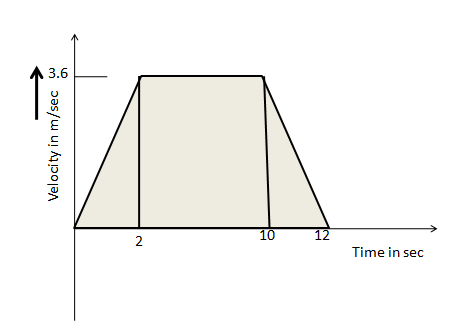
The value of the acceleration at \[t = {11^{th}}\sec \] can be found from the below graph,

$a = \dfrac{{{v_2} - {v_1}}}{t} = \dfrac{{3.6 - 0}}{2}$
$ \Rightarrow a = 1.8m/{s^2}$
Now if we draw a free body diagram showing the force acting on the lift such as,

Let, the $m$ is the total mass of lift and the person and $T$ is the tension of the rope.
The equation can be written from the above diagram, $mg = ma + T$
Here the total force acting upward is, $(ma + T)$ and the force acting downward is $mg$ (the total weight of the lift and person).
Given, $m = 1500kg$
And we know, the gravitational acceleration $g = 9.8m/{s^2}$
$\therefore mg = ma + T$
$ \Rightarrow T = m(g - a)$
$ \Rightarrow T = 1500(9.8 - 1.8)$
$ \Rightarrow T = 1500 \times 8$
$ \Rightarrow T = 12000N$
Hence, the tension in the given rope pulling of the lift at \[t = {11^{th}}\sec \] will be $ \Rightarrow T = 12000N$.

Hence, the correct answer is in the option $(C)$.

Note: Generally when a lift is going up and a person is inside the lift, the acceleration works towards the upward direction. In this case, the relative weight is greater than the actual weight, hence the person inside the lift feels heavier.
And, when a lift is going down, the acceleration works towards the downward direction. In this case, the relative weight is lesser than the actual weight, hence the person inside the lift feels lighter.