Question

A mass M is suspended by two springs of force constants \[{K_1}\] and \[{K_2}\] respectively as shown in the diagram. The total elongation (stretch) of the two springs is

A. \[\dfrac{{Mg}}{{{K_1} + {K_2}}} \\ \]
B. \[\dfrac{{Mg\left( {{K_1} + {K_2}} \right)}}{{{K_1}{K_2}}} \\ \]
C. \[\dfrac{{Mg\left( {{K_1}{K_2}} \right)}}{{{K_1} + {K_2}}} \\ \]
D. \[\dfrac{{{K_1} + {K_2}}}{{{K_1}{K_2}Mg}}\]

Answer 1

Hint: Force on the system will be a product of effective constant for the series combination and elongation in the spring.

Formula used:
The expression of restoring force is,
\[F = Kx\]
Where, F = Force, k= Spring constant and x = Elongation (stretch) of the spring.

Complete step by step solution:
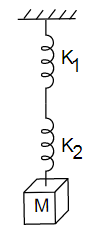
Given here is a spring mass system of two springs having constant \[{K_1}\] and \[{K_2}\] respectively. Springs are combined together in series combination and a mass M is suspended by the springs, we have to find the elongation in springs.

First we need to find the effective constant for the given combination of springs and it will be,
\[{K_{eff}} = \dfrac{{{K_1}{K_2}}}{{{K_1} + {K_2}}}\,.........(1)\]
Let the elongation in spring be x then free body diagram of suspended mass M will be,

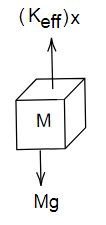
Image: Free body diagram of mass M

From free body diagram we have,
\[{K_{eff}}x = Mg \\
\Rightarrow x = \dfrac{{Mg}}{{{K_{eff}}}}\,.......(2)\]
Substituting value of \[{K_{eff}}\] form equation (1) in equation (2) we get,
\[x = \dfrac{{Mg\left( {{K_1} + {K_2}} \right)}}{{{K_1}{K_2}}}\,\]
Hence, elongation in the spring will be \[\dfrac{{Mg\left( {{K_1} + {K_2}} \right)}}{{{K_1}{K_2}}}\,\].

Therefore, option B is the correct answer.

Note: Even though in combination of springs we have multiple springs connected to each other either in series or parallel, they behave like a single spring and to solve numerical problems for combination of springs their effective constant is to be calculated first.