Question

A magnetic needle suspended parallel to the magnetic field requires √3 J of work to turn it through 60°. What will be the torque needed to maintain the needle in this position?
(A) 3 J
(B) √3 J
(C) 3/2 J
(D) 2√3 J

Answer 1

Hint The needle of a compass is itself a magnet and thus the north pole of the magnet always points north, except when it is near a strong magnet or in a magnetic field. When you bring the compass near a strong magnetic field, the needle of the compass points in the direction of the south pole of the bar magnet.
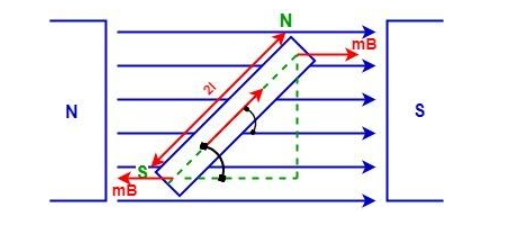
In a uniform magnetic field, the two poles of the magnetic needle experience equal and opposite forces. In other words, the force at one end nullifies the force at the other end. Hence, the needle experiences only the torque due to the magnetic field and there is no force. Torque is the measure of the force that can cause an object to rotate about an axis.
Formula used: Torque in vector form is given as:
τ = M Χ B
B is the magnetic field and M is the magnetic dipole moment.
Magnetic dipole moment is the product of strength of pole (m) and the magnetic length (2l) of the magnet.
M = m (2l)

Complete step by step solution
When a magnetic dipole moment M is held at an angle θ with the direction of a uniform magnetic field B, the magnitude of torque acting on the dipole is
= Μ Χ Β
τ=MBsin
This torque tends to align the dipole in the direction of the field. Wok has to be done in rotating the dipole against the action of the torque. This work is stored in the magnetic dipole as potential energy of the dipole. Now small amount of work done in rotating the dipole through a small angle dθ is:
dW= dθ
dW=MBsinθ


\[\begin{align}
  & \text{Total work done in rotating the dipole from }\theta ={{\theta }_{1\,}}\text{ to }\theta \text{=}{{\theta }_{2\ }}\text{ is:} \\
 & W=\int\limits_{{{\theta }_{1}}}^{{{\theta }_{2}}}{MB\sin \theta d\theta } \\
 & =MB\left( -\cos {{\theta }_{2}}+\cos {{\theta }_{1}} \right) \\
 & =-MB(\cos {{\theta }_{2}}-\cos {{\theta }_{1}}) \\
 & \text{Therefore, potential energy of the dipole is:} \\
 & U=W \\
 & =-MB(\cos {{\theta }_{2}}-\cos {{\theta }_{1}})\text{ }....\text{ (}i) \\
 & \text{In our case, it is given that U=}\sqrt{3}J \\
\end{align}\]
$\begin{align}
  & \text{and }{{\theta }_{1}}=0{}^\circ \\
 & {{\theta }_{2}}=60{}^\circ \\
 & \text{putting these values in equation 1 we get;} \\
 & \sqrt{3}=-MB(\cos 60{}^\circ -\cos 0{}^\circ ) \\
 & =-MB\left( \frac{1}{2}-1 \right) \\
 & =\frac{MB}{2} \\
 & MB=2\sqrt{3} \\
 & \text{Torque required to hold at 60}{}^\circ \text{ is given by:} \\
 & \tau =2\sqrt{3}\sin 60{}^\circ \\
 & =2\sqrt{3}\times \frac{\sqrt{3}}{2} \\
 & =3\text{ J} \\
\end{align}$

Therefore, the 3 J torque is needed to maintain the needle in 60° position.

Note Knowledge of how torque changes the rotation of an object is important. We can find the potential energy of the dipole very easily and using potential energy concept we can easily find the value of torque. In this solution, we derived the formula for potential energy or work. It is not compulsory to derive this in every question and formula can be used directly.