Question

A magnet is moving into a coil of wire as shown, there is a small reading on the sensitive meter. Which change would increase the size of the reading?

A. Moving the south pole in
B. Pulling the magnet out
C. Pushing the magnet in faster
D. Unwinding some of the turns of wire

Answer 1

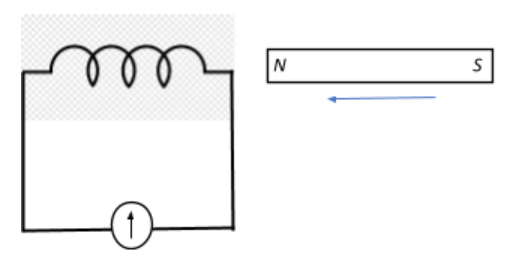
Hint: Think of why there is a small reading on the sensitive meter when a magnet is moved towards the coil. In other words, we know that current induced in a conductor as a result of change in magnetic flux is a consequence of Faraday’s second law of electromagnetic induction.
The law suggests that the magnitude of induced emf in a coil is equivalent to the rate of change of flux linkage between the coil and the magnet. Numerically express this law and deduce how the rate of change of flux and consequently the induced emf can be increased.

Formula used: Induced emf $\epsilon = -N\dfrac{\Delta \phi}{\Delta t}$, where $\epsilon$ is the emf induced, $\phi$ is the magnetic flux, and $N$ is the number of turns in the coil.

Complete answer:
The behaviour of an electric circuit under the influence of changing magnetic flux was analysed by Michael Faraday who formulated two laws to describe electromagnetic induction.
Faraday’s first law of electromagnetic induction states that an electromotive force (emf) is induced whenever a conductor is played in a varying magnetic field. This produces a current in the circuit of the conductor, and this current is called induced current.
Thus, in the context of our question we can establish that the small reading on the sensitive meter is justified due to the varying magnetic flux of the magnet on the coil as a result of the magnet’s motion towards the coil that produces an emf and an induced current.
Now, Faraday’s second law of electromagnetic induction states that the magnitude of induced emf in a coil is equivalent to the rate of change of flux linked with the coil. This is quantised as:
$\epsilon = -N\dfrac{\Delta \phi}{\Delta t}$, where $\epsilon$ is the emf induced, $\phi$ is the magnetic flux, and $N$ is the number of turns in the coil. Note that the negative sign indicates that the direction of induced emf and the change in the direction of magnetic fields have opposite signs.
From the above relation, we see that the emf produced will increase if the magnetic flux incident on the coil changes rapidly with time. This higher rate of change of magnetic flux is brought about by moving the magnet rapidly towards the wire. This causes an increase in the magnitude of the emf produced and this would increase the deflection in the sensitive meter.
Thus, the correct choice would be C. Pushing the magnet in faster.

Note: The other ways to experience a change in magnetic field besides moving the magnet towards the coil are by:
1. Moving the coil into or out of the magnetic field.
2. Changing the plane of the coil through which the magnet moves.
3. Rotating a coil relative to the magnet.
And the other ways to increase the emf induced in a coil besides having an increase in the relative speed of motion between the coil and magnet are by:
1. Increasing the number of turns in the coil
2. By increasing the strength of the magnetic field itself, which in turn increases the magnetic flux.