Answer
64.8k+ views
Hint: The dot is invisible, due to the phenomenon of total internal reflection. Total internal reflection takes place when the angle of incidence exceeds the critical angle.
Complete step by step answer:
Let us understand the concept of total internal Reflection from the following definitions:
The phenomenon of reflection of total light when the light travelling in a denser medium strikes the interface separating the denser and the rarer medium at an angle greater than the critical angle, this is referred to as total internal reflection.
Now, we come across a term, critical angle. This critical angle is the angle of incidence in the denser medium, for which the angle of refraction in the rarer medium becomes\[{90^o}\] .
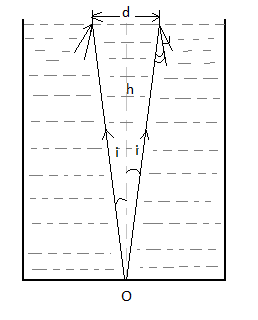
Now coming to the question, it is given that:
Height of the jar=h
Refractive index of the transparent medium is =\[\mu \]
We need to find the diameter of the ring, which when placed symmetrically on the top surface, the dot O at the bottom surface becomes invisible.
In order to make the dot invisible, rays marked OA and OB must suffer total internal Reflection.
Let \[\angle AOC\] be the angle of incidence marked as I in the diagram.
In\[\Delta AOC\] :
\[\tan i = \dfrac{{AC}}{{OC}} = \dfrac{{d/2}}{h}\]
Now, we know, for total internal reflection to occur, \[\angle i \geqslant \angle c\]
So, \[\sin i = \sin c\]
Applying Snell’s law:
We know,
\[\dfrac{{\sin i}}{{\sin r}} = \mu \]
Where, r= angle of refraction, which is \[{90^o}\] .
We know, if refractive index of media a with respect to b (air to water) is denoted by\[\mu \], then refractive index of b with respect to a ( water to air) is denoted by \[\dfrac{1}{\mu }\]
Therefore, the previous equation can also be written as:
\[\sin i = \dfrac{1}{\mu }\]
Drawing the triangle from the above equation:

\[\tan i = \dfrac{1}{{\sqrt {{\mu ^2} - 1} }}\]
Equating values of\[\tan i\] :
\[\dfrac{d}{{2h}} = \dfrac{1}{{\sqrt {{\mu ^2} - 1} }}\]
Solving the equation, we get:
\[d = \dfrac{{2h}}{{\sqrt {{\mu ^2} - 1} }}\]
This is the required value of diameter.
Note: Total internal reflection takes place when light ray travels from optically denser medium to the rarer medium. Here, (water-air surface). The rarer medium is the medium, in which the speed of light is more whereas the denser medium is the one where the speed of light is less.
Complete step by step answer:

Let us understand the concept of total internal Reflection from the following definitions:
The phenomenon of reflection of total light when the light travelling in a denser medium strikes the interface separating the denser and the rarer medium at an angle greater than the critical angle, this is referred to as total internal reflection.
Now, we come across a term, critical angle. This critical angle is the angle of incidence in the denser medium, for which the angle of refraction in the rarer medium becomes\[{90^o}\] .
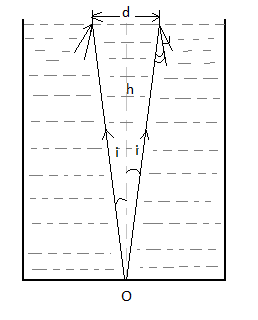
Now coming to the question, it is given that:
Height of the jar=h
Refractive index of the transparent medium is =\[\mu \]
We need to find the diameter of the ring, which when placed symmetrically on the top surface, the dot O at the bottom surface becomes invisible.
In order to make the dot invisible, rays marked OA and OB must suffer total internal Reflection.
Let \[\angle AOC\] be the angle of incidence marked as I in the diagram.
In\[\Delta AOC\] :
\[\tan i = \dfrac{{AC}}{{OC}} = \dfrac{{d/2}}{h}\]
Now, we know, for total internal reflection to occur, \[\angle i \geqslant \angle c\]
So, \[\sin i = \sin c\]
Applying Snell’s law:
We know,
\[\dfrac{{\sin i}}{{\sin r}} = \mu \]
Where, r= angle of refraction, which is \[{90^o}\] .
We know, if refractive index of media a with respect to b (air to water) is denoted by\[\mu \], then refractive index of b with respect to a ( water to air) is denoted by \[\dfrac{1}{\mu }\]
Therefore, the previous equation can also be written as:
\[\sin i = \dfrac{1}{\mu }\]
Drawing the triangle from the above equation:

\[\tan i = \dfrac{1}{{\sqrt {{\mu ^2} - 1} }}\]
Equating values of\[\tan i\] :
\[\dfrac{d}{{2h}} = \dfrac{1}{{\sqrt {{\mu ^2} - 1} }}\]
Solving the equation, we get:
\[d = \dfrac{{2h}}{{\sqrt {{\mu ^2} - 1} }}\]
This is the required value of diameter.
Note: Total internal reflection takes place when light ray travels from optically denser medium to the rarer medium. Here, (water-air surface). The rarer medium is the medium, in which the speed of light is more whereas the denser medium is the one where the speed of light is less.
Recently Updated Pages
Write a composition in approximately 450 500 words class 10 english JEE_Main

Arrange the sentences P Q R between S1 and S5 such class 10 english JEE_Main

What is the common property of the oxides CONO and class 10 chemistry JEE_Main

What happens when dilute hydrochloric acid is added class 10 chemistry JEE_Main

If four points A63B 35C4 2 and Dx3x are given in such class 10 maths JEE_Main

The area of square inscribed in a circle of diameter class 10 maths JEE_Main

Other Pages
A boat takes 2 hours to go 8 km and come back to a class 11 physics JEE_Main

Electric field due to uniformly charged sphere class 12 physics JEE_Main

In the ground state an element has 13 electrons in class 11 chemistry JEE_Main

According to classical free electron theory A There class 11 physics JEE_Main

Differentiate between homogeneous and heterogeneous class 12 chemistry JEE_Main

Excluding stoppages the speed of a bus is 54 kmph and class 11 maths JEE_Main