Question

A combination of five resistors is connected to a cell of emf 10 V the potential difference \[{V_B} - {V_E}\] will be

A) +5 V
B) +3 V
C) +1 V
D) Zero

Answer 1

Hint: In this solution, we will determine the net resistance of the circuit. Then we will find the net current in the circuit using Kirchhoff’s law and then calculate the potential difference across B and E. The centre resistance will form a Wheatstone bridge and won’t contribute to the resistance of the circuit.
Formula used: In this solution, we will use the following formula:
1- Ohm’s law: $V = IR$ where $V$ is the potential difference between two points between which current $I$ is flowing through a resistor $R$

Complete step by step answer:
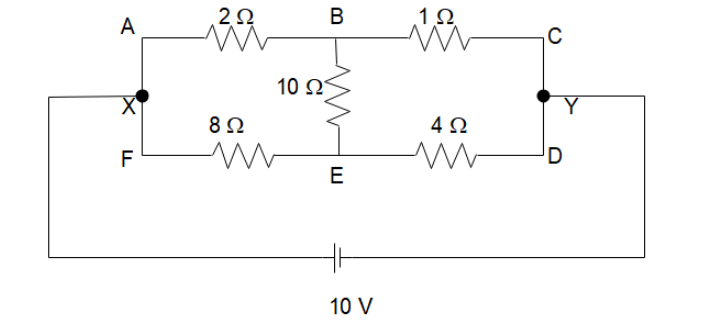
IN the circuit given to us, the ratio of resistances formed by the resistors on top of each other with their side-by-side counterpart will be $2:1\,{\text{and}}\,{\text{8:4}}$. Since both the ratios are equivalent, the resistor in the middle will be the Wheatstone bridge and it won’t contribute to the circuit. This also implies that the potential difference between the points B and E will be zero. So, the current will not pass through the branch connecting B and E
Then, the current in the circuit can be found out a follows:
The \[2\Omega ,1\Omega \] resistors are connected in series as are the \[8\Omega \,\] and \[4\Omega \] resistors.
The individual net resistance of the top branch of resistors will be
$R = 2 + 1 = 3\,\Omega $
Similarly, the net resistance of the bottom branch will be
$R = 8 + 4 = 12\,\Omega $
These two net resistances will be in parallel i.e., the top branch and the bottom branch will be in parallel so the net resistance will be:
$\dfrac{1}{{{R_{net}}}} = \dfrac{1}{3} + \dfrac{1}{{12}}$
$ \Rightarrow {R_{net}} = 12/5\,\Omega $
We can find the current in the circuit using Kirchhoff’s law as
$I = \dfrac{V}{R}$
$ \Rightarrow I = \dfrac{{10}}{{12/5}}$
Which gives us
$I = 50/12\,A$
This current will flow the top and the bottom branches separately and no current will flow between points B and E. So, the net potential difference across the two points according to the relationship $V = IR$ will also be zero.

So, option (D) is the correct choice.

Note: The value of the potential in the branch connecting B and E is inconsequential since the conditions of a Wheatstone bridge depend on the points around the Wheatstone bridge and not on the resistor in the branch itself. So, even if the resistance of $10\,\Omega $ changes, there will be no current flowing through the branch.