Answer
64.8k+ views
Hint:
1.Potential difference is rising or changing with time. And current is constant, therefore, circuit elements can’t be resistance and inductance.
2. Potential difference is changing so the battery emf can’t be constant 6 voltage. It should be time varying.
Formula Used:
1.For a purely resistive circuit element. we know, V=IR …… (1)
Where,
V = voltage across resistor
R=resistance in the circuit
I = current in the circuit
2. For a purely inductive circuit we know, $ - I\dfrac{{di}}{{dt}} = Voltage$ …… (2)
Where,
I = inductance across the inductor
V = Voltage across inductive coil
3. For a purely capacitive circuit we know, $\dfrac{{dq}}{{dt}} = C\dfrac{{dV}}{{dt}}$ $ \Rightarrow \dfrac{i}{C} = \dfrac{{dV}}{{dt}}$ …… (3)
Where,
Q = charge stored in capacitor
V = Voltage across plates
i = current in the circuit
C= capacitance
Complete step by step answer:
Let’s find the correct by rejecting the wrong choices: Hit and trial approach:
Step 1:
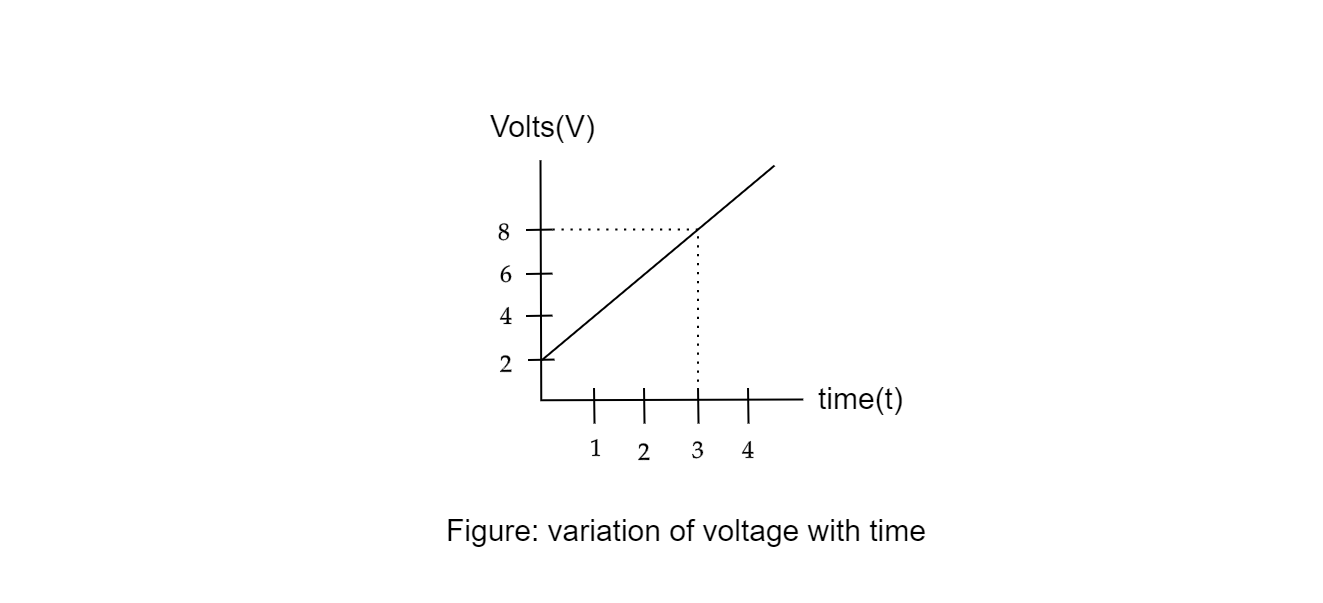
From equation (1) we can say with time, Voltage would be constant. Since there is no time term in it. Therefore, the above graph should have 0 slope. But slope is non zero. So, it can’t be a resistor.
\[ \Rightarrow \]Hence, option (a) Not Possible
Step 2:
Given Voltage is time varying with constant slope. But in option (b) it says emf is constant= 6V
\[ \Rightarrow \]Hence, option (b) Not Possible
Step 3:
From equation (2), we can say with time, current is also changing. But in question, it is given current is constant and equals 1 amp. Therefore, circuits can’t be inductive.
\[ \Rightarrow \]option (b) Not Possible
Step 4:
From equation (3), we can say with time Voltage is varying and current is constant. Therefore, conditions satisfied for a capacitive circuit. So, Circuit is capacitive.
Step 5:
From the graph, we can see the graph is constant. So, slope of graph=$\dfrac{i}{C} = \dfrac{{dV}}{{dt}}$
Evaluating slope of graph using 2-point slope form we get,
$\dfrac{i}{C} = \dfrac{{{y_2} - {y_1}}}{{{t_2} - {t_1}}} = \dfrac{{8 - 2}}{{3 - 0}} = 2$ where $({x_1},{y_1})$ and $({x_2},{y_2})$ are two separate points ……. (4)
Step 6:
Substitute value of current i=1 amp, in equation (4) we get,
$\dfrac{1}{2} = C \Rightarrow C = 0.5F$ required capacitance of circuit.
Final Answer
Hence, option (d) capacitance of 0.5F
Note: Sometimes information given from a question is too less to decide how to proceed toward solution of the problem. Therefore, one should find what not to do first using Hit and trial approach. Also one should get an intuition that as current is constant basically with time more and more charge is provided to the circuit element. As capacitance is constant(only depends on the geometry of the capacitor) the charge to voltage ratio should be constant and as charge increases potential difference should increase too.
1.Potential difference is rising or changing with time. And current is constant, therefore, circuit elements can’t be resistance and inductance.
2. Potential difference is changing so the battery emf can’t be constant 6 voltage. It should be time varying.
Formula Used:
1.For a purely resistive circuit element. we know, V=IR …… (1)
Where,
V = voltage across resistor
R=resistance in the circuit
I = current in the circuit
2. For a purely inductive circuit we know, $ - I\dfrac{{di}}{{dt}} = Voltage$ …… (2)
Where,
I = inductance across the inductor
V = Voltage across inductive coil
3. For a purely capacitive circuit we know, $\dfrac{{dq}}{{dt}} = C\dfrac{{dV}}{{dt}}$ $ \Rightarrow \dfrac{i}{C} = \dfrac{{dV}}{{dt}}$ …… (3)
Where,
Q = charge stored in capacitor
V = Voltage across plates
i = current in the circuit
C= capacitance
Complete step by step answer:
Let’s find the correct by rejecting the wrong choices: Hit and trial approach:
Step 1:
From equation (1) we can say with time, Voltage would be constant. Since there is no time term in it. Therefore, the above graph should have 0 slope. But slope is non zero. So, it can’t be a resistor.
\[ \Rightarrow \]Hence, option (a) Not Possible
Step 2:
Given Voltage is time varying with constant slope. But in option (b) it says emf is constant= 6V
\[ \Rightarrow \]Hence, option (b) Not Possible
Step 3:
From equation (2), we can say with time, current is also changing. But in question, it is given current is constant and equals 1 amp. Therefore, circuits can’t be inductive.
\[ \Rightarrow \]option (b) Not Possible
Step 4:
From equation (3), we can say with time Voltage is varying and current is constant. Therefore, conditions satisfied for a capacitive circuit. So, Circuit is capacitive.
Step 5:
From the graph, we can see the graph is constant. So, slope of graph=$\dfrac{i}{C} = \dfrac{{dV}}{{dt}}$
Evaluating slope of graph using 2-point slope form we get,
$\dfrac{i}{C} = \dfrac{{{y_2} - {y_1}}}{{{t_2} - {t_1}}} = \dfrac{{8 - 2}}{{3 - 0}} = 2$ where $({x_1},{y_1})$ and $({x_2},{y_2})$ are two separate points ……. (4)
Step 6:
Substitute value of current i=1 amp, in equation (4) we get,
$\dfrac{1}{2} = C \Rightarrow C = 0.5F$ required capacitance of circuit.
Final Answer
Hence, option (d) capacitance of 0.5F
Note: Sometimes information given from a question is too less to decide how to proceed toward solution of the problem. Therefore, one should find what not to do first using Hit and trial approach. Also one should get an intuition that as current is constant basically with time more and more charge is provided to the circuit element. As capacitance is constant(only depends on the geometry of the capacitor) the charge to voltage ratio should be constant and as charge increases potential difference should increase too.
Recently Updated Pages
Write a composition in approximately 450 500 words class 10 english JEE_Main

Arrange the sentences P Q R between S1 and S5 such class 10 english JEE_Main

What is the common property of the oxides CONO and class 10 chemistry JEE_Main

What happens when dilute hydrochloric acid is added class 10 chemistry JEE_Main

If four points A63B 35C4 2 and Dx3x are given in such class 10 maths JEE_Main

The area of square inscribed in a circle of diameter class 10 maths JEE_Main

Other Pages
A boat takes 2 hours to go 8 km and come back to a class 11 physics JEE_Main

Electric field due to uniformly charged sphere class 12 physics JEE_Main

In the ground state an element has 13 electrons in class 11 chemistry JEE_Main

According to classical free electron theory A There class 11 physics JEE_Main

Differentiate between homogeneous and heterogeneous class 12 chemistry JEE_Main

Excluding stoppages the speed of a bus is 54 kmph and class 11 maths JEE_Main