Question

A bead can slide on a smooth straight wire and a particle of mass $\mathrm{m}$ is attached to the bead by light string of length L. The particle is held in contact with the wire with the string taut and then let fall. The bead has mass $2 \mathrm{m}$. When the string makes an angle $\theta$ with the wire find the distance moved by the bead.

(A) $L(1-\cos \theta )$
(B) $\dfrac{L}{2}(1-\cos \theta )$
(C) $\dfrac{L}{3}(1-\cos \theta )$
(D) $\dfrac{L}{6}(1-\cos \theta )$

Answer 1

Hint: We know that momentum is a physics term that refers to the quantity of motion that an object has. A sports team that is on the move has the momentum. If an object is in motion (on the move) then it has momentum. One example is the use of air bags in automobiles. Air bags are used in automobiles because they are able to minimize the effect of the force on an object involved in a collision. Air bags accomplish this by extending the time required to stop the momentum of the driver and passenger. Momentum is mass in motion, and any moving object can have momentum. An object's change in momentum is equal to its impulse. Impulse is a quantity of force times the time interval. Impulse is not equal to momentum itself; rather, it's the increase or decrease of an object's momentum. Based on this concept we have to solve this question.

Complete step by step answer
Given,
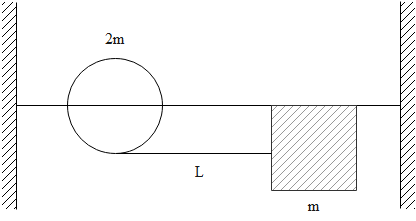
A particle having mass $=\mathrm{m}$
length $=\mathrm{L}$
Mass of the bead is $2 \mathrm{m}$
since no force acts on the horizontal direction
So, the center of mass the beard and the particle should remain at the same x-coordinate.
Since no force acts on the horizontal direction.
So, the center of mass the beard and the particle should remain at the same x-coordinate.
We have a mass of bead is $2 \mathrm{m}$ and make an angle $\theta$ with the wire Initial x-coordinate of the center of mass from the original position of the bead is:

$=\dfrac{2 \mathrm{m} \times 0+\mathrm{m} \times 1}{3}$

$=\dfrac{\mathrm{L}}{3 \mathrm{m}} \ldots 1$

Now the final position of the center of mass if bead has moved by a distance:

$=\dfrac{[2 \mathrm{mx}+\mathrm{m}(\mathrm{x}+\mathrm{Lcosa})]}{3 \mathrm{m}}$

$=\dfrac{3 \mathrm{x}+\mathrm{Lcosa}}{3 \mathrm{m}} \ldots 2$

By solving equation 1 and 2 then we get,

$3 x+L \cos a=L$

$\mathrm{x}=\dfrac{\mathrm{L}(1-\cos \mathrm{a})}{3}$

Hence the correct answer is option C.

Note: We should know that linear momentum is defined as the product of a system's mass multiplied by its velocity. In symbols, linear momentum is expressed as$p=mv$. Momentum is directly proportional to the object's mass and also its velocity. Thus, the greater an object's mass or the greater its velocity, the greater its momentum. Note that the linear moment is a vector quantity and is conserved in any direction. In the center-of-mass system, the total momentum is always zero, before and after the interaction, in any direction. Angular momentum is inertia of rotation motion. Linear momentum is inertia of translation motion. The big difference is that the type of motion which is related to each momentum is different. It is important to consider the place where the force related to rotation applies, which is appears as 'r' in the formula