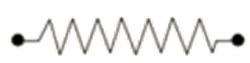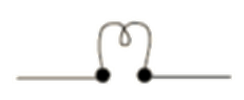CBSE Class 10 Chapter 11 Electricity Notes Class 10: FREE PDF Downloads






Electricity Class 10 Notes: CBSE Science Chapter 11
FAQs on Electricity Class 10 Notes: CBSE Science Chapter 11
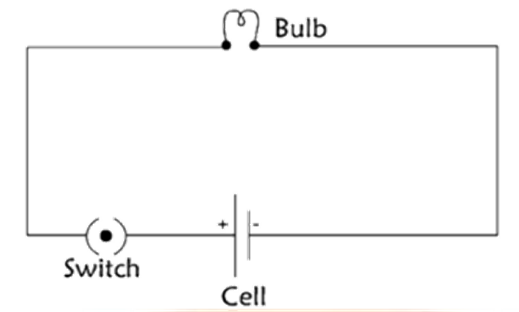
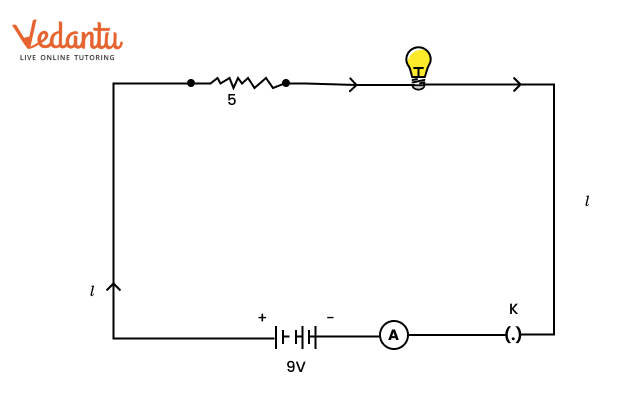
Q1. How can Anyone Make a Simple Circuit?
Ans: Students need to follow these given steps to construct a circuit.
One end of the wire should be connected to the light bulb holder.
In the negative end of the battery, connect the free-end wire.
The other end of the wire should be connected to the positive end of the battery.
Q2. What is the Heating Effect of Current?
Ans: As per Joule’s law, heat is directly proportional to the square of the current, directly proportional to the resistance of a given circuit, and heat is directly proportional to time for which current flows through the conductor.
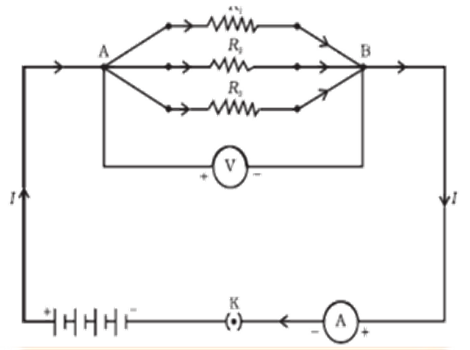
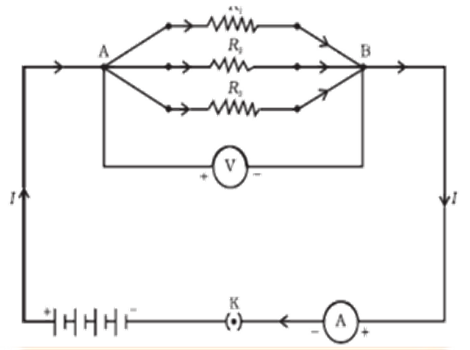
Q3. What are the Types of Combinations of Resistors?
Ans: There are two types of combinations of resistors such as series and parallel.
In Series: The resistors carry the same current.
In Parallel: The resistors have the same potential difference.
Q4. Why Should you Choose Vedantu as your Learning Partner?
Ans: Vedantu has some significant features that are helpful for students. These features are as follows.
Time - saving learning skills.
Declaration about the decent result of a student.
Shape an assertive and robust concentration about the exam.
Enter anytime to our website and read from wherever you are either on your mobile or laptop.
Q5. What is electricity according to Class 10 Chapter 11 notes?
Electricity has been defined as the presence or rate of flow of electric charges in a conductor like copper wires. Thales, a well-known Greek Philosopher, observed the presence of an attractive capacity in certain materials when they were rubbed together. These two materials were later classified as positive and negative charges. Students can find a more detailed explanation in the Electricity Class 10 Notes Chapter 11 Science provided by Vedantu.
Q6. What is resistance according to Class 10 Science Chapter 11?
Resistance, as explained in Class 10 Science Chapter 11, is known as the opposition that is faced by the flow of current in an electric circuit. The amount of resistance depends on the following features of a particular material:
Resistance is directly proportional to the length, nature, and temperature of the conductor.
- Resistance and the conductor's cross-sectional area are inversely proportional to each other.
Q7. What are the topics covered in Class 10 Science Chapter 11 notes?
The Electricity Class 10 Notes Chapter 11 Science covers all the topics that are a part of the NCERT book and the latest syllabus provided by CBSE. These topics include:
Atomic Structure
Electric Charge
Usage of Conductors and Insulators
Definition of Electric Potential and Potential Difference
Electric Current Models
Model of Electron Sea
Electron’s Drift Velocity
Working of Battery
Electric Circuit
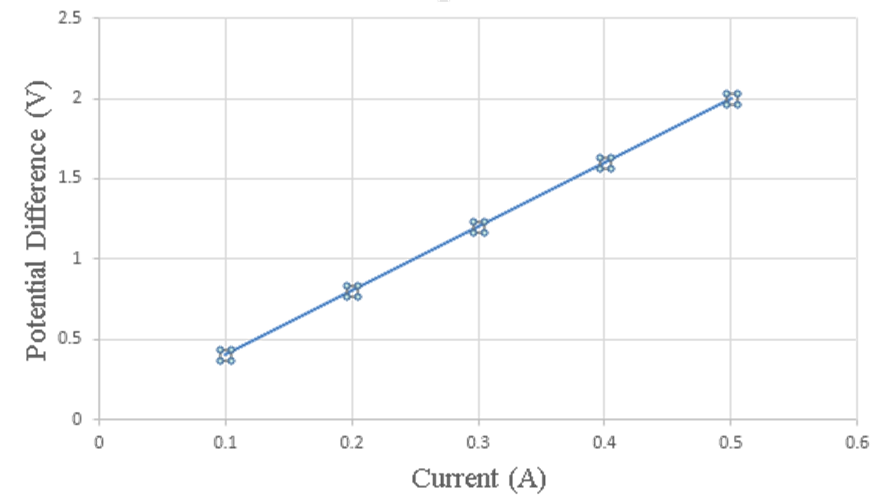
Ohm’s Law
Resistance and Resistivity
Q8. Define an electric circuit.
According to the CBSE Notes for Class 10 Science Chapter 10, a closed-loop path that has the presence of current traveling through it is called an electric circuit. The closed-loop provides the flowing current a return path, helping it go from one terminal of the power source to the other terminal. A full electric circuit can be represented with the help of circuit diagrams drawn using symbols for each component of the circuit.
Q9. Why should I refer to Vedantu CBSE Notes for Class 10 Science Chapter 11?
Physics in particular can be the branch of science that some students may find difficult to study. With the help of CBSE Notes for Class 10 Physics, understanding the concepts taught in Chapter 11 will become easier for students. Vedantu provides notes free of cost that have been prepared by expert and skilled professionals in an easy-to-understand language to help students get a strong grip over the subject and its different chapters in Class 10. These notes are 100% reliable and are based on the latest CBSE guidelines.




















 Watch Video
Watch Video