NCERT Solutions for Maths Class 8 Chapter 9 Mensuration Exercise 9.1 - FREE PDF Download
FAQs on NCERT Solutions for Class 8 Maths Chapter 9 Mensuration Ex 9.1
1. Can I avail NCERT Solutions for Class 8 Maths Chapter 9Mensuration Exercise 9.1 online?
Yes, you can definitely avail NCERT Solutions for class 8 maths chapter Mensuration exercise 9.1 as well as other exercises online. It is available on Vedantu, India’s top online learning platform. It provides the free PDF of exercise-wise NCERT Solutions for 8 Maths Chapter 9 Mensuration. These solutions are really helpful while preparing for exams. These solutions are prepared by subject experts who have years of teaching experience and vast subject knowledge. Without any further delay, avail NCERT Solutions for Class 8 chapter 9 maths exercise 9.1.
2. What will students learn from class 8 maths Mensuration exercise 9.1?
Students will learn how to calculate areas of different shapes including a Trapezium, a general quadrilateral and a polygon. These are two-dimensional figures. Students will be taught how to solve problems based on such figures in class 8 math ex 9.1. For having a clear understanding of the various topics associated with the exercise, students must download the free PDF of maths class 8 chapter 9 exercise 9.1 available on Vedantu.
3. How can NCERT Solutions maths chapter Mensuration ex 9.1 class 8 ensure optimum revision?
NCERT Solutions for maths chapter Mensuration ex 9.1 class 8 is a great material for revision. The free PDF of NCERT Solutions for class 8 ch 9 maths ex 9.1 can be utilized to learn the chapter effectively at first place and then for revision during the exam. As it includes stepwise explanations for the exercise problems, it allows students to have a deeper understanding, which is important during exams. Vedantu’s NCERT Solutions for class 8 maths chapter Mensuration exercise 9.1 are easily available over the internet. Mensuration is an important chapter from the exam perspective. In order to revise and score well, students must download these solutions available on Vedantu.
4. What is required to find the area of a trapezium?
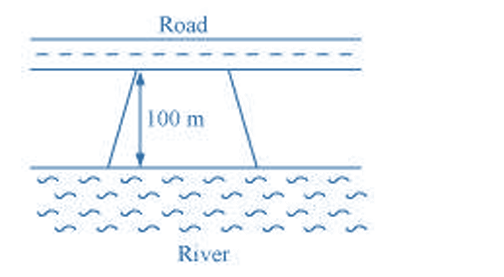
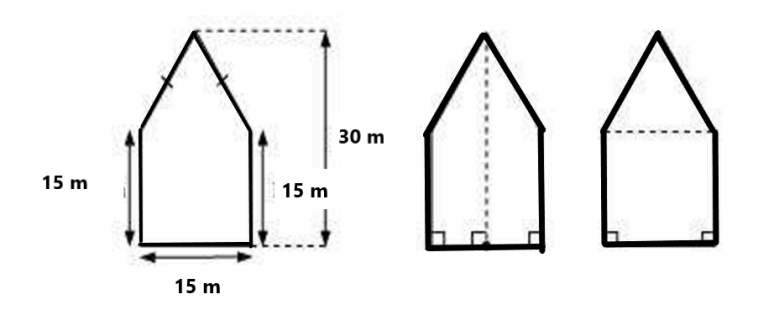
We need to know the length of the parallel sides and the perpendicular distance between these two parallel sides to find the area of a trapezium. The area of trapezium is given by half the product of the sum of the lengths of parallel sides and the perpendicular distance between them.
5. What is the total number of problems in Exercise 9.1 of Chapter 9 of Class 8 Maths?
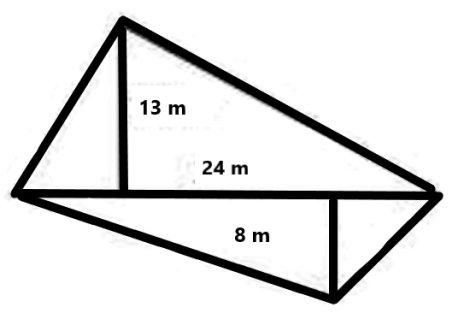
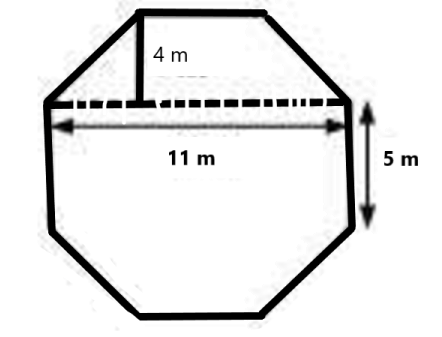
Exercise 9.1 of Chapter 9 Class 8 Maths contains 11 questions. These questions are based on the topics named “Area of a General Quadrilateral”, “Area of Special Quadrilaterals” and “Area of Polygon”. By solving these questions, students will get a brief introduction about the topics, and it will help in understanding them. The answers to the questions are available free of cost on the Vedantu website and the Vedantu app, in the form of a PDF file. They can take help from these solutions to clarify their doubts.
6. What is trapezium discussed in Exercise 9.1 of Chapter 9 of Class 8 Maths?
The topic “Trapezium” is discussed in Exercise 9.1 of Chapter 9 “Mensuration” of Class 8 Maths.
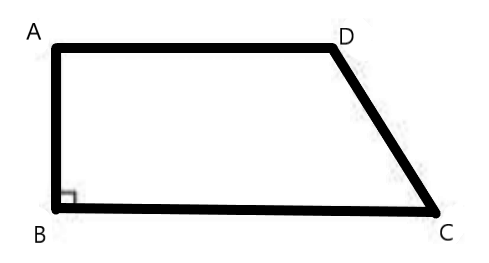
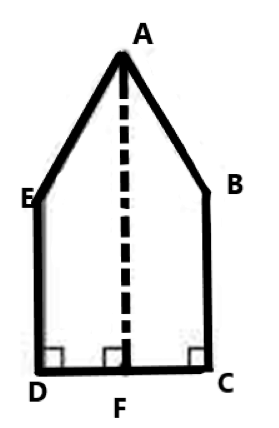
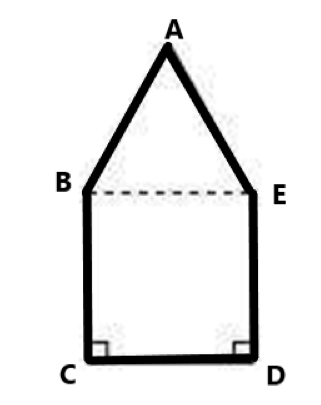
The quadrilateral whose two opposite sides are parallel and the other two sides are non-parallel is termed trapezium. This figure can be split into other two figures, i.e. triangle and rectangle. This splitting of the trapezium is helpful in finding its area.
The formula for finding the area of trapezium is:
Area of trapezium = ½ (a + b) * h
7. Note down some properties of trapezium which will be helpful in solving questions of Exercise 9.1 of Chapter 9 Class 8 Maths.
Some of the properties of a trapezium are discussed below:
There are three types of the trapezium. These are-
Isosceles Trapezium
Scalene Trapezium
Right Trapezium
The opposite sides or the bases are parallel to each other.
The diagonals are of equal length in the trapezium.
The intersecting property is shown by the diagonals of the trapezium.
The sum of the adjacent interior angles of the trapezium is 180 degrees.
The interior angles of the trapezium sum up to 360 degrees.
8. Where can I find the NCERT Solutions of Exercise 9.1 of Chapter 9 Class 8 Maths?
The NCERT Solutions of Exercise 9.1 of Chapter 9 Class 8 Maths are easily available on Vedantu. You just have to follow some steps to get them:
Visit the page NCERT Solutions for Exercise 9.1 of Chapter 9 of Class 8 Maths.
On the screen of your device, you will find that there are the NCERT Solutions of Exercise 9.1 of Chapter 9 Class 8 Maths PDF format.
Above this PDF file, visit the option of “Download PDF” to get the PDF file.
9. How can I attain satisfactory marks in Exercise 9.1 of Chapter 9 Class 8 Maths?
Chapter 9 “Mensuration” of Class 8 Maths requires lots of calculation. Students need to practice more in order to score well in Exercise 9.1 of Chapter 9 of Class 8 Maths. Firstly, read the theory and learn the formulas used in Exercise9.1. After that, solve each example and the questions of the exercise to have a better understanding of the concepts. Be careful while doing the calculation part. Check your answer twice after solving it.