




What is Real Depth and Apparent Depth - Exploring How Light Refraction Alters Our Perception
When light passes from one medium to another, such as from water to air, it bends or refracts. This refraction causes objects submerged in water to appear shallower than they are. The difference between the actual depth (real depth) and the perceived depth (apparent depth) is an important concept in optics. Understanding real depth and apparent depth not only helps in physics but also has practical applications in fields such as underwater exploration, photography, and even fishing. In this article, we’ll explore the causes of this optical phenomenon and how it affects our perception of underwater objects for JEE Main 2025.
What is Refraction and Refractive Index?
Refraction is a phenomenon in which there is bending of light when it propagates from one medium to the other. From Snell’s law, we know that
The incident ray, refracted ray and normal to the interface at the point of incidence lie in the same plane.
The ratio of sine of angle of incidence and sine of angle of refraction is constant.
Here, i is the angle of incidence and r is the angle of refraction.
n21 is the refractive index of the medium 2 with respect to 1.
When light travels from rarer to denser, the light bends towards the normal. When it travels from denser to rarer, the light bends away from the normal. The amount of bending is governed by Snell’s law. This concept was necessary to understand the concept of apparent depth. Next, we will be discussing the relation between real depth and apparent depth.
What is Real Depth and Apparent Depth?
Real Depth
Definition: Real depth can be defined as the depth at which an object is placed in a beaker or liquid such that the observer and object are in the same medium. The propagation of light from the observer to the object or vice versa should be in the same medium.
Factors Affecting Real Depth
Medium Density: The density of the medium, such as water or air, does not affect the real depth, but it can influence the force required to measure it. For example, measuring real depth in denser liquids might require different techniques due to buoyant forces.
Object's Position: The real depth of an object is influenced by its position in the medium (e.g., the distance from the surface). This factor is fundamental in calculating real depth.
Measurement Tool: The accuracy and method of measurement affect how the real depth is determined. Using instruments like depth gauges, sonar, or rulers provides the true measurement of real depth.
Water Level or Surface Variations: Changes in water level or surface due to tides, waves, or environmental factors can temporarily affect the measurement of real depth.
Gravitational Forces: In extreme cases, gravitational forces, such as in large bodies of water, can influence the vertical measurement of depth, but this effect is negligible in typical conditions.
Apparent Depth
Definition: Apparent depth can be defined as the depth of the object measured by the observer when the object and observer are in different media. The propagation of light from the observer to the object or vice versa should be in a different medium.
Factors Affecting Apparent Depth
Refractive Index of the Medium: The refractive index of the medium plays a crucial role in determining the apparent depth.
Angle of Observation: The apparent depth is influenced by the angle at which the observer looks at the object. When viewed directly from above (normal view), the apparent depth is less affected.
Object's Real Depth: The deeper the object is in the medium, the greater the difference between the real depth and the apparent depth. Objects at shallower depths are less affected by refraction compared to objects at greater depths.
Medium's Optical Properties: Different materials or mediums (such as air, water, glass) have different refractive indices.
Surface Curvature: If the surface of the medium is curved (like a curved water surface), the apparent depth can change due to the bending of light across the curved surface. This effect is often seen in lenses or curved water bodies.
Temperature of the Medium: Temperature can slightly change the refractive index of a medium, especially in water.
Real Depth and Apparent Depth Derivation
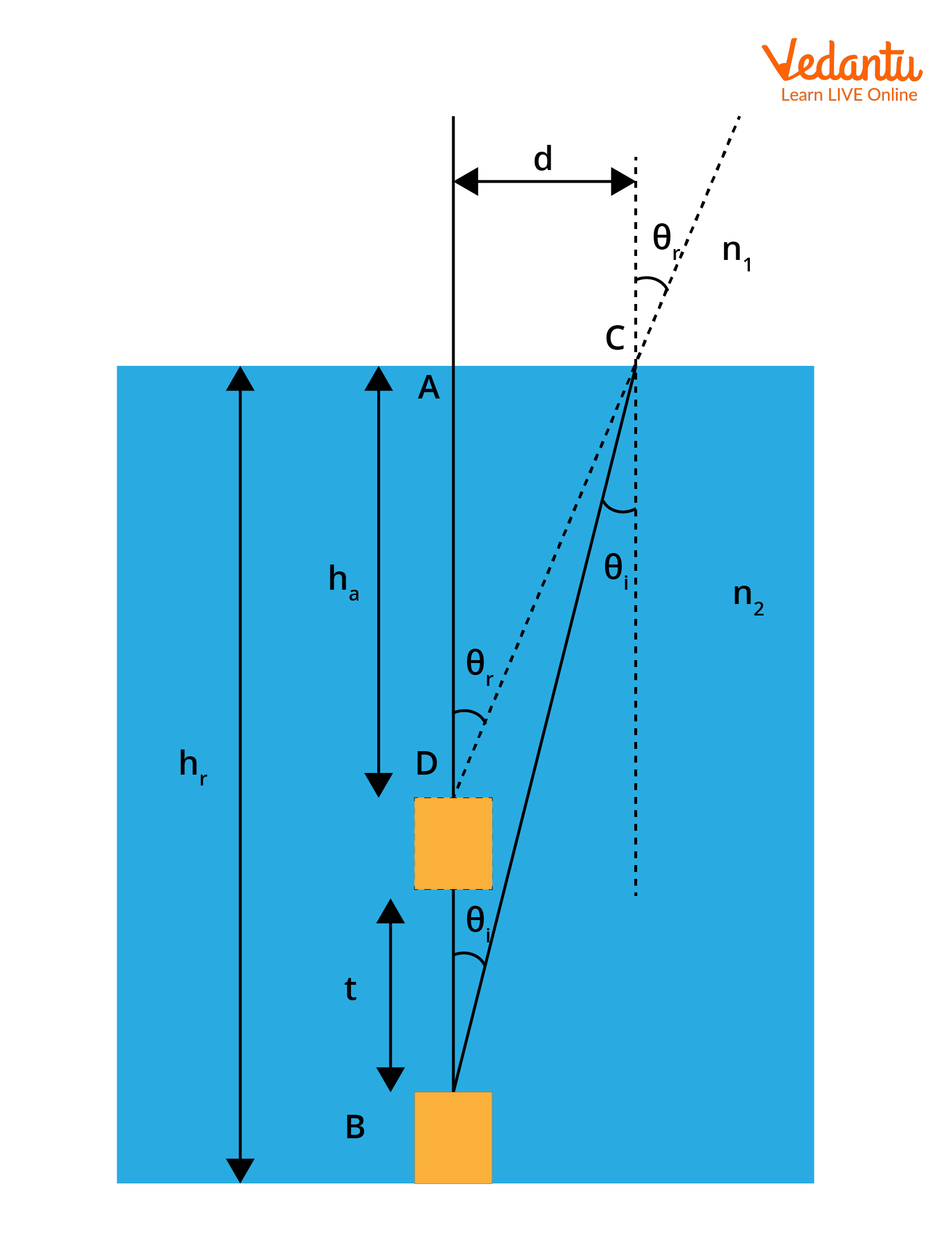
The real depth and apparent depth formula of refractive index can be mathematically derived. Consider a beaker filled with water and a square block placed at the bottom. Now, we measure the position of the square block in the empty glass, which will be the real depth given by the symbol ‘r’ in image 1. We will fill the beaker with a liquid and observe the apparent depth given by ha
The apparent depth will be given as
ha=hr-t.
For
For
From Snell’s law, we know
We will be assuming a paraxial limit; the human eye has a diameter of 4mm - 7mm and the ray can only make small angles with it. So, the Snell’s law equation can be written as
follows:
Here, we see the real depth and apparent depth formula in terms of refractive index. Now, in the next section, we will be discussing other phenomena occurring due to apparent depth.
Shift in Position and Lateral Magnification
The shift in position of the image concerning the object can be easily calculated. It is just the difference between the real depth minus the apparent depth.
For derivation of lateral magnification:
Consider an object of height ‘t’ placed in the glass beaker as shown in Image 2.
Distance of the image of N from interface is
Distance of the image of N from interface is
Then height of image observed will be
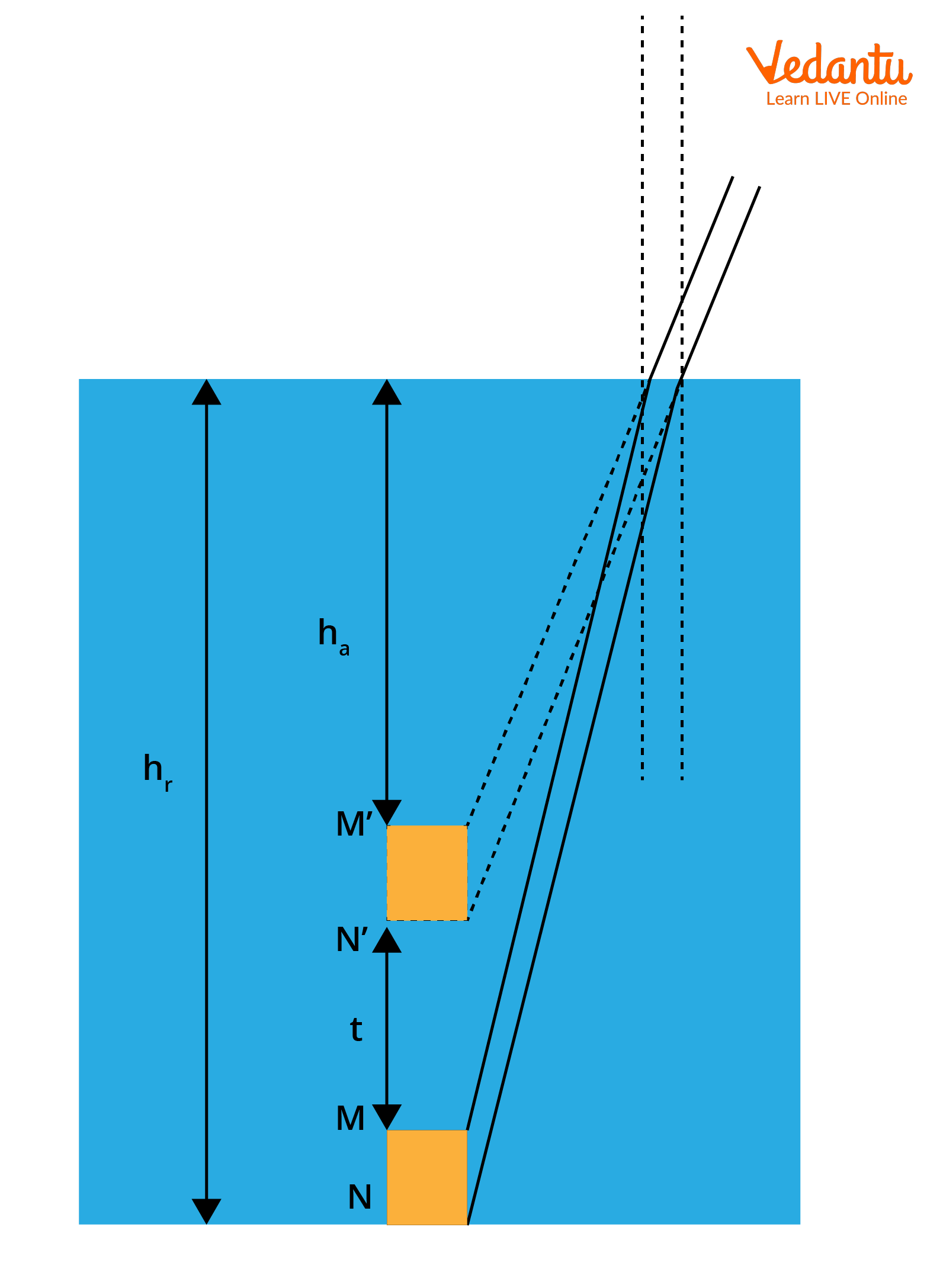
Difference Between Real Depth and Apparent Depth
Real Depth and Apparent Depth Numericals
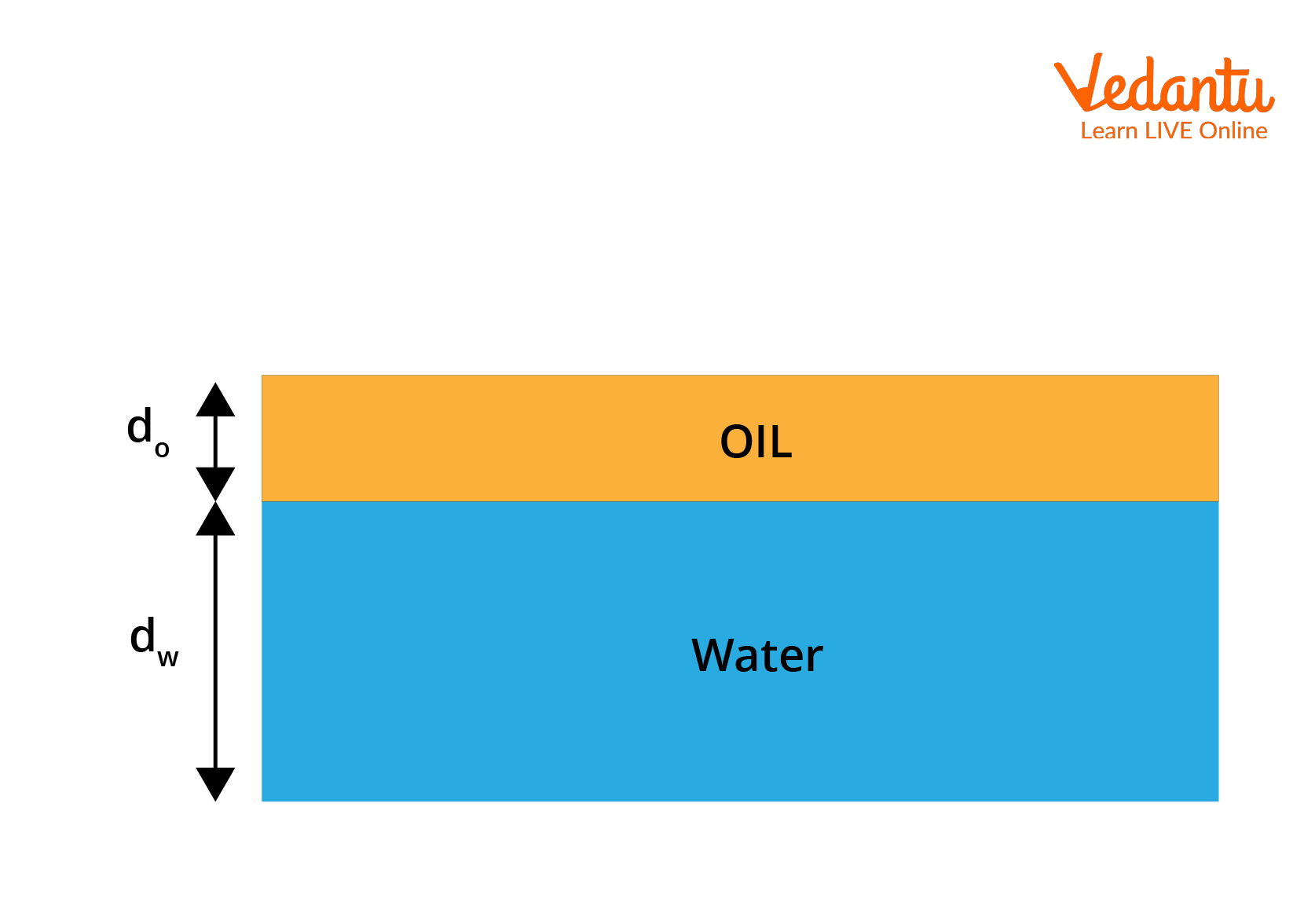
Example 1: A vessel contains water upto the height of 30cm and above it, and oil up to another 20cm of height. The refractive indices of water and oil are 1.33 and 1.30, respectively. Find the apparent depth of the vessel.
Solution:
We know the formula relating real depth, apparent depth and refractive index.
So apparent depth for water and oil is given by:
Total apparent depth is given by:
dw+d0=48.87
dw is the real depth in water.
dw’ is the apparent depth in water.
do is the real depth in oil.
do’ is the apparent depth in oil.
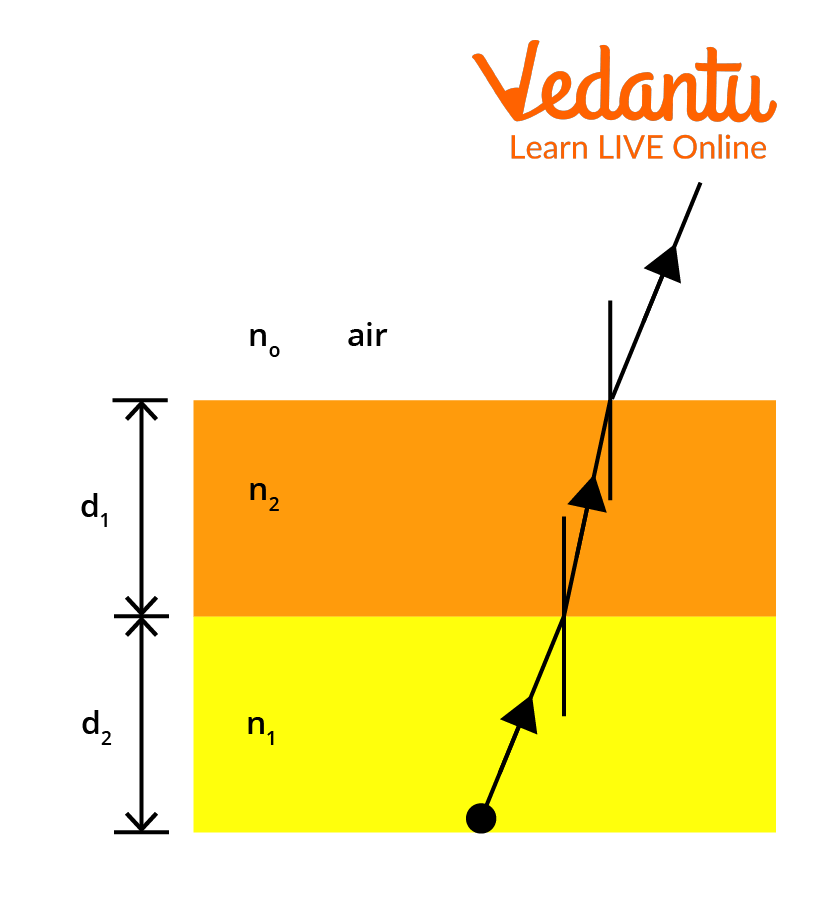
Example 2: A bucket of total height 50 cm is half filled with a liquid of refractive index 1.5 and half with another liquid of refractive index 2. Find the apparent depth of the bucket for an observer directly above the bucket.
Solution:
Since there are two different media, the apparent depths due to both media have to be added. d1 is the depth of the liquid of refractive index 1.5 and d2 is the depth of liquid with refractive index 2. Since the liquids fill half of the bucket, both d1 and d2 will be 25cm. Now the apparent depth due to both media will be given as
So, the apparent depth is 29.16cm.
Conclusion
Apparent depth can be said to be a lateral shift in the image of the object due to refraction. As discussed in the article, apparent and real depth are related through a refractive index of the medium, where the object is present or what the observer is viewing. The propagation of light from different media leads to refraction and when an observer views the object, they observe a shift in the object from its original position. This phenomena can be seen in nature during sunrise and sunset. Even when the sun has not crossed the horizon, we can see the sun due to the effect of refraction. This can be described as an apparent shift in the position of its image, although the object is not there at position.
JEE Main 2025 Subject-wise Important Chapters
The JEE Main 2025 subject-wise important chapters provide a focused strategy for Chemistry, Physics, and Maths. These chapters help students prioritise their preparation, ensuring they cover high-weightage topics for better performance in the exam.
Important Links for JEE Main and Advanced 2025
Access a curated collection of study materials and tips to excel in JEE Main and Advanced 2025, helping you prepare effectively for these prestigious engineering entrance exams.






FAQs on Real Depth and Apparent Depth for JEE Main Physics 2025
1. What are the factors on which the apparent depth of the object depends?
The apparent depth of the object only depends upon the refractive index of the medium. The refractive index regulates the amount of deviation of light rays coming from the object to the observer’s eye. The refractive index is determined as the ratio of the sine angle of incidence to the sine angle of refraction. The refraction light follows Snell’s law. The refraction of light also matters on behalf of the density of the mediums. The only factor which affects the apparent depth of the object is the refractive index.
2. Why is it important to calculate the critical angle?
In optics, the critical angle is the angle of incidence beyond which the total internal reflection of the light will happen. When the angle of incidence is more than the critical angle, then no refraction will take place. In that case, if a light ray strikes the interface of two different mediums, the incident ray will automatically be reflected into the same medium. It is found to be one of the largest incidence angles for which the reflection of light takes place in the same medium.
3. What is the weightage of this topic in the JEE exam?
The weightage of the topic real and apparent depth is 3%, while the weightage of optics is 6%. This concept is introduced in the section on ray optics which was found as the most important topic for the JEE advanced examination. Nearly 5-6 questions can be expected in the given competitive examination of JEE. It is necessary to practice many questions in preparation for the JEE examination. In this chapter, it is required to keep a check on every topic for better performance in this section.
4. Is real depth always greater than apparent depth? How does the concept of apparent depth help swimmers?
No, real depth is not always greater than apparent depth. In the case of an observer observing the object from a medium whose refractive index is less than the medium in which the object is there, real depth is greater than apparent depth. However, when the observer is viewing from a medium of higher refractive index than the medium in which the object is present, apparent depth is greater than real depth.
Due to the apparent depth, the floor of the swimming pool seems to be less deep than it is. Due to this an inexperienced swimmer may drown. However, prior knowledge of it helps in preventing such accidents.
5. If the viewer shifts from a normal view then will it affect the correct calculation of apparent depth? What are the factors affecting the apparent depth?
Yes, it does affect the calculation if the observer shifts his/her viewing from normal to a more inclined angle. This is due to the approximations we consider via paraxial limits when deriving the relation between apparent depth, real depth and refractive index. The limit does not allow inclination greater than four degrees. The factors that affect the apparent depth are the refractive index of the medium and the depth up to which the liquid is present.
6. What is the formula for calculating real depth and apparent depth?
The formula for calculating the apparent depth and real depth is:
Where:
Real Depth is the actual depth of the object in the medium.
Apparent Depth is the perceived depth due to the refraction of light.
Refractive Index is the ratio of the speed of light in a vacuum to the speed of light in the medium.
This formula helps in calculating how much shallower an object appears due to the light's refraction at the interface between two media.
7. How do you derive the Real Depth And Apparent Depth Formula Refractive Index?
The derivation of real depth and apparent depth uses Snell’s law, which relates the angle of incidence and the angle of refraction at the interface between the two media. By applying Snell’s law and considering the geometry of the situation, the apparent depth can be derived as:
The refractive index determines how much the light rays bend as they pass through the different mediums, leading to the perceived shallowness of objects.
8. What is the difference between real depth and apparent depth?
Real depth refers to the actual depth of an object in a medium, while apparent depth is how deep the object seems to be when viewed from above the surface, due to the bending of light (refraction). The apparent depth is always less than the real depth when viewed from a denser medium like water to air, due to the light bending away from the normal.
9. How does the refractive index affect the apparent depth?
The refractive index of the medium plays a crucial role in determining the apparent depth. A higher refractive index causes light to bend less, making the object appear closer to the surface, thus decreasing the apparent depth. Conversely, a lower refractive index causes greater bending of light, making the object appear deeper than it is.
10. Can the real depth and apparent depth be equal?
Yes, real depth and apparent depth can be equal when the refractive index of the medium is 1 (such as in a vacuum or when light is passing through the same medium). In this case, there is no refraction, and the object appears at its actual depth.
11. How does the diagram of real and apparent depth help in understanding the concept?
A diagram of real depth and apparent depth helps visually represent the change in the perceived depth due to refraction. The diagram typically shows an object submerged in a medium, with the real depth being the actual distance from the object to the surface and the apparent depth being the perceived distance, which is shallower due to the bending of light rays as they move from the denser medium (like water) to air.
12. How does the refractive index of different mediums affect the apparent depth?
The refractive index of a medium affects how light bends when passing through the medium. A higher refractive index makes the light bend less, causing the object to appear closer to the surface (less depth), while a lower refractive index makes the light bend more, increasing the apparent depth. For example, an object in water will appear shallower when viewed from the air due to the refractive index of water being greater than air.
13. How do different angles of observation affect the apparent depth?
The angle of observation affects the apparent depth because the light rays bend differently depending on the angle at which they are observed. If the observer is looking straight down (normal view), the apparent depth is calculated using the refractive index. However, when viewed at an angle, the apparent depth can seem even shallower due to the change in the angle of refraction, following the principles of Snell’s law.
14. How do the real depth and apparent depth concepts apply in daily life?
In daily life, the concept of real depth and apparent depth is observed when looking into pools, lakes, or any body of water. For example, when you look at a swimming pool, the floor appears much closer than it is because of the refraction of light as it moves from water (denser medium) to air (less dense medium).
















