Concise Mathematics Class 10 ICSE Solutions for Chapter 18 - Tangents and Intersecting Chords

Very Short A Type

1. The radius of a circle is 8cm. Calculate the length of a tangent drawn to this circle from a point at a distance of 10cm from its centre.

Ans: 

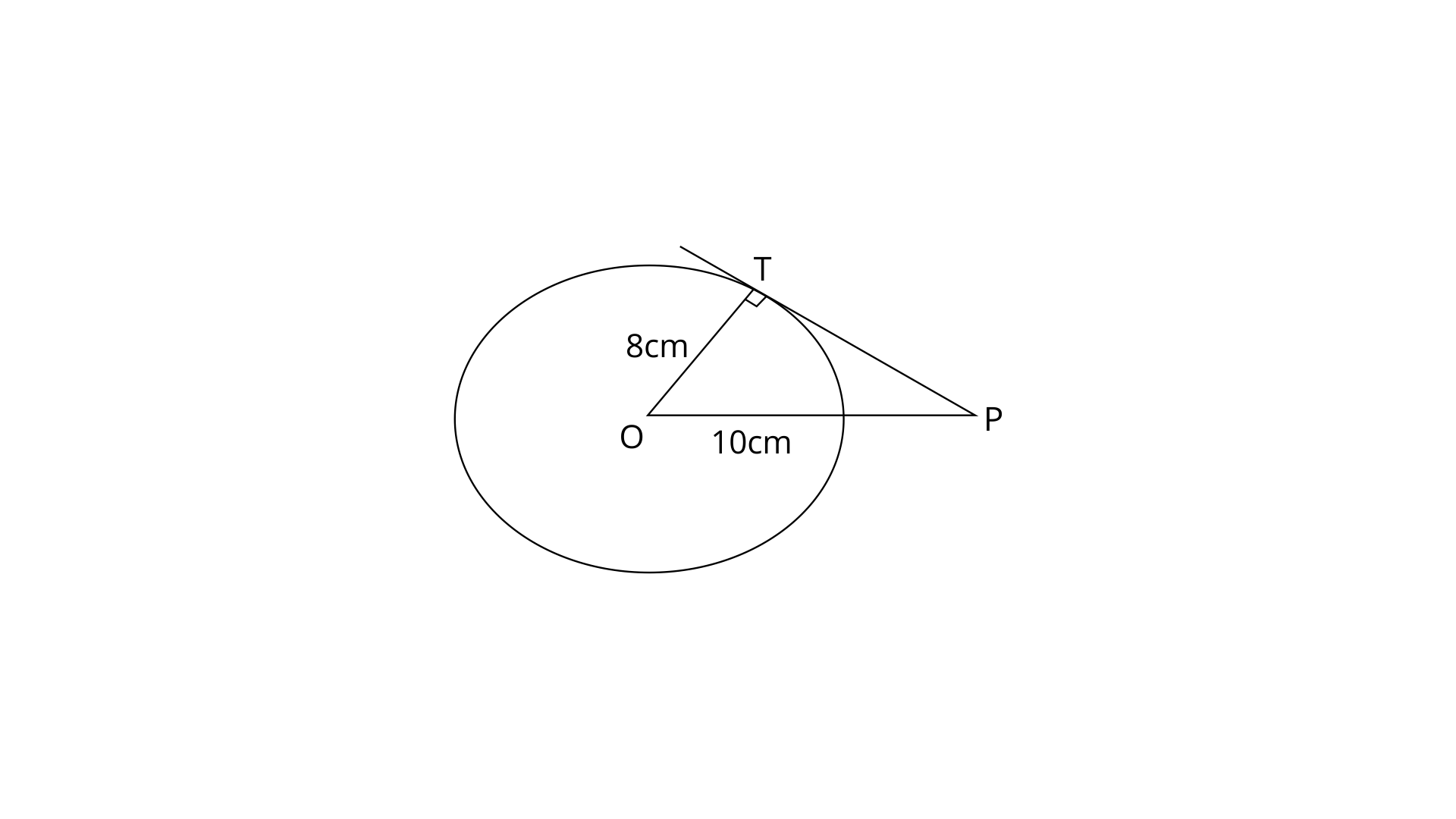
Given, Radius OT=8cm

OP=10cm

Radius is perpendicular to the tangent on the circle. Therefore $\Delta OTP$ is a right angle triangle.

Now by using the pythagoras theorem in $\Delta OTP$

$O{{P}^{2}}=O{{T}^{2}}+T{{P}^{2}}$

$\Rightarrow T{{P}^{2}}={{\left( 10 \right)}^{2}}-{{\left( 8 \right)}^{2}}=100-64=36$

$\Rightarrow TP=\sqrt{36}=6$

Thus the length of the tangent is 6cm.

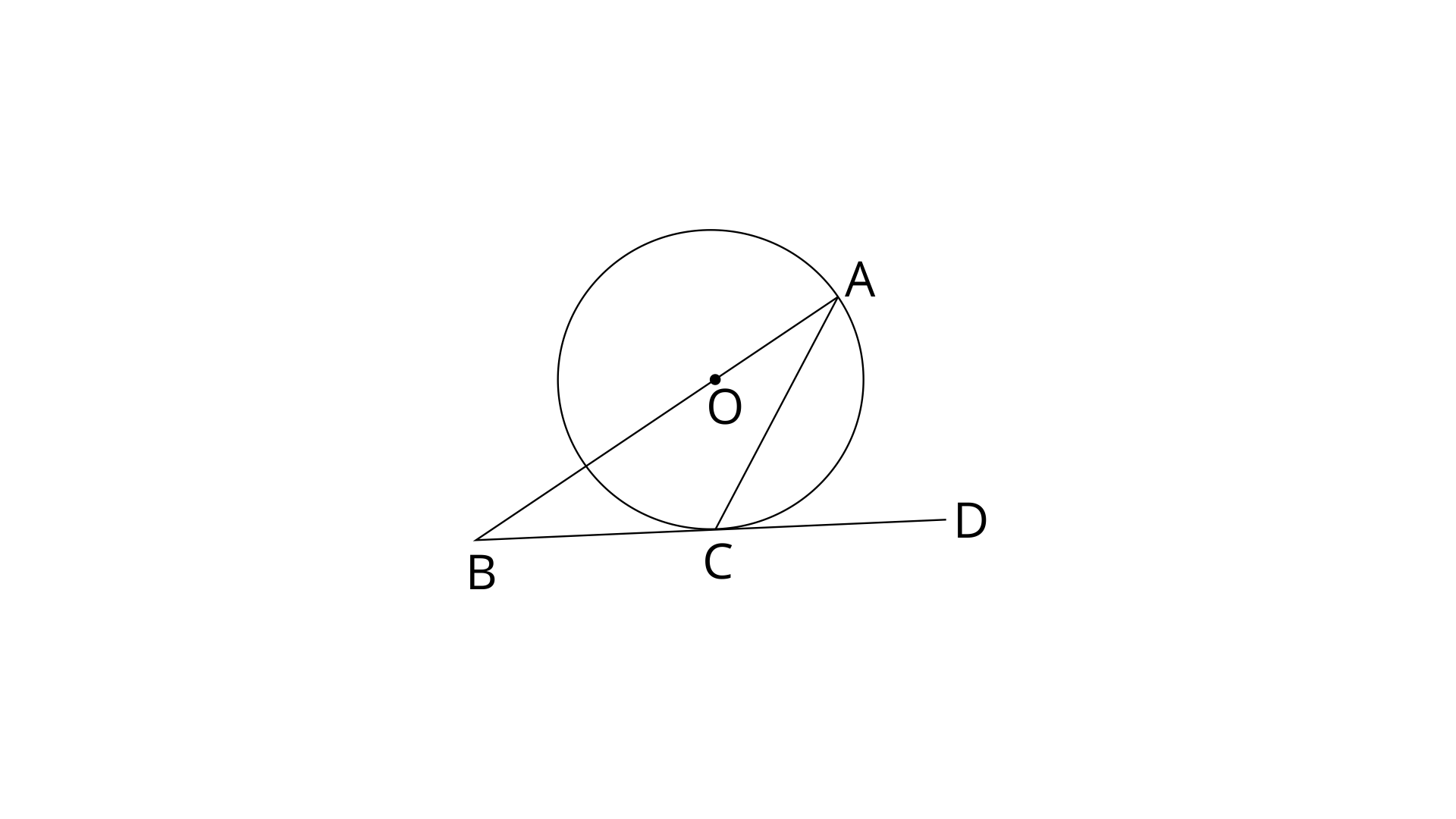
2. In the given figure, O is the centre of the circle and AB is a tangent at B. If AB=15cm and AC=7.5cm, calculate the radius of the circle.

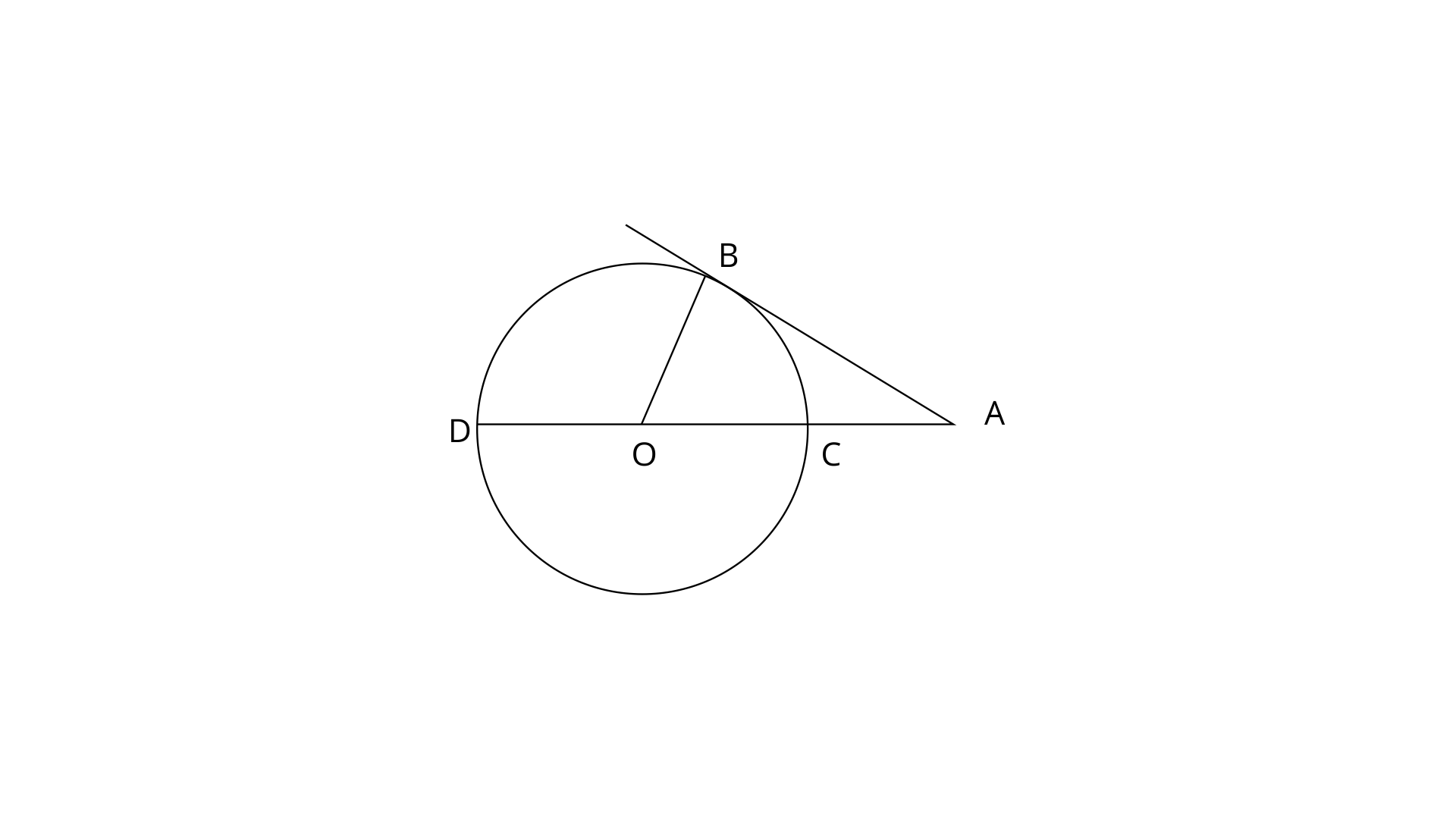
Ans: 

Given, AB=15cm, AC=7.5cm

Let the radius of the circle is r.

OB=OC=r

$OA=OC+AC$

$\Rightarrow OA=r+7.5$

Radius is perpendicular to the tangent, therefore $\Delta OBA$is a right angle triangle. 

Now by using pythagoras theorem in $\Delta OBA$

$O{{A}^{2}}=O{{B}^{2}}+A{{B}^{2}}$

$\Rightarrow {{\left( r+7.5 \right)}^{2}}={{r}^{2}}+{{15}^{2}}$

$\Rightarrow {{r}^{2}}+15r+56.25={{r}^{2}}+225$

$\Rightarrow 15r=225-56.25$

$\Rightarrow 15r=168.75$

$\Rightarrow r=11.25$

Therefore the radius of circle is $11.25$cm.

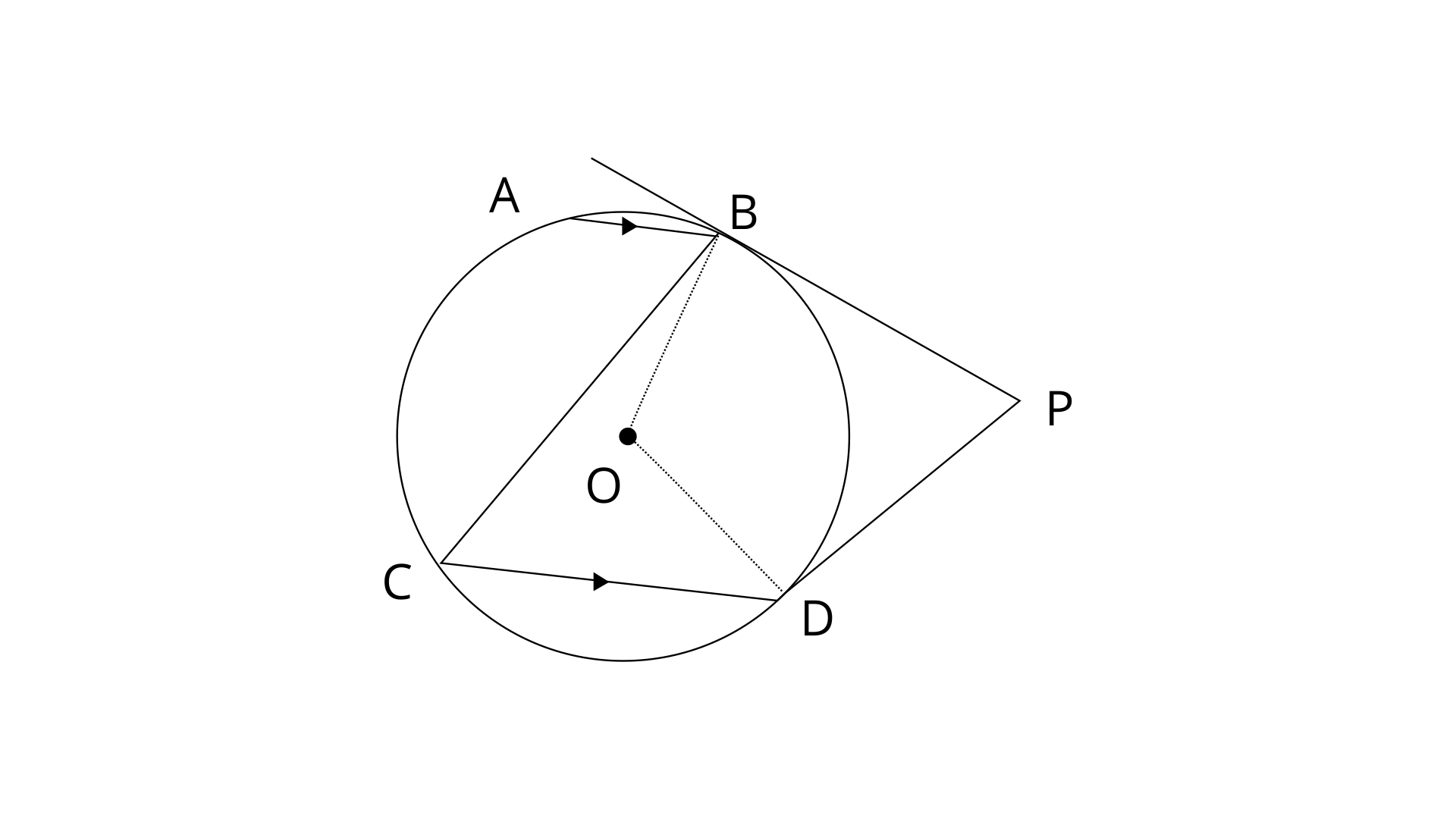
3. Two circles touch each other externally at point P. Q is a point on the common tangent through P. Prove that the tangents QA and QB are equal.

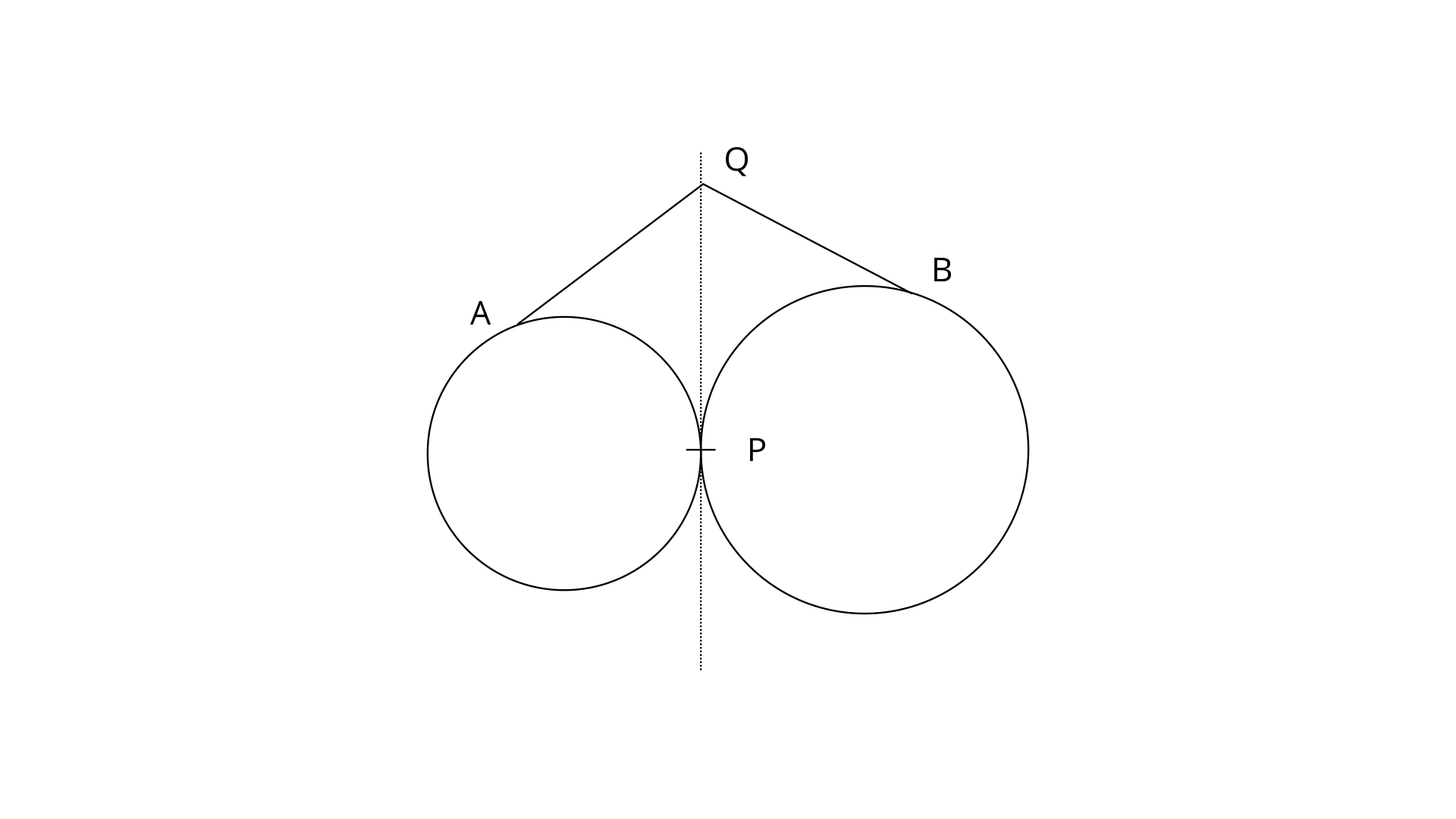
Ans: Tangents to  circle from an exterior point are equal. 

So, AQ=PQ------(1)

Again, BQ=PQ-----------(2)

From eq.(1) and (2)

AQ=BQ. (Hence proved)

4. Two circles touch each other internally. Show that the tangents drawn to the two circles from any point on the common tangent, are equal in length.

Ans: 

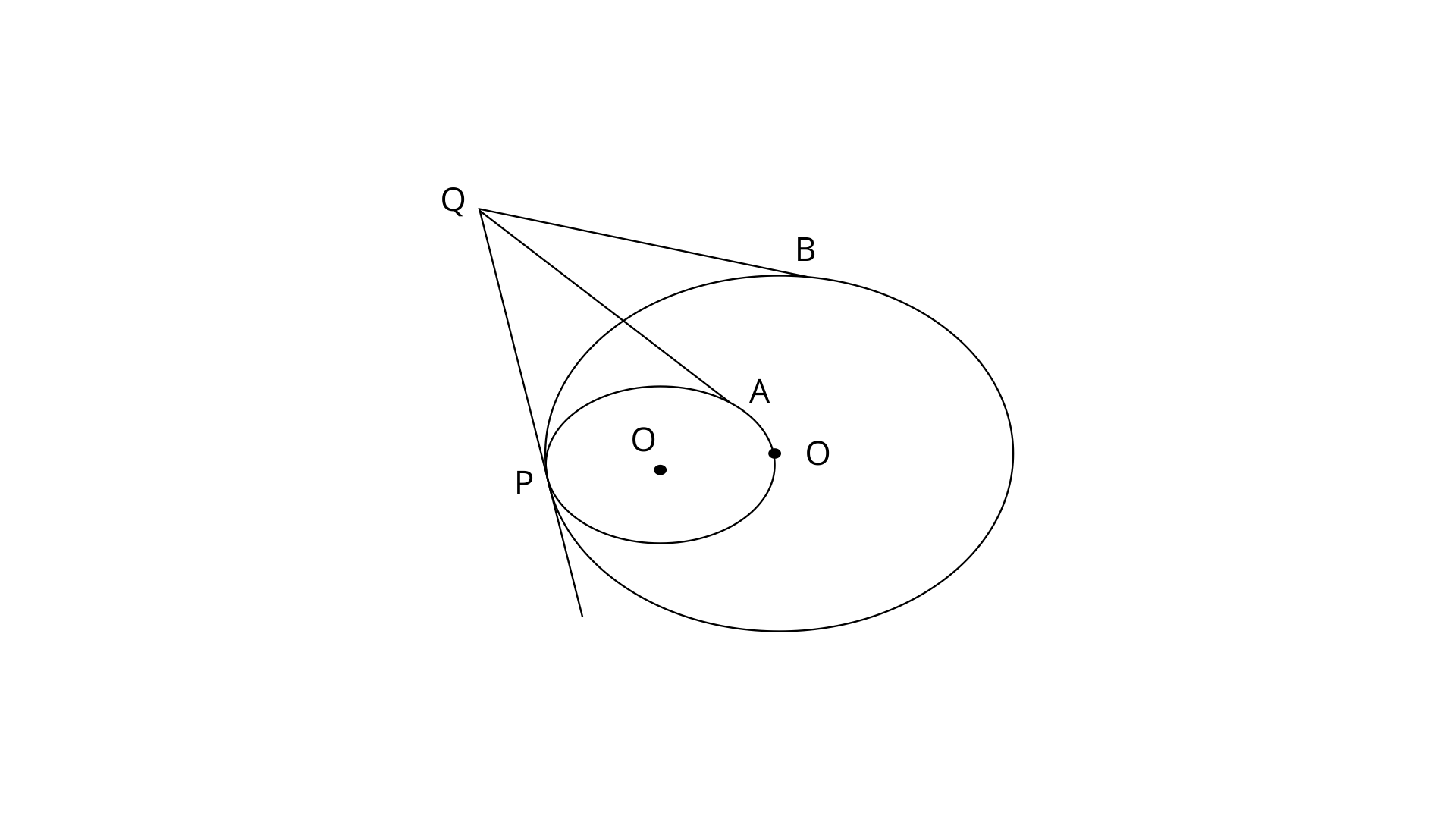
Tangents to a circle from an exterior point are equal.

Here PQ and QA are two tangents to the smaller circle from point exterior point Q.

$\Rightarrow PQ=QA$------(1)

Now, PQ and QB are two tangents to the large circle from point Q.

$\Rightarrow PQ=QB$--------(2)

From eq. (1) and (2)

$QA=QB$

Hence proved.

5. Two circles of radii 5cm and 3cm are concentric. Calculate the length of a chord of the outer circle which touches the inner.

Ans:

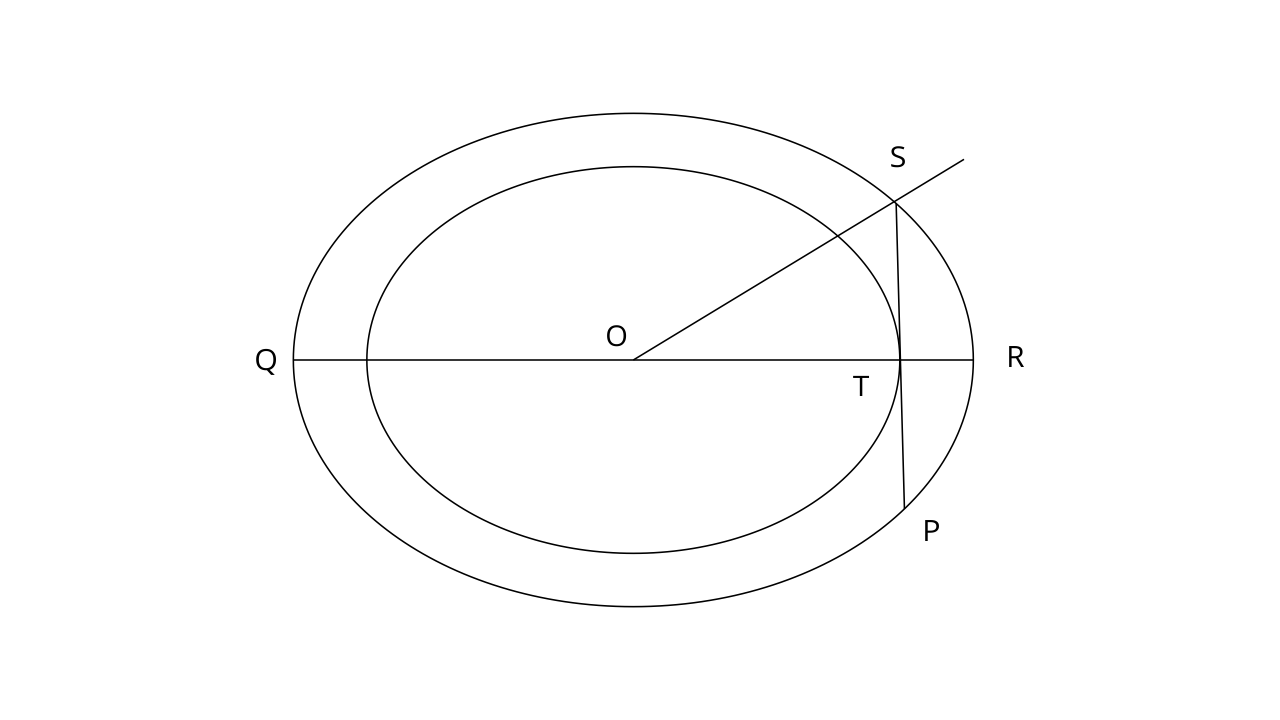
The radii of the concentric circles are 5cm and 3cm.

Sinc, SP is the chord of the larger circle and tangent to the smaller circle as well. OT is the radius of the smaller circle and we know that radius is perpendicular to the tangent. Therefore, OST is a right angle triangle. 

By using pythagoras theorem in $\Delta OST$

$O{{S}^{2}}=O{{T}^{2}}+S{{T}^{2}}$

$\Rightarrow S{{T}^{2}}=O{{S}^{2}}-O{{T}^{2}}$

$\Rightarrow S{{T}^{2}}={{\left( 5 \right)}^{2}}-{{\left( 3 \right)}^{2}}=25-9=16$

$\Rightarrow ST=4cm$

OT is perpendicular to the chord SP, Hence SP bisects the SP.

Therefore, $SP=2\times SP=2\times 4=8$ 

Thus the length of the chord SP is 8cm.

6. Three circles touch each other externally. A triangle is formed when the centres of these circles are joined together. Find the radii of the circles, if the sides of the triangle formed are 6cm, 8cm and 9cm.

Ans: 

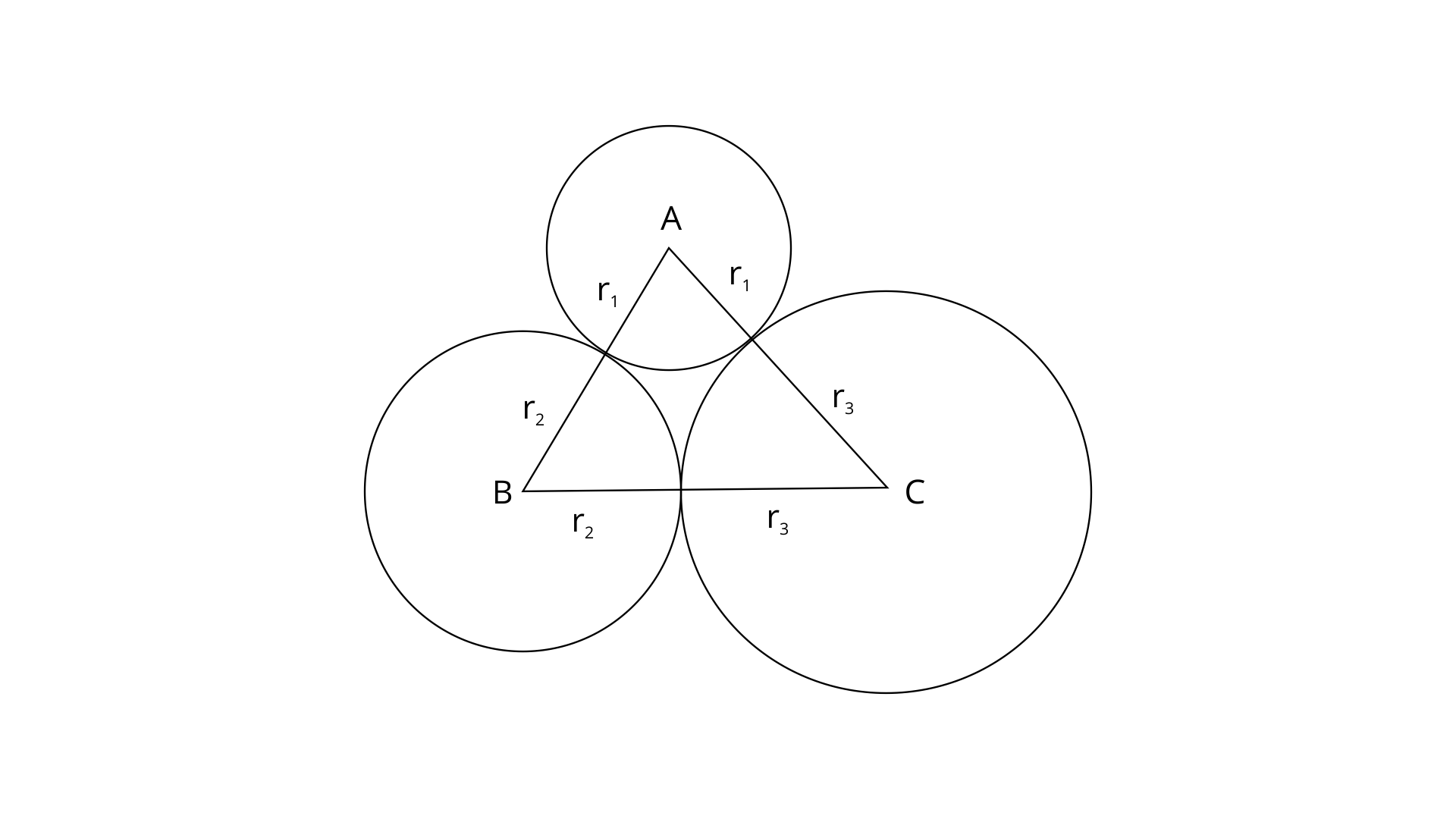
Let the radii of the circles are ${{r}_{1}},~{{r}_{2}},~{{r}_{3}}$.

Given, The sides of the triangle are 6cm, 8cm, 9cm.

From the diagram, 

${{r}_{1}}+{{r}_{2}}=6cm$--------(1)

${{r}_{2}}+{{r}_{3}}=9cm$--------(2)

${{r}_{1}}+{{r}_{3}}=8cm$--------(3)

Adding eq.(1), (2) and (3)

${{r}_{1}}+{{r}_{2}}+{{r}_{2}}+{{r}_{3}}+{{r}_{1}}+{{r}_{3}}=8+9+6$

$\Rightarrow 2\left( {{r}_{1}}+{{r}_{2}}+{{r}_{3}} \right)=8+9+6$

$\Rightarrow \left( {{r}_{1}}+{{r}_{2}}+{{r}_{3}} \right)=11.5$

$\Rightarrow {{r}_{1}}=11.5-9=2.5$

Substituting  ${{r}_{1}}=2.5$in equation (1).

${{r}_{2}}=6-2.5=3.5$

Now, substituting ${{r}_{2}}=3.5$in equation (2).

${{r}_{2}}+{{r}_{3}}=9$

$\Rightarrow {{r}_{3}}=9-3.5=5.5$

Therefore, the radii of circles are 2.5cm, 3.5cm, 5.5cm.

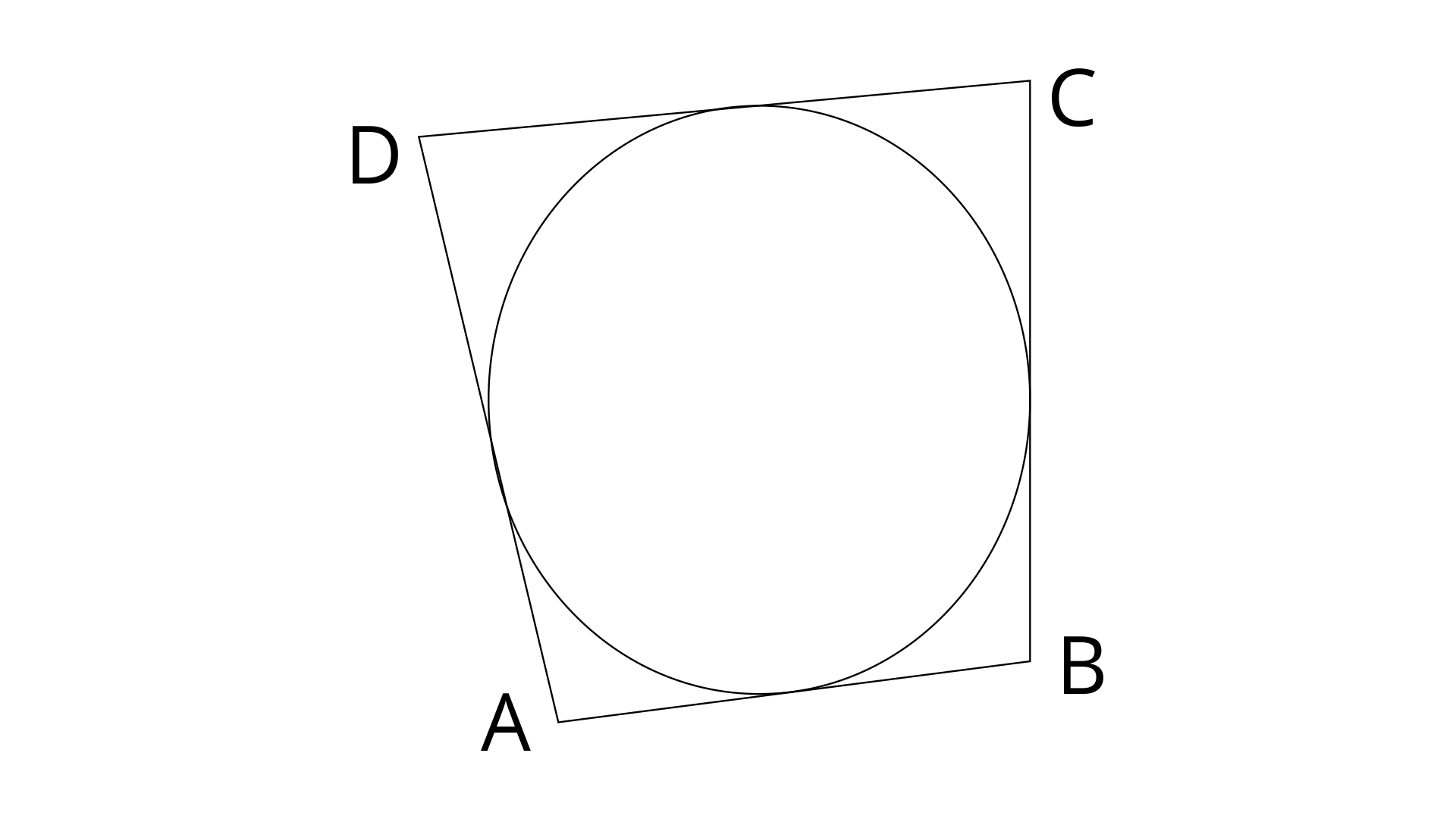
7. If the sides of a quadrilateral ABCD touch a circle, prove that:

AB+CD=BC+AD.

Ans:

Let the points P,Q,R and S are the points on sides AB, BC, CD and AD respectively.

Tangents to the circle from an exterior angle are equal.

Since AP and AS are the tangents to the circle from external point A.

AP=AS-----------(1)

Similarly, 

BP=BQ-----------(2)

CR=CQ----------(3)

DR=DS-----------(4)

Add equation (1),(2),(3) and (4)

$\left( AP+PB \right)+\left( CR+DR \right)=\left( AS+DS \right)+\left( BQ+CQ \right)$

$\Rightarrow AB+CD=BC+AD$ 

[since, AP+BP=AB, CR+DR=CD and AS+DS=AD, BQ+QC=BC]

Hence proved.

8. If the sides of a parallelogram touch a circle (refer figure of Q.7), prove that the parallelogram is a rhombus.

Ans: 

Let P, Q, R and S are the points on the sides AB, BC, CD and AD respectively of the quadrilateral ABCD.

We know that the tangents to a circle from an external point are always equal.

Tangents from point A to the circle are AP and AS.

So, AP=AS -------------(1)

Similarly, BP=BQ ----------(2)

CR=CQ -----------(3)

DR=DS -----------(4)

On adding eq. (1),(2),(3) and (4)

AP+BP+CR+DR=AS+BQ+CQ+DS

(AP+BP)+(CR+DR)=(AS+DS)+(BQ+CQ)

[Since, (AP+BP)=AB, (CR+DR)=CD and (AS+DS)=AD, BQ+CQ=BC]

$\Rightarrow AB+CD=AD+BC$

Since ABCD is a parallelogram. Therefore AD=BC and AB=CD

Now, $\Rightarrow AB+AB=BC+BC$

$\Rightarrow AB=BC$

Therefore, $AB=BC=CD=DA$

Thus the parallelogram ABCD is a rhombus.

9. From the given figure, prove that:

AP+BQ+CR=BP+CQ+AR. Also, Show that:

AP+BQ+CR=$\frac{1}{2}\times $Perimeter of $\Delta ABC$.

Ans: Tangents drawn to a circle from an exterior point are always equal.

AP and AR are drawn to the circle from exterior point A. 

Hence, AP=AR -----------(1)

Similarly, BQ=BP --------(2)

And, CR=CQ -------------(3) 

On adding eq. (1), (2) and (3)

$AP+BQ+CR=AR+BP+CQ~$

On adding AP+BQ+CR both sides

$AP+BQ+CR+AP+BQ+CR=AR+BP+CQ+AP+BQ+CR~$

$\Rightarrow 2\left( AP+BQ+CR \right)=\left( AP+BP \right)+\left( AR+CR \right)+\left( BQ+CQ \right)$

$\Rightarrow 2\left( AP+BQ+CR \right)=AB+AC+BC$

$\Rightarrow AP+BQ+CR=\frac{1}{2}\times \left( AB+BC+AC \right)$ 

Perimeter of a triangle is the sum of all the sides.$\left( AB+BC+AC \right)$=Perimeter of the triangle.

Therefore, $AP+BQ+CR=\frac{1}{2}\times Perimeter~of~the~triangle~ABC.$

10. In the given figure of Q.9; if AB=AC then prove that: BQ=CQ.

Ans: 

Tangents to a circle from an exterior point are always equal.

Here, AP and AR the tangents to the circle from the point A.

Hence, AP=AR ---------(1)

Similarly, BP=BQ -----------(2) and CQ=CR ----------(3)

On adding eq.(1), (2) and (3)

$\left( AP+BP \right)+CQ=\left( AR+CR \right)+BQ$

$AB+CQ=AC+BQ$

Given, $AB=AC$

Therefore, $CQ=BQ$ (Hence Proved)

11. Radii of two circles are 6.3cm and 3.6cm. State the distance between their centres if :

(i). They touch each other externally.

(ii). They touch each other internally.

Ans: 

(i).

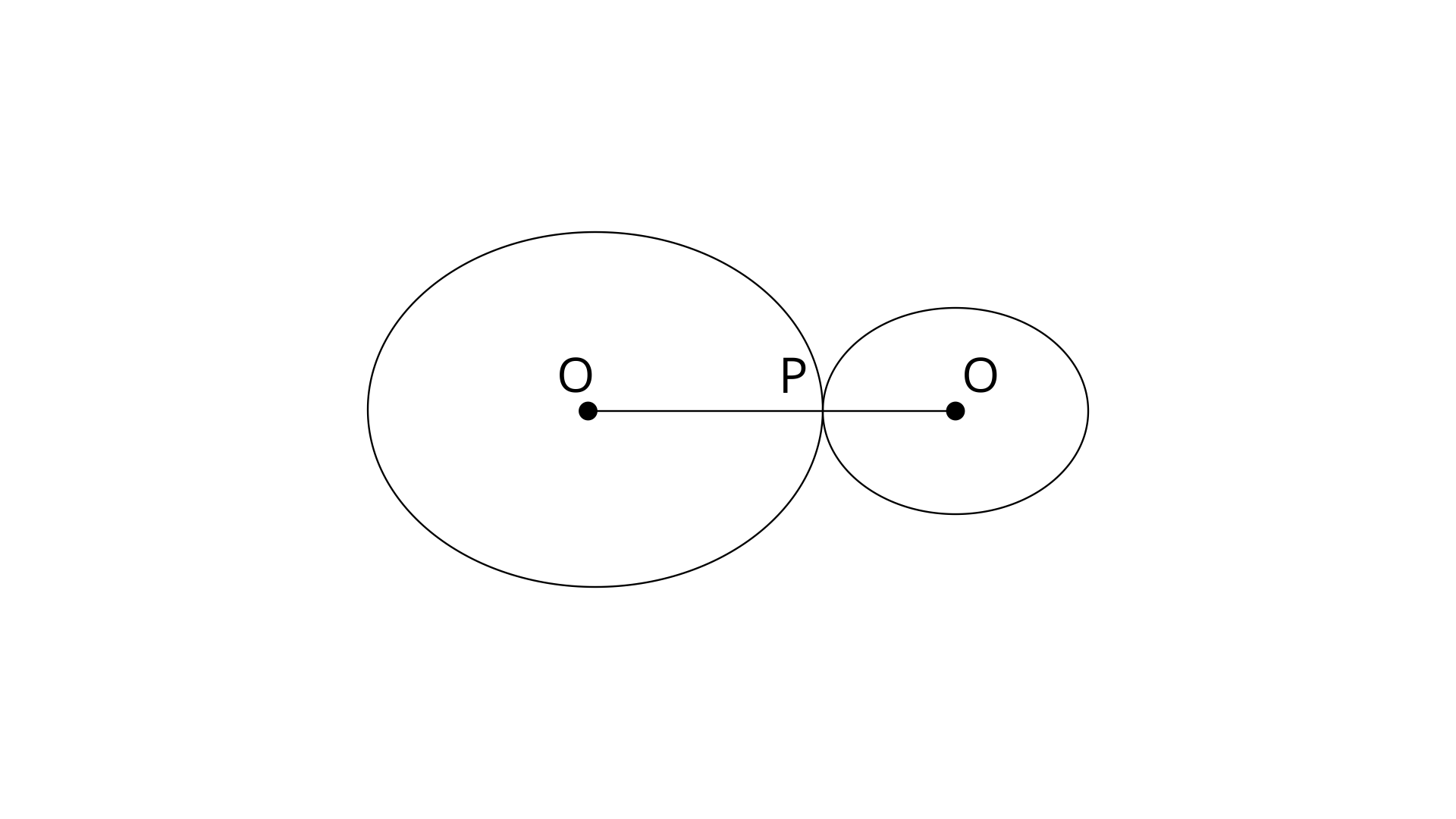
Let the centres of the circle be O,O’ and P is the touching points of the circles.

Radii of the circles are 6.3cm and 3.6cm.

Now the distance between centres is OO’=OP+O’P

                                                                  =6.3cm+3.6cm=9.9cm

(ii). 

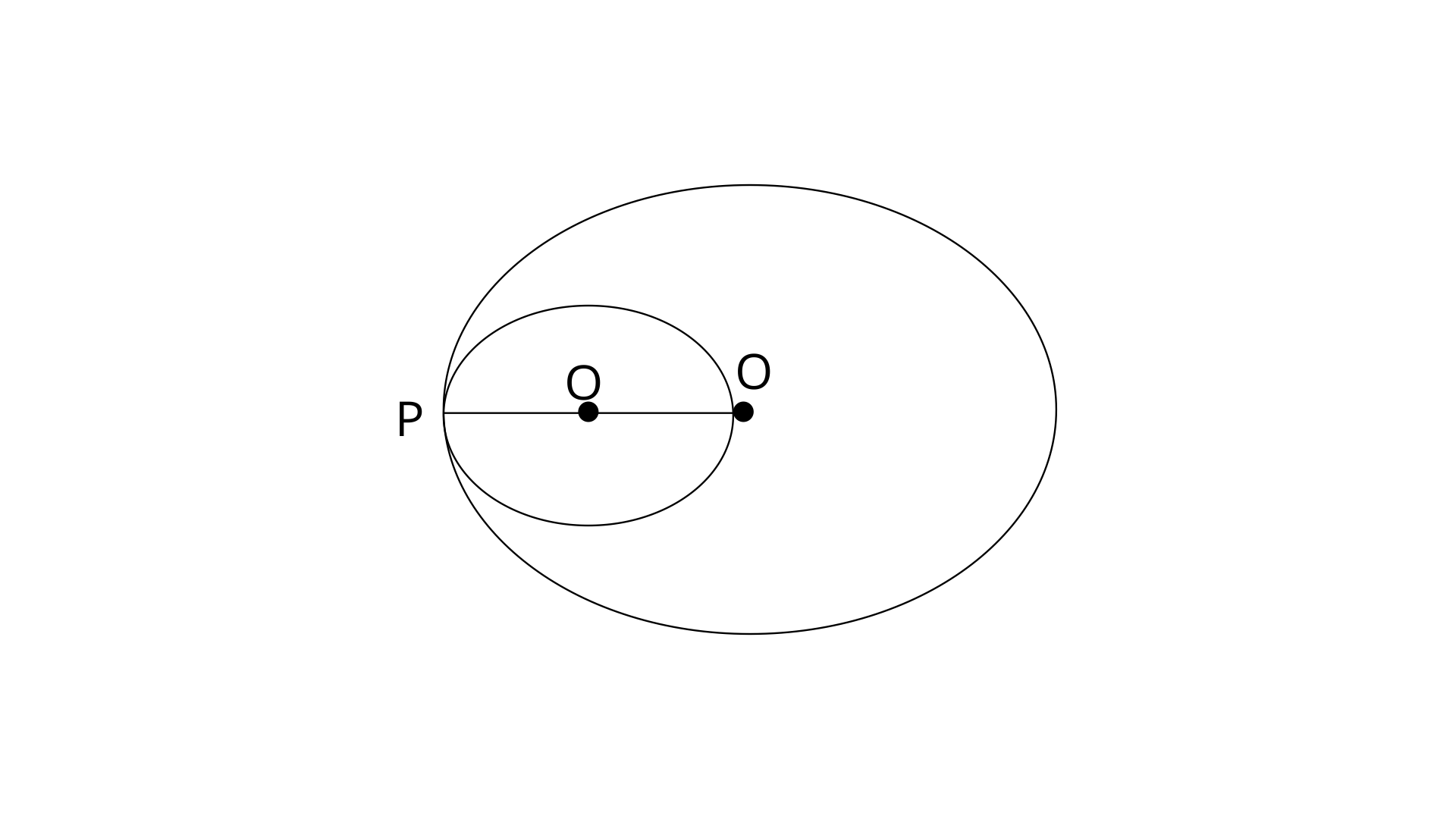
Radii of the circles are 6.3cm and 3.6cm. OP=6.3cm and O’P=3.6cm

Now the distance between centres is OO’=OP-O’P=6.3-3.6=2.7cm

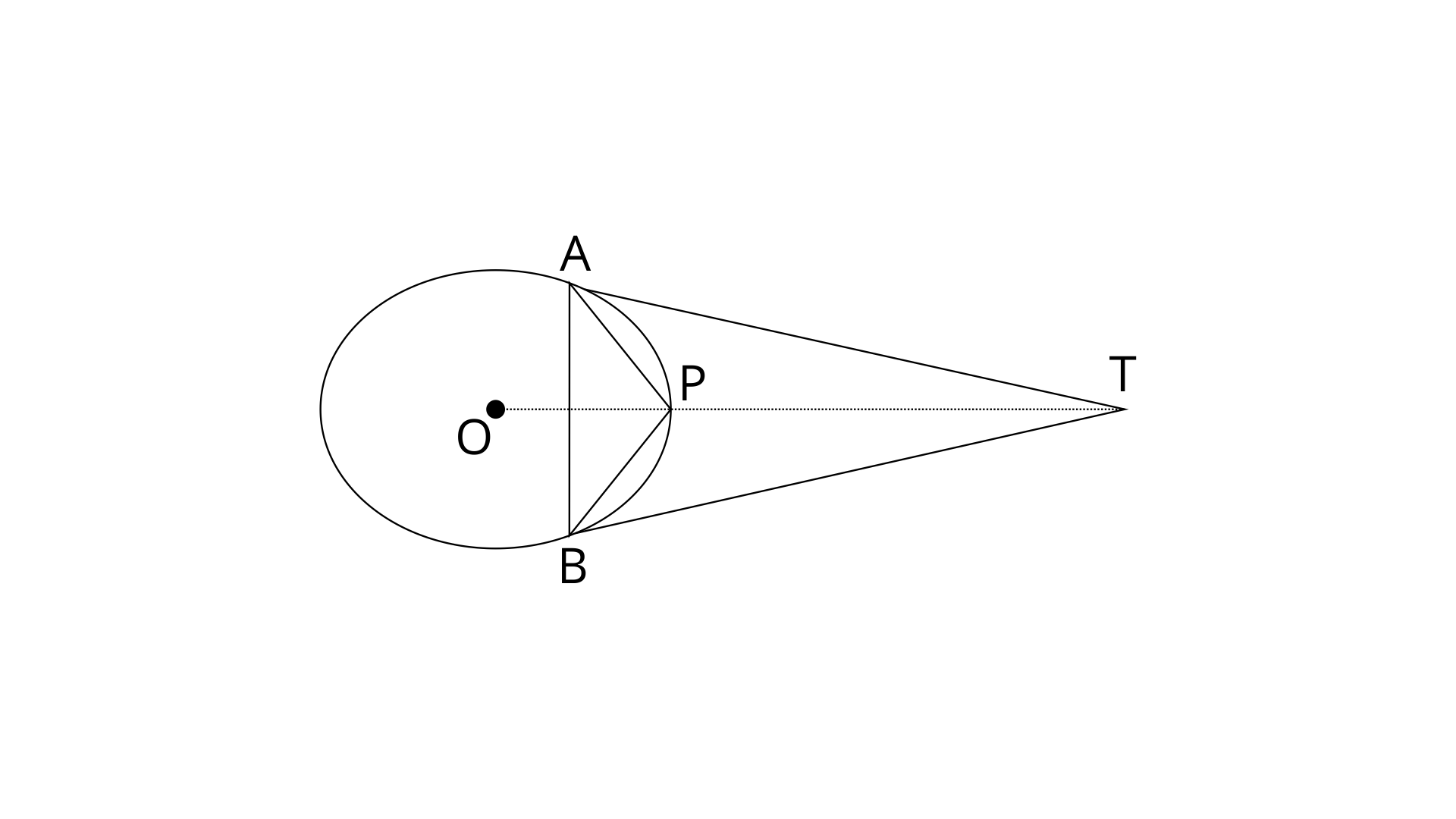
12. From a point P outside a circle, with centre O, tangents PA and PB are drawn. Prove that :

(i).$\angle AOP=\angle BOP$

Ans:

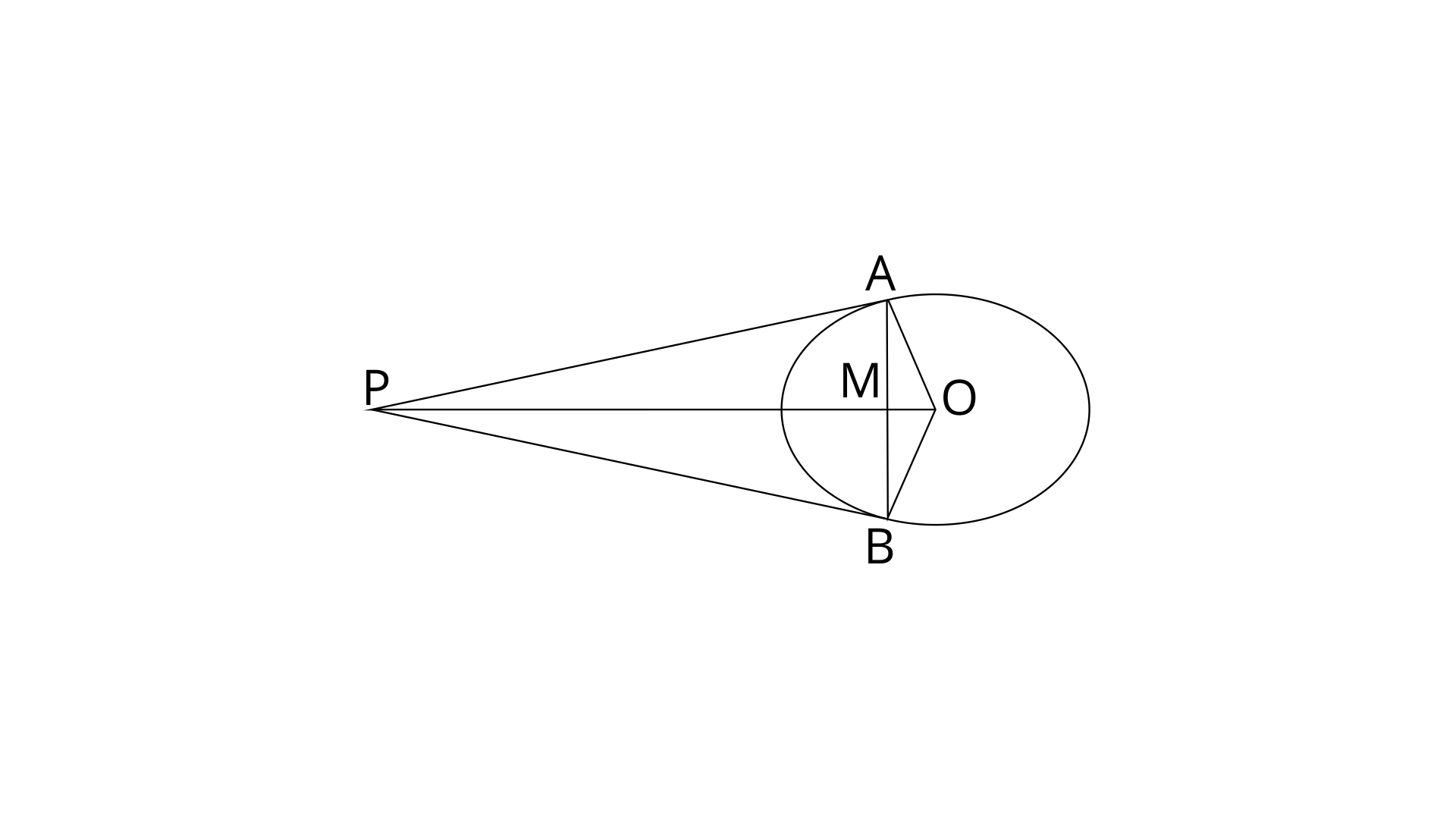
In $\Delta AOP~and~\Delta BOP~$

AP=BP (Tangents from an external points are equal)

OP=OP (common sides)

OA=OB (Radii of circle)

$\Delta AOP~\Delta BOP$ (By SSS congruence law)

Therefore, $\angle AOP=\angle BOP$

(ii). OP is the 丄 bisector of chord AB.

Ans: In $\Delta OAM~and~\Delta OBM$

OM=OM (common sides)

OA=OB (Radii of same circle)

$\angle AOM=\angle BOM$(Since $\angle AOP=\angle BOP$)

$\Delta OAM~\Delta OBM$(By SAS congruence law)

Therefore, AM=MB, $\angle OMA=\angle OMB$

Since, $\angle OMA+\angle OMB={{180}^{\circ }}$

$\Rightarrow 2\angle OMA={{180}^{\circ }}$

$\Rightarrow \angle OMA={{90}^{\circ }}$

Thus OM and OP are perpendicular bisectors of chord AB.

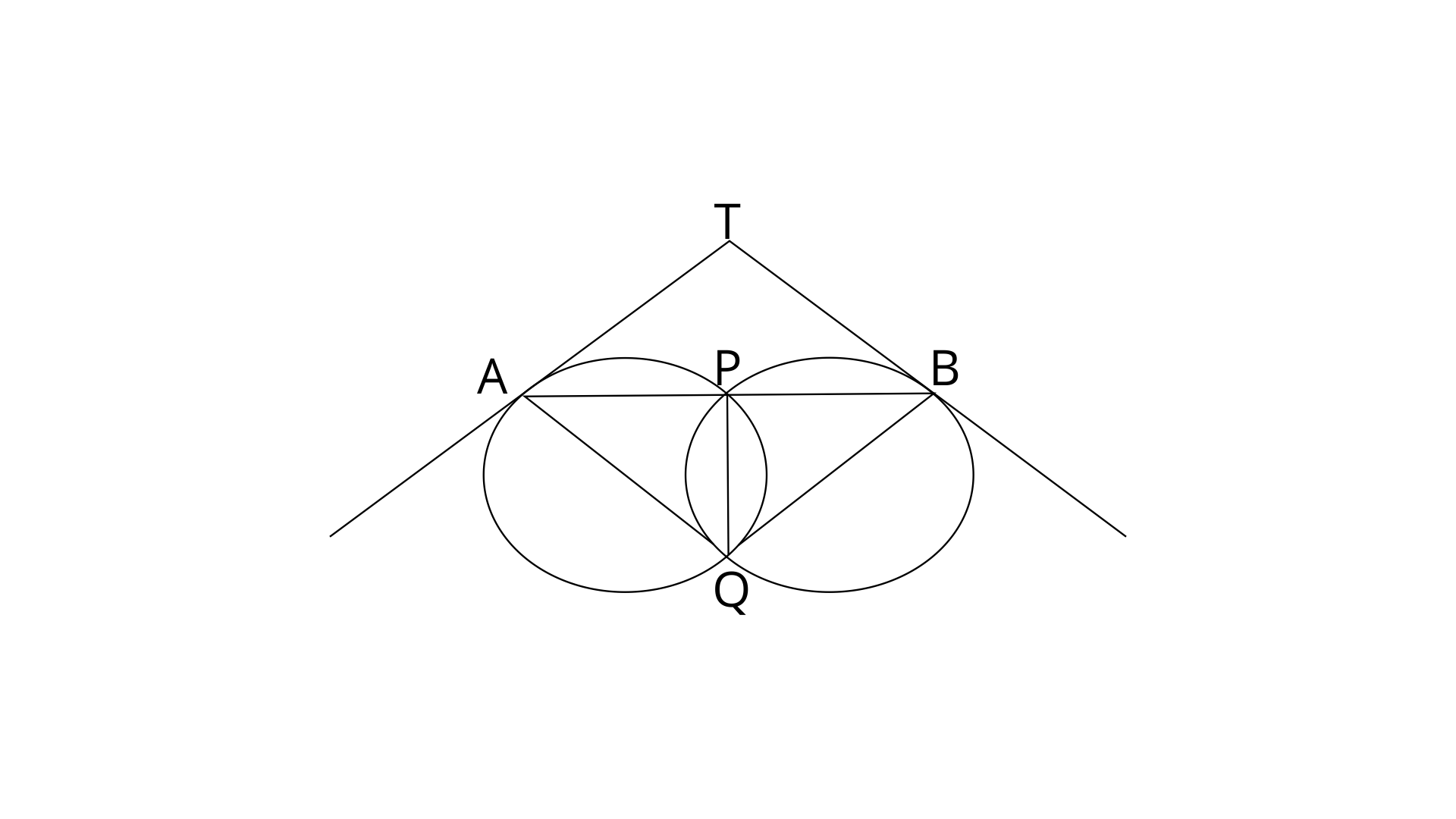
13. In the given figure, two circles touch each other externally at point P. AB is the direct common tangent of these circles. Prove that:

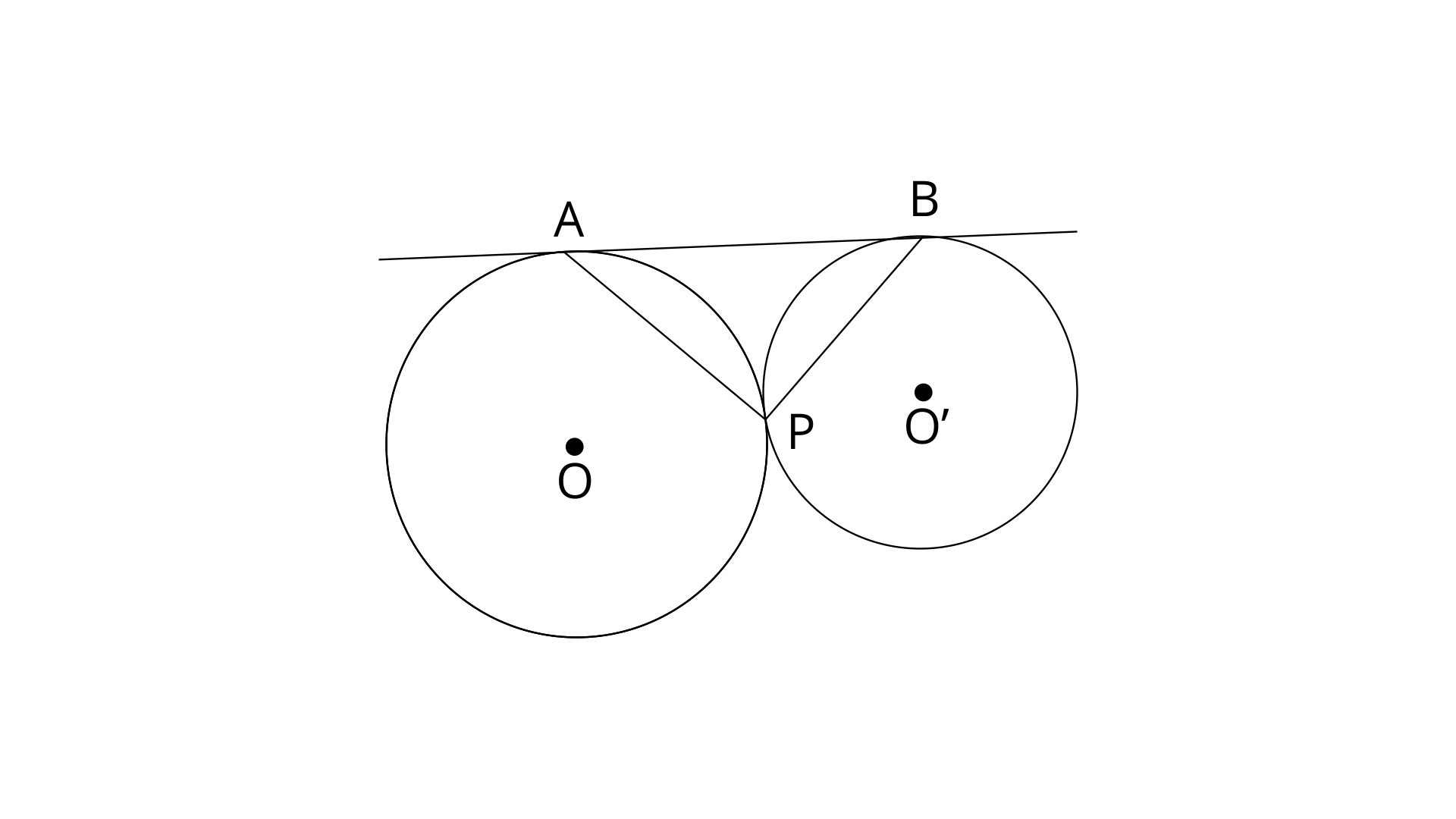
(i). Tangent at point P bisects AB, 

Ans:

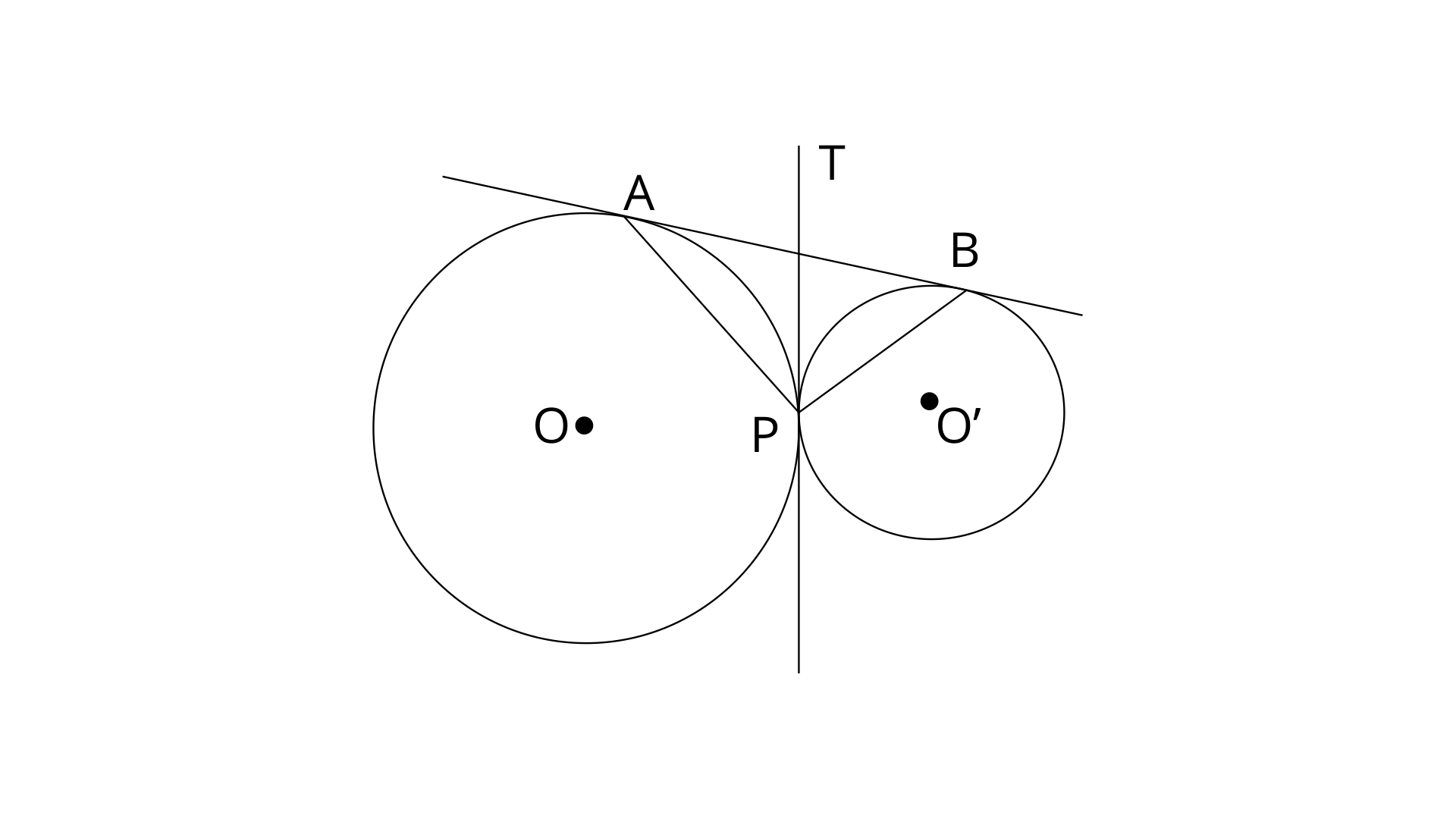
Let the common tangent touching both circles is TPT’

Tangents to a circle from an external point are equal. Here TP and TA are tangents to the circle from point T.

TA=TP ---------(1)

Similarly, AB=TB --------(2)

From eq.(1) and (2)

TA=TB=TP 

Therefore TPT’ is a bisector of AB.

(ii). Angle $\angle APB={{90}^{\circ }}.$

Ans: In $\Delta TAP$

Since, TA=TB 

$\Rightarrow \angle TAP=\angle TPA$-------(1)

Similarly, $\Delta TPB$

 TP=TB

$\Rightarrow \angle TBP=\angle TPB$ ----------(2)

On adding from eq. (1) and (2)

$\angle TAP+\angle TBP=\angle TPA+\angle TPB$

$\angle APB=\angle TAP+\angle TBP$  ------(3)

In $\Delta APB$ By using angle sum property,

$\angle APB+\angle TAP+\angle TBP={{180}^{\circ }}$

$\Rightarrow 2\angle APB={{180}^{\circ }}$

$\Rightarrow \angle APB={{90}^{\circ }}$

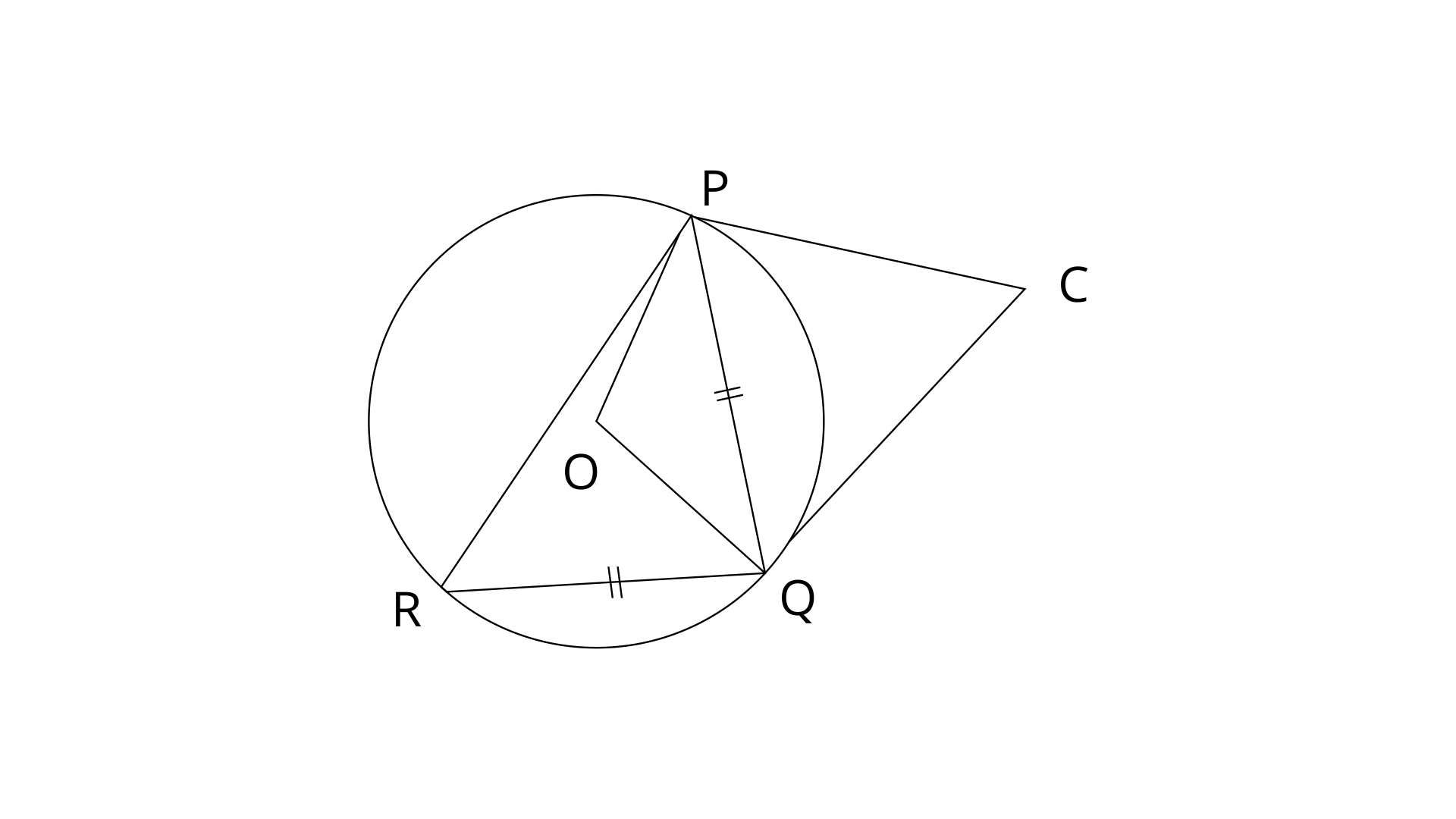
14. Tangents AP and AQ are drawn to a circle, with centre O, from an exterior point A. Prove that :

$\angle PAQ=2\angle OPQ$

Ans: 

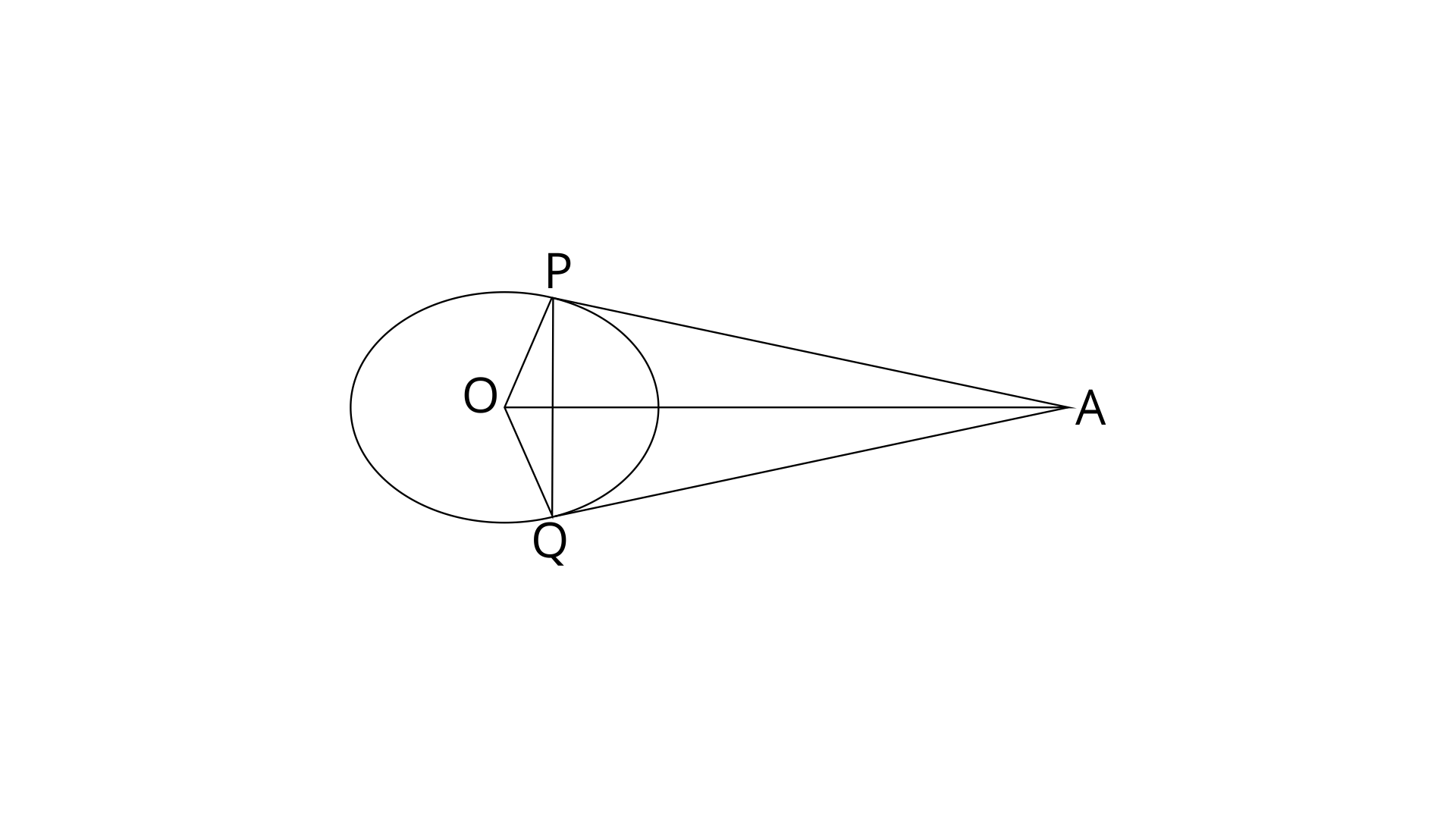
Radius is perpendicular to the tangent.

Therefore, $\angle OPA={{90}^{\circ }}$and $\angle OQA={{90}^{\circ }}$

OPAQ is a quadrilateral and the sum of all the angles in a quadrilateral is ${{360}^{\circ }}$.

$\angle OPA+\angle OQA+\angle PAQ+\angle POQ={{360}^{\circ }}$

$\Rightarrow \angle PAQ+\angle POQ={{360}^{\circ }}-{{180}^{\circ }}={{180}^{\circ }}$--------(1)

In $\Delta POQ$

OP=OQ (Radii of same circle)

$\angle OPQ=\angle OQP$(opposite angles of equal sides are equal)

Using angle sum property, 

$\angle OPQ+\angle OQP+\angle POQ={{180}^{\circ }}$

$2\angle OPQ+\angle POQ={{180}^{\circ }}$ ---------------(2)

From eq. (1) and (2)

$\angle PAQ=2\angle OPQ$

15. Two parallel tangents of a circle meet a third tangent at points P and Q. Prove that PQ subtends a right at the centre.

Ans:

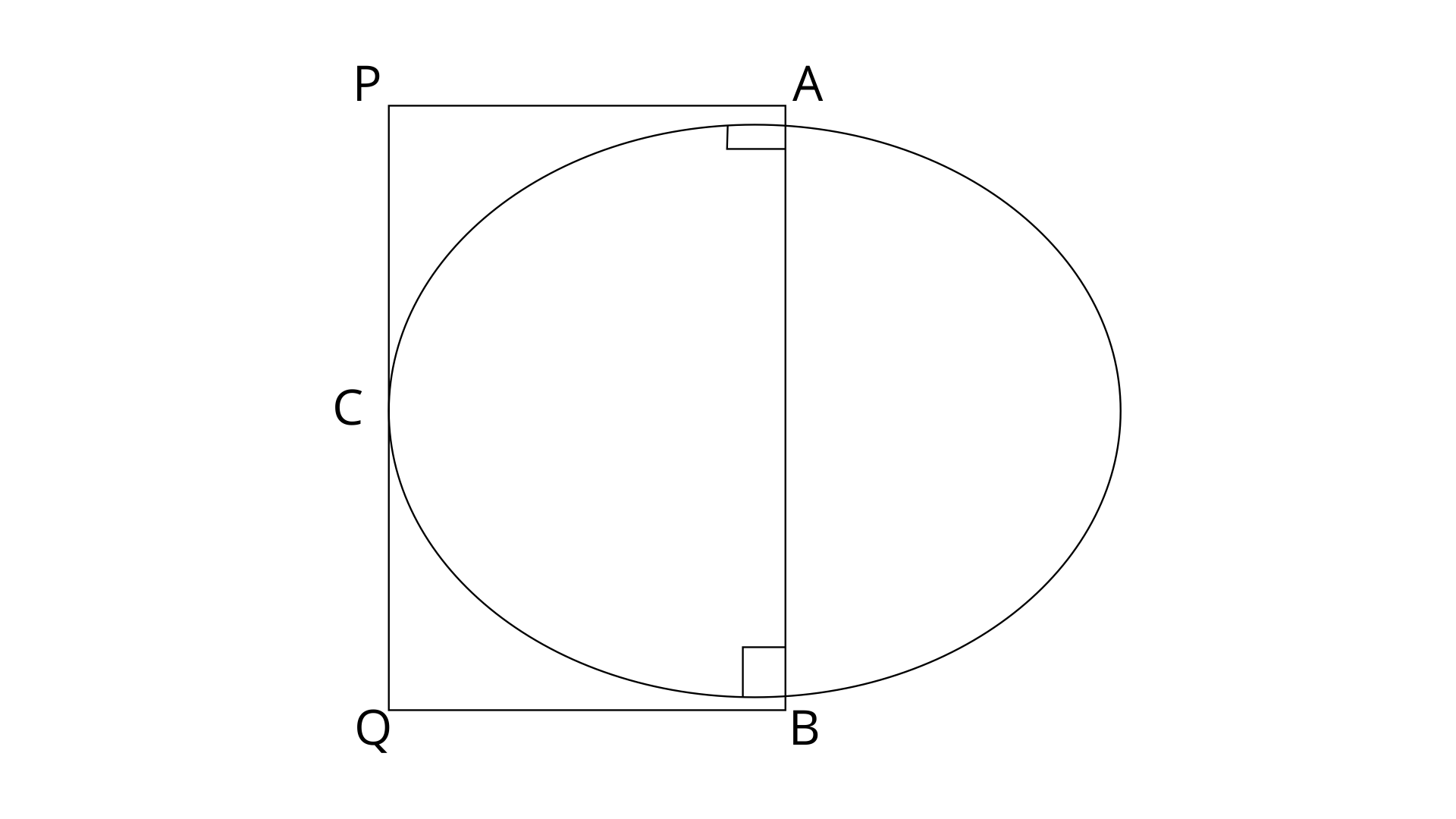
Join points OP, OQ and OC.

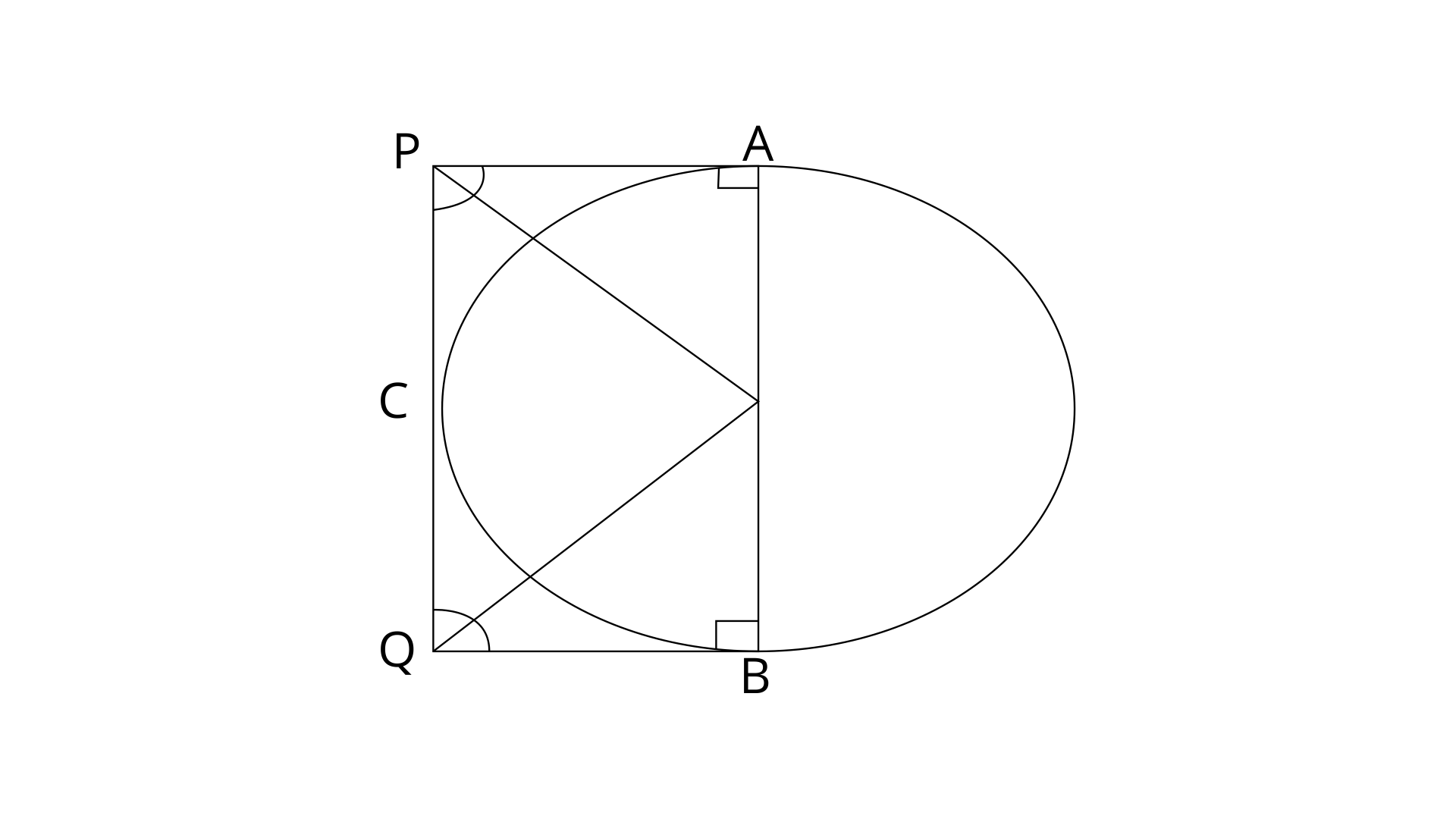
In $\Delta OAP$and $\Delta OCP$

OP=OP (Common side)

OA=OC (Radii of same circle)

PA=PC ( Tangents to the circle from external point)

$\Delta OAP~\Delta OCP$

$\angle APO=\angle CPO$ 

Therefore, $\angle APC=2\angle CPO$-------(1)

Similarly, $\Delta OBQ~\Delta OCQ$

$\angle OQC=\angle OQB$

Therefore, $\angle CQB=2\angle CQO$

Since, AP||BQ

$\angle APC+\angle CQB={{180}^{\circ }}$

$\Rightarrow 2\angle CPO+2\angle CQO={{180}^{\circ }}$

$\Rightarrow \angle CPO+\angle CQO={{90}^{\circ }}$

Now in $\Delta POQ$ by using angle sum property,

$\angle CPO+\angle CQO+\angle POQ={{180}^{\circ }}$

$\Rightarrow \angle POQ={{180}^{\circ }}-{{90}^{\circ }}={{90}^{\circ }}$

Thus PQ subtends the right angle at centre.

16. ABC is a right angled triangle with AB=12cm and AC=13cm. A circle, with centre O, has been inscribed inside the triangle.

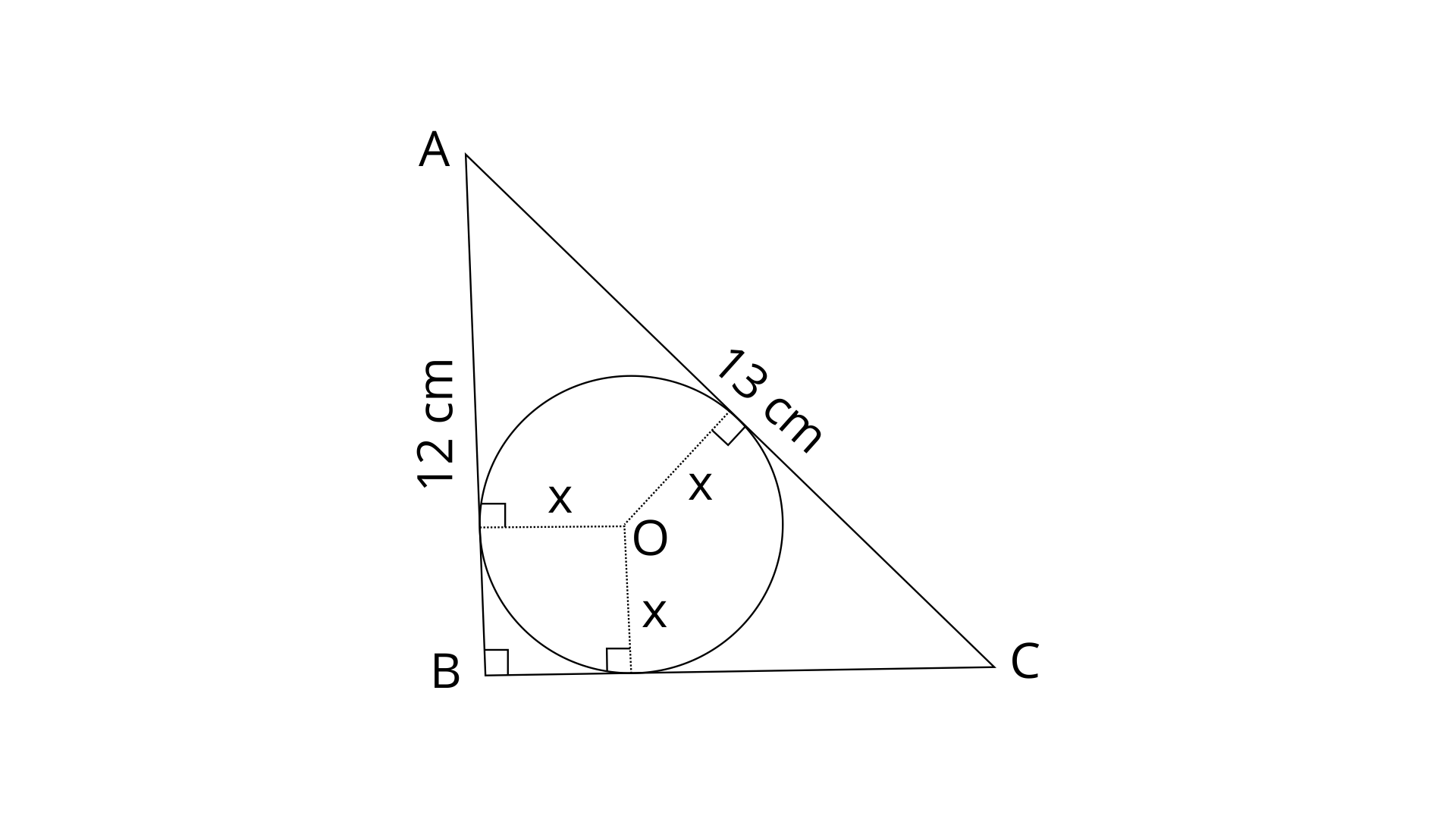
Calculate the value of x, the radius of the inscribed circle.

Ans: 

Let the altitude points are L, M and N on sides AB, BC and AC respectively.

In \[\Delta ABC\], $\angle B={{90}^{\circ }}$

$OL\bot AB,~OM\bot BC~and~ON\bot AC$

LBMO is a square.

LB=MB=MO=OL=LN=x.

AL=12-LB=12-x.

Tangents to a circle from an exterior point are equal.

AN=AL=12-x

Since, ABC is a right angle triangle. So by using pythagoras theorem

$A{{B}^{2}}+B{{C}^{2}}=A{{C}^{2}}$

$\Rightarrow B{{C}^{2}}=A{{C}^{2}}-A{{B}^{2}}=169-144=25$

$\Rightarrow BC=5$

Now, MC=BC-BM=5-x.

Tangents to a circle from an exterior point are equal.

NC=MC=5-x

Since, $AC=AN+NC$

$\Rightarrow 13=12-x+5-x$

$\Rightarrow 13=17-2x$

$\Rightarrow x=2cm$

Thus the value of x is $2cm$.

17. In a triangle ABC, the incircle(centre O) touches the BC, CA and AB at points P,Q and R respectively. Calculate that :

(i). $\angle QOR$

(ii). $\angle QPR$

given that $\angle A={{60}^{\circ }}$.

Ans:

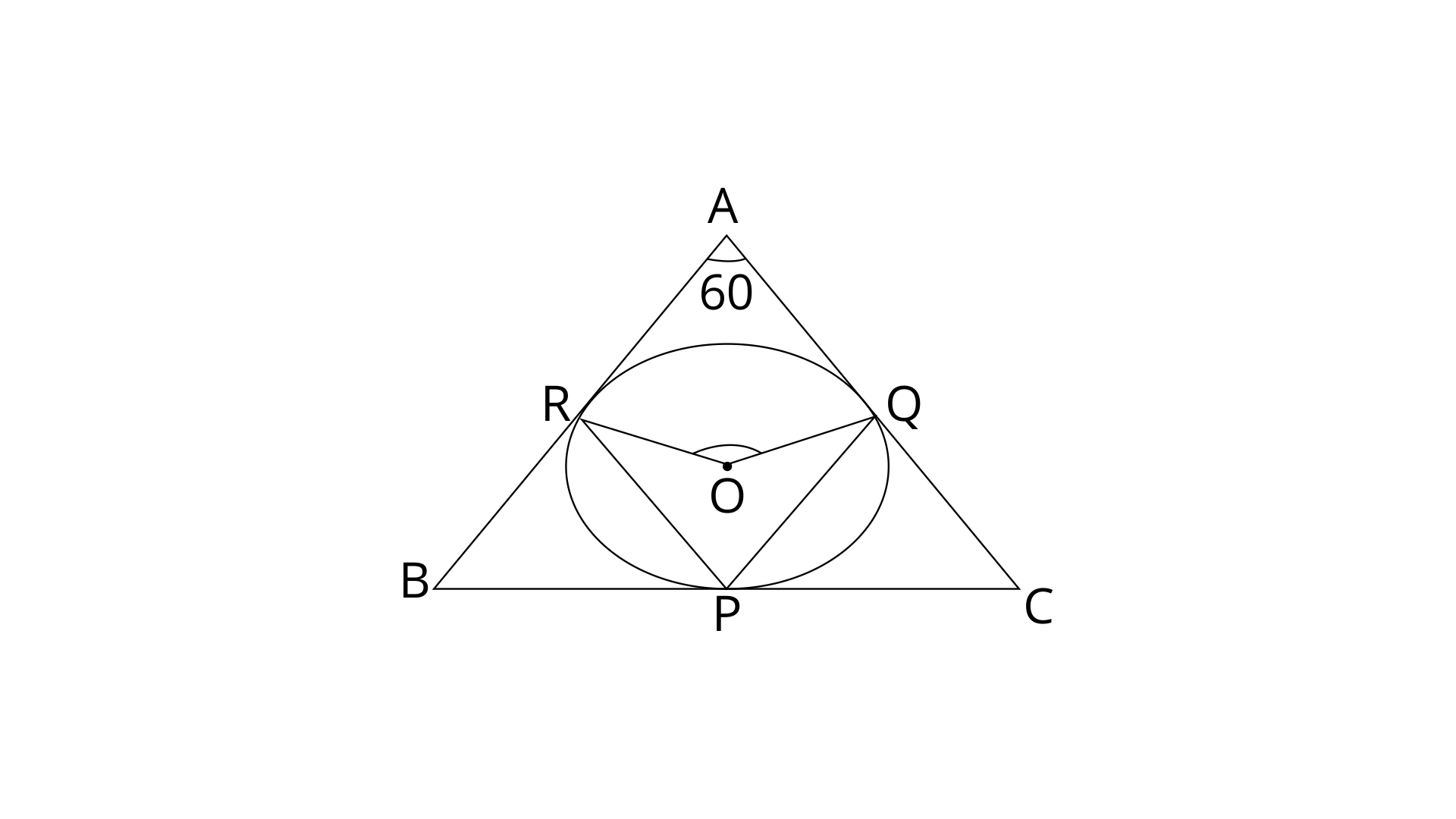
(i). $\angle QOR$

Ans: In triangle ABC, the circle touches AB, BC and AC at points R, P and Q respectively.

In quadrilateral AROQ the sum of all the angles is ${{360}^{\circ }}$

$\angle QOR+\angle OQA+\angle ORA+\angle A={{360}^{\circ }}$

$\Rightarrow \angle QOR={{360}^{\circ }}-\left( \angle OQA+\angle ORA+\angle A \right)$

Since OR, OQ are radii and AR, AQ are tangents to the circle from external point A. We know that the radius is perpendicular to the tangent.

Hence, $\angle ORA={{90}^{\circ }}$and $\angle OQA={{90}^{\circ }}$

$\Rightarrow \angle QOR={{360}^{\circ }}-\left( {{90}^{\circ }}+{{90}^{\circ }}+{{60}^{\circ }} \right)$

$\Rightarrow \angle QOR={{360}^{\circ }}-{{240}^{\circ }}={{120}^{\circ }}$

Thus the value of $\angle QOR$is ${{120}^{\circ }}$.

(ii). $\angle QPR$

Ans: In a circle, the angle at the centre is doubled the angle at circumference subtended by the same chord.

$\angle QOR=2\angle QPR$

$\Rightarrow \angle QPR=\frac{1}{2}\times \angle QOR$

$\Rightarrow \angle QPR=\frac{1}{2}\times {{120}^{\circ }}$

$\Rightarrow \angle QPR={{60}^{\circ }}$

Thus the value of angle $\angle QPR$is ${{60}^{\circ }}$.

18. In the following figure, PQ and PR are tangents to the circle, with centre O. If $\angle QPR={{60}^{\circ }}$, Calculate :

(i). $\angle QOR$

(ii). $\angle OQR$

(iii). $\angle QSR$

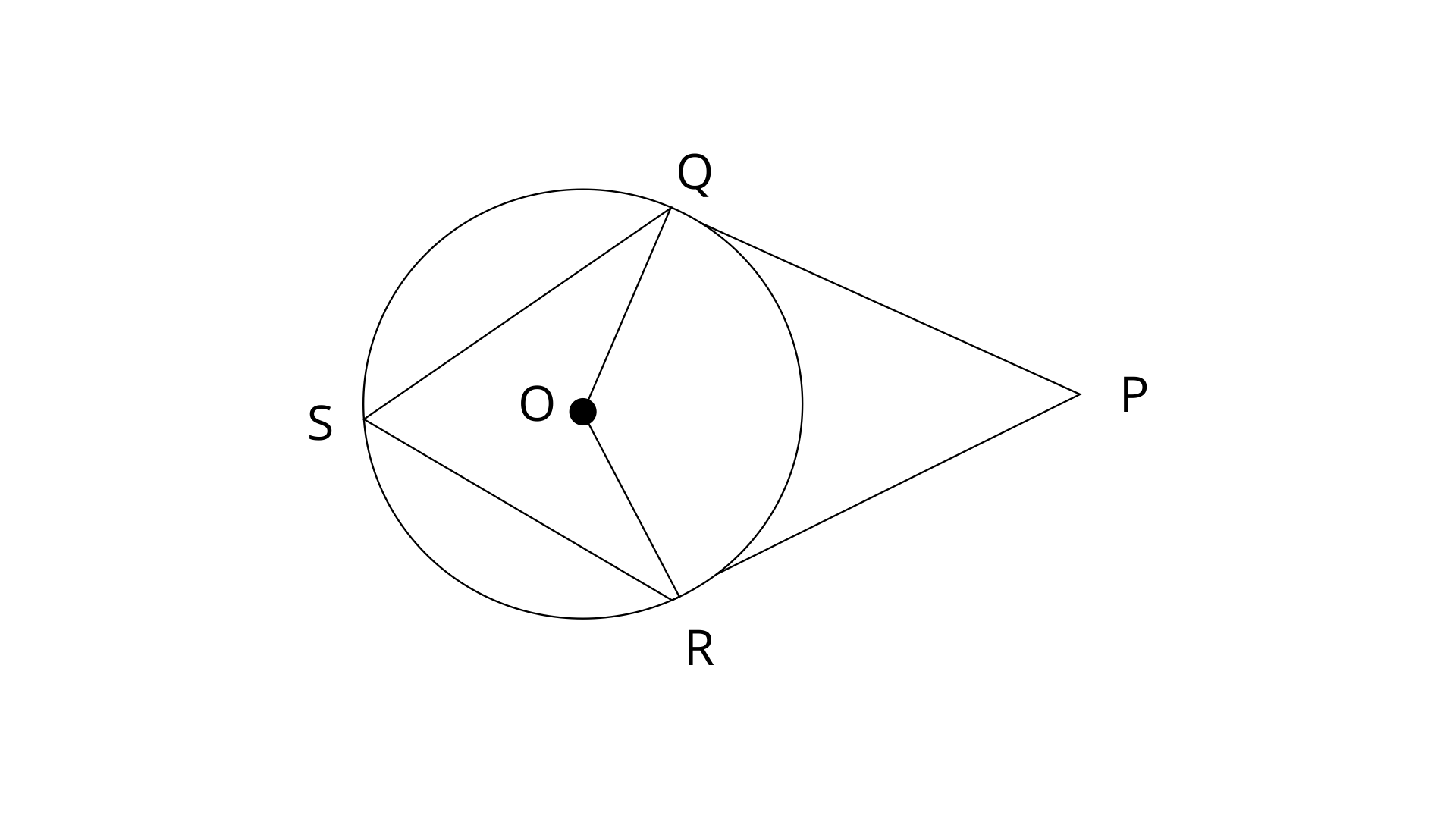
Ans: Join the points QR.

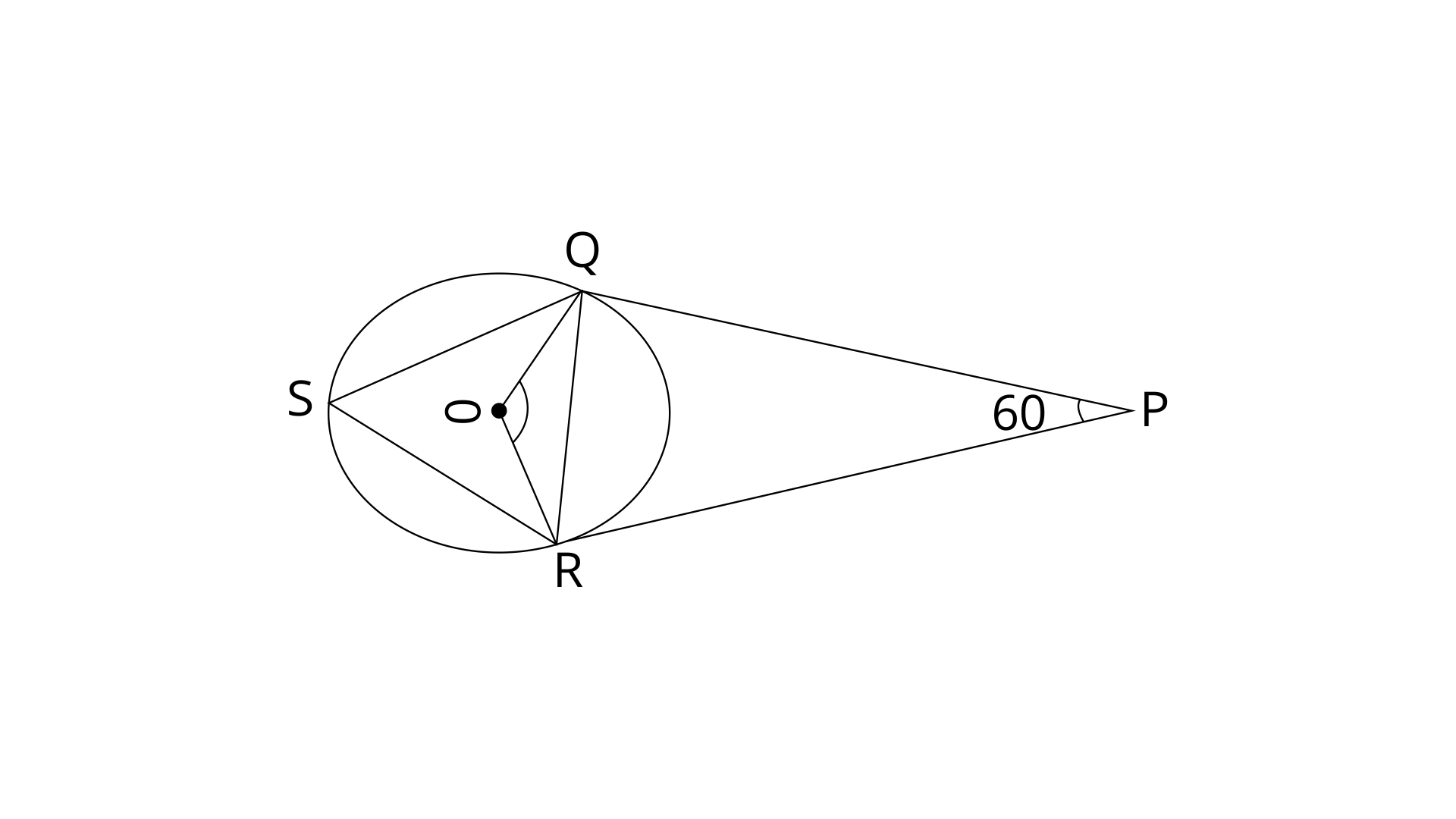
(i). $\angle QOR$

Ans: PQ and PR are the tangents to the circle from external point P.

Hence, PQ=PR 

Tangent is perpendicular to the radius. $OQ\bot PQ$and $OR\bot PR$. Hence $\angle OQP={{90}^{\circ }}~and~\angle ORP={{90}^{\circ }}$ 

PQOR is a quadrilateral and in a quadrilateral the sum of angles in a quadrilateral is ${{360}^{\circ }}$

$\angle QOR+\angle OQP+\angle ORP+\angle QPR={{360}^{\circ }}$

$\Rightarrow \angle QOR={{360}^{\circ }}-{{90}^{\circ }}-{{90}^{\circ }}-{{60}^{\circ }}={{120}^{\circ }}$

Thus the value of $\angle QOR$ is ${{120}^{\circ }}$.

(ii). $\angle OQR$

Ans: In $\Delta QOR$

OQ=OR (Radii of same circle)

Therefore, $\angle OQR=\angle ORQ$(opposite angles of equal sides are equal)

Now, By using angle sum property,

$\angle OQR+\angle ORQ+\angle QOR={{180}^{\circ }}$

$\Rightarrow 2\angle OQR={{180}^{\circ }}-{{120}^{\circ }}={{60}^{\circ }}$

$\Rightarrow \angle OQR={{30}^{\circ }}$

Thus the value of $\angle OQR$is ${{30}^{\circ }}$

(iii). $\angle QSR$

Ans: In a circle, Angle at the centre is doubled the angle at the circumference subtended by the same chord.

$\angle QOR=2\angle QSR$

$\Rightarrow \angle QSR=\frac{1}{2}\times \angle QOR=\frac{{{120}^{\circ }}}{2}={{60}^{\circ }}$

Thus the value of $\angle QSR$is ${{60}^{\circ }}$.

19. In the given figure, AB is the diameter of the circle, with centre O, and AT is the tangent. Calculate the numerical value of x. 

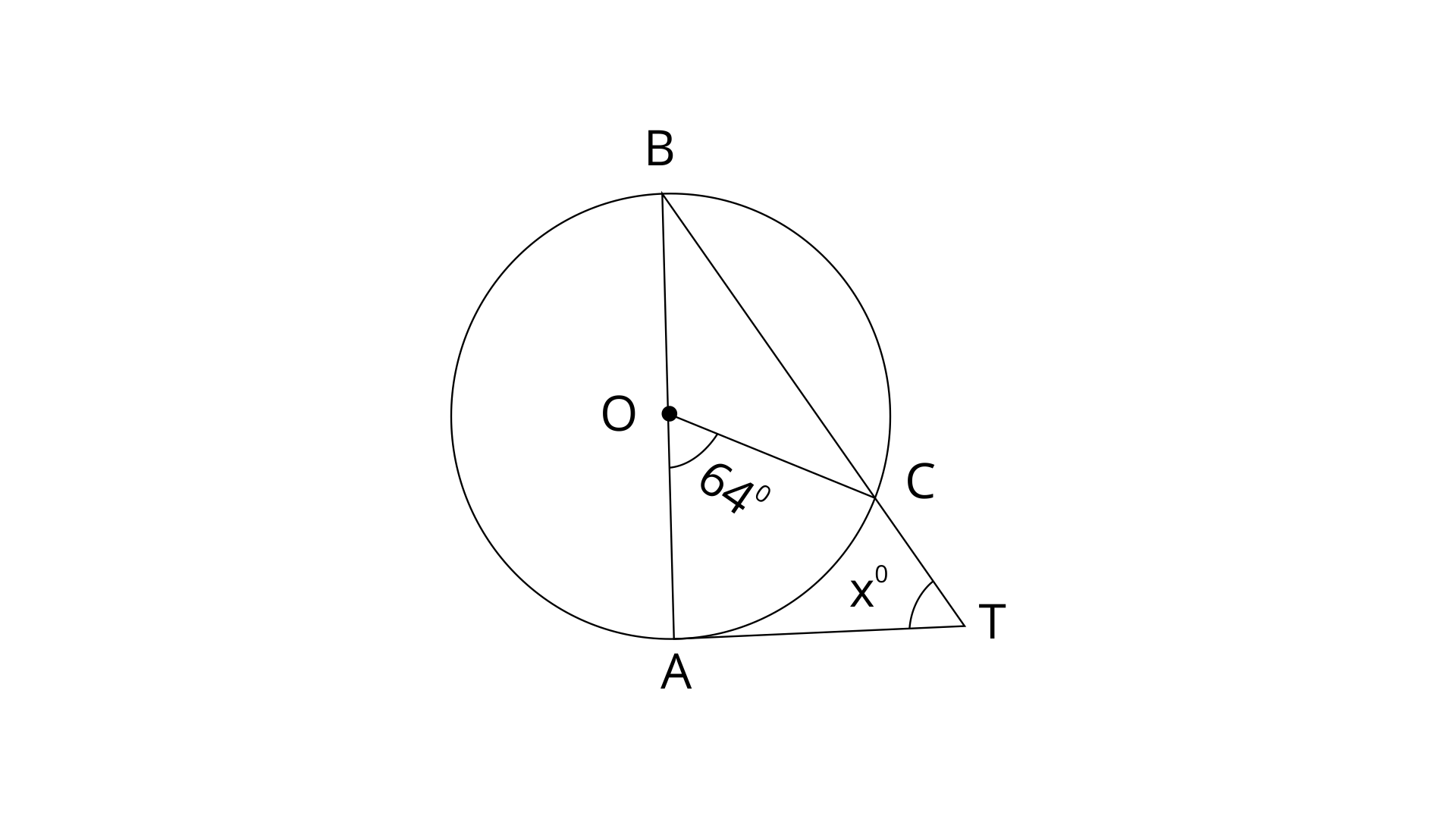
Ans: 

In $\Delta OBC$

OB=OC (Radii of same circle)

$\Rightarrow \angle OBC=\angle OCB$

Exterior angle in a triangle is the sum of the opposite angles. Here angle $\angle AOC$is the exterior angle of triangle $\Delta OBC$.

$\angle AOC=\angle OBC+\angle OCB$

$\Rightarrow {{64}^{\circ }}=2\angle OBC$

$\Rightarrow \angle OBC={{32}^{\circ }}$

Now in $\Delta ABT$by using angle sum property,

$\angle ABT+\angle BAT+x={{180}^{\circ }}$

Since AT is tangent and AB is a diameter. We know that the radius is perpendicular to the tangent. Hence, $\angle BAT={{90}^{\circ }}$

$\angle ABT=\angle OBC={{32}^{\circ }}$(Angles b/w same line segment)

$\Rightarrow \angle ABT+\angle BAT+x={{180}^{\circ }}$

$\Rightarrow x={{180}^{\circ }}-{{90}^{\circ }}-{{32}^{\circ }}={{58}^{\circ }}$

Thus the value of $x={{58}^{\circ }}$

20. In quadrilateral ABCD; angle $D={{90}^{\circ }}$, BC=38cm and DC=25cm. A circle is inscribed in this quadrilateral which touches AB at point Q such that QB=27cm. Find the radius of the circle.

Ans: 

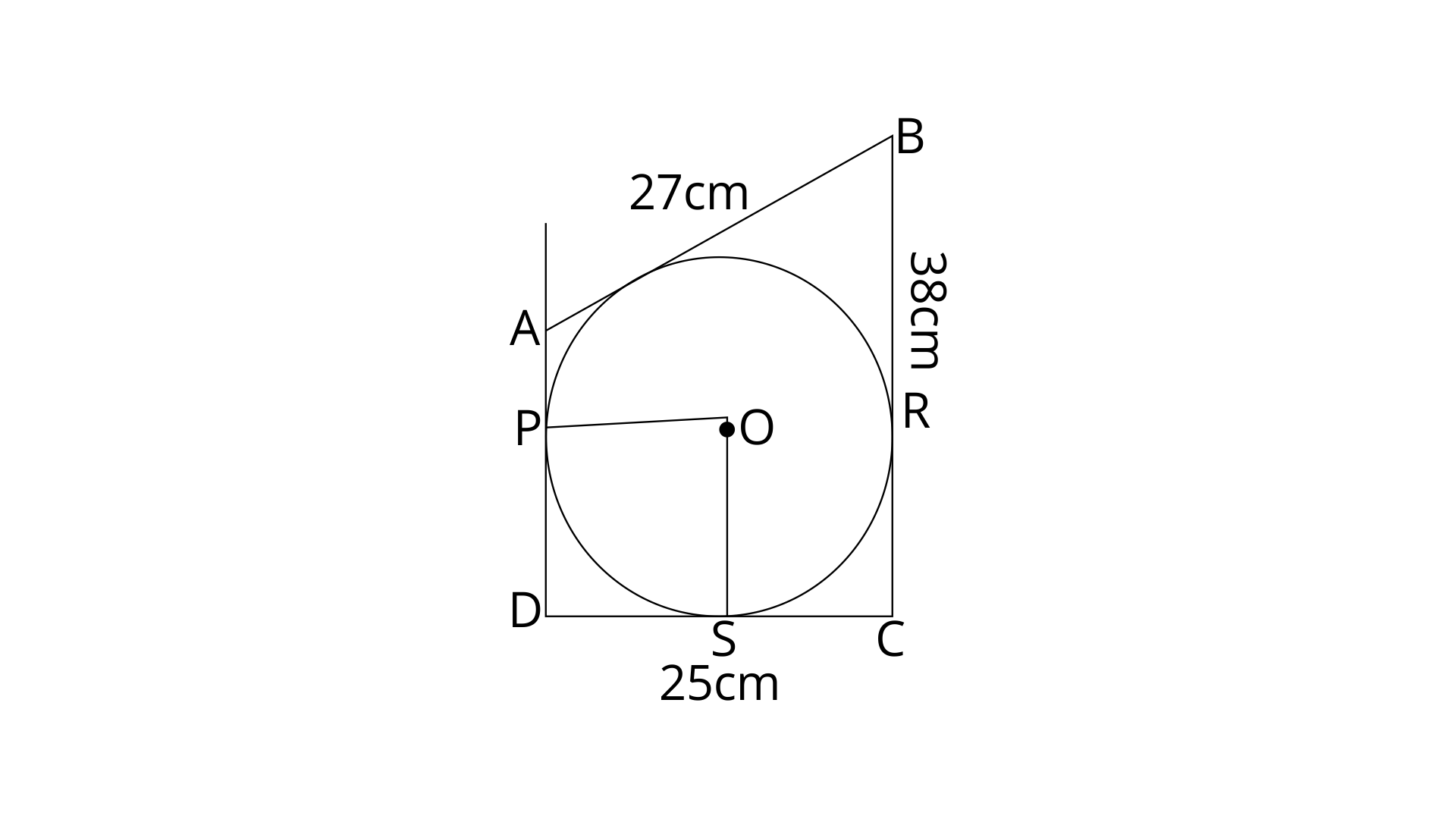
In quadrilateral ABCD,

$\angle D={{90}^{\circ }}$, BC=38cm, DC=25cm and QB=27cm

Tangents to a circle from an external point are equal. Here BQ and BR the tangents from the external points B.

Therefore, BQ=BR=27cm

∵BC=38cm

$\Rightarrow BR+RC=38cm$

$\Rightarrow RC=38-27=11cm$

Since, Tangents CS and CR are tangents from external point C.

Therefore CS=CR=11cm.

$DC=DS+CS$

$\Rightarrow DS=DC-CS=25-11=14cm$

Now, DS and DP are tangents to the circle from external point D.

Therefore, DP=DS=14cm

AD, DC are tangents and OP, OS are radius. Radius is perpendicular to the tangent.

$\angle PDS={{90}^{\circ }}$and $OP\bot AD$, $OS\bot DC$ and OS=DP

Therefore the radius OS is 14cm.

21. In the given figure, PT touches the circle with centre O at point R. Diameter SQ is produced to meet the tangent TR at P. Given $\angle SPR={{x}^{\circ }}$and $\angle QRP={{y}^{\circ }}$;

Prove that:

(i).  $\angle ORS={{y}^{\circ }}$

(ii). Write an expression connecting x and y.

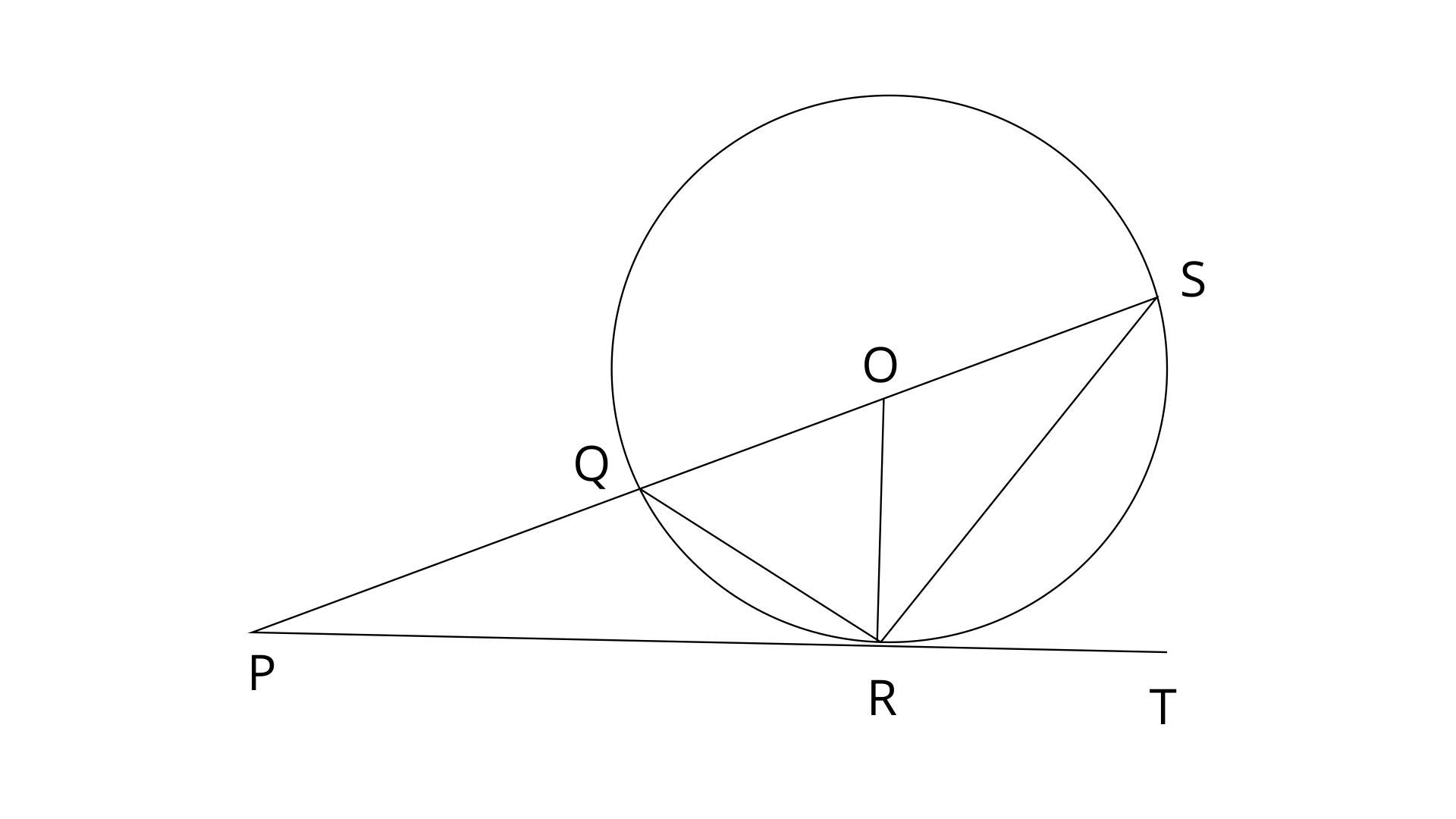
Ans:

Since PT is a tangent at point R and OR is the radius of the circle.

Hence, $\angle ORT=\angle ORP={{90}^{\circ }}$

Given, $\angle SPR={{x}^{\circ }}$and $\angle QRP={{y}^{\circ }}$

Since, $\angle ORP={{90}^{\circ }}$and $\angle QRP={{y}^{\circ }}$

Therefore, $\angle ORQ={{90}^{\circ }}-{{y}^{\circ }}$

$\angle QRP=\angle QSR={{y}^{\circ }}$(Angles in alternate segments)

Since, OS=OR (Radii of same circle)

$\angle ORS=\angle OSR={{y}^{\circ }}$(Opposite angles of equal sides)

(ii). Since, OR=OQ (Radii of same circle)

$\angle OQR=\angle ORQ={{90}^{\circ }}-{{y}^{\circ }}$ (opposite angles of equal sides)

ext.$\angle OQR=\angle QPR+\angle QRP$

${{90}^{\circ }}-y=x+y$

$\Rightarrow {{90}^{\circ }}=x+2y$

22. PT is a tangent to the circle at T. If $\angle ABC={{70}^{\circ }}$and $\angle ACB={{50}^{\circ }}$; Calculate :

(i). $\angle CBT$

(ii). $\angle BAT$

(iii). $\angle APT$

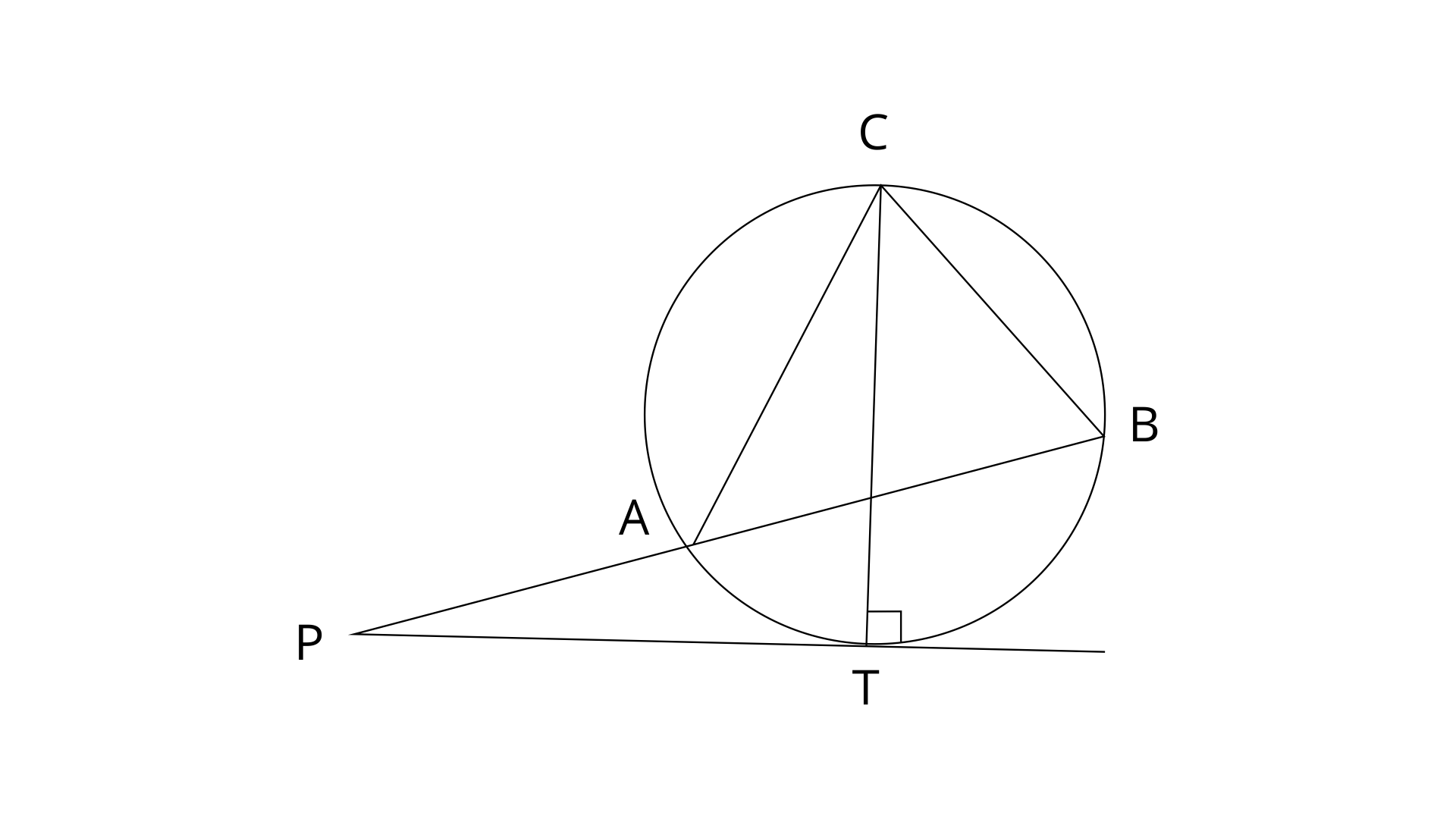
Ans: join the points AT, BT

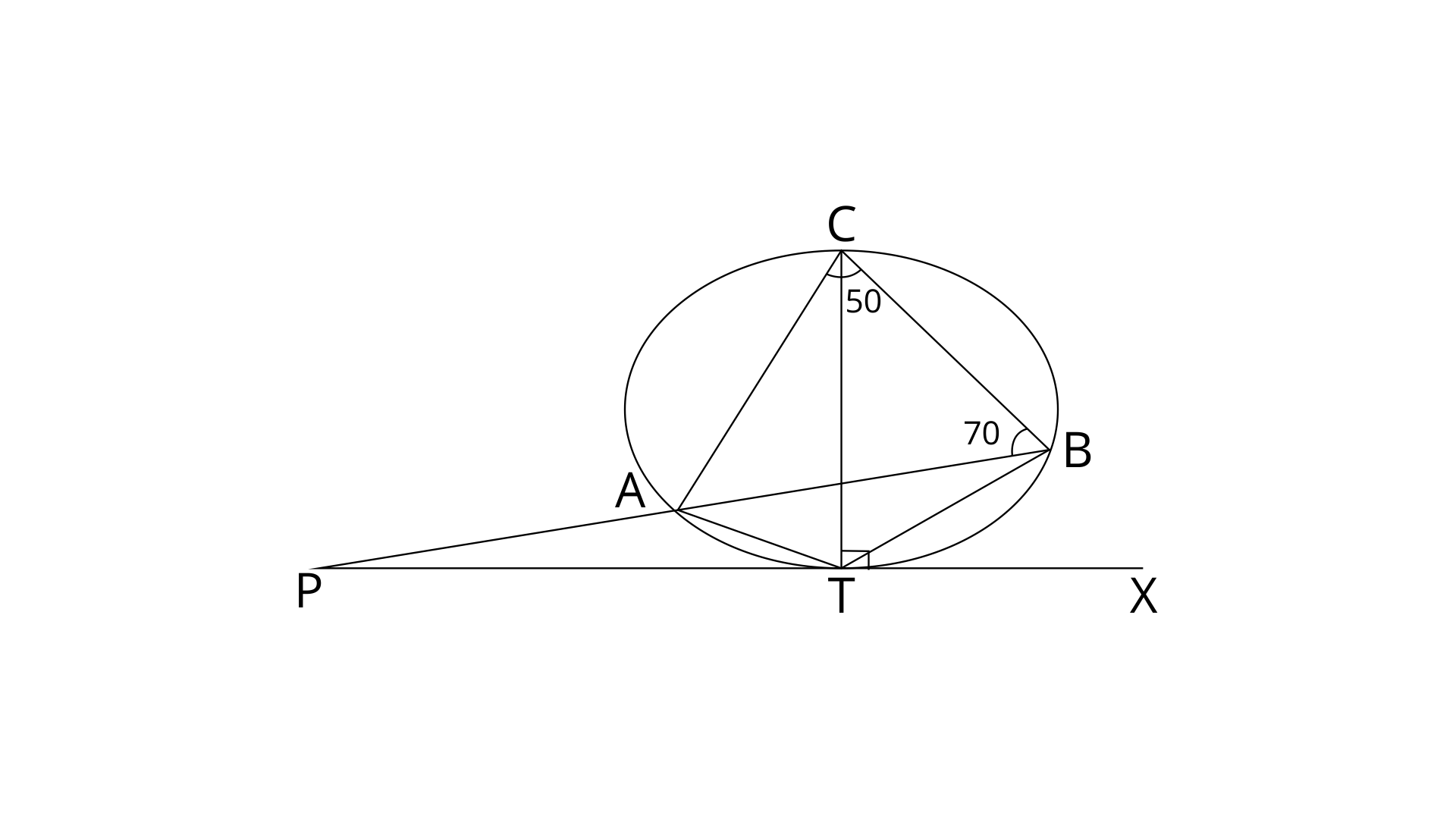
(i). $\angle CBT$

Ans: PX is a tangent to the circle at point T. 

Angle $\angle CBT$is subtended by the diameter at the circumference and we know that the angle subtended by the diameter at the circumference is ${{90}^{\circ }}$.

Hence, $\angle CBT={{90}^{\circ }}$

$\angle ABT={{90}^{\circ }}-\angle CBA={{90}^{\circ }}-{{70}^{\circ }}={{20}^{\circ }}$

(ii). $\angle BAT$

Ans: In $\Delta ABC$by using angle sum property

$\angle ACB+\angle ABC+\angle CAB={{180}^{\circ }}$

$\Rightarrow \angle CAB={{180}^{\circ }}-{{50}^{\circ }}-{{70}^{\circ }}={{60}^{\circ }}$

$\angle CAT$subtended by the diameter at the circumference and we know that the angle subtended by the diameter at the circumference is ${{90}^{\circ }}$.

Hence, $\angle CAT={{90}^{\circ }}$

$\angle CAT=\angle CAB+\angle BAT$

$\Rightarrow \angle CAB+\angle BAT={{90}^{\circ }}$

$\Rightarrow \angle BAT={{90}^{\circ }}-{{60}^{\circ }}={{30}^{\circ }}$

(iii). $\angle APT$

Ans: PT is tangent and AT is a chord,

$\angle ATP=\angle ABT={{20}^{\circ }}$(Angles in alternate segments)

$\angle BAT$is exterior angle of triangle APT,

$\angle BAT=\angle APT+\angle ATP$

$\Rightarrow \angle APT={{30}^{\circ }}-{{20}^{\circ }}={{10}^{\circ }}_{{}}$

23. In the given figure, O is the centre of the circumcircle ABC. Tangents at A and C intersect at P. Given angle $AOB={{140}^{\circ }}$and angle $APC={{80}^{\circ }}$; Find the angle BAC.

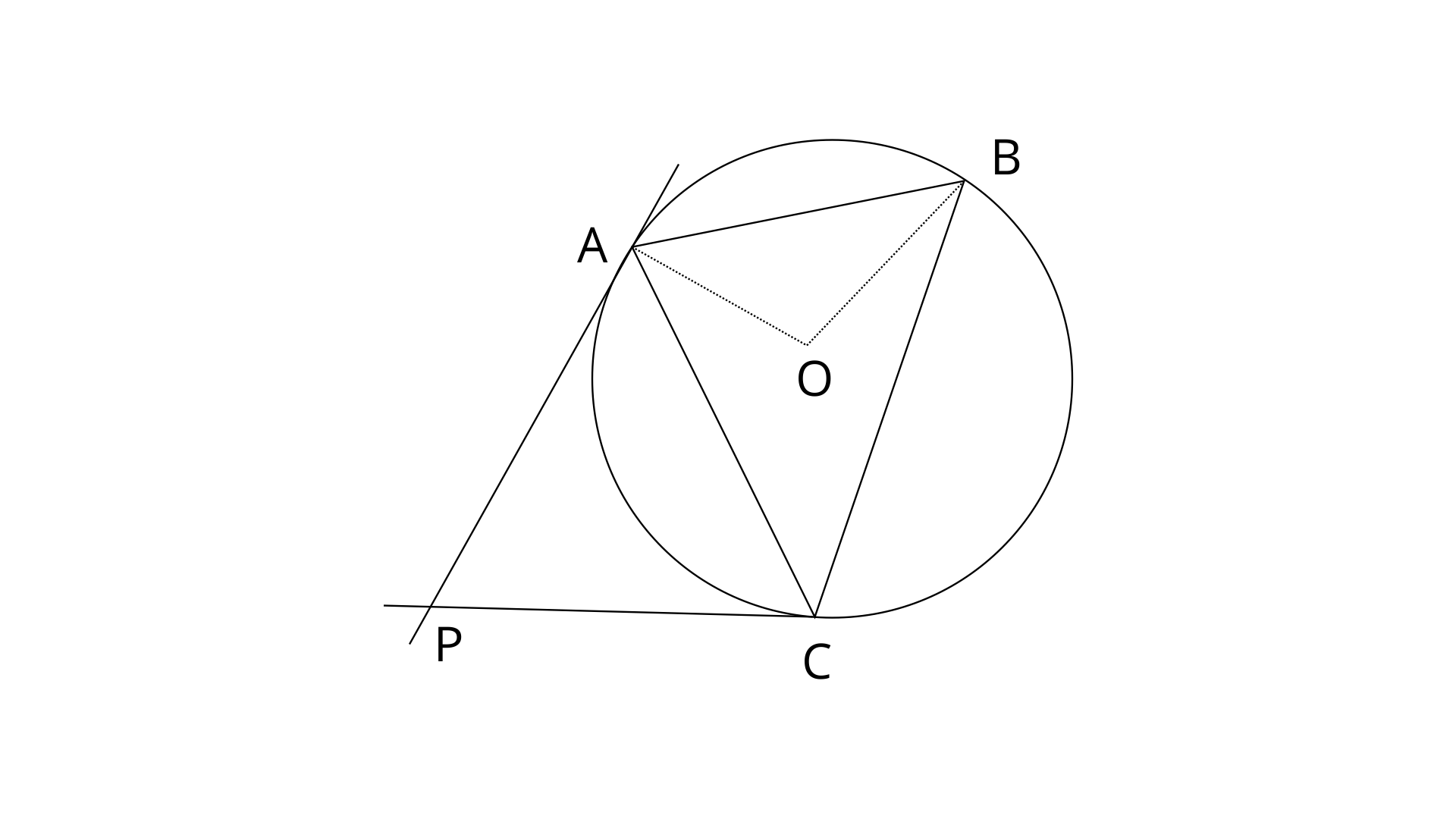
Ans: Join points OC.

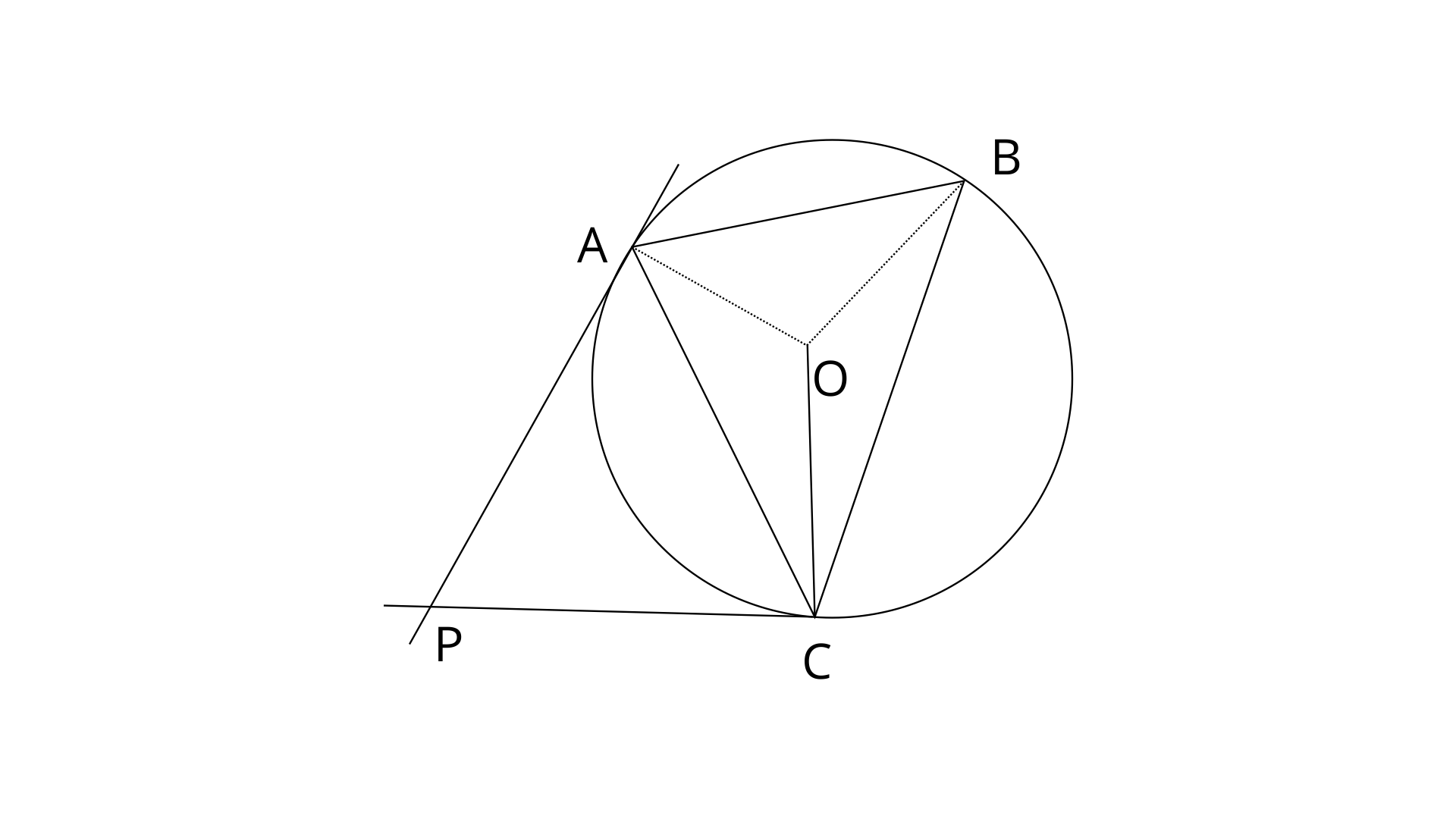
PA and PC are two tangents to the circle at points A and C.

In quadrilateral APCO,

$\angle APC+\angle AOC={{180}^{\circ }}$

$\Rightarrow \angle AOC={{180}^{\circ }}-{{80}^{\circ }}={{100}^{\circ }}$

Now, 

$\angle BOC={{360}^{\circ }}-\angle AOC-\angle AOB$

$\Rightarrow \angle BOC={{360}^{\circ }}-{{100}^{\circ }}-{{140}^{\circ }}={{120}^{\circ }}$

Angle at the centre is doubled the angle at the circumcentre subtended by the same chord. Here $\angle BOC$and $\angle BAC$are subtended by the chord BC.

Therefore, $\angle BOC=2\angle BAC$

$\Rightarrow {{120}^{\circ }}=2\times \angle BAC$

$\Rightarrow \angle BAC={{60}^{\circ }}$

24. In the given figure, PQ is a tangent to the circle at A. AB and AD are bisectors of $\angle CAQ$and $\angle PAC$. If $\angle BAQ={{30}^{\circ }}$prove that : BD is the diameter of circle.

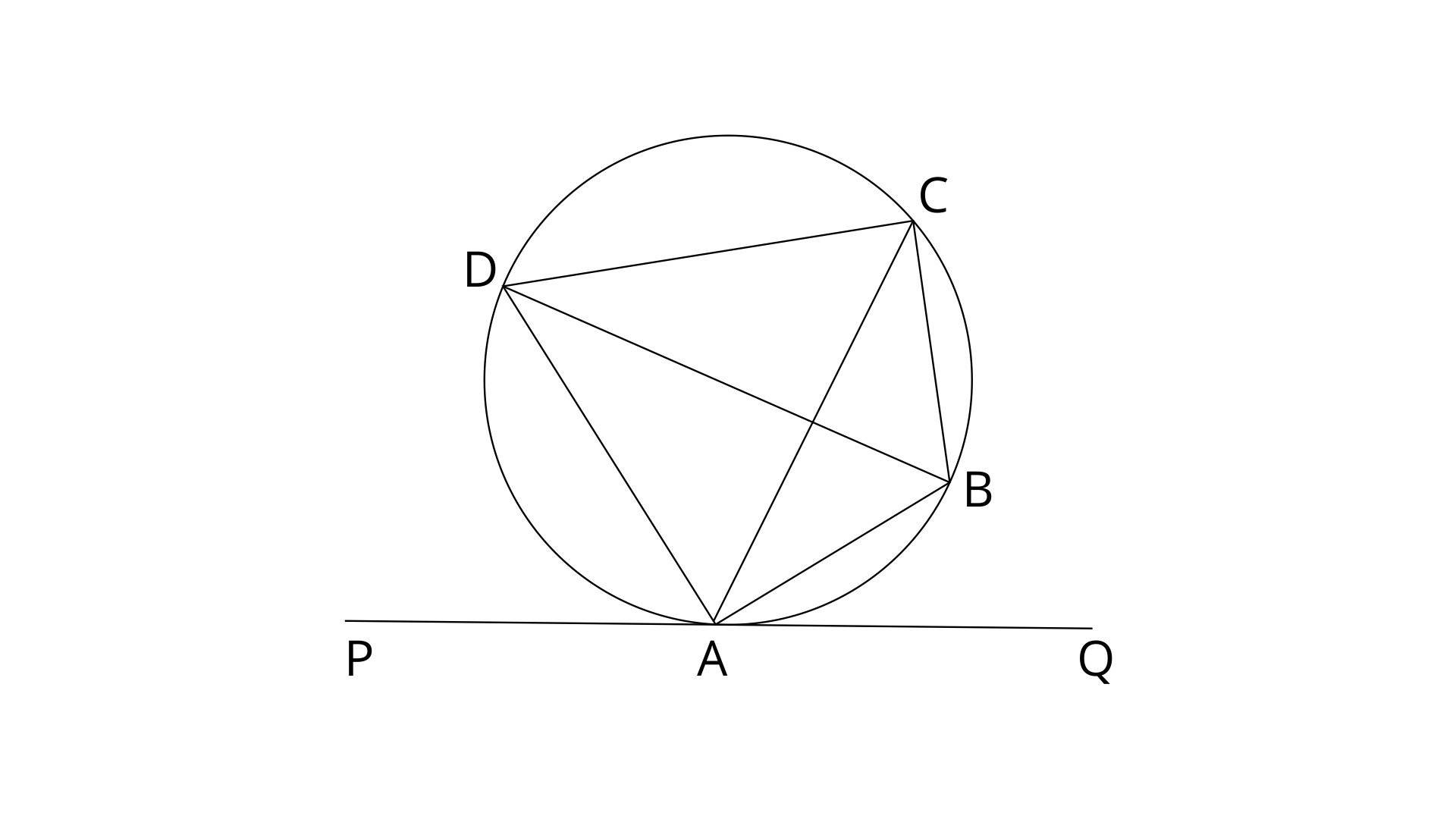
Ans: Since PQ is tangent to the circle at point A.

AB is bisector of $\angle CAQ$. 

Therefore, $\angle CAB=\angle BAQ={{30}^{\circ }}$ and $\angle CAQ=2\angle BAQ$ 

$\angle CAQ=2\times {{30}^{\circ }}={{60}^{\circ }}$

Since PQ is a straight line. 

Hence, $\angle CAQ+\angle CAP={{180}^{\circ }}$(linear pair)

$\angle CAP={{180}^{\circ }}-{{60}^{\circ }}={{120}^{\circ }}$

AD is bisector of $\angle CAP$. 

$\Rightarrow \angle CAD=\angle DAP$and $\angle CAP=\angle CAD+\angle DAP=2\angle CAD$

$\angle CAP=2\angle CAD$

$\Rightarrow \angle CAD=\frac{{{120}^{\circ }}}{2}={{60}^{\circ }}$

Now, $\angle CAD+\angle CAB=\angle BAD$

$\Rightarrow \angle BAD={{60}^{\circ }}+{{30}^{\circ }}={{90}^{\circ }}$

$\angle BAD$is subtended by BD at the circumference and is at a right angle. We know that the angle at the circumference subtended by diameter is a right angle.

Thus we can say $\angle BAD$subtended by the diameter BD. 

Therefore, BD is the diameter of the circle.

Exercise 18.2

1. (i) In the given figure, $3\times CP=PD=9cm$and $AP=4.5cm$. Find BP.

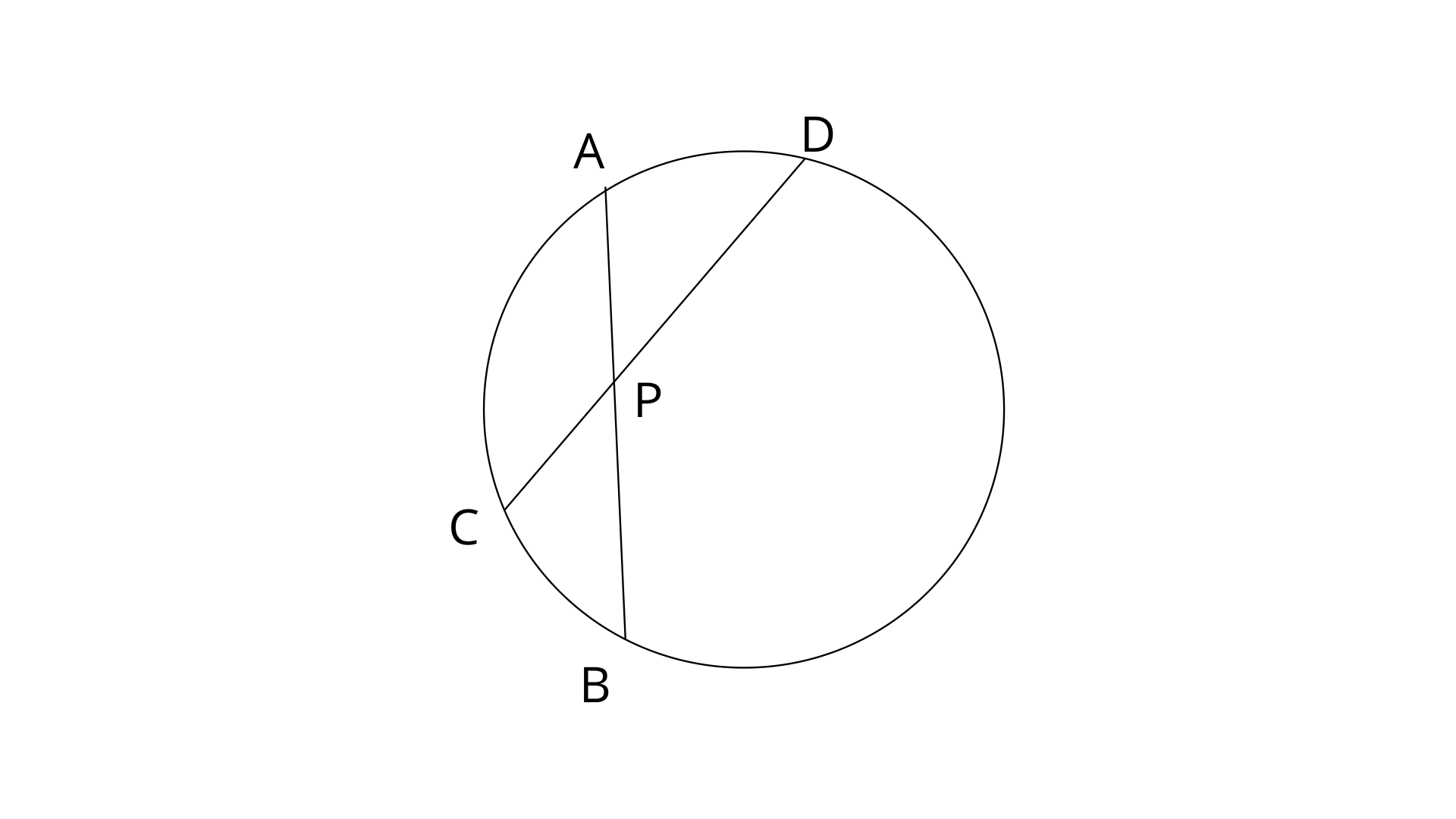
Ans: 

Given that $3\times CP=PD=9cm$

$\Rightarrow CP=\frac{9}{3}=3cm$

$PD=9cm$ and $AP=4.5cm$

Since two chords AB and CD intersect at point P. 

Therefore, $AP\times PB=CP\times PD$

$\Rightarrow 4.5\times PB=3\times 9$

$\Rightarrow PB=\frac{27}{4.5}=6cm$

Thus the value of PB is $6cm$.

(ii). In the given figure, $3\times PA=3\times AB=30cm$and $PC=4cm$. Find the CD.

Ans: Given that $5\times PA=3\times AB=30cm$and $PC=4cm$

$5\times PA=30cm$

$\Rightarrow PA=6cm$

And, $3\times AB=30cm$

$\Rightarrow AB=10cm$

$BP=BA+AP=10+6=16cm$

Since two chords intersect each other at point P.

Therefore,

$AP\times PB=CP\times PD$

$\Rightarrow 6\times 16=4\times PD$

$\Rightarrow PD=24cm$

Now, $CD=PD-CP$

$\Rightarrow CD=24-4=20cm$

Thus the value of $CD$is $20cm$.

(iii). In the given figure, tangent $PT=12.5cm$and $PA=10cm$; find AB.

Ans: PAB is the secant and PT is the tangent, both intersect each other externally at point P.

$P{{T}^{2}}=PA\times PB$

$\Rightarrow {{\left( 12.5 \right)}^{2}}=10\times PB$

$\Rightarrow {{\left( 12.5 \right)}^{2}}=10\times PB$

$\Rightarrow \frac{156.25}{10}=PB$

$\Rightarrow PB=15.625$

Now, $AB=PB-PA$

$\Rightarrow AB=15.625-10=5.625cm.$

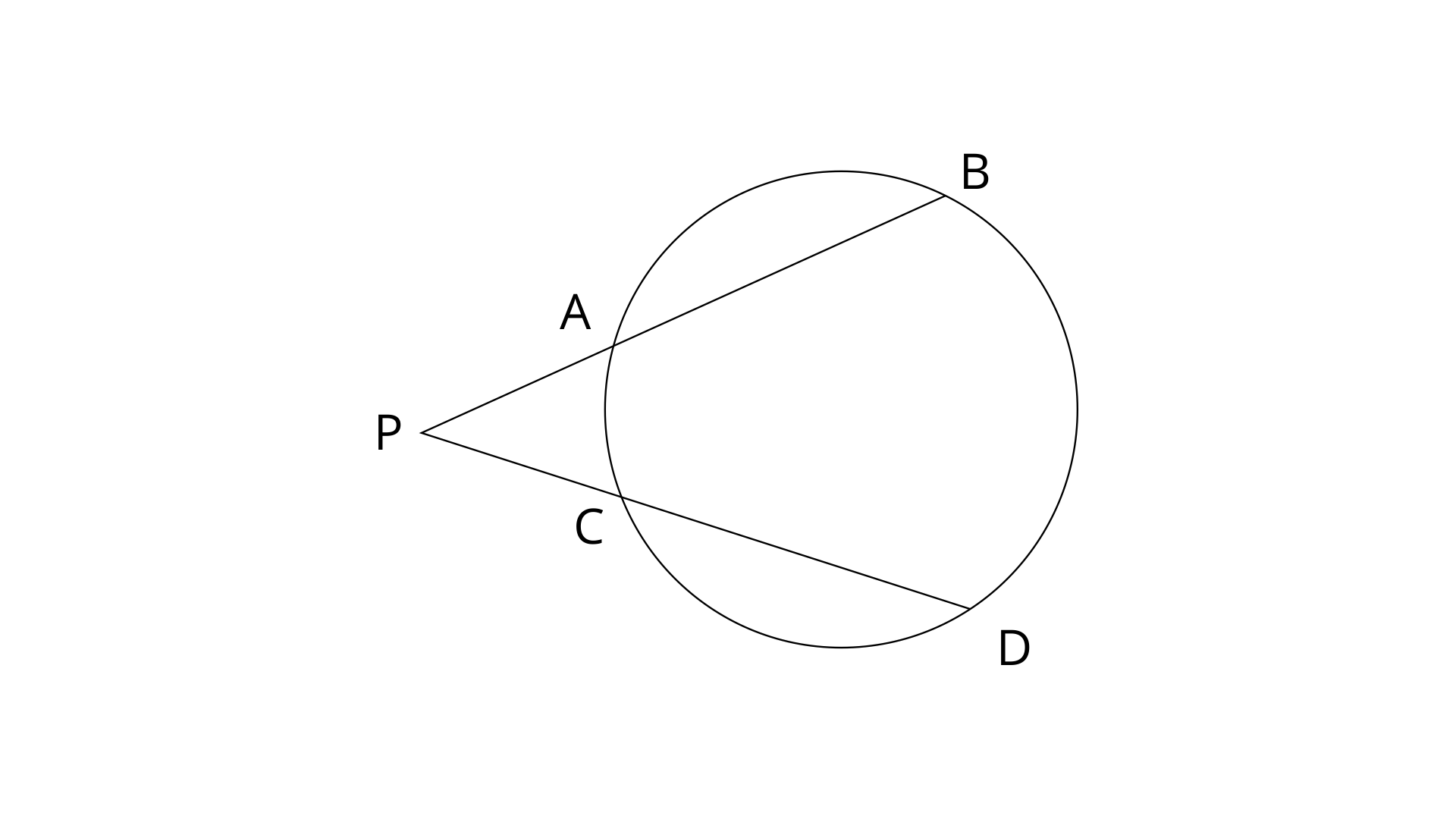
2. In the given figure, diameter AB and chord CD of a circle meet at P. PT is a tangent to the circle at T. CD=7.8cm, PD=5cm, PB=4cm. Find :

(i). AB

Ans: Chords AB and CD intersect externally at point P.

Therefore, $PA\times PB=PC\times PD$--------(1)

$PA=AB+BP=AB+4$

$PC=CD+PD=7.8+5=12.8cm$

Now substituting these values in eq. (1)

$PA\times PB=PC\times PD$

$\Rightarrow \left( AB+4 \right)\times 4=12.8\times 5$

$\Rightarrow \left( AB+4 \right)=16$

$\Rightarrow AB=12cm$

Thus the value of AB is 12cm.

(ii). The length of the tangent

Ans: Tangent PT and chord CD intersect each other externally at point P.

Therefore, $P{{T}^{2}}=PC\times PD$

$\Rightarrow P{{T}^{2}}=12.8\times 5=64cm$

$\Rightarrow PT=8cm$

Thus the length of the tangent is 8cm.

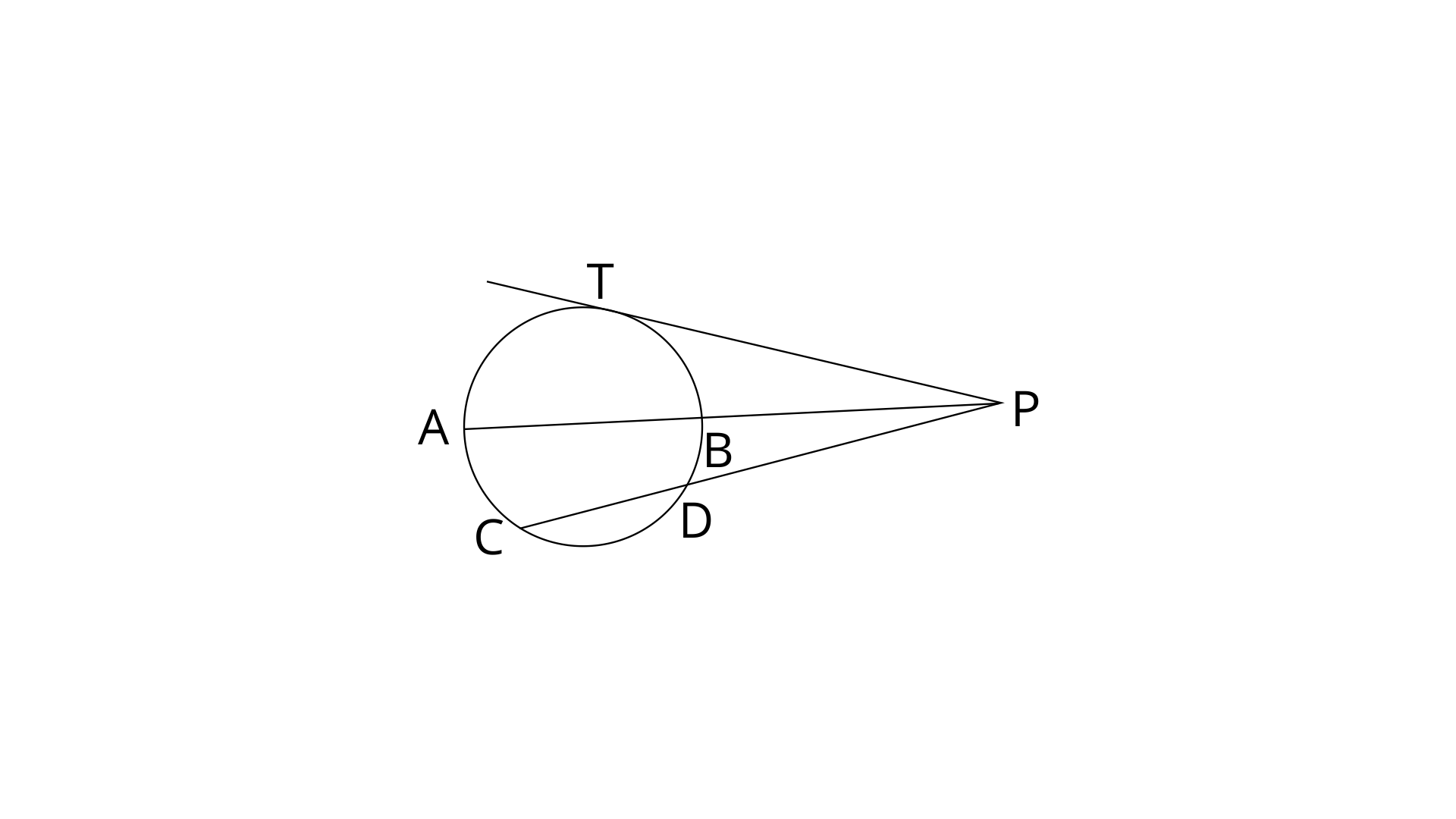
3. In the following figure, PQ is tangent to the circle at A, DB is the diameter and O is the centre of the circle. If $\angle ADB={{30}^{\circ }}$and $\angle CBD={{60}^{\circ }},$calculate :

(i). $\angle QAB$

Ans: In a circle, Angle subtended by the diameter at the circumference is the right angle. Therefore, $\angle OAQ={{90}^{\circ }}$

In $\Delta ABD$by using angle sum property, 

$\angle ADB+\angle ABD+\angle BAD={{180}^{\circ }}$

$\Rightarrow \angle ABD={{180}^{\circ }}-{{90}^{\circ }}-{{30}^{\circ }}={{60}^{\circ }}$

OA=OB (Radii of same circle)

$\angle ABO=\angle AOB={{60}^{\circ }}$

Now, in $\Delta ABO$by using angle sum property,

$\angle ABo+\angle AOB+\angle OAB={{180}^{\circ }}$

$\Rightarrow \angle OAB={{180}^{\circ }}-{{60}^{\circ }}-{{60}^{\circ }}={{60}^{\circ }}$

$\Rightarrow \angle OAQ=\angle OAB+\angle QAB={{60}^{\circ }}+\angle QAB$

$\Rightarrow {{90}^{\circ }}-{{60}^{\circ }}=\angle QAB$

$\Rightarrow \angle QAB={{30}^{\circ }}$

Thus the value of $\angle QAB$is ${{30}^{\circ }}$

(ii). $\angle PAD$

Ans: Since, OD=OA(Radii of same circle)

$\angle ODA=\angle OAD$(opposite angles of equal sides)

$\Rightarrow \angle OAD={{30}^{\circ }}$[∵$\angle ODA={{30}^{\circ }}$]

OA is radius and PQ is tangent. Radius is perpendicular to the tangent.

Therefore, $\angle OAP={{90}^{\circ }}$

$\angle PAD=\angle PAO-\angle OAD={{90}^{\circ }}-{{30}^{\circ }}={{60}^{\circ }}$

Thus the value of $\angle PAD$is ${{60}^{\circ }}$.

(iii). $\angle CDB$

Ans: BD is diameter of the circle and $\angle BCD$is subtended by the diameter. We know that the angle at the circumference subtended by the diameter is the right angle. Hence $\angle BCD={{90}^{\circ }}$

Now by using angle sum property in $\Delta BCD$

$\angle BCD+\angle CDB+\angle CBD={{180}^{\circ }}$

$\Rightarrow \angle CDB={{180}^{\circ }}-{{90}^{\circ }}-{{60}^{\circ }}={{30}^{\circ }}$

Thus the value of $\angle CDB$is ${{30}^{\circ }}$.

4. If PQ is a tangent to the circle at R; calculate :

(i). $\angle PRS$

(ii). $\angle ROT$

Given O is the centre of the circle and angle $\angle TRQ={{30}^{\circ }}$.

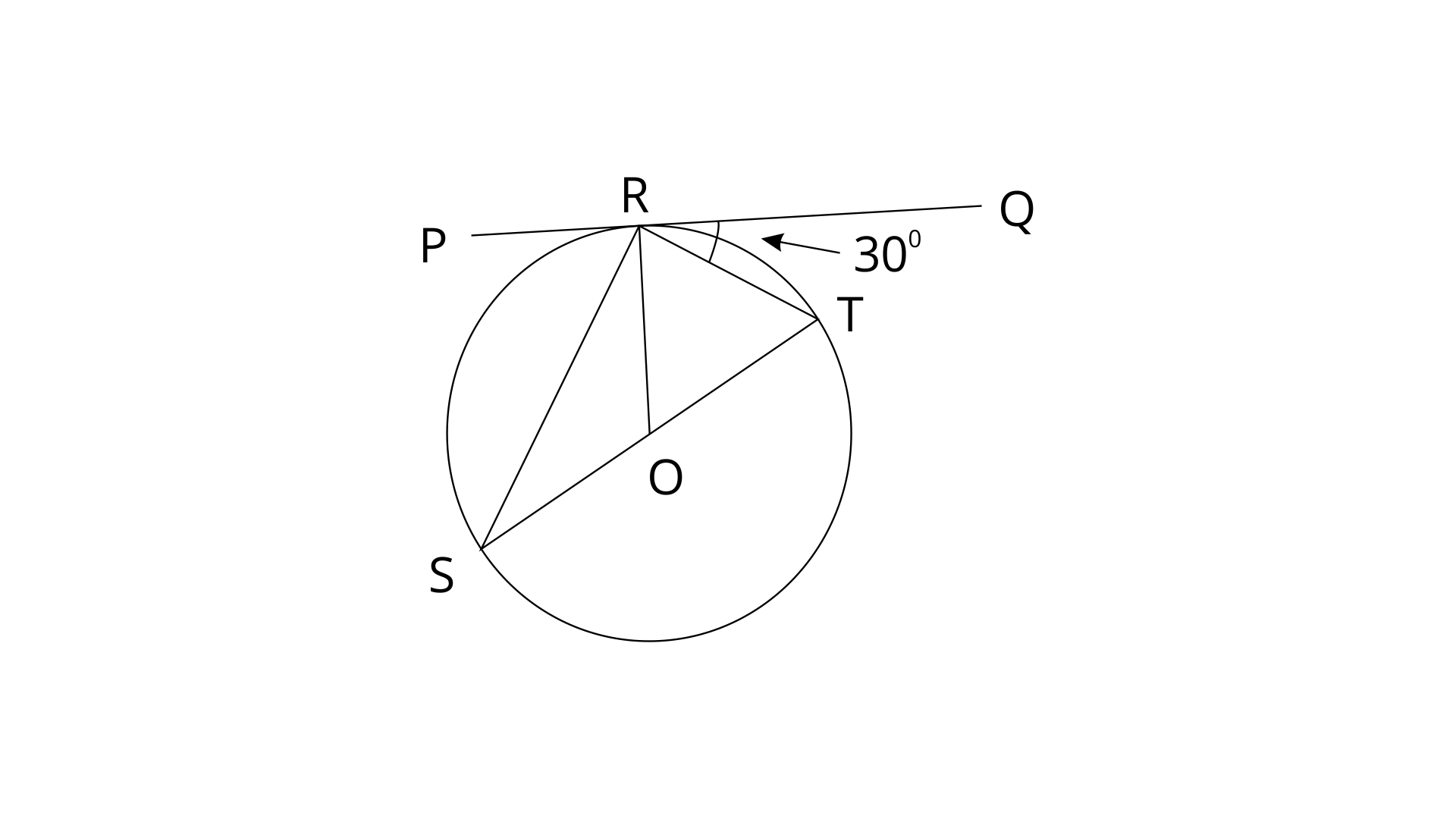
(i). $\angle PRS$

Ans: In a circle, Angle at the circumference subtended by the diameter is the right angle. Therefore, $\angle SRT={{90}^{\circ }}$

PQ is a straight line.

$\angle PRS+\angle SRT+\angle TRQ={{180}^{\circ }}$

$\Rightarrow \angle PRS={{180}^{\circ }}-{{30}^{\circ }}-{{90}^{\circ }}={{60}^{\circ }}$

Thus the value of $\angle PRS$is ${{60}^{\circ }}$.

(ii). $\angle ROT$

Ans: PQ is tangent and OR is radius. Radius is perpendicular to the tangent.

Therefore, $\angle ORQ={{90}^{\circ }}$

$\Rightarrow \angle ORQ=\angle ORT+\angle TRQ={{90}^{\circ }}$

$\Rightarrow \angle ORT={{90}^{\circ }}-{{30}^{\circ }}={{60}^{\circ }}$

OR=OT(Radii of same circle)

$\angle ORT=\angle OTR={{60}^{\circ }}$(opposite angles of equal sides)

Now, By using angle sum property in $\Delta ORT$

$\angle ORT+\angle OTR+\angle ROT={{180}^{\circ }}$

$\Rightarrow \angle ROT={{180}^{\circ }}-{{60}^{\circ }}-{{60}^{\circ }}={{60}^{\circ }}$

Thus the value of $\angle ROT$is ${{60}^{\circ }}$.

5. AB is the diameter and AC is a chord of a circle with centre O such that angle $BAC={{30}^{\circ }}$. The tangent to the circle at c intersects AB produced in D. Show that : BC=BD.

Ans: 

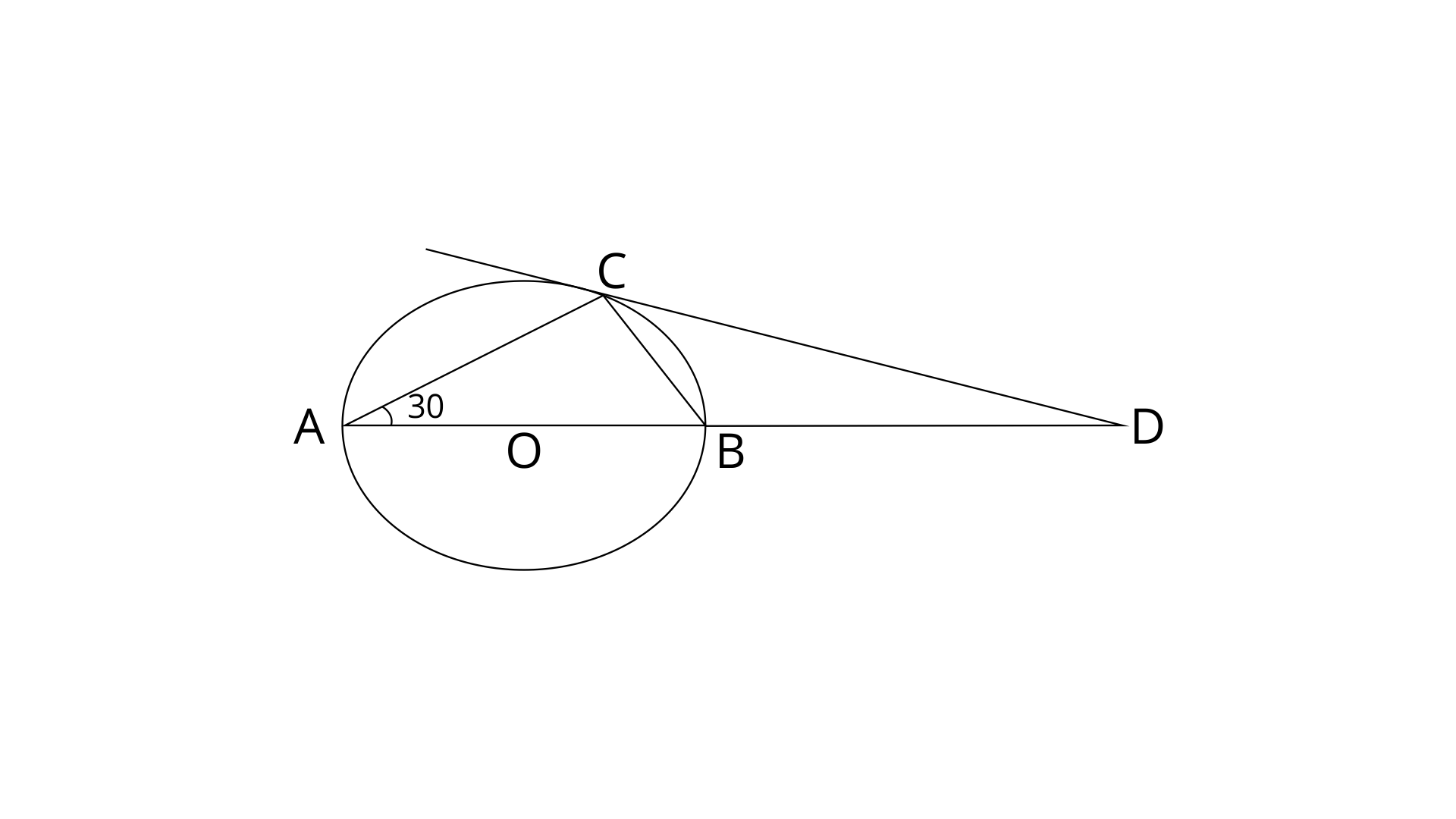
OA=OC (Radii of same circle)

$\angle OAC=\angle OCA={{30}^{\circ }}$(opposite angles of equal sides)

In $\Delta OAC$by using angle sum property,

$\angle OAC+\angle OCA+\angle AOC={{180}^{\circ }}$

$\angle AOC={{180}^{\circ }}-{{30}^{\circ }}-{{30}^{\circ }}={{120}^{\circ }}$

AB is diameter and a straight line.

$\angle AOC+\angle BOC={{180}^{\circ }}$(linear pair)

$\Rightarrow \angle BOC={{180}^{\circ }}-{{120}^{\circ }}={{60}^{\circ }}$

$\angle BOC=\angle DOC={{60}^{\circ }}$(Angles b/w same line segments)

In a circle, Angle at the circumference subtended by the diameter is the right angle.

Therefore, $\angle ACB={{90}^{\circ }}$

$\angle OCA+\angle OCB={{90}^{\circ }}$

$\Rightarrow \angle OCB={{90}^{\circ }}-{{30}^{\circ }}={{60}^{\circ }}$

$OC\bot CD~$(Radius is perpendicular to tangent)

$\angle OCD={{90}^{\circ }}$

$\angle OCB+\angle BCD={{90}^{\circ }}$

$\angle BCD={{90}^{\circ }}-{{60}^{\circ }}={{30}^{\circ }}$---------(1)

By using angle sum property in $\Delta OCD$

$\angle OCD+\angle ODC+\angle COD={{180}^{\circ }}$

$\Rightarrow \angle ODC={{180}^{\circ }}-{{90}^{\circ }}-{{60}^{\circ }}={{30}^{\circ }}$

$\angle BDC=\angle ODC={{30}^{\circ }}$(Angles b/w same line segments)------(2)

From eq. (1) and (2)

$\angle BDC=\angle BCD={{30}^{\circ }}$

$\Rightarrow BC=BD$(opposite sides of equal angles)

Hence proved.

6. Tangent at P to the circumcircle of triangle PQR is drawn. If this tangent is parallel to side QR show that $\Delta PQR$is isosceles.

Ans: 

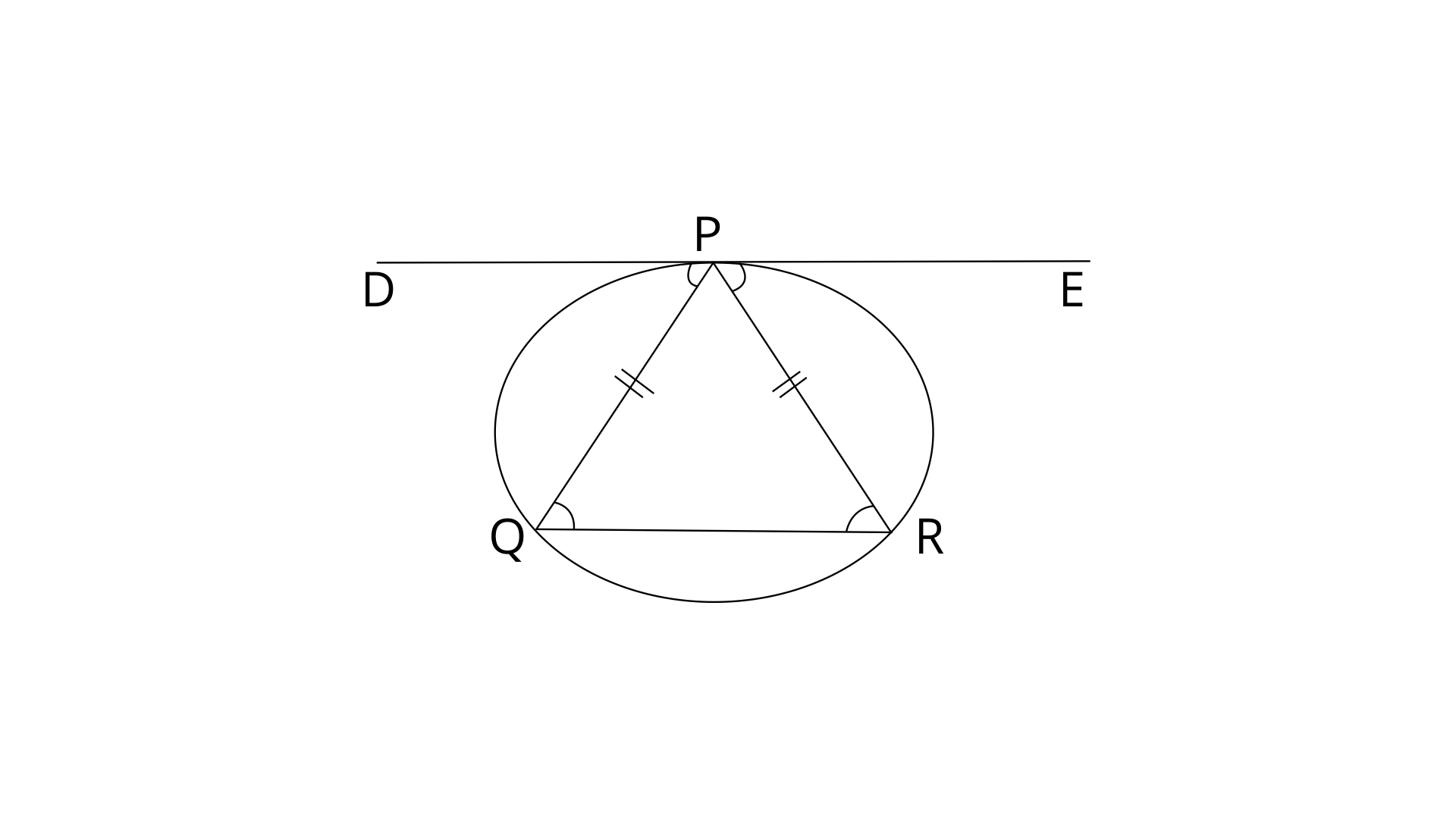
DE is tangent to the circle at point P.

DE||QR

Therefore, 

$\angle DPQ=\angle PQR$(Alternate angles)----------(1)

$\angle EPR=\angle PRQ$(Alternate angles) ---------(2)

Since DP is a tangent and PQ is chord such as PQ=PR

Therefore, $\angle DPQ=\angle PRQ$ -----------(3)

From eq.(1),(2) and (3)

$\angle DPQ=\angle PRQ=\angle EPR=\angle PQR$

$\Rightarrow \angle PRQ=\angle PQR$

PQ=PR (opposite sides of equal angles)

Therefore $\Delta PQR$is an isosceles triangle.

7. Two circles with centres O and O’ are drawn to intersect each other at points A and B. centres O of the circle lies on the circumference of the other circle and CD is drawn tangent to the circle with centre O’ at A. Prove that OA bisects angle BAC.

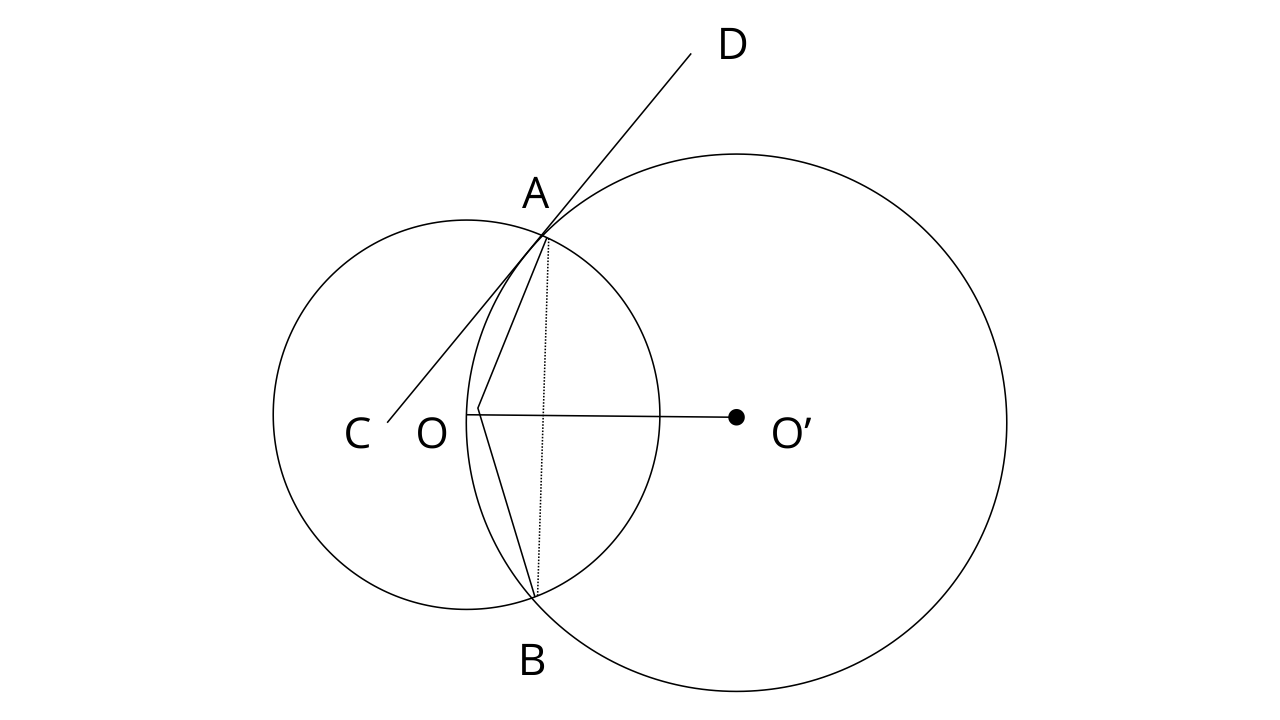
Ans: CD is a tangent to the circle and AO is chord.

Therefore, $\angle OAC=\angle OBA$(Angles in alternate segments) ----------(1)

Since, OA=OB (Radii of same circle)

Therefore, $\angle OAB=\angle OBA$(opposite angles of equal sides) ----------(2)

From eq. (1) and (2)

$\angle OAC=\angle OAB$

Therefore OA is angle bisector of $\angle BAC$.

8. Two circles touch each other internally at a point P. A chord AB of the bigger circle intersects the other circle in C and D. Prove that :$\angle CPA=\angle DPB$.

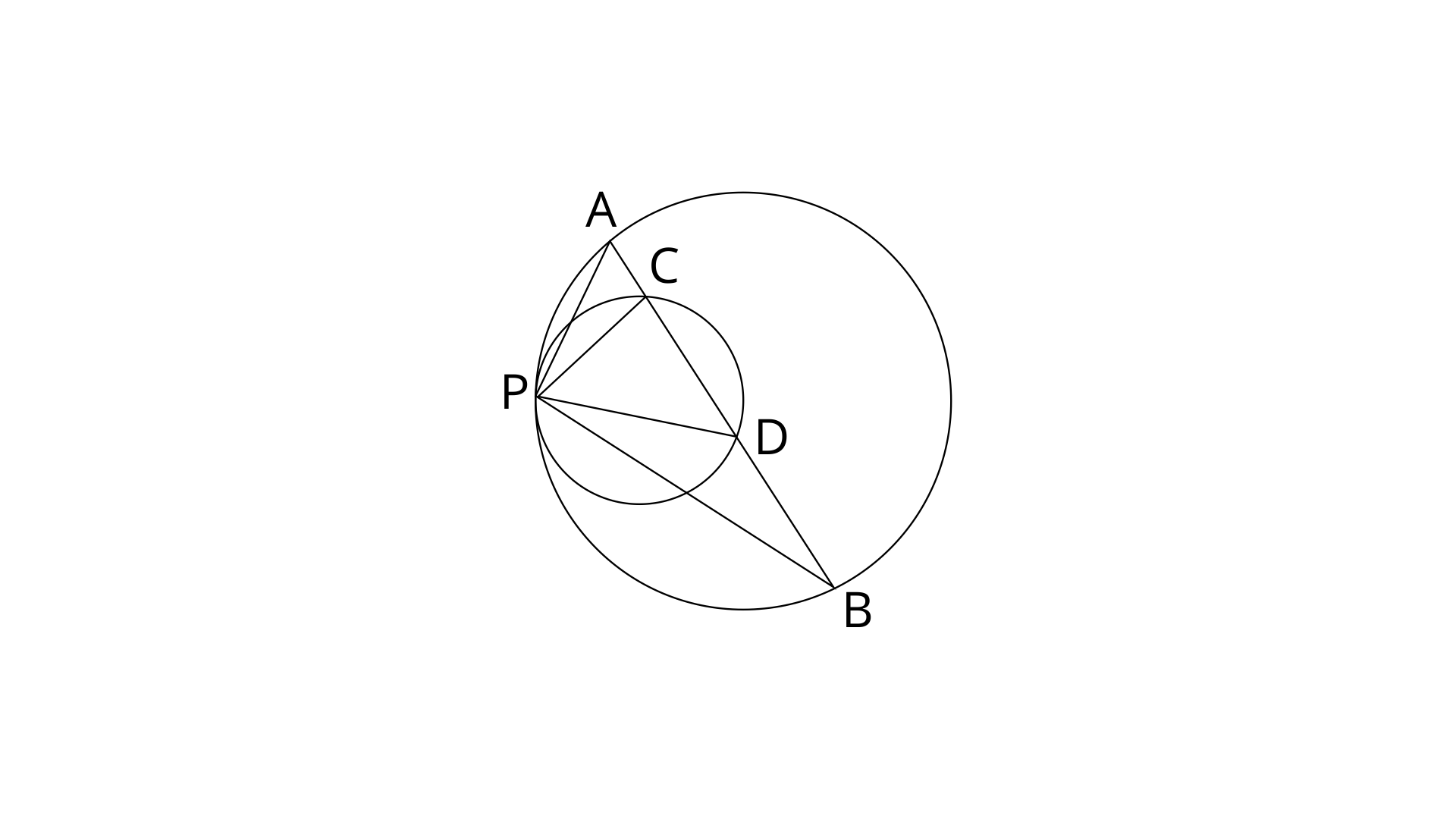
Draw tangent at point P.

Ans: We draw a tangent TS at P.

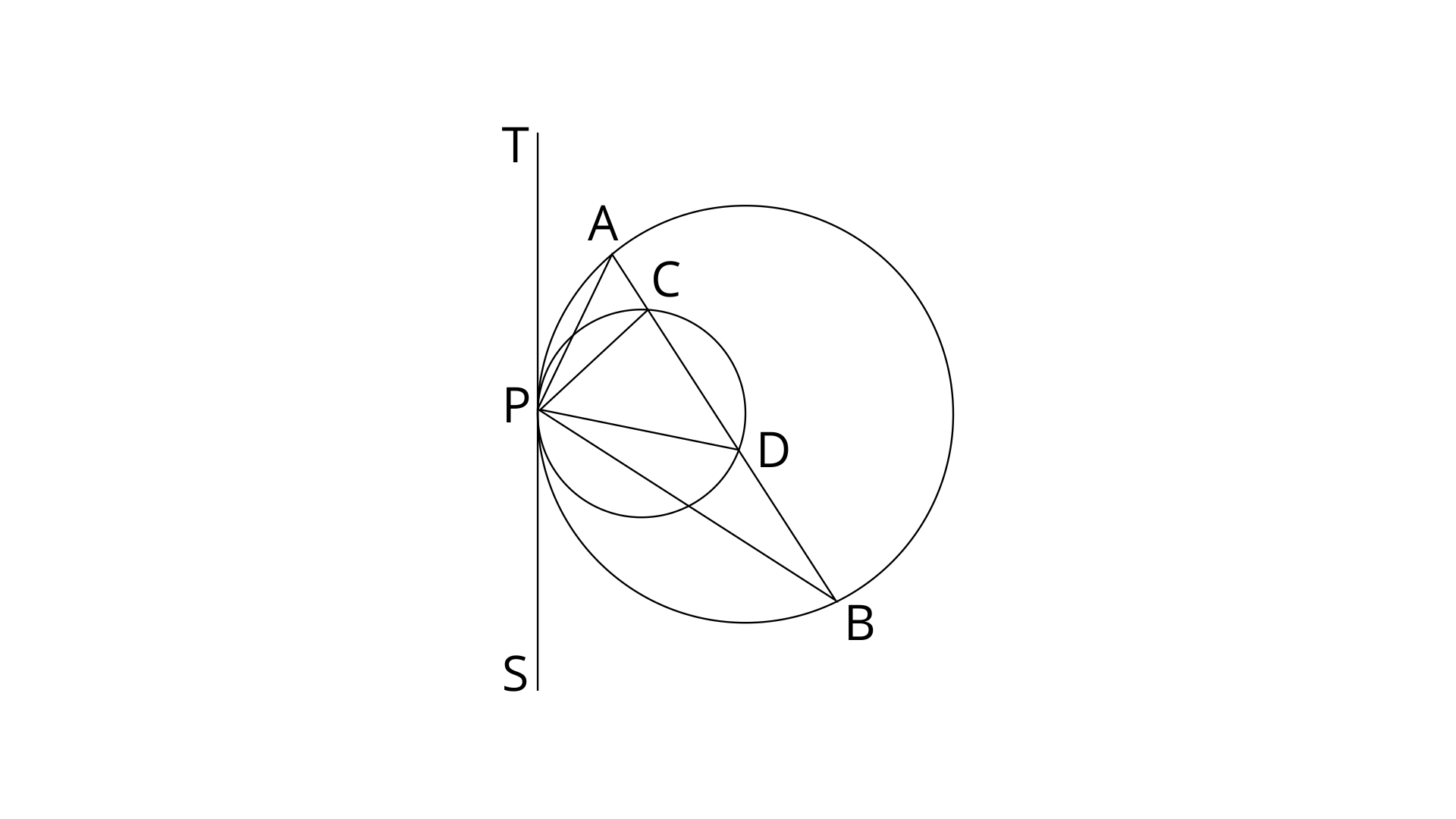
TPS is a tangent and PB is a chord.

$\angle BPS=\angle PAB$(Angles in alternate segments) -------(1)

Similarly, $\angle PCD=\angle DPS$------(2)

Subtract eq. (1) from eq. (2)

$\angle PCD-\angle BPS=\angle DPS-\angle PAB$

$\angle PCD-\angle PAB=\angle DPS-\angle BPS$-----------(3)

By using exterior angle property in $\Delta PAC$

$\angle PCD=\angle PAB+\angle CPA$  --------------(4)

Now on substituting the value from eq.(4) in eq.(3)

$\angle PAB+\angle CPA-\angle PAB=\angle DPS-\angle BPS$

$\angle CPA=\angle DPB$ [∵From figure $\angle DPS-\angle BPS=\angle DPB$]

(Hence proved)

9. In a cyclic quadrilateral ABCD, the diagonal AC bisects the angle BCD. Prove that the diagonal BD is parallel to the tangent to the circle at point A.

Ans: 

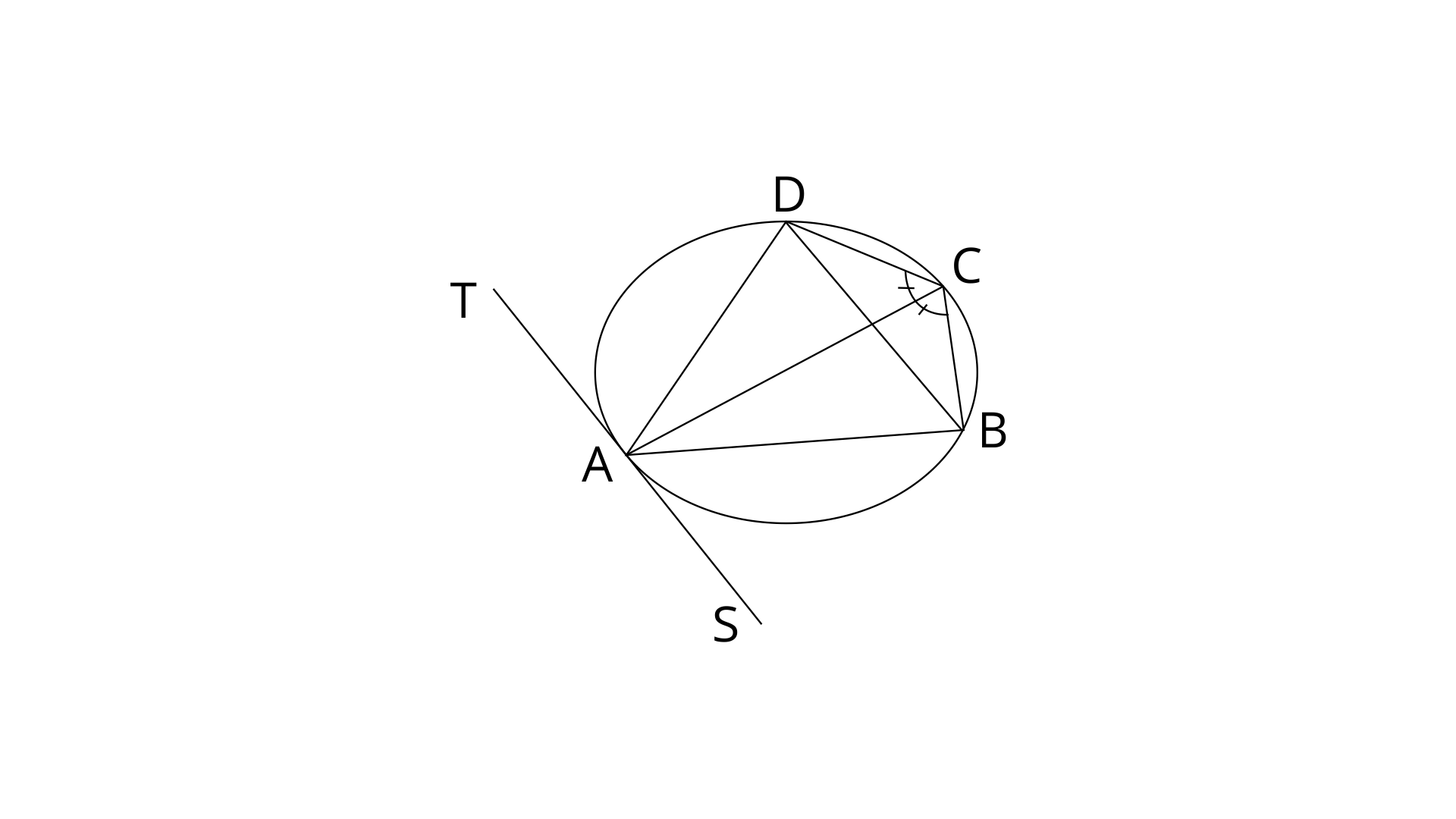
In a circle, Angles at the circumference subtended by the same chord are equal.

$\angle ADB=\angle ACB$ --------(1)

Similarly, 

$\angle ABD=\angle ACD$--------(2)

AC is angle bisector of $\angle BCD$,

$\angle ACB=\angle ACD$------(3)

From eq. (1), (2) and (3)

$\angle ADB=\angle ABD$

TAS is a tangent and AB is a chord.

Therefore, $\angle BAS=\angle ADB$

 Since, $\angle ADB=\angle ABD$

Therefore, $\angle BAS=\angle ABD$

If two alternate angles are equal then the sides are equal.

$\because \angle BAS=\angle ABD$

Therefore TAS is parallel to BD.

10. In the figure, ABCD is a cyclic quadrilateral with BC=CD. TC is tangent to the circle at point C and DC is produced to point G. If $\angle BCG={{108}^{\circ }}$and O is the centre of the circle, find :

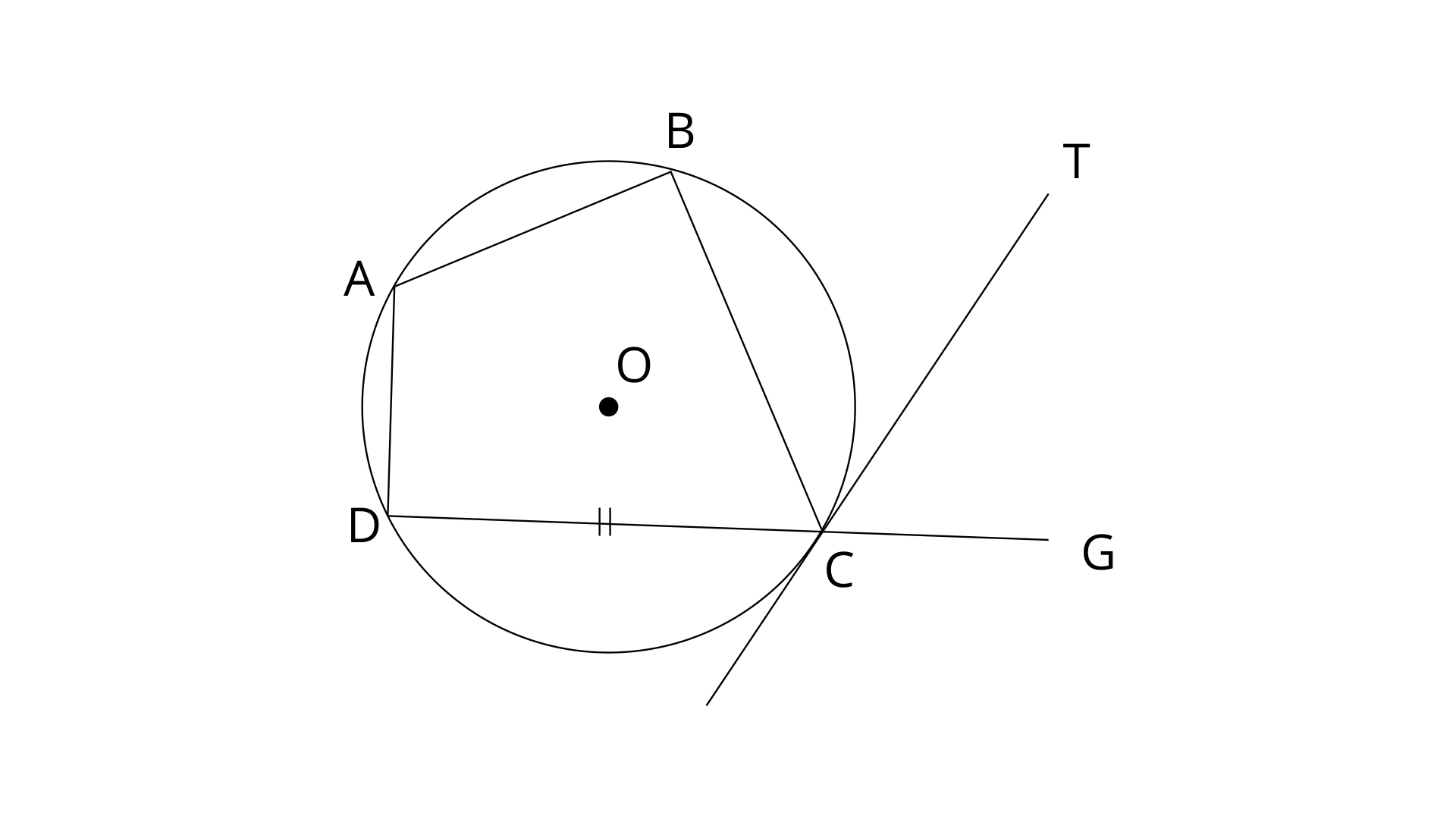
(i). Angle BCT

(ii). Angle DOC

Ans: Join AC and OD.

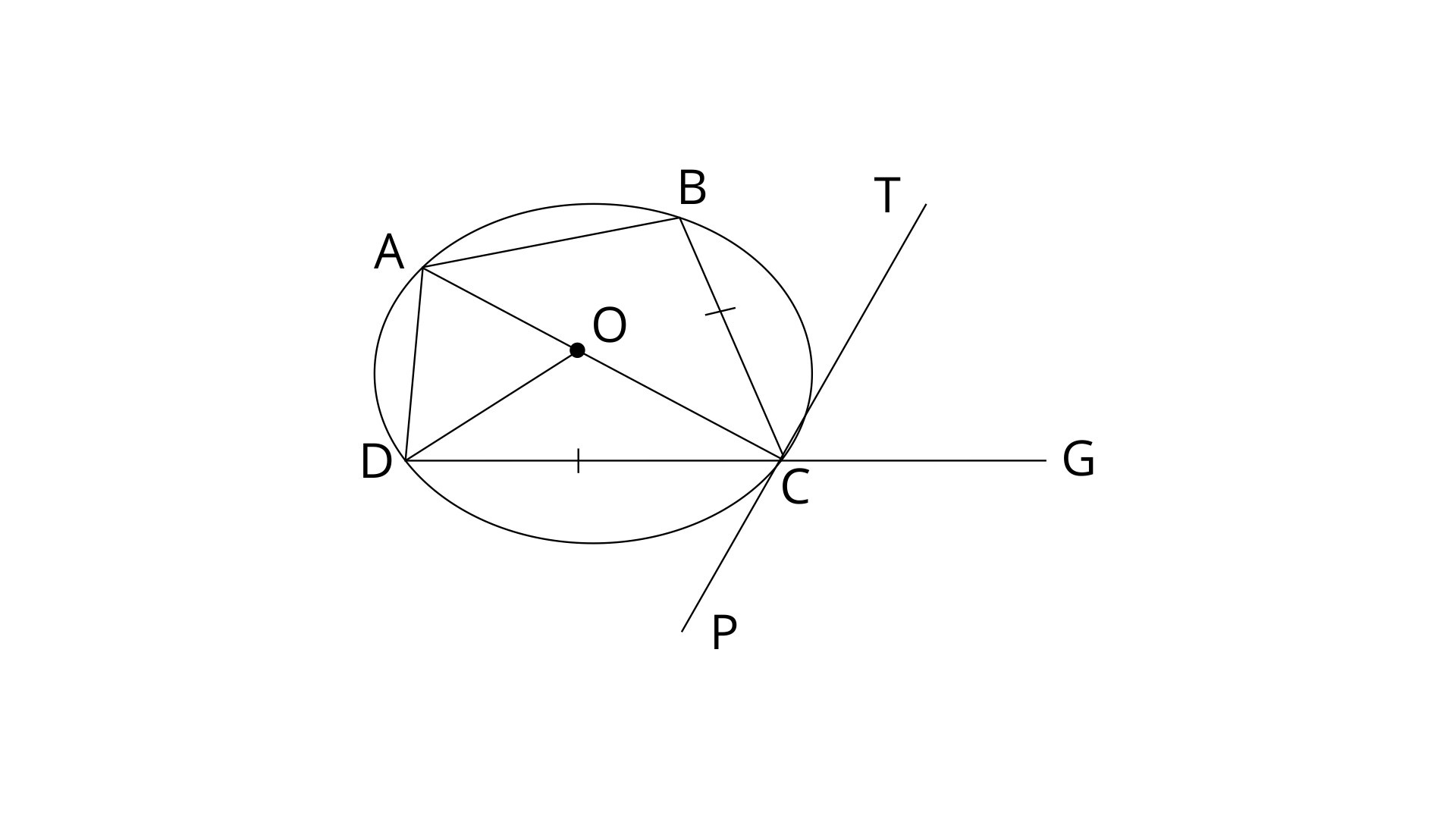
DCG is a straight line.

$\angle BCG+\angle BCD={{180}^{\circ }}$

$\Rightarrow \angle BCD={{180}^{\circ }}-{{108}^{\circ }}={{72}^{\circ }}$

Here BC=CD

Angles opposite to equal sides are equal.

$\angle DCP=\angle BCT$

PCT is a straight line. 

$\angle DCP+\angle BCT+\angle BCD={{180}^{\circ }}$

$\Rightarrow 2\angle BCT={{180}^{\circ }}-{{72}^{\circ }}={{108}^{\circ }}$

$\Rightarrow \angle BCT={{54}^{\circ }}$

Thus the value of $\angle BCT$is ${{54}^{\circ }}$.

(ii). Angle DOC

Ans: Since $\angle DCP=\angle BCT={{54}^{\circ }}$

PT is tangent and DC is chord.

$\angle DAC=\angle DCP={{54}^{\circ }}$ (Angles is alternate segments)

In a circle, Angle at the centre is doubled the angle at the circumference subtended by the same chord. Here $\angle DAC$and $\angle DOC$are subtended by chord DC.

$\angle DOC=2\times \angle DAC$

$\Rightarrow \angle DOC=2\times {{54}^{\circ }}={{108}^{\circ }}$

Thus the value of $\angle DOC$is ${{108}^{\circ }}$.

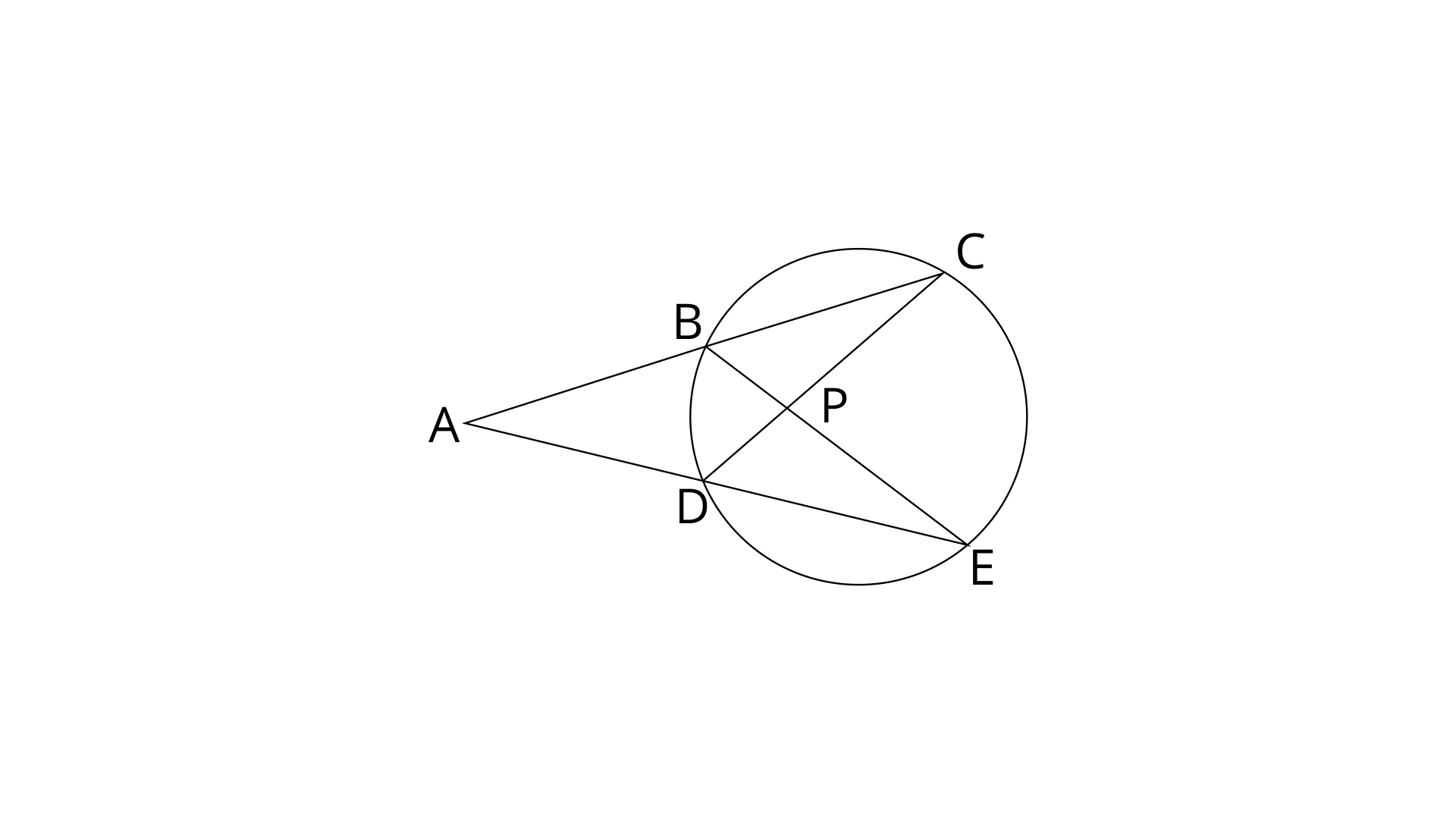
11. Two circles intersect each other at points A and B. A straight line PAQ cuts the circles at P and Q. If the tangents at P and Q intersect at point T, show that the points P, B, Q and T are concyclic.

Ans: Join AB, PB and BQ.

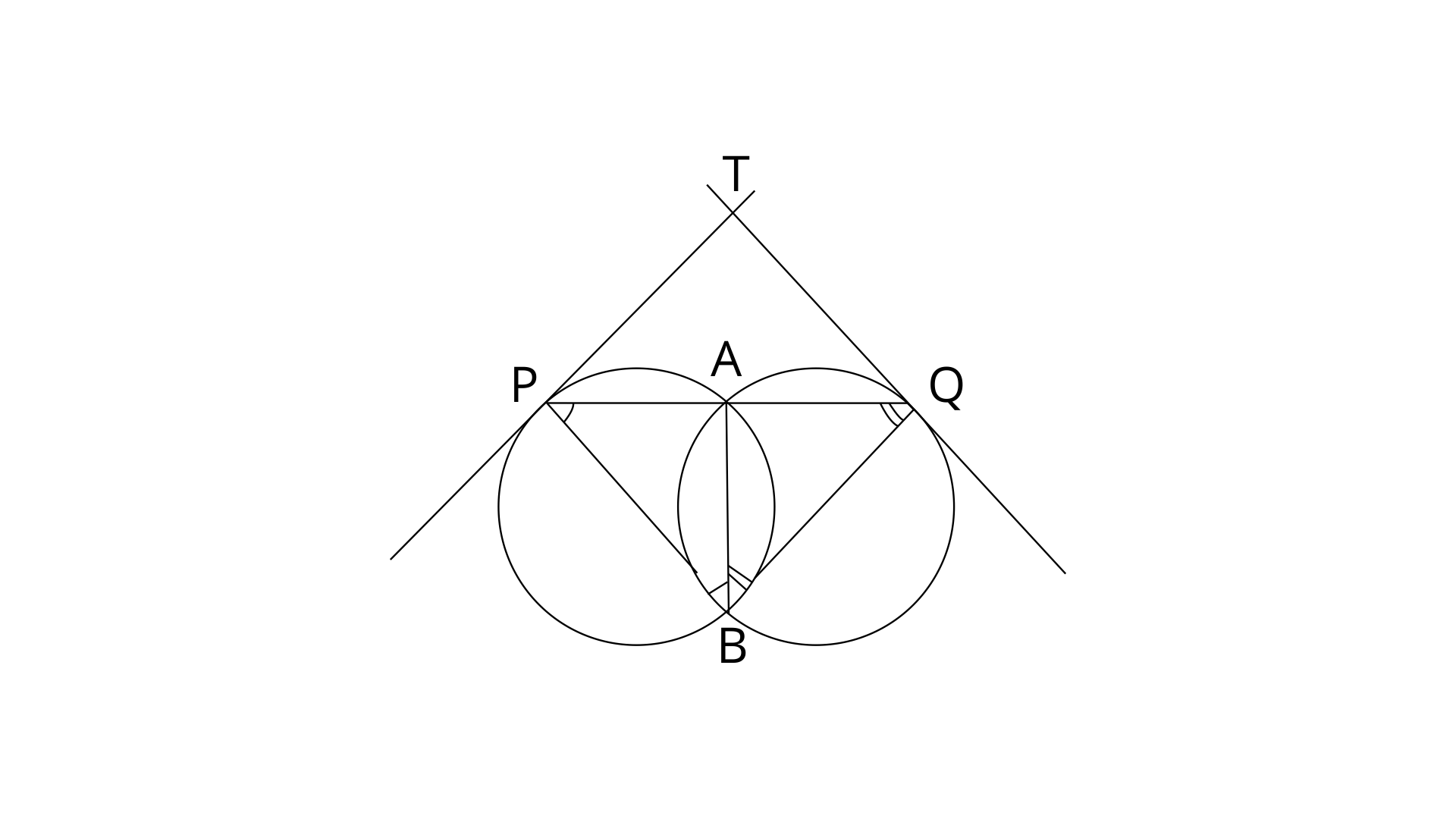
TP is the tangent and AB is a chord.

$\angle TPA=\angle ABP$(Angles in alternate segments) ----------(1)

Similarly, $\angle TQA=\angle ABQ$(Angles in alternate segments) --------(2)

On adding equations (1) and (2)

$\angle TPA+\angle TQA=\angle ABP+\angle ABQ$

By using angle sum property in $\Delta PTQ$

$\angle TPA+\angle TQA+\angle PTQ={{180}^{\circ }}$

$\Rightarrow \angle TPA+\angle TQA={{180}^{\circ }}-\angle PTQ$ 

From eq.(1) and (2) by putting $\angle TPA=\angle ABP$and $\angle TQA=\angle ABQ$

$\angle ABP+\angle ABQ={{180}^{\circ }}-\angle PTQ$ 

$\Rightarrow \angle PBQ={{180}^{\circ }}-\angle PTQ$

$\Rightarrow \angle PBQ+\angle PTQ={{180}^{\circ }}$

Since $\angle PBQ$ and $\angle PTQ$are opposite angles of quadrilateral PBQT. If the sum of opposite angles in a quadrilateral is ${{180}^{\circ }}$then the quadrilateral is cyclic quadrilateral. Therefore P, B, Q and T are cyclic.

12. In the figure; PA is tangent to the circle, PBC is secant and AD bisects angle BAC. Show that triangle PAD is an isosceles triangle. Also, show that :

$\angle CAD=\frac{1}{2}\left[ \angle PBA-\angle PAB \right]$

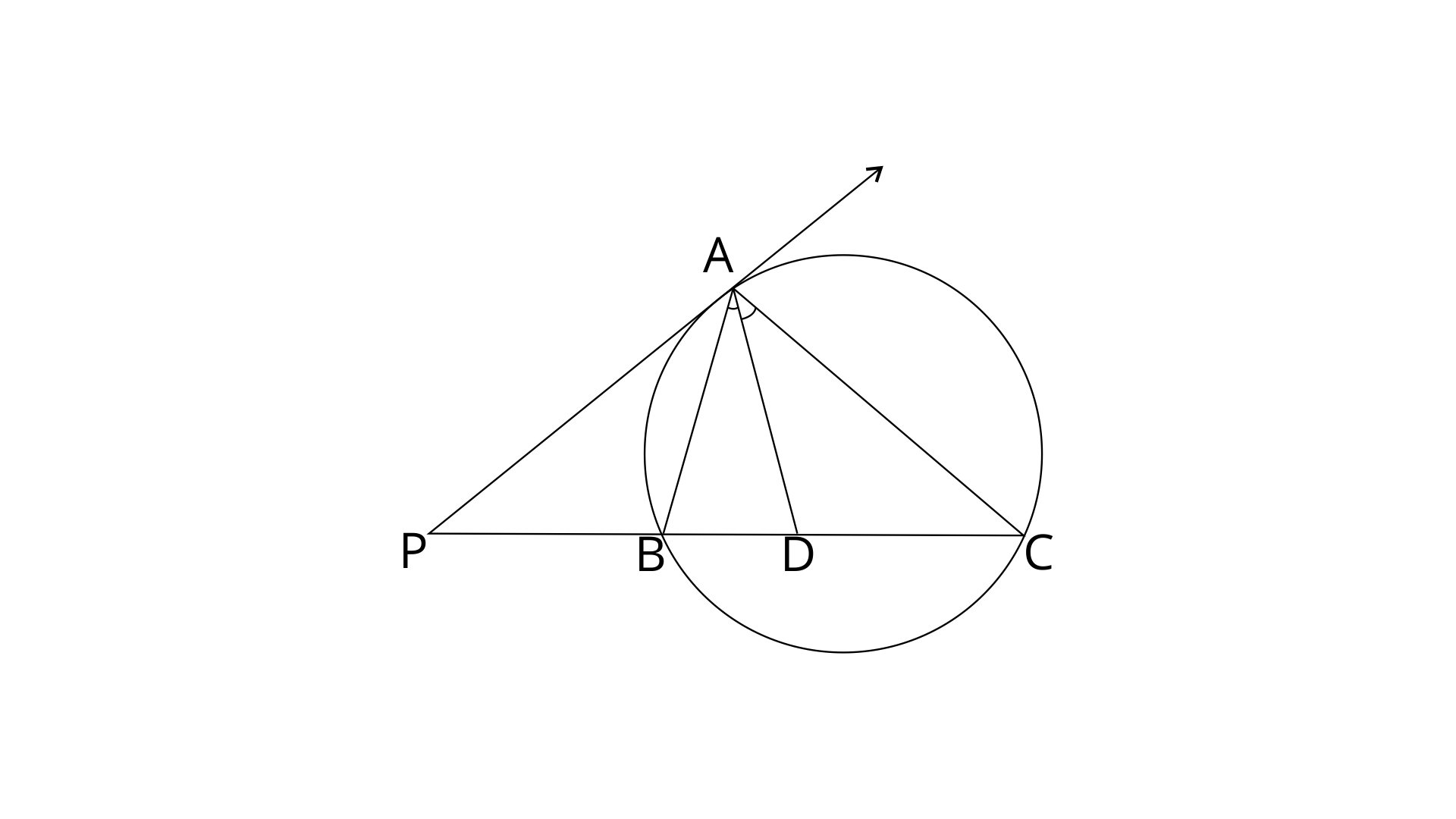
Ans: 

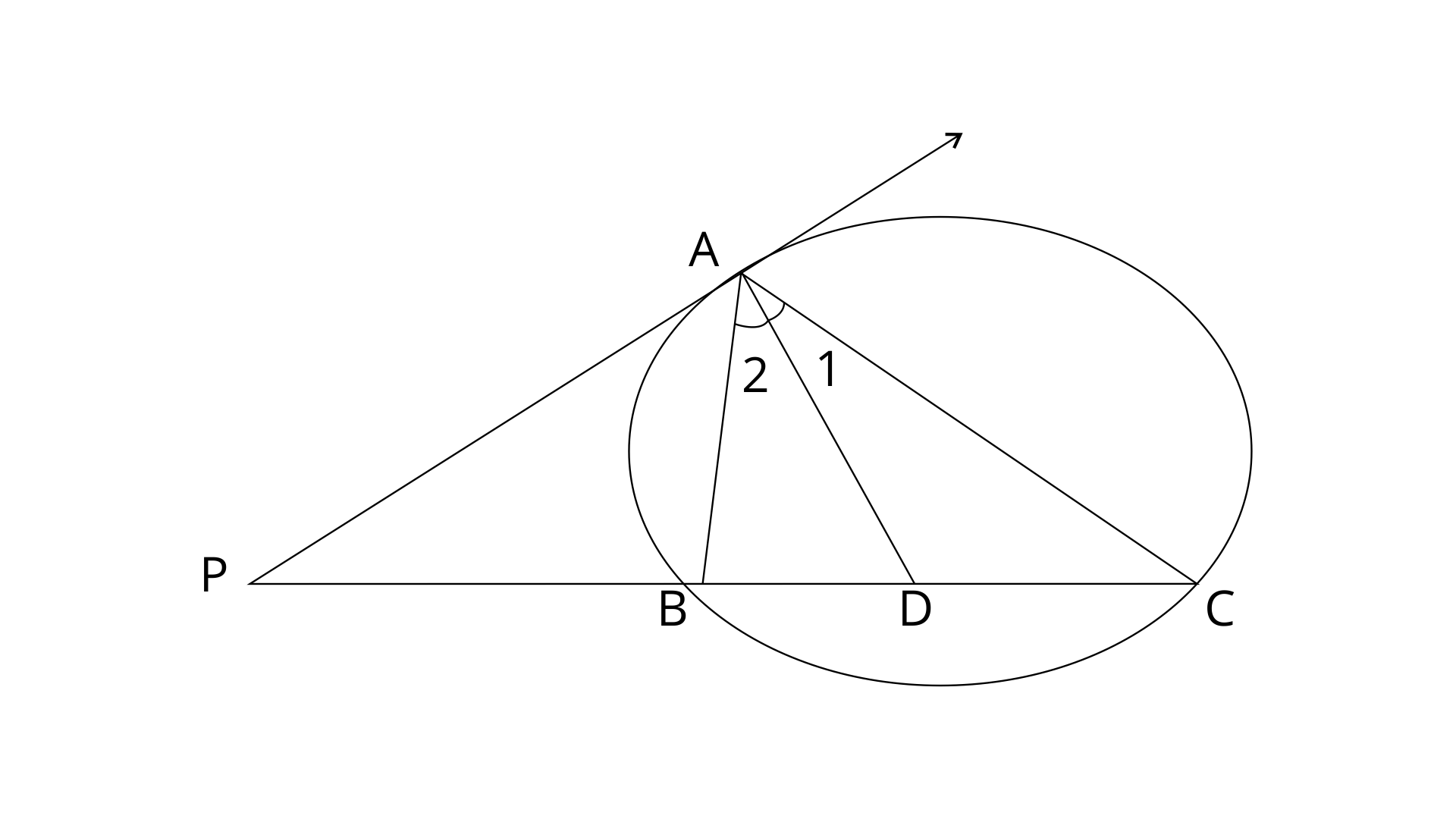
PA is tangent to the circle and AB is chord.

$\angle PAB=\angle C$(Angles in alternate segments) ------------(1)

AD is bisector of angle BAC.

Therefore, $\angle 1=\angle 2$  --------(2)

In $\Delta ADC$by using angle sum property,

$\angle ADP=\angle 1+\angle C$

$\Rightarrow \angle ADP=\angle 2+\angle PAB$

$\Rightarrow \angle ADP=\angle PAD$ 

In $\Delta ABC$, By using exterior angle property.

$\angle PBA=\angle 1+\angle 2+\angle C$

$\Rightarrow \angle 1+\angle 2=\angle PBA-\angle C$

$\Rightarrow \angle 1+\angle 2=\angle PBA-\angle PAB$[∵ From eq. 1 $\angle PAB=\angle C$]

$\Rightarrow 2\angle 1=\left[ \angle PBA-\angle PAB \right]$

$\Rightarrow \angle CAD=\frac{1}{2}\times \left[ \angle PBA-\angle PAB \right]$  [From fig. $\angle 1=\angle CAD$]

13. Two circles intersect each other at points A and B. Their common tangent touches the circles at points P and Q as shown in the figure. Show that the angles PAQ and PBQ are supplementary.

Ans: Join AB.

PQ is a tangent and AB is a chord.

$\angle QPA=\angle PBA$(Angles in Alternate segments)  ----------------(1)

$\angle PQA=\angle QBA$ (Angles in Alternate segments)  ----------------(2)

On adding eq.(1) and (2)

$\angle PQA+\angle QPA=\angle QBA+\angle PBA$

In $\Delta QPA$by using angle sum property,

$\angle PQA+\angle QPA+\angle PAQ={{180}^{\circ }}$

$\angle QPA+\angle PQA={{180}^{\circ }}-\angle PAQ$------(3)

$\angle PBA+\angle QBA=\angle PBQ$

$\angle PBQ=\angle QPA+\angle PQA$ [$\angle QPA=\angle PBA$and $\angle 

PQA=\angle QBA$]-----(4)

From eq. (3) and (4)

$\angle PBQ={{180}^{\circ }}-\angle PAQ$

$\Rightarrow \angle PBQ+\angle PAQ={{180}^{\circ }}$

Therefore, $\angle PBQ$and $\angle PAQ$ are supplementary.

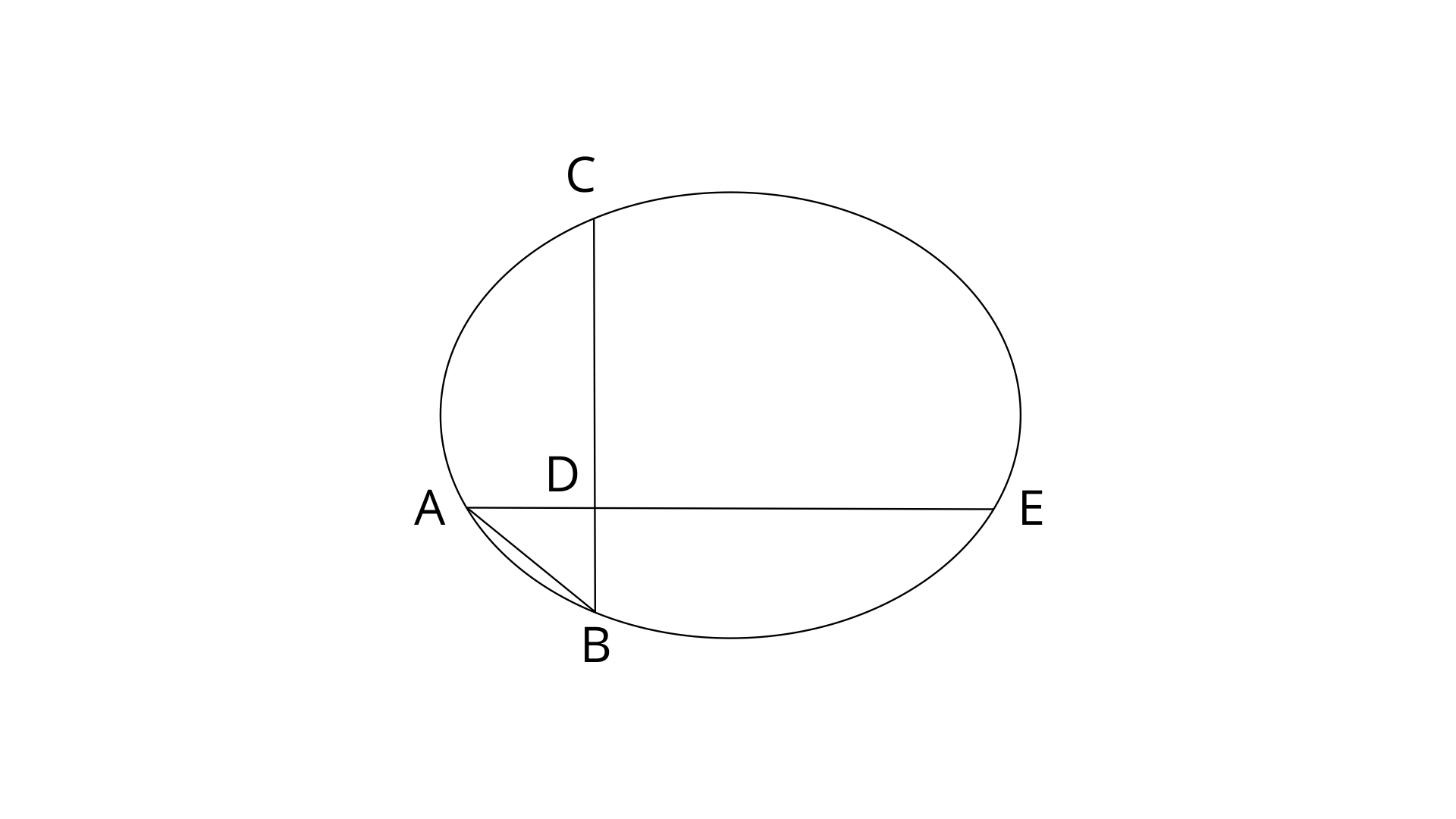
14. In the figure, chords AE and BC intersect at each other at point D. 

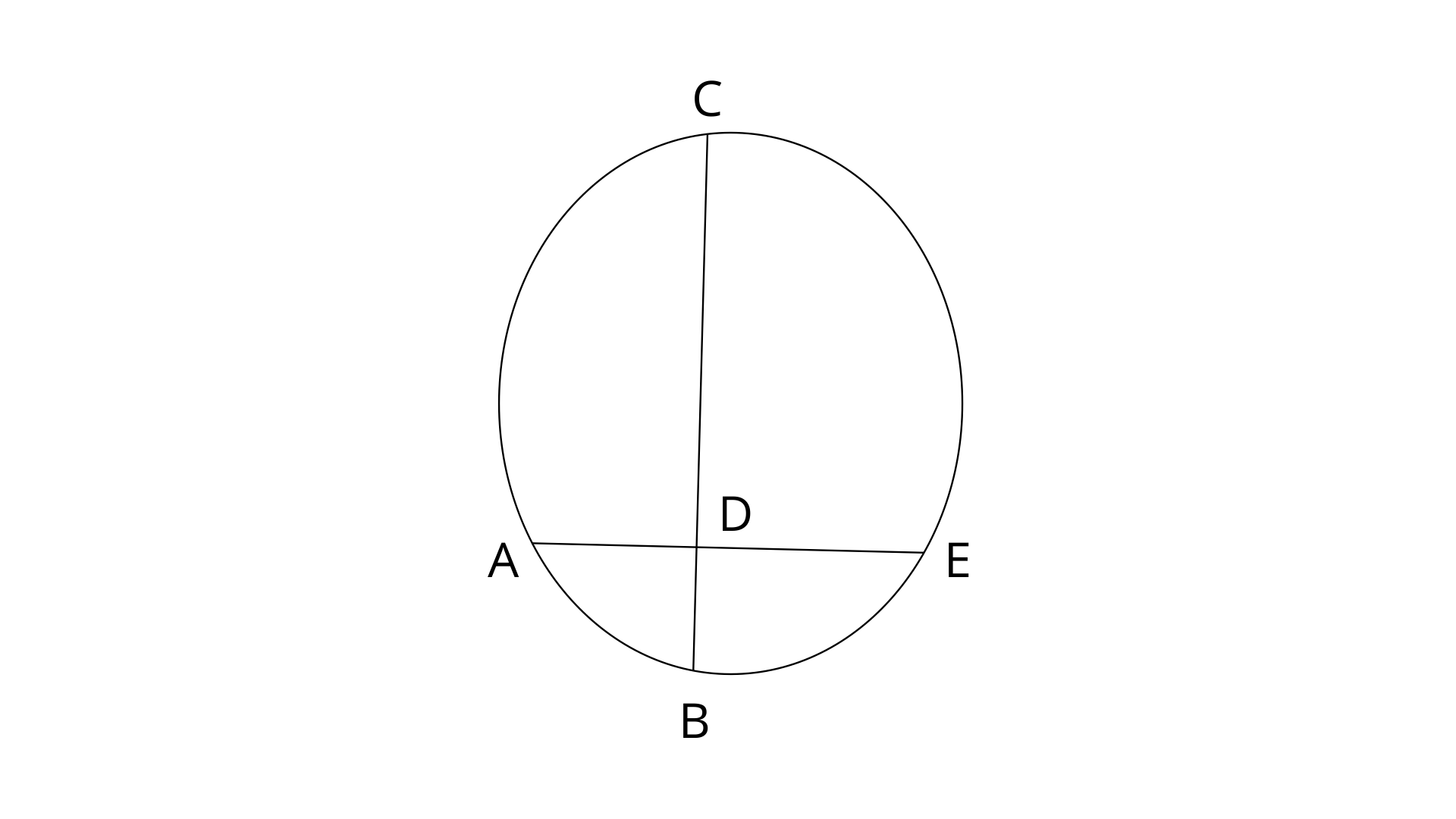
(i). If $\angle CDE={{90}^{\circ }}$, AB=5cm, BD=4cm and CD=9cm: Find DE.

(ii). If AD=BD, show that : AE=BC.

Ans: 

Join points AB.

(i). If $\angle CDE={{90}^{\circ }}$, AB=5cm, BD=4cm and CD=9cm: Find DE.

Ans: Since, $\angle CDE={{90}^{\circ }}\Rightarrow \angle ADB={{90}^{\circ }}$. Therefore $\Delta ADB$is a right angled triangle. By using pythagoras theorem.

$A{{B}^{2}}=A{{D}^{2}}+B{{D}^{2}}$

$\Rightarrow {{5}^{2}}=A{{D}^{2}}+{{4}^{2}}$

$\Rightarrow A{{D}^{2}}=25-16=9$

$\Rightarrow AD=3cm$

Chords AE and BC intersect each other at point D.

$AD\times DE=BD\times CD$

$\Rightarrow 3\times DE=4\times 9$

$\Rightarrow DE=12cm$

Thus the value of DE is 12cm.

(ii). If AD=BD, show that : AE=BC.

Ans: Since, AD=BD-------(1)

$AD\times DE=AD\times CD$

$\Rightarrow DE=CD$-------(2)

On adding eq.(1) and (2)

AD+DE=BD+CD

$\Rightarrow AE=BC$  (Hence proved)

15. Circles with centres P and Q intersect at points A and B are shown in the figure. CBD is a line segment and EBM is tangent to the circle, with centre Q, at point B. If the circles are congruent; show that : CE=BD.

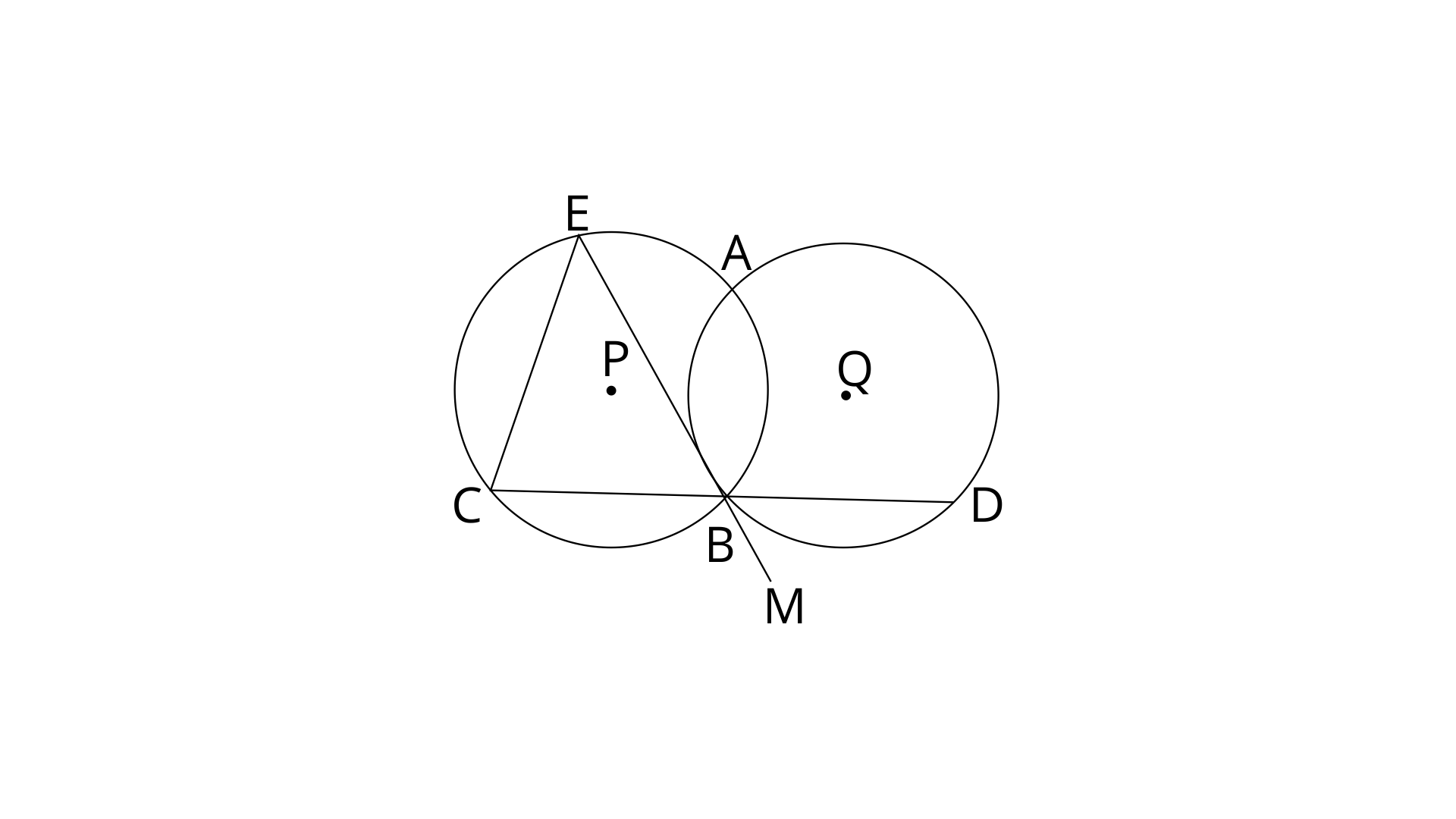
Ans: Join AB and AD.

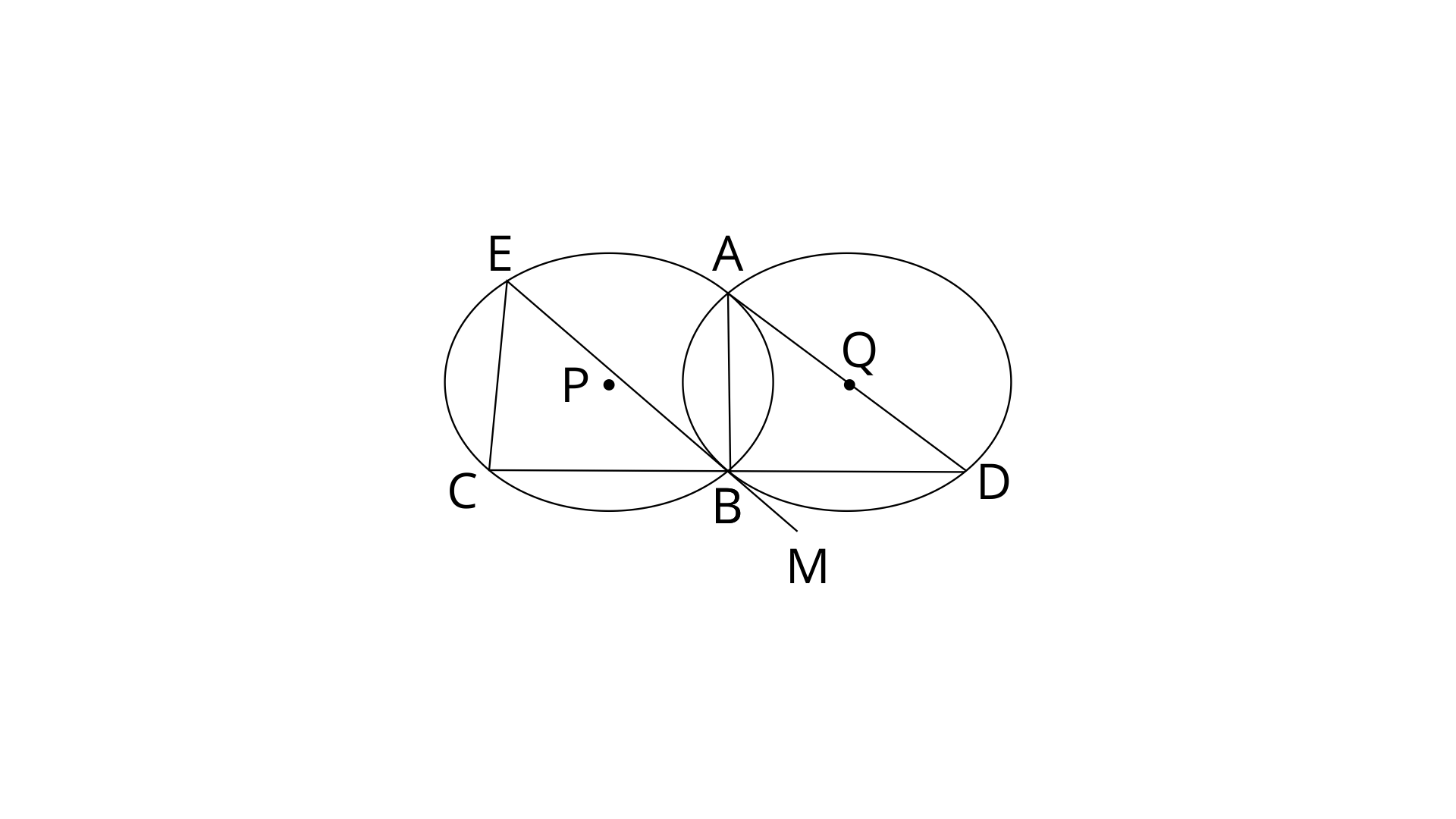
Given that circles are congruent. Therefore the radii of circles are equal.

EBM is tangent to the circle having centre O and BD is chord.

$\angle DBM=\angle BAD$(Angles in alternate segments)--------(1)

$\angle DBM=\angle CBE$(opposite angles) -------(2)

From eq. (1) and (2)

$\angle CBE=\angle BAD$

CE=BD (opposite sides of equal angles are equal)

Therefore, CE=BD (Hence proved)

16. In the adjoining figure, O is the centre of the circle and AB is a tangent to it at point B. $\angle BDC={{65}^{\circ }}.$Find $\angle BAO$.

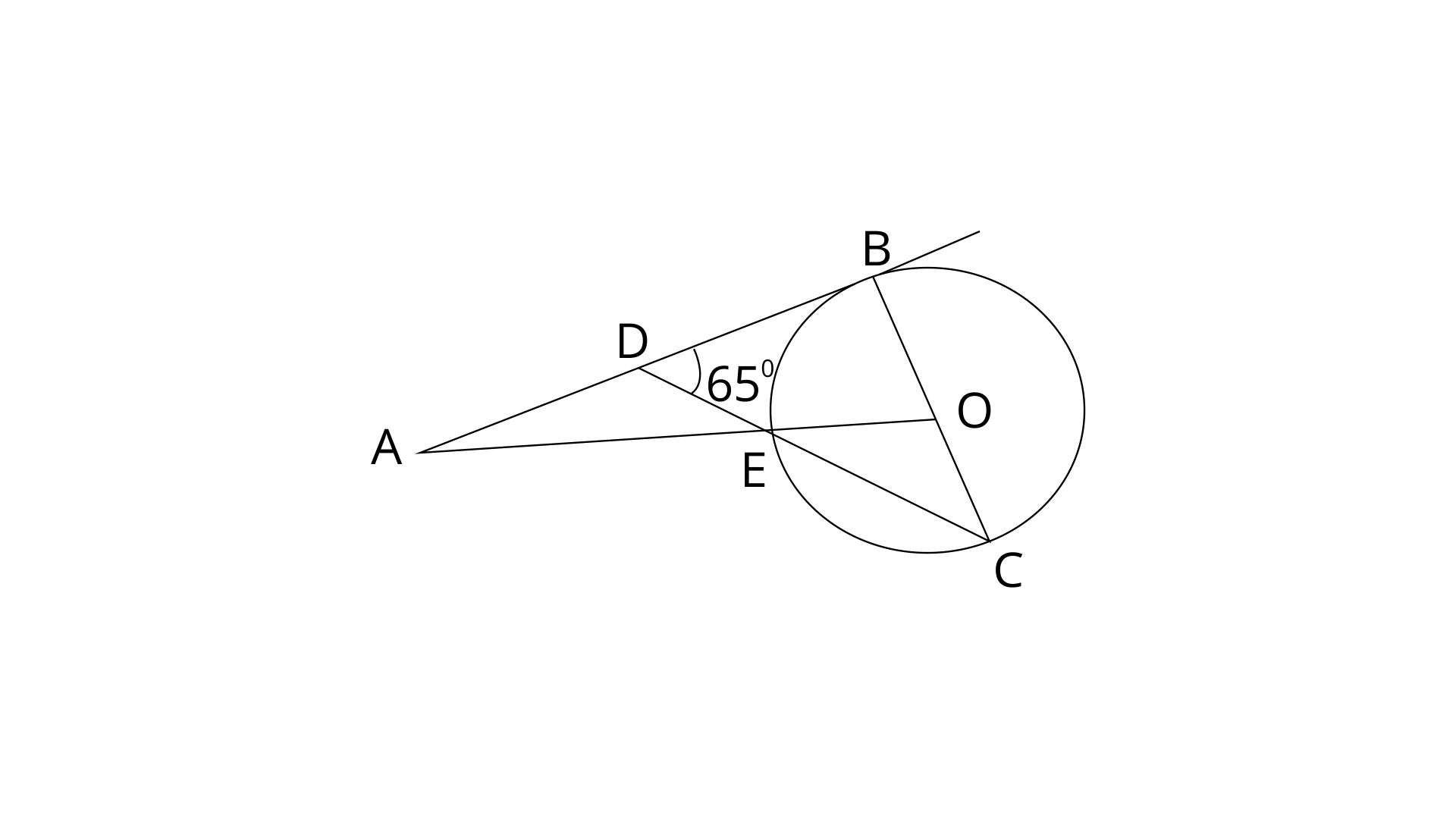
Ans: AB is a straight line and tangent to the circle.

$\angle BDE+\angle ADE={{180}^{\circ }}$(Linear pair)

$\Rightarrow \angle ADE={{180}^{\circ }}-{{65}^{\circ }}={{115}^{\circ }}$

DB is tangent and OB is radius. Radius is perpendicular to the tangent.

$\angle DBC={{90}^{\circ }}$

By using angle sum property in $\Delta BCD$

$\angle DBC+\angle BDC+\angle BCD={{180}^{\circ }}$

$\Rightarrow \angle BCD={{180}^{\circ }}-{{90}^{\circ }}-{{65}^{\circ }}={{25}^{\circ }}$

OE=OC (radii of same circle)

$\angle OEC=\angle OCE$ (opposite angles of equal sides)’

$\angle OCE=\angle BCD={{25}^{\circ }}$ (Angles b/w same line segments)

$\angle OEC=\angle OCE={{25}^{\circ }}$

$\angle OEC=\angle DAE={{25}^{\circ }}$(Opposite angles)

By using angle sum property in $\Delta ADE$

$\angle DAE+\angle ADE+\angle AED={{180}^{\circ }}$

$\Rightarrow \angle DAE={{180}^{\circ }}-{{115}^{\circ }}-{{25}^{\circ }}={{40}^{\circ }}$

$\angle BAO=\angle DAE={{40}^{\circ }}$(Angles b/w same line segments)

Therefore, $\angle BAO={{40}^{\circ }}$.

Thus the value of $\angle BAO$is ${{40}^{\circ }}$.

EXERCISE-18(C)

1. Prove that, of any two chords of a circle, the greater chord is nearer to the centre.

Ans: 

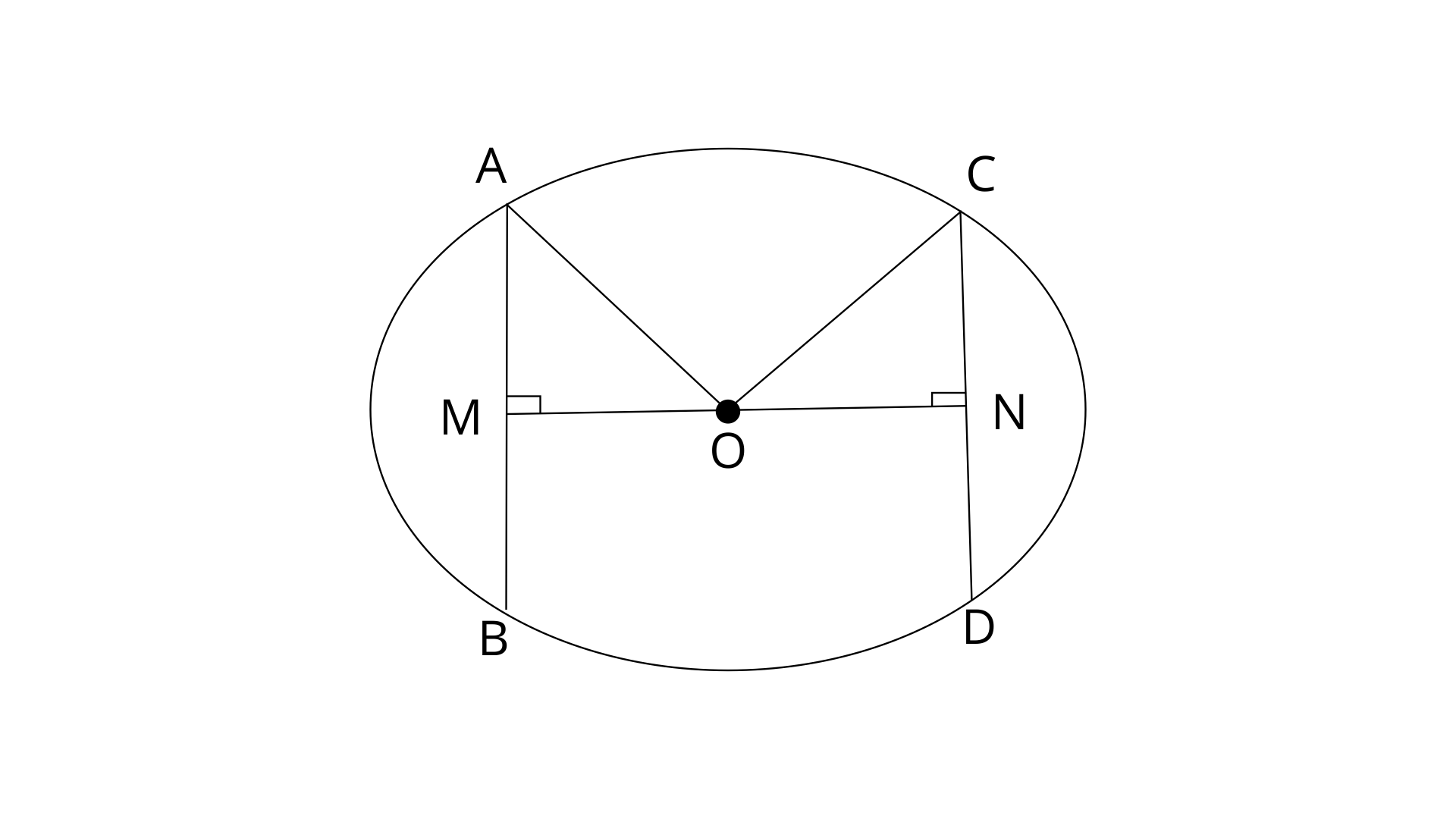
The circle has radius r and AB, CD are chords.

$OM\bot AB,~ON\bot CD$Let AB>CD

Then we have to prove OM<ON

By using pythagoras theorem in $\Delta OMA$

$O{{A}^{2}}=A{{M}^{2}}+O{{M}^{2}}$

${{r}^{2}}={{\left( \frac{1}{2}AB \right)}^{2}}+O{{M}^{2}}$-------(1)

Similarly in $\Delta ONC$

$O{{C}^{2}}=C{{N}^{2}}+O{{N}^{2}}$

$\Rightarrow {{r}^{2}}={{\left( \frac{1}{2}CD \right)}^{2}}+O{{N}^{2}}$-------(2)

From eq. (1) and (2)

${{\left( \frac{1}{2}AB \right)}^{2}}+O{{M}^{2}}={{\left( \frac{1}{2}CD \right)}^{2}}+O{{N}^{2}}$

Given, $AB>CD$

Therefore, $ON>OM$

Hence chord AB is nearer to the centre than CD.

2. OABC is a rhombus whose three vertices A, B and C lie on a circle with centre O.

(i). If the radius of the circle is 10cm, find the area of the rhombus.

(ii). If the area of rhombus is $32\sqrt{3}~c{{m}^{2}}$find the radius of the circle.

Ans:

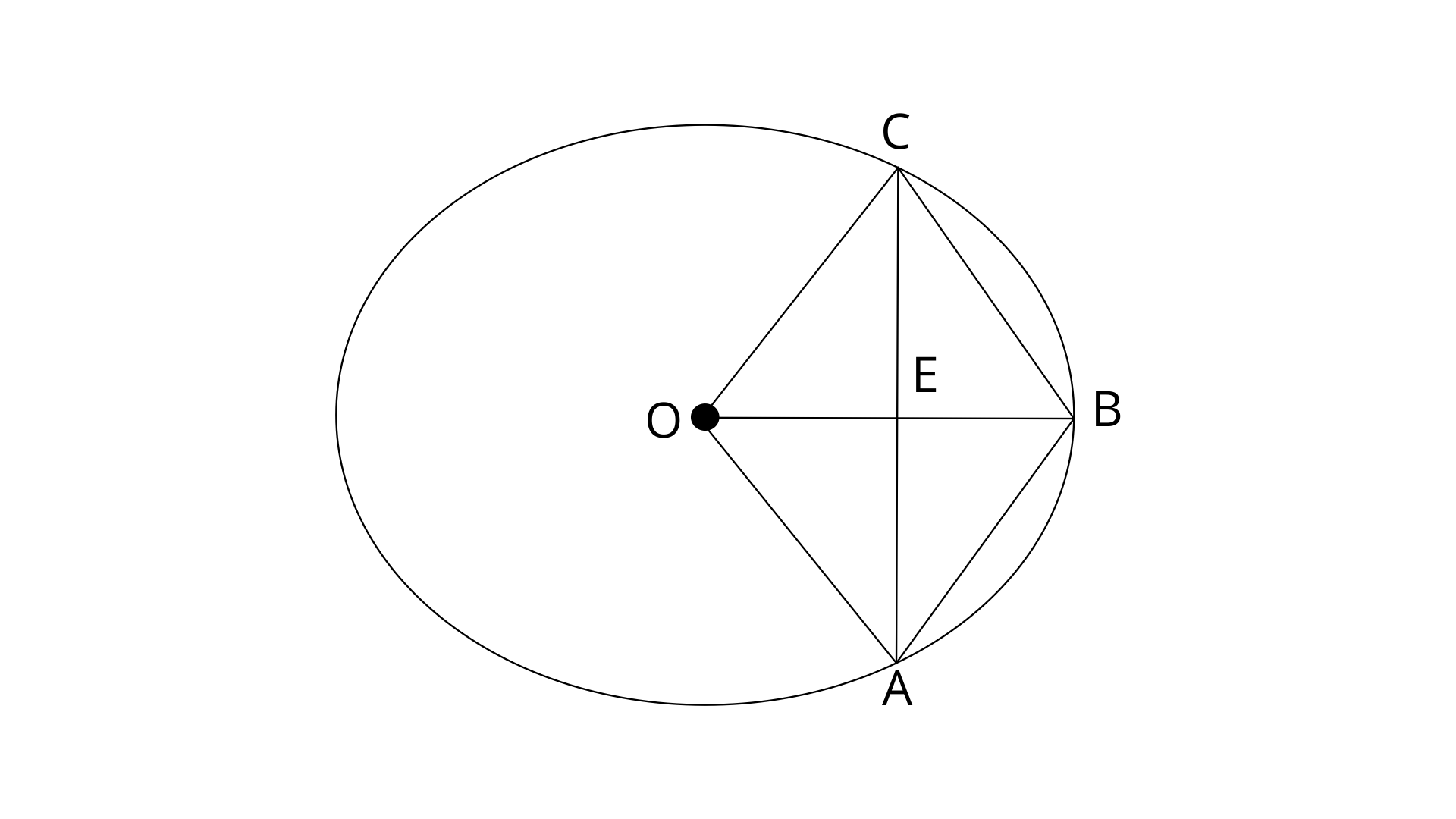
(i). If the radius of the circle is 10cm, find the area of the rhombus.

Ans: 

Radius=10cm

In rhombus OABC, 

OB=OC=OA=10cm

In a rhombus diagonal are perpendicular and bisect each other.

Therefore, $OE=\frac{1}{2}\times OB=\frac{10}{2}=5cm$

On using pythagoras theorem in $\Delta OEC$

$O{{C}^{2}}=O{{E}^{2}}+E{{C}^{2}}$

$\Rightarrow {{10}^{2}}={{5}^{2}}+E{{C}^{2}}$

$\Rightarrow E{{C}^{2}}=75$

$\Rightarrow EC=5\sqrt{3~}cm$

Now, $AC=2EC=10\sqrt{3~}$

Area of rhombus=$\frac{1}{2}\times OB\times AC$

                             $=\frac{1}{2}\times 10\times 10\sqrt{3~}=50\sqrt{3~}$

                              $\approx 86.6~c{{m}^{2}}$

Therefore the area of rhombus is $86.6~c{{m}^{2}}$

(ii). If the area of rhombus is $32\sqrt{3}~c{{m}^{2}}$find the radius of the circle.

Ans: Area of rhombus is $32\sqrt{3}~c{{m}^{2}}$.

The area of rhombus = $2\times area~of~\Delta OAB$ 

$Area~of~\Delta OAB=\frac{1}{2}\times Base\times Height=\frac{1}{2}\times r\times \sqrt{{{r}^{2}}-{{\left( \frac{r}{2} \right)}^{2}}}=\frac{\sqrt{3}}{4}{{r}^{2}}$

The area of rhombus=$2\times \frac{\sqrt{3}}{4}{{r}^{2}}$ 

$2\times \frac{\sqrt{3}}{4}{{r}^{2}}=32\sqrt{3}~$

$\Rightarrow {{r}^{2}}=64$

$\Rightarrow r=8$

Therefore the radius of the rhombus is 8cm.

3. Two circles with centres A and B, and radii 5cm and 3cm, touch each other internally. If the perpendicular bisector of the segment AB meets the bigger circle in P and Q; find the length of PQ.

Ans: 

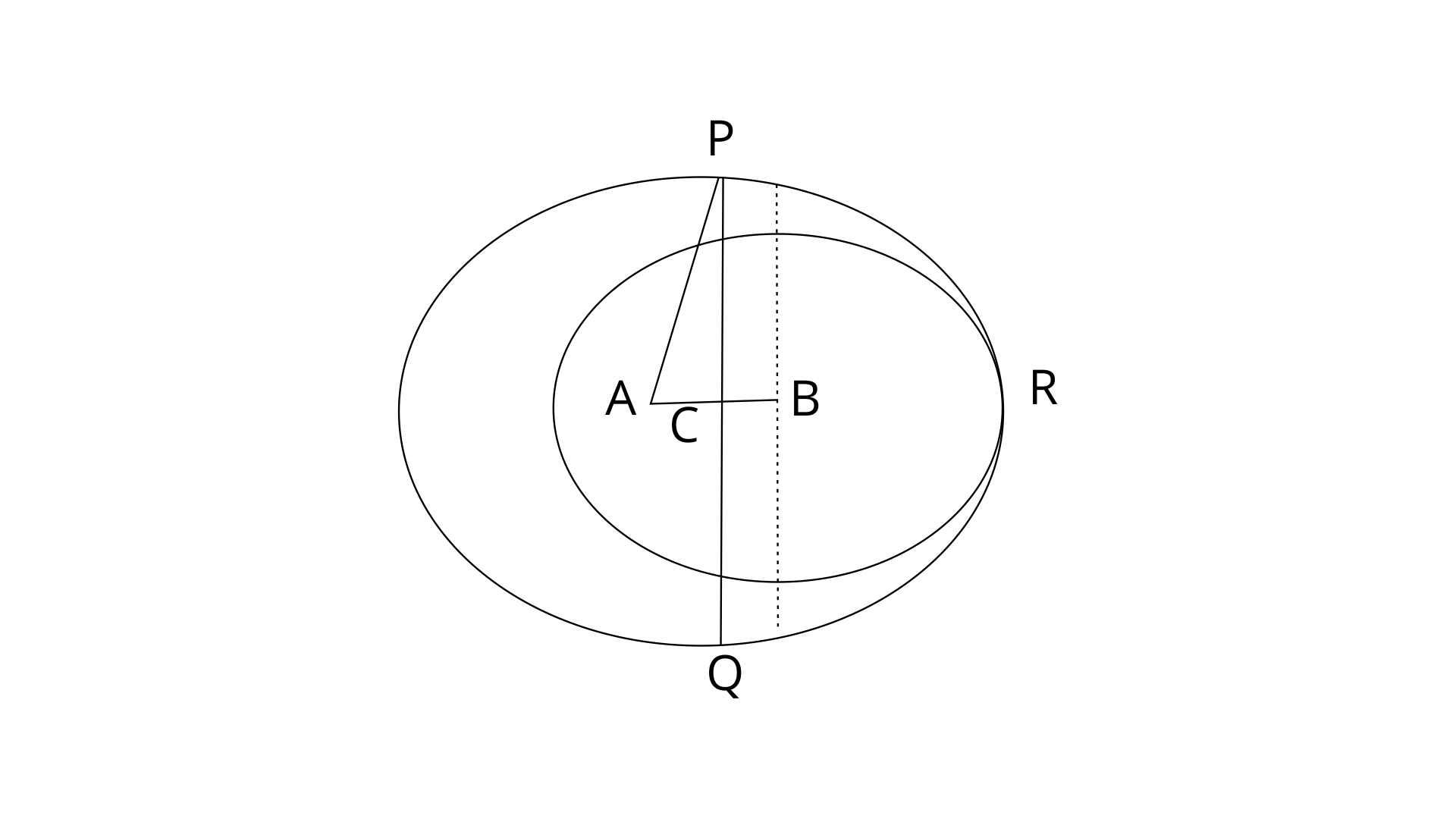
If circles touch internally then the distance between their centres is equal to the difference of their radii.

So, AB=(5-3)=2cm

Given, the common chord PQ is perpendicular to the bisector of AB.

Therefore, $AC=CB=\frac{1}{2}\times AB=1cm$

By using pythagoras theorem in $\Delta ACP$

$A{{P}^{2}}=A{{C}^{2}}+P{{C}^{2}}$

$\Rightarrow P{{C}^{2}}={{5}^{2}}-{{1}^{2}}=24$

$\Rightarrow PC=2\sqrt{6}~cm$

Now, $PQ=2PC=4\sqrt{6}~cm$

Therefore the length of $PQ$is $4\sqrt{6}~cm$

4. Two chords AB and AC of a circle are equal. Prove that the centre of the circle lies on the circle of angle BAC.

Show that the bisector of angle BAC is a perpendicular bisector of chord BC.

Ans:

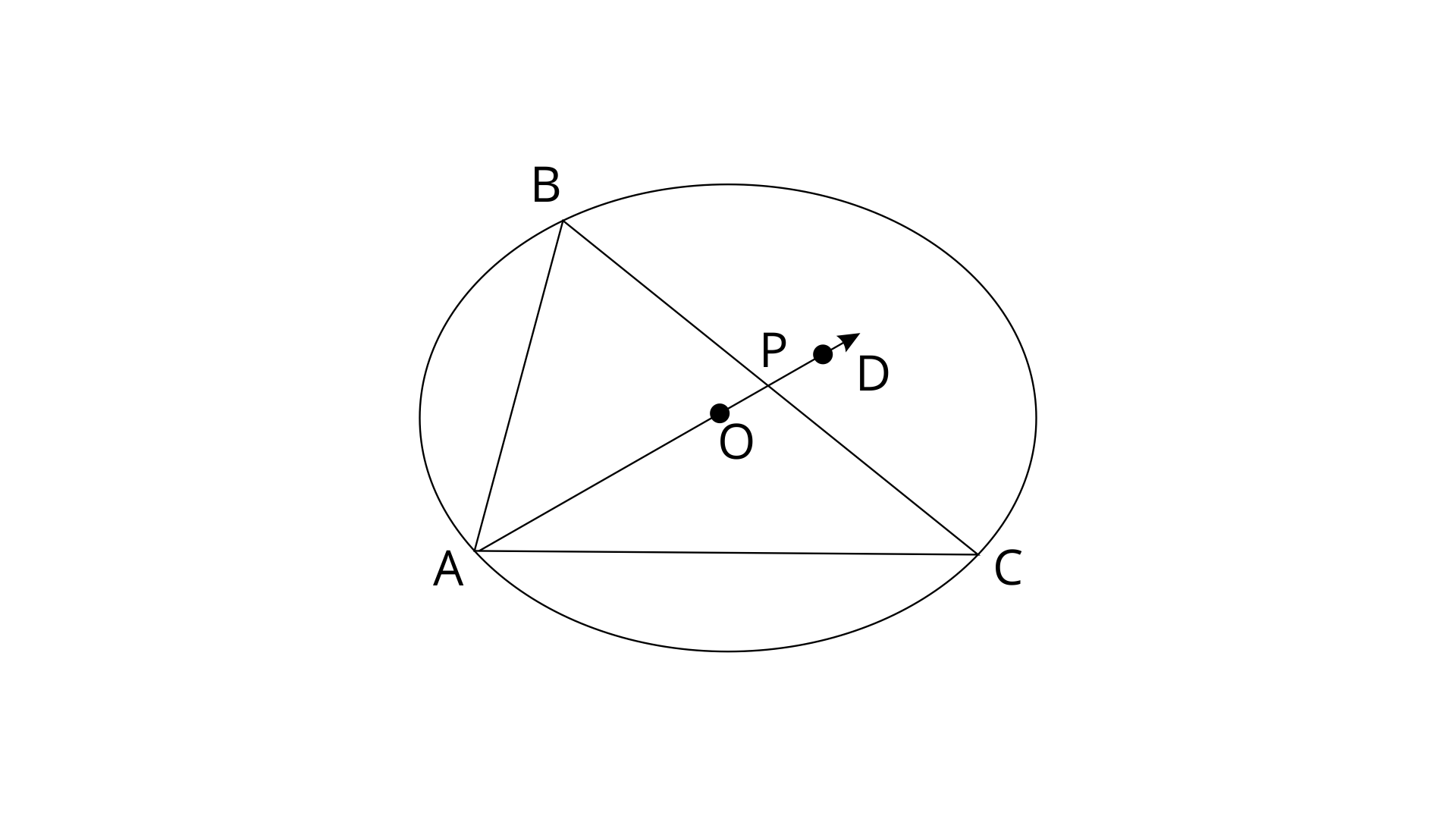
In $\Delta APB~and~\Delta APC$

AB=AC (Given)

AP=AP (common sides)

$\angle BAP=\angle CAP$(Given)

$\Delta APB\cong \Delta APC$ (SAS congruence criterion)

Therefore, BP=PC and $\angle APB=\angle APC$

$\angle APB+\angle APC={{180}^{\circ }}$(Linear pair)

$\Rightarrow 2\angle APB={{180}^{\circ }}$

$\Rightarrow \angle APB={{90}^{\circ }}$

Since BP=CP and $\angle APB={{90}^{\circ }}$

Therefore the angle bisector of BAC is perpendicular to BC.

5. The diameter and a chord of a circle have a common end-point. If the length of the diameter is 20cm and the length of the chord is 12cm, how far is the chord from the centre of the circle ?

Ans: 

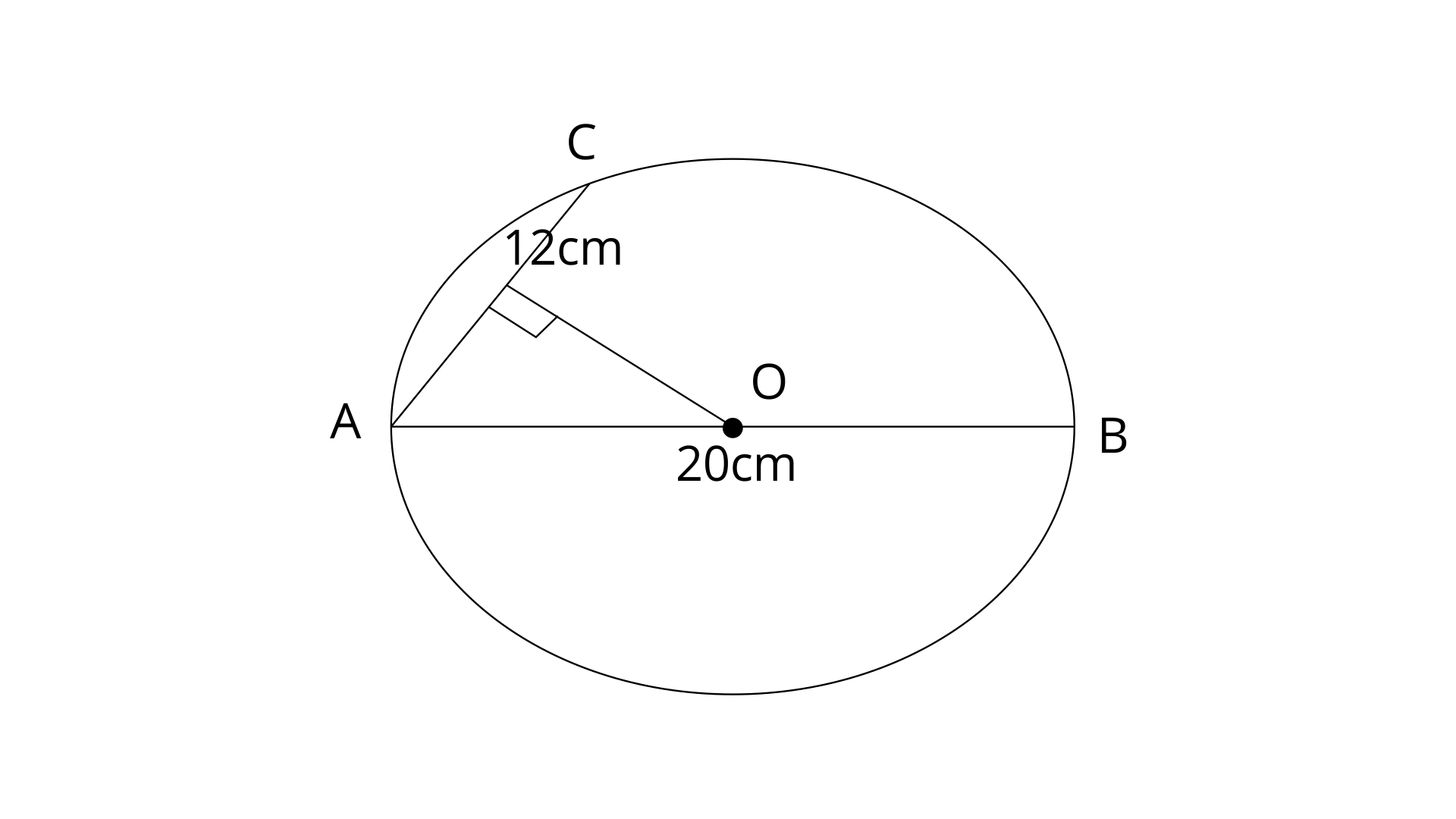
AB is diameter and AC is a chord. A is the common point of chord and diameter.

Draw $OL\bot AC$

The perpendicular on a chord drawn from the centre bisects the chord.

Therefore, AL=LC=$\frac{12}{2}=6cm$

$AO=\frac{AB}{2}==\frac{20}{2}=10cm$

Now by using pythagoras theorem in $\Delta ALO$

$O{{A}^{2}}=A{{L}^{2}}+O{{L}^{2}}$

$\Rightarrow O{{L}^{2}}={{\left( 10 \right)}^{2}}-{{\left( 6 \right)}^{2}}=64$

$\Rightarrow OL=8cm$

Therefore the chord is at a distance of 8cm from the centre of the circle.

6. ABCD is a cyclic quadrilateral in which BC is parallel to AD, angle $ADC={{110}^{\circ }}$and angle $BAC={{50}^{\circ }}$. Find angle DAC and angle DCA.

Ans: 

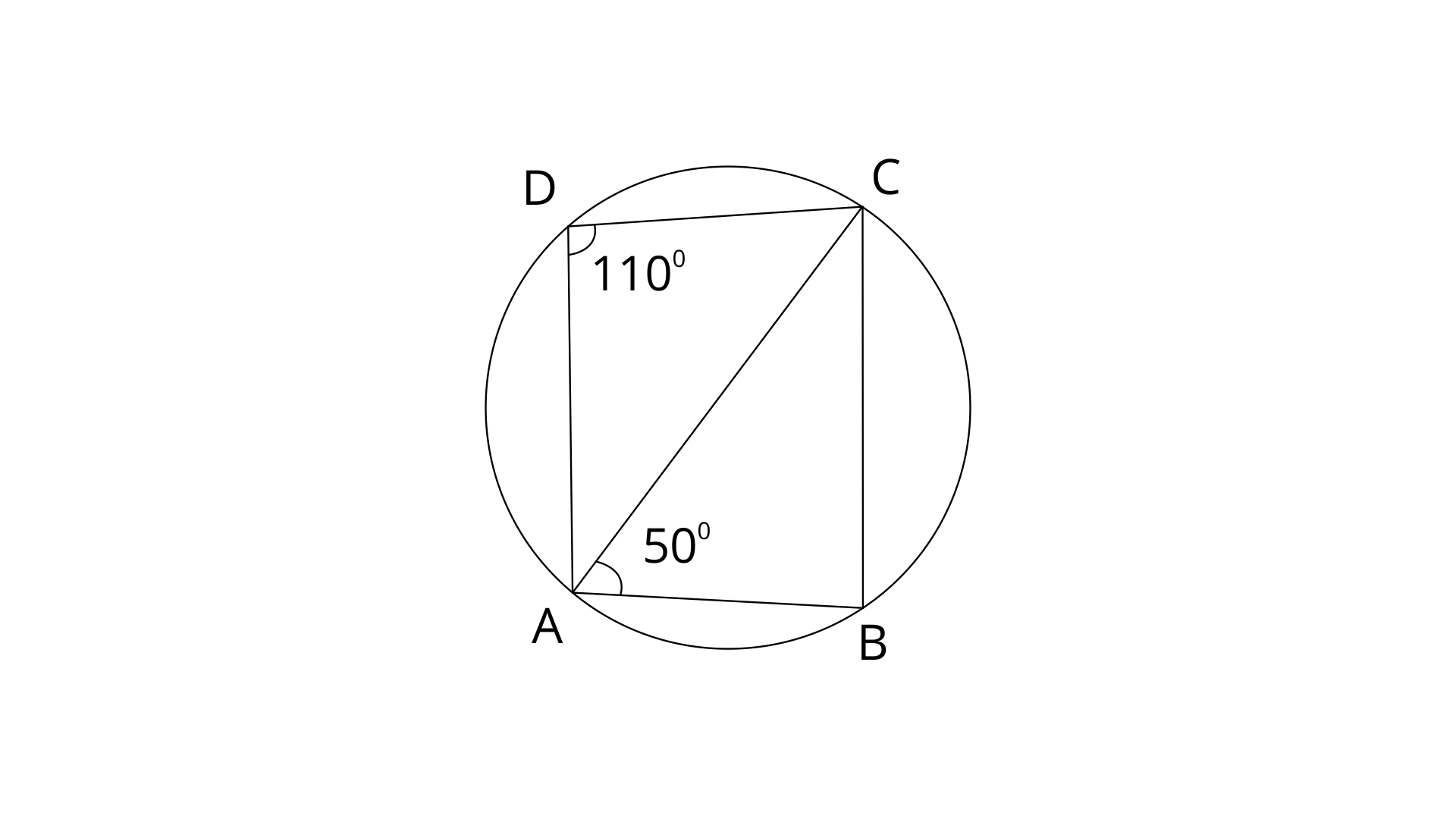
ABCD is a cyclic quadrilateral.

Therefore, $\angle B+\angle D={{180}^{\circ }}_{{}}$

$\Rightarrow \angle B={{180}^{\circ }}-{{110}^{\circ }}={{70}^{\circ }}$

By using angle sum property in $\Delta ABC~$

$\angle B+\angle BAC+\angle ACB={{180}^{\circ }}$

$\Rightarrow \angle ACB={{180}^{\circ }}-{{50}^{\circ }}-{{70}^{\circ }}={{60}^{\circ }}$

Since, AD||BC

Therefore, $\angle DAC=\angle ACB={{60}^{\circ }}$

Thus the value of $\angle DAC$is ${{60}^{\circ }}$.

By using angle sum property in $\Delta ADC~$

$\angle DAC+\angle ADC+\angle DCA={{180}^{\circ }}$

$\angle DCA={{180}^{\circ }}-{{110}^{\circ }}-{{60}^{\circ }}={{10}^{\circ }}$

Thus the value of $\angle DCA$is ${{10}^{\circ }}$.

7. In the given figure, C and D are points on the semi-circle described on AB as diameter. Given angle $BAD={{70}^{\circ }}$and angle $DBC={{30}^{\circ }}$, calculate angle BDC.

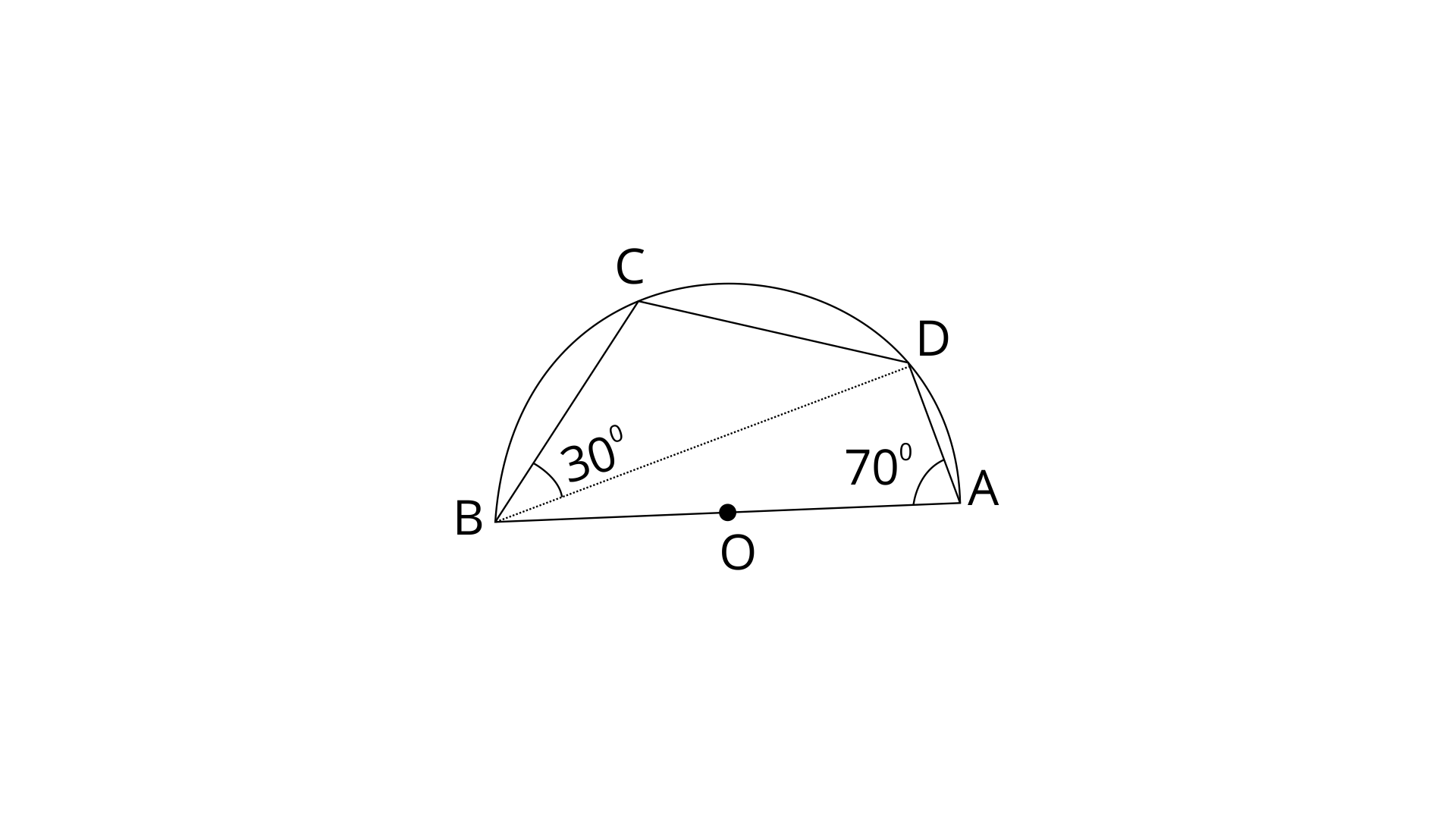
Ans: ABCD is a cyclic quadrilateral. In a cyclic quadrilateral the sum of opposite angles is ${{180}^{\circ }}$.

$\angle BCD+\angle BAD={{180}^{\circ }}$

$\Rightarrow \angle BCD={{180}^{\circ }}-{{70}^{\circ }}={{110}^{\circ }}$

By using angle sum property in $\Delta BCD$

$\angle BCD+\angle BDC+\angle CBD={{180}^{\circ }}$

$\angle BDC={{180}^{\circ }}-{{110}^{\circ }}-{{30}^{\circ }}={{40}^{\circ }}$

Thus the value of $\angle BDC$is ${{40}^{\circ }}$.

8. In a cyclic quadrilateral ABCD, $\angle A=3\angle C$and $\angle D=5\angle B$. Find the measure of each angle of the quadrilateral.

Ans: 

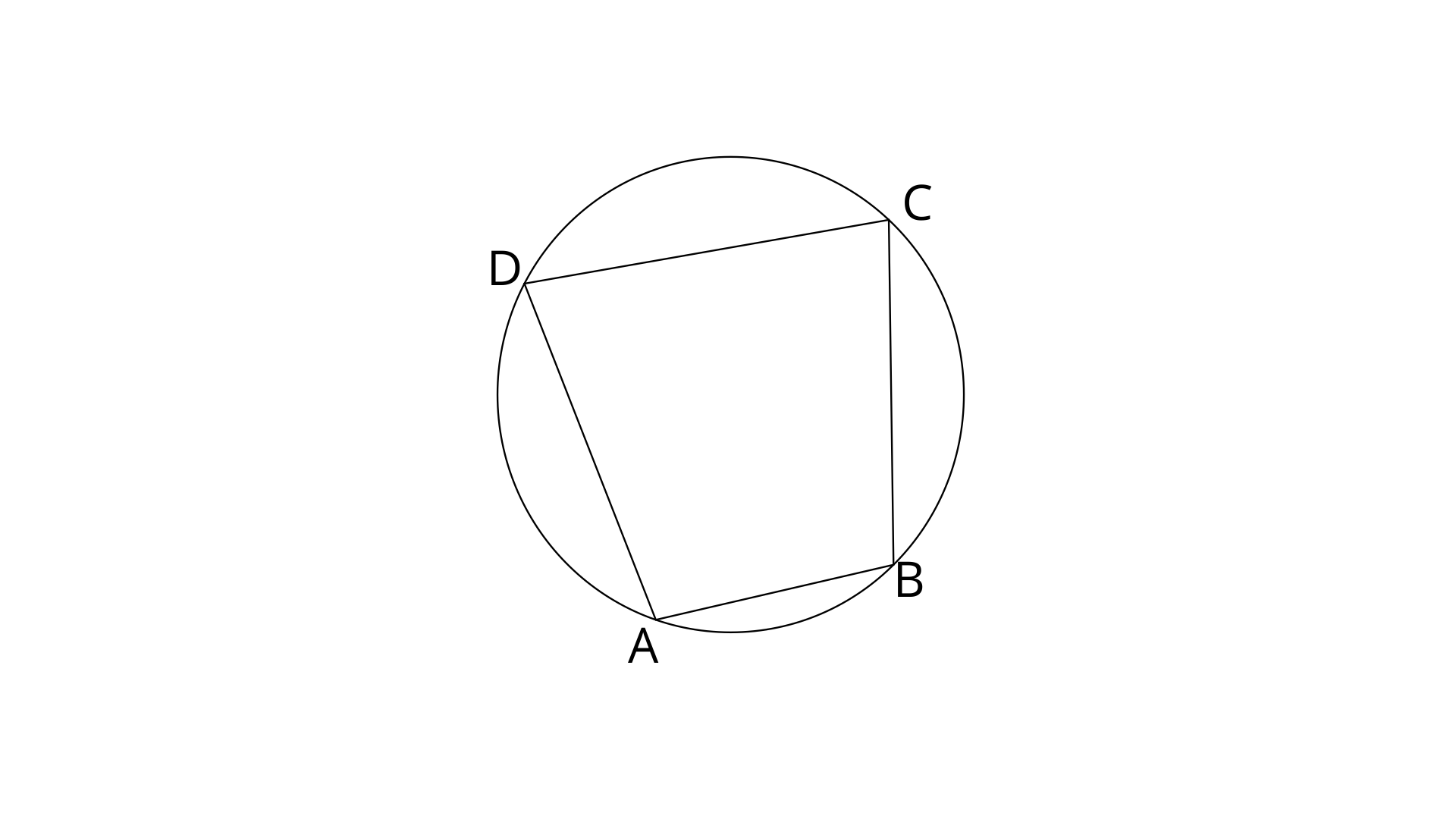
ABCD is a cyclic quadrilateral and in a cyclic quadrilateral the sum of opposite angles is ${{180}^{\circ }}$.

Therefore, $\angle A+\angle C={{180}^{\circ }}$

$\Rightarrow 3\angle C+\angle C={{180}^{\circ }}$ [∵ Given $\angle A=3\angle C$]

$\Rightarrow 4\angle C={{180}^{\circ }}$

$\Rightarrow \angle C={{45}^{\circ }}$

$\Rightarrow \angle A=3\angle C=3\times {{45}^{\circ }}={{135}^{\circ }}$

Similarly, $\angle B+\angle D={{180}^{\circ }}$

$\Rightarrow \angle B+5\angle B={{180}^{\circ }}$

$\Rightarrow 6\angle B={{180}^{\circ }}$

$\Rightarrow \angle B={{30}^{\circ }}$

$\Rightarrow \angle D=5\angle B=5\times {{30}^{\circ }}={{150}^{\circ }}$

Thus the value of $\angle A={{135}^{\circ }},\angle B={{30}^{\circ }},~\angle C={{45}^{\circ }},~\angle D={{150}^{\circ }}~$

9. Show that the circle drawn on any one of the equal sides of an isosceles triangle as diameter bisects the base.

Ans: 

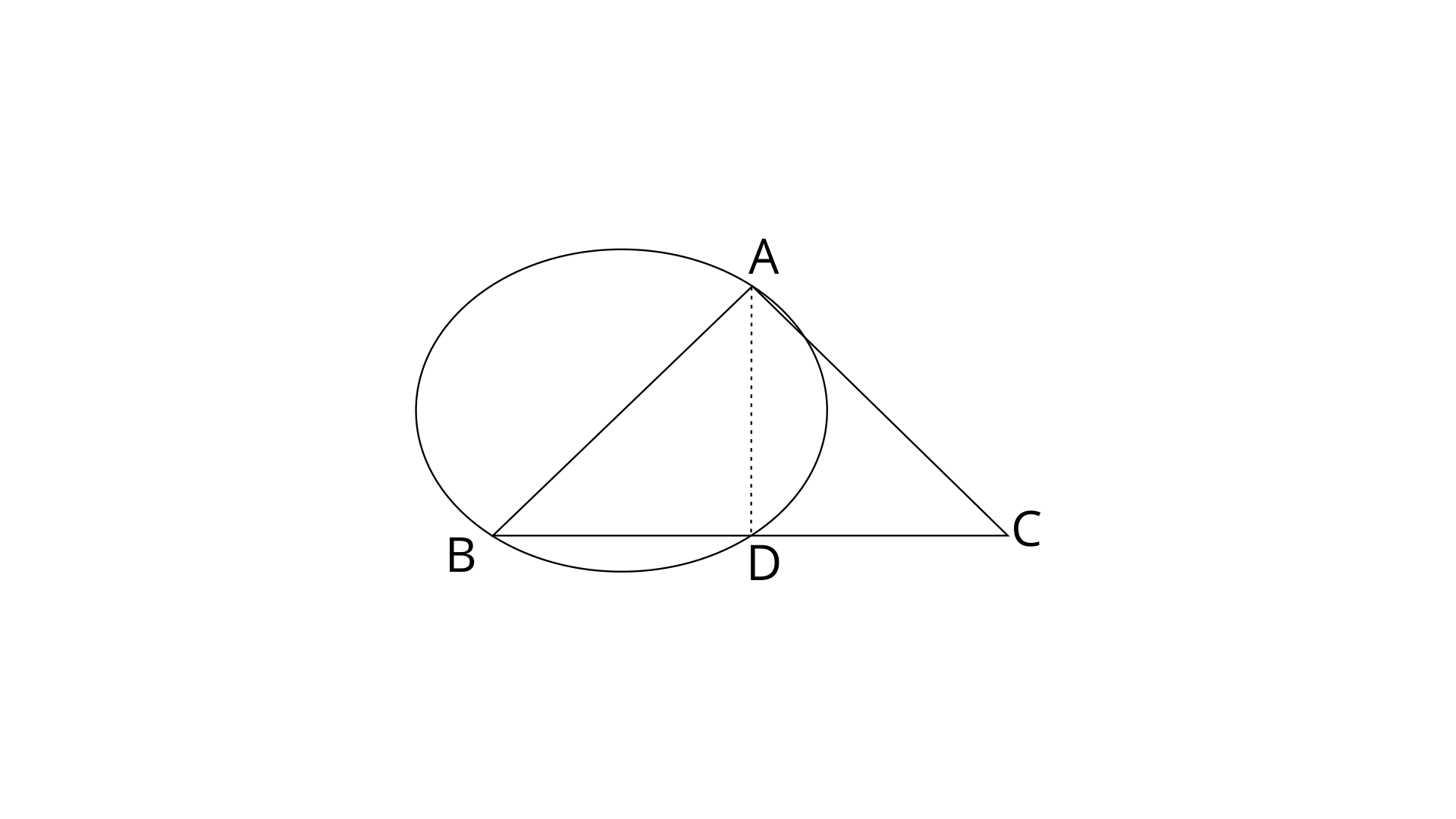
AB is a diameter and $\angle ADB$is subtended by the diameter of the circumference. In a circle, Angle at the circumference subtended by diameter is the right angle. Therefore, $\angle ADB={{90}^{\circ }}$.

BDC is straight line. 

$\angle ADB+\angle ADC={{180}^{\circ }}$

$\Rightarrow \angle ADC={{180}^{\circ }}-{{90}^{\circ }}={{90}^{\circ }}$

In $\Delta ADB~and~\Delta ADC$

AD=AD (common side)

$\angle ADB=\angle ADC={{90}^{\circ }}$

AB=AC (Given)

$\Delta ADB~\cong ~\Delta ADC$(SAS congruence criterion)

Therefore, BD=CD (C.P.C.T)

Hence, The circle bisects the base BC at D.

10. Bisectors of vertex angles A, B and C of a triangle ABC intersect its circumcircle at the point D, E and F respectively. Prove that angle $EDF={{90}^{\circ }}-\frac{1}{2}\angle A$.

Ans:

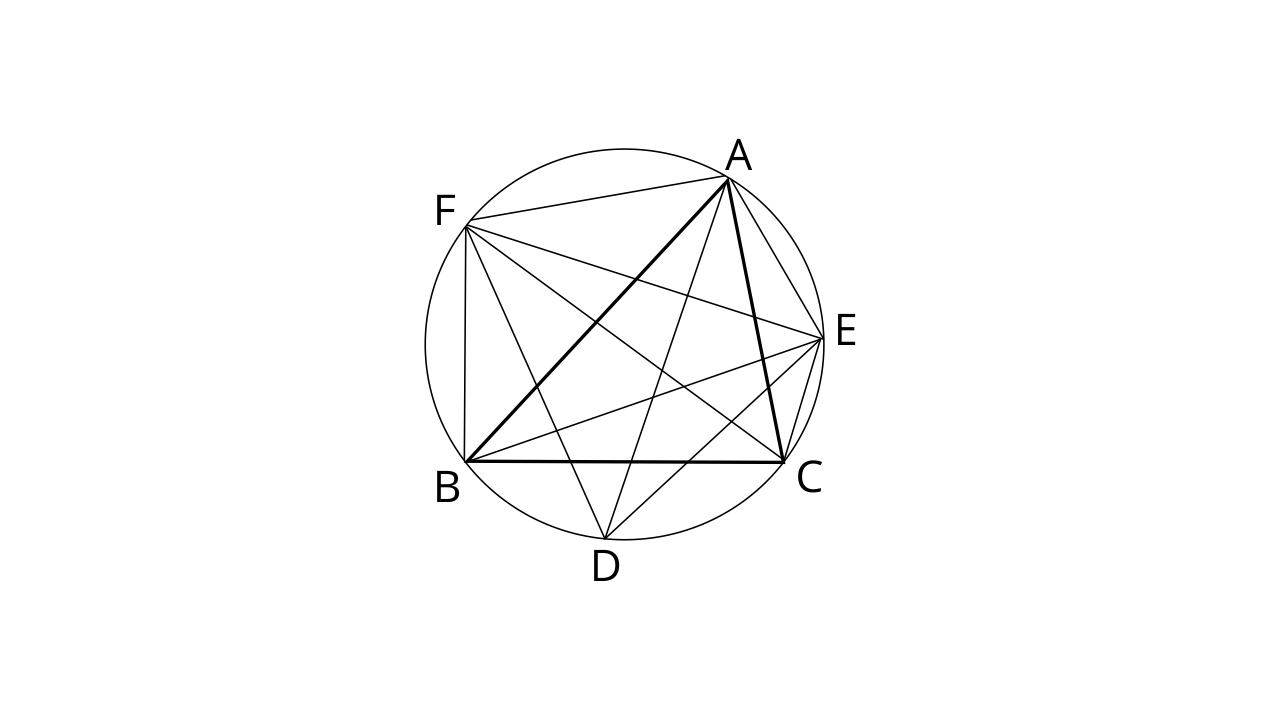
AD, BE and CF are angle bisectors of $\angle A,~\angle B~and~\angle C$.

Therefore, $\angle ABE=\frac{\angle B}{2}$

$\angle ABE=\angle ADE=\frac{\angle B}{2}$(Angles subtended by same chord AE)

Similarly, $\angle ACF=\angle ADF=\frac{\angle C}{2}$(Angle in the same segment for chord AF )

$\angle D=\angle ADE+\angle ADF$

$\Rightarrow \angle D=\frac{\angle B}{2}+\frac{\angle C}{2}$----------(1)

Sum of all interior angle in a triangle is ${{180}^{\circ }}$

$\angle A+\angle B+\angle C={{180}^{\circ }}$

$\Rightarrow \angle B+\angle C={{180}^{\circ }}-\angle A$

Now substituting the above value in eq. (1)

$\Rightarrow \angle D=\frac{1}{2}\left( {{180}^{\circ }}-\angle A \right)={{90}^{\circ }}-\frac{1}{2}\angle A$   (Hence proved)

11. In the figure, AB is the chord of a circle with centre O and DOC is a line segment such that BC=DO. If $\angle C={{20}^{\circ }}$, find angle AOD. 

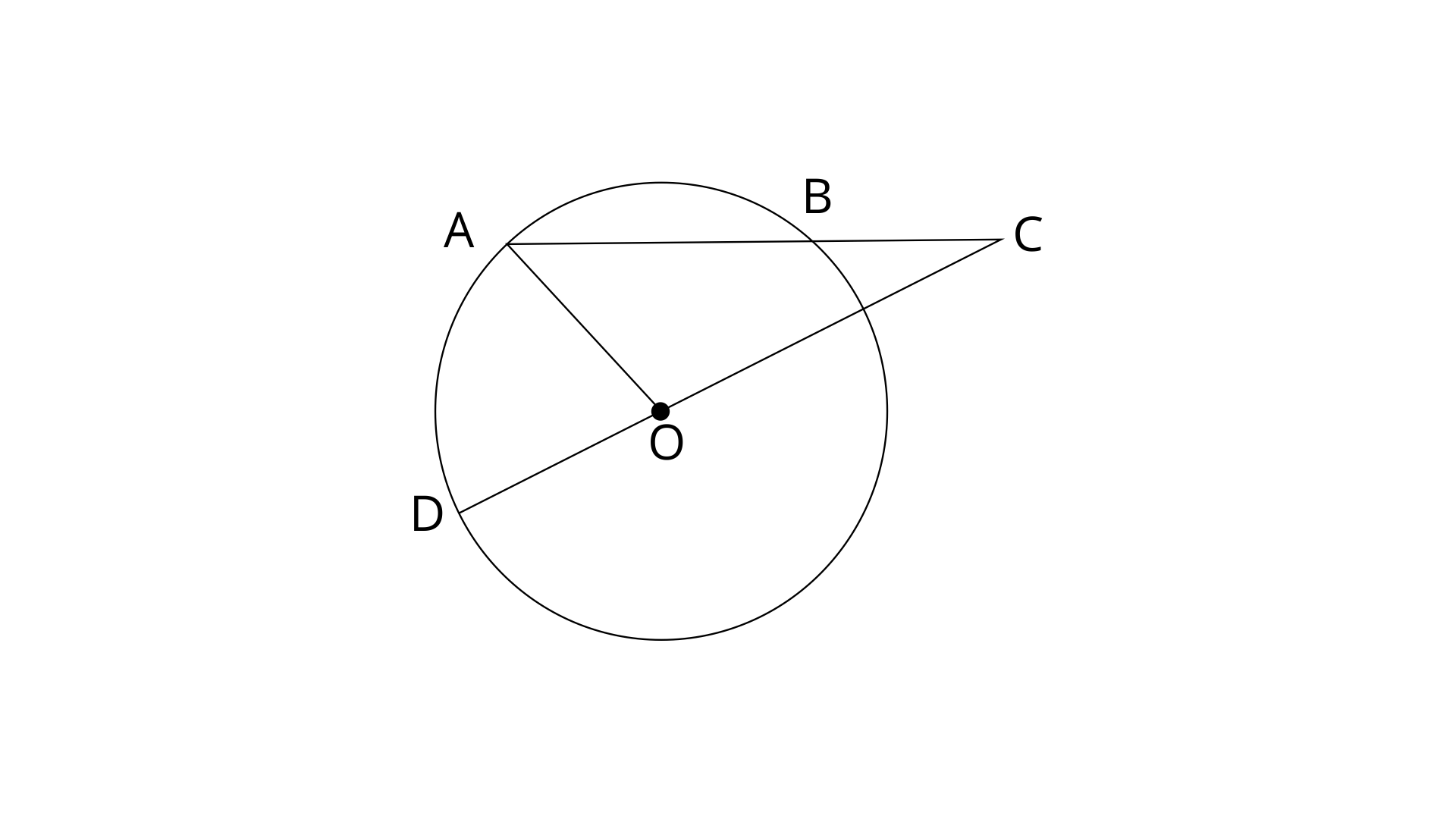
Ans: Join OB

BC=OD (Given)

OD=OA=OB (Radii of same circle)

Therefore, OD=OB=BC 

$\therefore \angle BOC=\angle BCO={{20}^{\circ }}$

In $\Delta BCO$by using exterior angle  property,

$\angle ABO=\angle BOC+\angle BCO={{20}^{\circ }}+{{20}^{\circ }}={{40}^{\circ }}$

Since, OA=OB(Radii of same circle)

$\angle OAB=\angle ABO={{40}^{\circ }}$

In $\Delta AOB$by using angle sum property,

$\angle AOB+\angle ABO+\angle OAB={{180}^{\circ }}$

$\Rightarrow \angle AOB={{180}^{\circ }}-{{40}^{\circ }}-{{40}^{\circ }}={{100}^{\circ }}$

DOC is a straight line, 

$\angle AOD+\angle AOB+\angle BOC={{180}^{\circ }}$

$\Rightarrow \angle AOD={{180}^{\circ }}-{{100}^{\circ }}-{{20}^{\circ }}={{60}^{\circ }}$

Thus the value of $\angle AOD$is ${{60}^{\circ }}$.

12. Prove that the perimeter of a right triangle is equal to the sum of the diameter of its incircle and twice the diameter of its circumcircle.

Ans: 

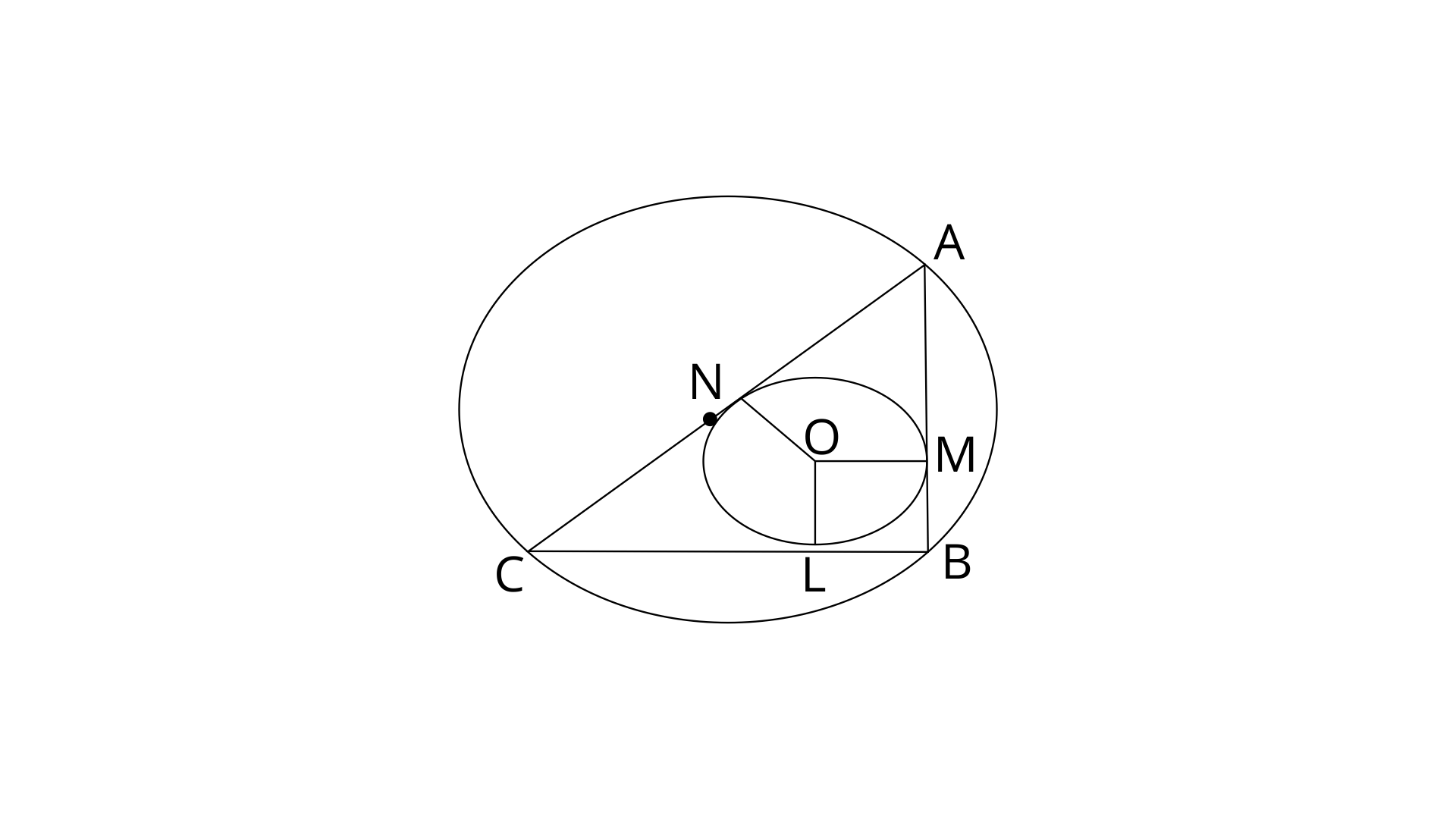
Let D and R the diameter and radius of circumcircle and let d and r the diameter and r the diameter and radius of incircle.

In $\Delta ABC$

$\angle B={{90}^{\circ }}$

AC is the diameter of the circumcircle Therefore, AC=D

And, OL=OM=ON=r

Now, from B, BL and BM are tangents.

BL=BM=r (∵BL=OM)

Similarly, AN=AM=R (Tangents from C) and CL=CN=R (Tangents from C)

Perimeter of triangle is sum of all the sides,

$AB+BC+AC=\left( AM+BM \right)+\left( AN+CN \right)+\left( CL+BL \right)$

$=\left( R+r \right)+\left( R+R \right)+\left( R+r \right)$

$=4R+2r$

$=2D+d$ (∵2R=D and 2r=d)

13. P is the midpoint of an arc APB of a circle. Prove that the tangent drawn at P will be parallel to the chord AB.

Ans:

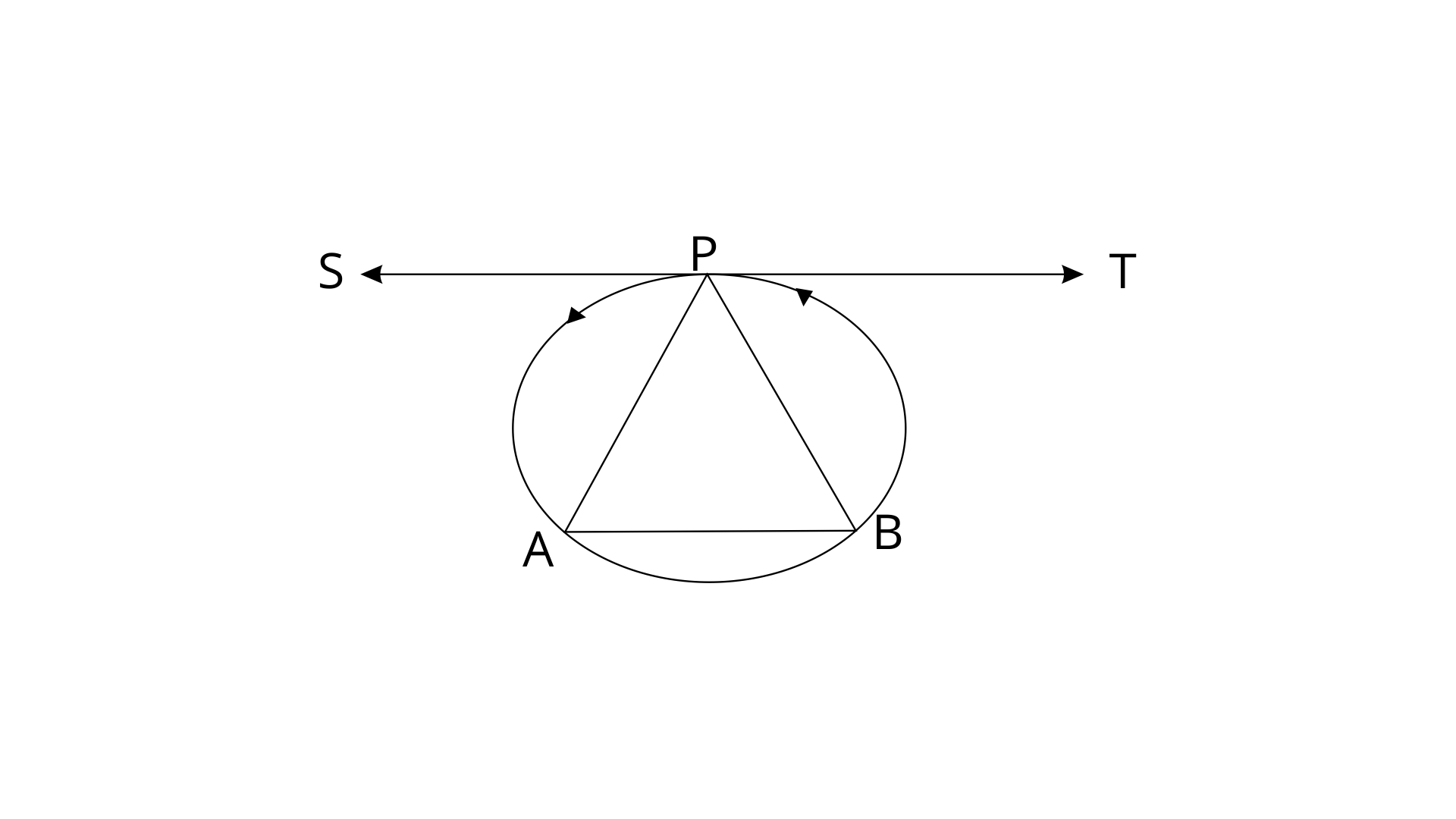
Since TPS is tangent to the circle and PB is chord.

$\angle BPT=\angle PAB$(Angles in alternate segments) ---------(1)

Since P is the midpoint of arc AB. Hence Chord PA=PB

$\angle PAB=\angle PBA$ ($\because PA=PB$)  -------(2)

From eq.(1) and (2)

$\angle BPT=\angle PBA$

But these are alternate angles. 

Therefore, TPS||AB

14. In the given figure, MN is the common chord of two intersecting circles and AB is their common tangent.

Prove that the line NM produced bisects AB at P. 

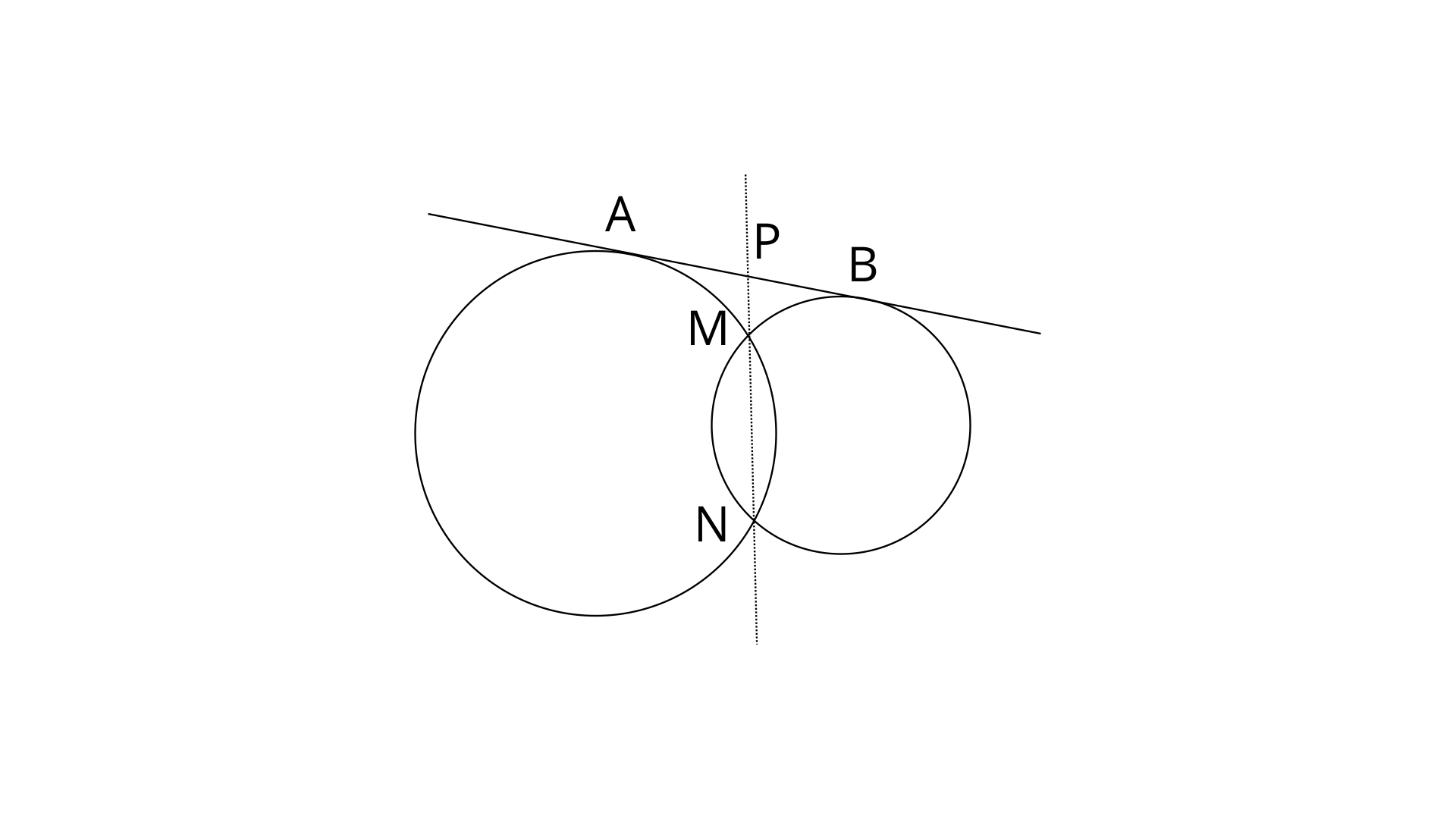
Ans:

AP is a tangent to the circle from the point P and PMN is secant.

Therefore, $A{{P}^{2}}=PM\times PN$-------(1)

Similarly, PB is tangent to the circle from point P and PMN is secant.

Therefore, $P{{B}^{2}}=PM\times PN$ --------(2)

From eq.(1) and (2)

$A{{P}^{2}}=P{{B}^{2}}$

$\Rightarrow AP=PB$

Therefore, Line NM produced bisects AB at P.

15. In the given figure, ABCD is a cyclic-quadrilateral, PQ is tangent to the circle at point C and BD is its diameter.

If $\angle DCQ={{40}^{\circ }}$and $\angle ABD={{60}^{\circ }}$, find :

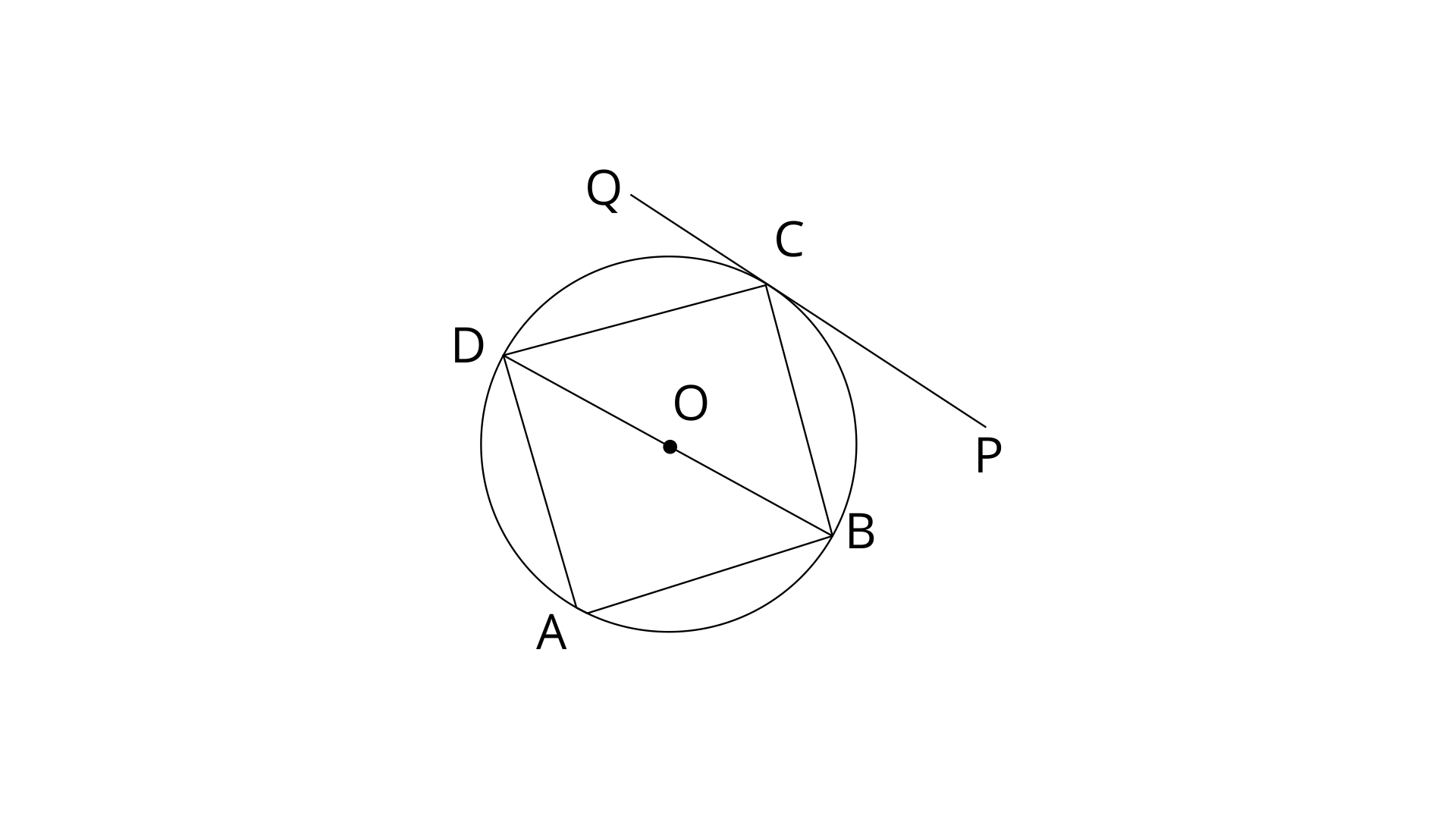
(i)$\angle DBC$

Ans: PQ is a tangent to the circle and DC is the chord.

Therefore, $\angle DCQ=\angle DBC$(Angles in alternate segments)

∴ $\angle DBC={{40}^{\circ }}$

(ii). $\angle BCP$

Ans: Angle $\angle DCB$is subtended by diameter BD. The angle subtended by the diameter is right angle. Therefore, $\angle DCB={{90}^{\circ }}$

PCQ is straight line.

$\angle DCQ+\angle DCB+\angle BCP={{180}^{\circ }}$

$\Rightarrow \angle BCP={{180}^{\circ }}-{{90}^{\circ }}-{{40}^{\circ }}={{50}^{\circ }}$

(iii). $\angle ADB$

Ans: $\angle DAB$is subtended by the diameter BD. Therefore, $\angle DAB={{90}^{\circ }}$

By using angle sum property in $\Delta ABD$,

$\angle ADB+\angle ABD+\angle DAB={{180}^{\circ }}$ 

$\Rightarrow \angle ADB={{180}^{\circ }}-{{90}^{\circ }}-{{60}^{\circ }}={{30}^{\circ }}$

Thus the value of $\angle ADB$is${{30}^{\circ }}$.

16. The given figure shows a circle with centre O and BCD is tangent to it at C. Show that: $\angle ACD+\angle BAC={{90}^{\circ }}$.

Ans: Join OC.

BCD is a tangent and OC is radius. Radius is perpendicular to the tangent.

Therefore,  $\angle OCB=\angle OCD={{90}^{\circ }}$

$\angle OCA+\angle ACD=\angle OCD={{90}^{\circ }}$----(1)

In $\Delta OCA$

OC=OA (Radii of same circle)

Therefore, $\angle OCA=\angle OAC$--------(2)

From eq.(1) and (2)

$\angle OAC+\angle ACD={{90}^{\circ }}$

$\angle BAC+\angle ACD={{90}^{\circ }}$ (∵$\angle BAC=\angle OAC$Angles b/w same line segments)

17. ABC is a right triangle with angle $B={{90}^{\circ }}$. A circle with BC as diameter meets hypotenuse AC at point D.Prove that :

(i). $AC\times AD=A{{B}^{2}}$

Ans:

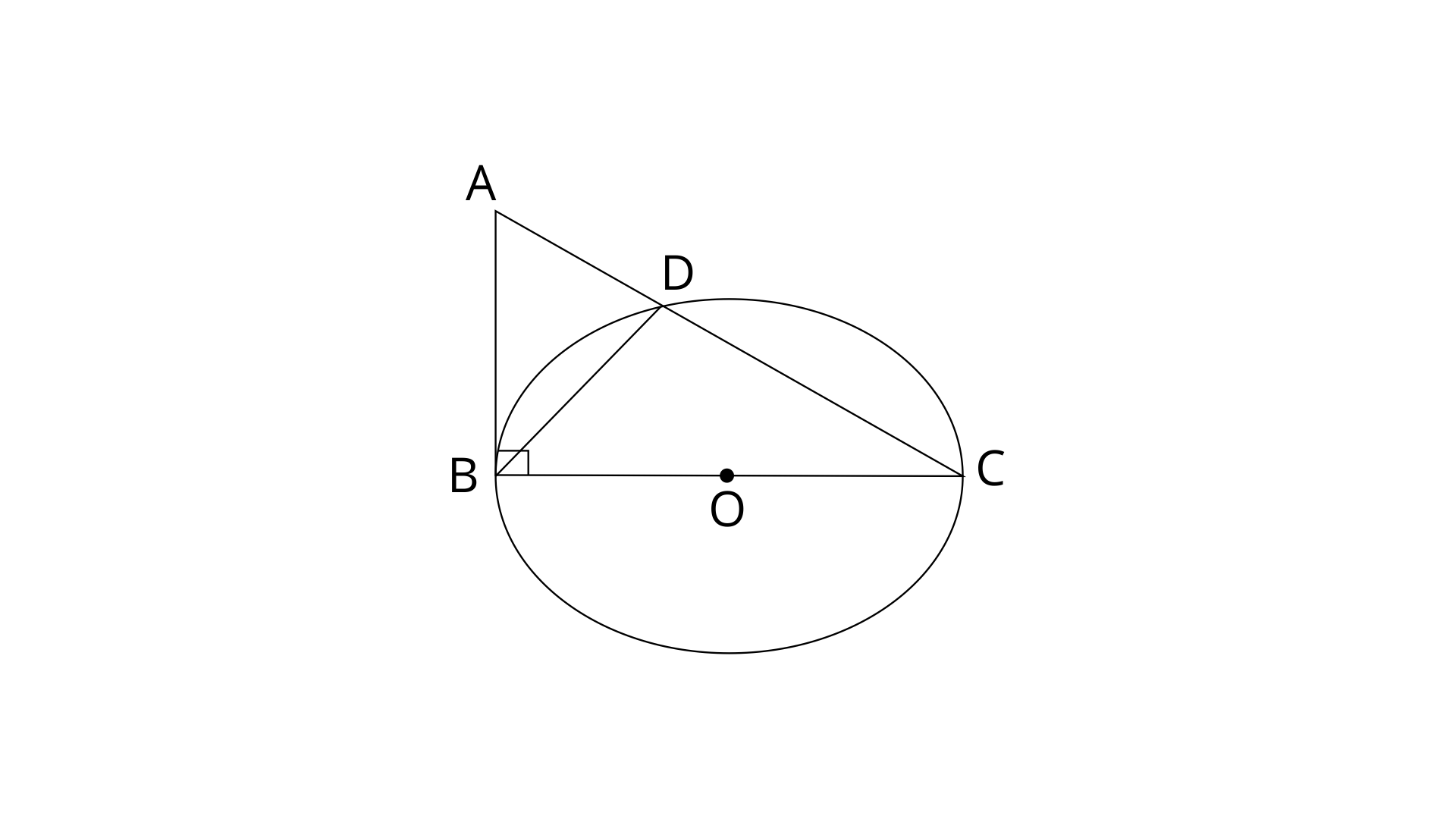
In $\Delta ABC$

$\angle B={{90}^{\circ }}$and BC is the diameter of a circle. 

Therefore, AB is tangent.

AB is tangent and ADC is secant. 

Therefore, $A{{B}^{2}}=AD\times AC$

(ii). $B{{D}^{2}}=AD\times DC$.

Ans: In $\Delta ABD$

By using exterior angle property, 

$\angle BAD+\angle ABD=\angle BDC={{90}^{\circ }}$--------(1)

Now, In $\Delta ABC$

$\angle A+\angle C={{90}^{\circ }}$

Since $\angle A=\angle BAD$(Angles b/w same line segments)

Therefore, $\angle BAD+\angle C={{90}^{\circ }}$------(2)

From eq. (1) and (2)

$\angle BAD+\angle C=\angle BAD+\angle ABD$

$\Rightarrow \angle C=\angle ABD$

Now in $\Delta ABD$and $\Delta CBD$

$\angle BDA=\angle BDC={{90}^{\circ }}$

$\angle ABD=\angle C$ 

BD=BD (common side)

Therefore, $\Delta ABD$and $\Delta CBD$are similar.

$\frac{BD}{CD}=\frac{AD}{BD}$

$B{{D}^{2}}=AD\times CD$

18. In the given figure, AC=AE. Show that :

(i). $CP=EP$

Ans: In $\Delta ADC$and $\Delta ABE$

$\angle A=\angle A$(Common angle)

AC=AE (Given)

$\angle ACD=\angle AEB$(Angles in same segments)

∴ $\Delta ADC~~\Delta ABE$

Therefore, AB=AD

But AC=AE

$\therefore AC-AB=AE-AD$

$\Rightarrow BC=DE$

In $\Delta BPC$and $\Delta DPE$

$\angle C=\angle E$(Alternate angles)

BC=DE 

$\angle CBP=\angle CDE$(Angles subtended by the same chord)

$\Delta BPC\cong \Delta DPE$

Therefore, CP=EP (C.P.C.T)

(ii). $BP=DP$

Ans: Since, $\Delta BPC\cong \Delta DPE$

Therefore, $BP=DP$(C.P.C.T)

19. ABCDE is a cyclic pentagon with centre of its circumcircle at point O such that AB=BC=CD and angle $ABC={{120}^{\circ }}$. Calculate :

(i). $\angle BEC$

Ans:

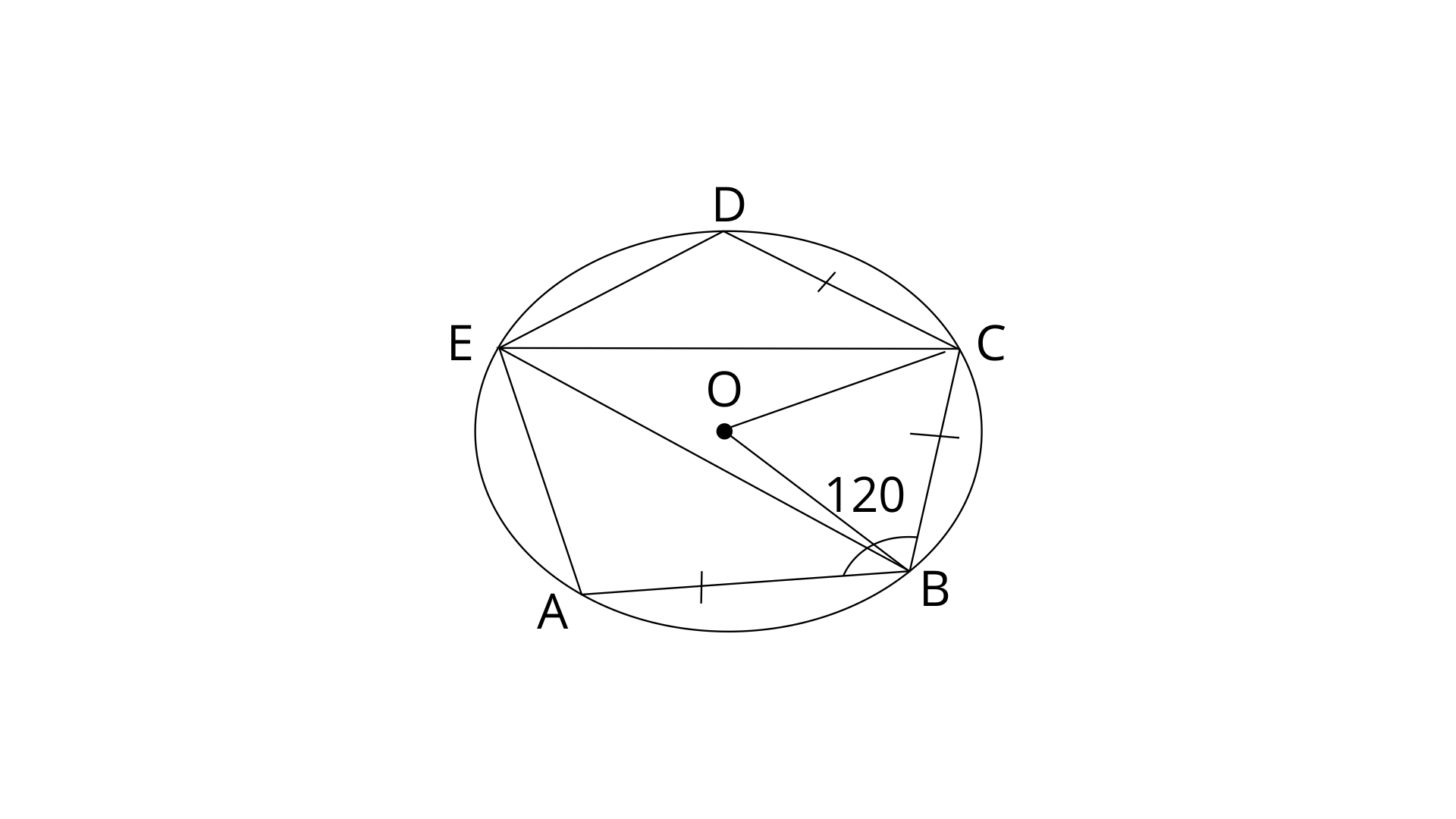
Given, AB=BC=CD and $\angle ABC={{120}^{\circ }}$

Therefore, $\angle ABC=\angle BCD={{120}^{\circ }}$

OB and OC are the bisectors of $\angle ABC$and $\angle BCD$ respectively.

$\angle OBC=\angle OCB={{60}^{\circ }}$

In $\Delta OBC$by using angle sum property,

$\angle OBC+\angle BOC+\angle OCB={{180}^{\circ }}$

$\Rightarrow \angle BOC={{180}^{\circ }}-{{60}^{\circ }}-{{60}^{\circ }}={{60}^{\circ }}$

In a circle, Angle at the centre is doubled the angle at the circumference subtended by the same chord.

Therefore, $\angle BOC=2\angle BEC$

$\angle BEC=\frac{{{60}^{\circ }}}{2}={{30}^{\circ }}$

(ii). $\angle BED$

Ans: BCDE is cyclic quadrilateral and in a cyclic quadrilateral the sum of opposite angles is ${{180}^{\circ }}$

$\angle BED+\angle BCD={{180}^{\circ }}$

$\Rightarrow \angle BED={{180}^{\circ }}-{{120}^{\circ }}={{60}^{\circ }}$

Thus the value of $\angle BED$is ${{60}^{\circ }}$.

20. In the given figure, O is the centre of the circle. Tangents at A and B meet at C. If $\angle ACO={{30}^{\circ }}$, find :

(i). $\angle BCO$

(ii). $\angle AOB$

(iii). $\angle APB$

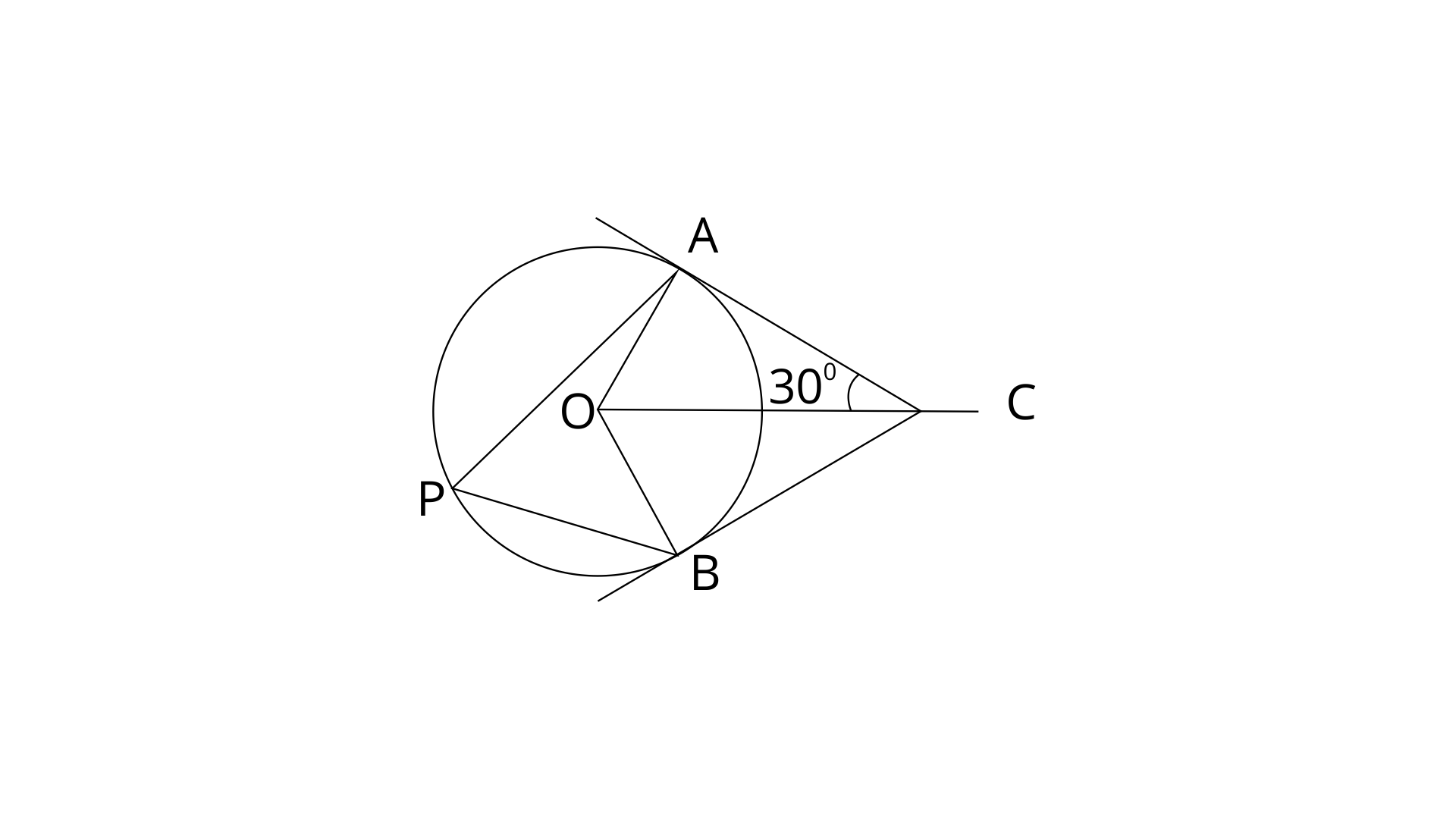
(i). $\angle BCO$

Ans: In $\Delta OAC$and $\Delta OBC$

OC=OC (common side)

OA=OB (Radii of same circle)

AC=BC (Tangents to the circle from external point C)

$\Delta OAC\cong \Delta OBC$(SSS congruence criterion)

Therefore, $\angle ACO=\angle BCO={{30}^{\circ }}$.

(ii). $\angle AOB$

Ans: $\angle ACB=\angle ACO+\angle BCO={{30}^{\circ }}+{{30}^{\circ }}={{60}^{\circ }}$

Sum of opposite angles in quadrilateral is ${{180}^{\circ }}$

$\angle ACB+\angle AOB={{180}^{\circ }}$

$\Rightarrow \angle AOB={{180}^{\circ }}-{{60}^{\circ }}={{120}^{\circ }}$

(iii). $\angle APB$

Ans: In a circle, Angle at the centre is doubled the angle at the circumference subtended by the same chord.

$\angle AOB=2\angle APB$

$\Rightarrow \angle APB=\frac{\angle AOB}{2}=\frac{{{120}^{\circ }}}{2}={{60}^{\circ }}$

21. ABC is a triangle with AB=10cm, BC=8cm and AC=6cm (not drawn to scale). There circles are drawn touching each other with the vertices as their centres. Find the radii of the three circles.

Ans: Given, $AC=6cm,~AB=10cm,~BC=8cm$

Let PA=QA=x (Radii of circle having centre A)

CR=CQ=y (Radii of circle having centre C)

BP=BR=z (Radii of circle having centre B)

$AC=AQ+QC=x+y=6cm$ ---------------(1)

$AB=AP+PB=x+z=10cm$--------------(2)

$BC=BR+RC=z+y=8cm$ ---------------(3)

On adding eq. (1), (2) and (3)

$2\left( x+y+z \right)=24cm$

$\Rightarrow \left( x+y+z \right)=12cm$------(4)

Subtracting eq.(1) from (4)

$z=12-6=6cm$

Subtracting eq.(2) from eq.(4)

$y=12-10=2cm$

Subtracting eq.(3) from eq.(4)

$x=12-8=4cm$

Therefore the radii of circles are 2cm, 4cm and 6cm.

22. In a square ABCD, its diagonals AC and BD intersect each other at point O. The bisector of angle DAO meets BD at point M and the bisector of angle ABD meets AC at N and AM at L. Show that :

(i). $\angle ONL+\angle OML={{180}^{\circ }}$

Ans: 

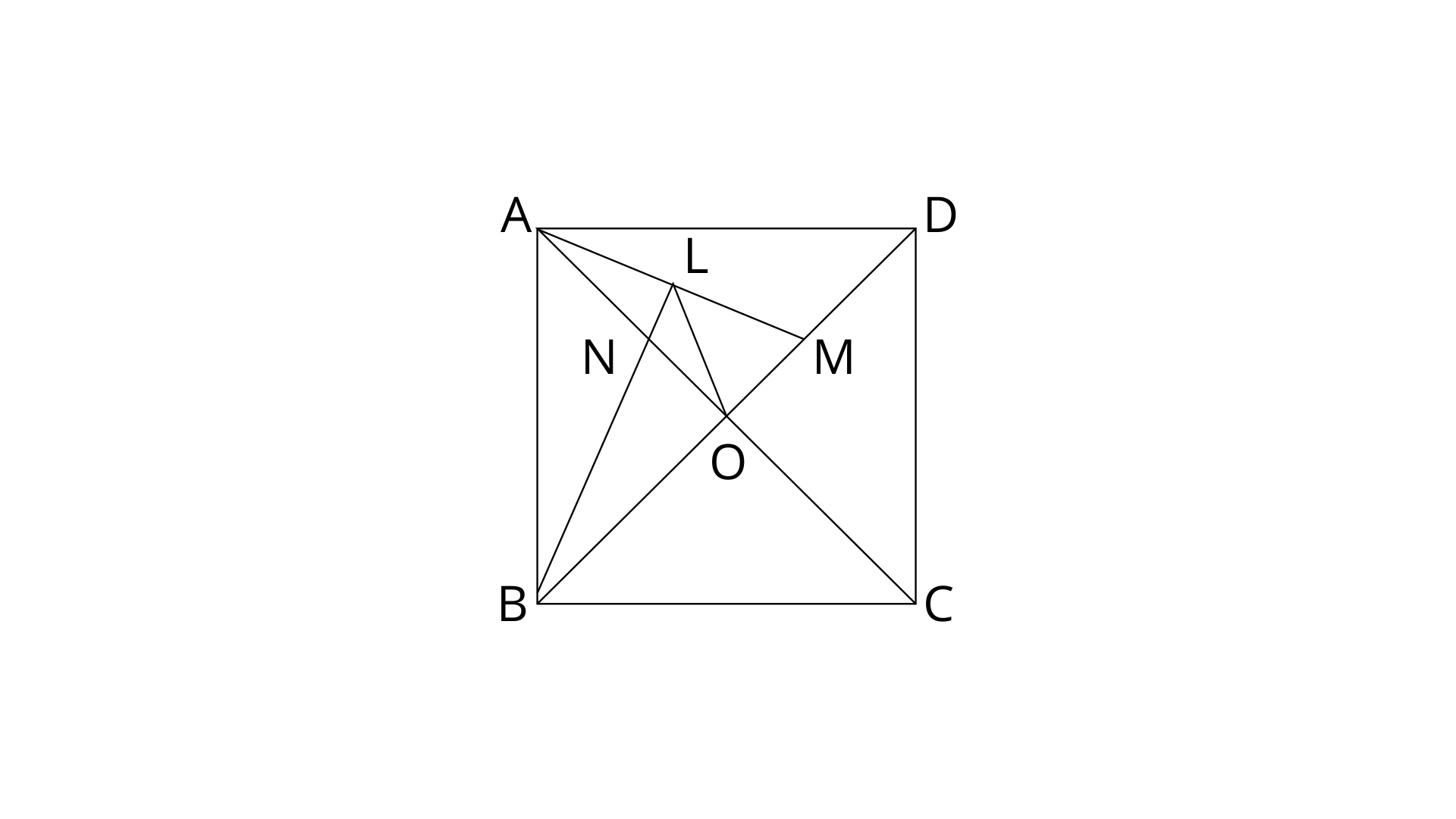
ABCD is a square. Hence, All four angles are right angle and diagonals are also intersect at ${{90}^{\circ }}$

$\angle AOB=\angle AOD={{90}^{\circ }}$

In $\Delta ANB$by using angle sum property,

$\angle ANB+\angle ABN+\angle BAN={{180}^{\circ }}$

$\Rightarrow \angle ANB={{180}^{\circ }}-{{45}^{\circ }}-\left( \frac{{{45}^{\circ }}}{2} \right)$ (NB is bisector of $\angle ABO$)

$\Rightarrow \angle ANB={{135}^{\circ }}-\left( \frac{{{45}^{\circ }}}{2} \right)$

Since, $\angle ANB=\angle ONL$(Vertically opposite angles)

Therefore, $\angle ONL={{135}^{\circ }}-\left( \frac{{{45}^{\circ }}}{2} \right)$    --------(1)

Again, In $\Delta AOM$by using angle sum property

$\angle AOM+\angle AMO+\angle OAM={{180}^{\circ }}$

$\Rightarrow \angle AMO={{180}^{\circ }}-{{90}^{\circ }}-\left( \frac{{{45}^{\circ }}}{2} \right)={{90}^{\circ }}-\left( \frac{{{45}^{\circ }}}{2} \right)$(AM is bisector of angle DAO)

$\Rightarrow \angle AMO={{90}^{\circ }}-\left( \frac{{{45}^{\circ }}}{2} \right)$-----------(2)

On adding eq. (1) and (2)

$\angle AMO+\angle ONL={{90}^{\circ }}-\left( \frac{{{45}^{\circ }}}{2} \right)+{{135}^{\circ }}-\left( \frac{{{45}^{\circ }}}{2} \right)={{135}^{\circ }}+{{45}^{\circ }}={{180}^{\circ }}$

Since, $\angle AMO=\angle OML$(Angles b/w same line segments)

Therefore, $\angle OML+\angle ONL={{180}^{\circ }}$

(ii). $\angle BAM=\angle BMA$

Ans: $\angle BAM=\angle BAO+\angle OAM={{45}^{\circ }}+\left( \frac{{{45}^{\circ }}}{2} \right)={{67}^{\circ }}\frac{1}{2}$

By using angle sum property in $\Delta AOM$

$\angle OAM+\angle OMA+\angle AOM={{180}^{\circ }}$

$\angle OMA={{180}^{\circ }}-{{90}^{\circ }}-\left( \frac{{{45}^{\circ }}}{2} \right)={{67}^{\circ }}\frac{1}{2}$

Since, $\angle BMA=\angle OMA={{67}^{\circ }}\frac{1}{2}$(Angles b/w same line segments)

Therefore, $\angle BMA=\angle BAM$

(iii). ALOB is a cyclic quadrilateral.

Ans: $\angle ABO+\angle ALO={{45}^{\circ }}+{{90}^{\circ }}+{{45}^{\circ }}={{180}^{\circ }}$

23. The given figure shows a semi-circle with centre O and diameter PQ. If PA=AB and $\angle BCQ={{140}^{\circ }}$; find measures of angles PAB and AQB. Also, show that AO is parallel to BQ. 

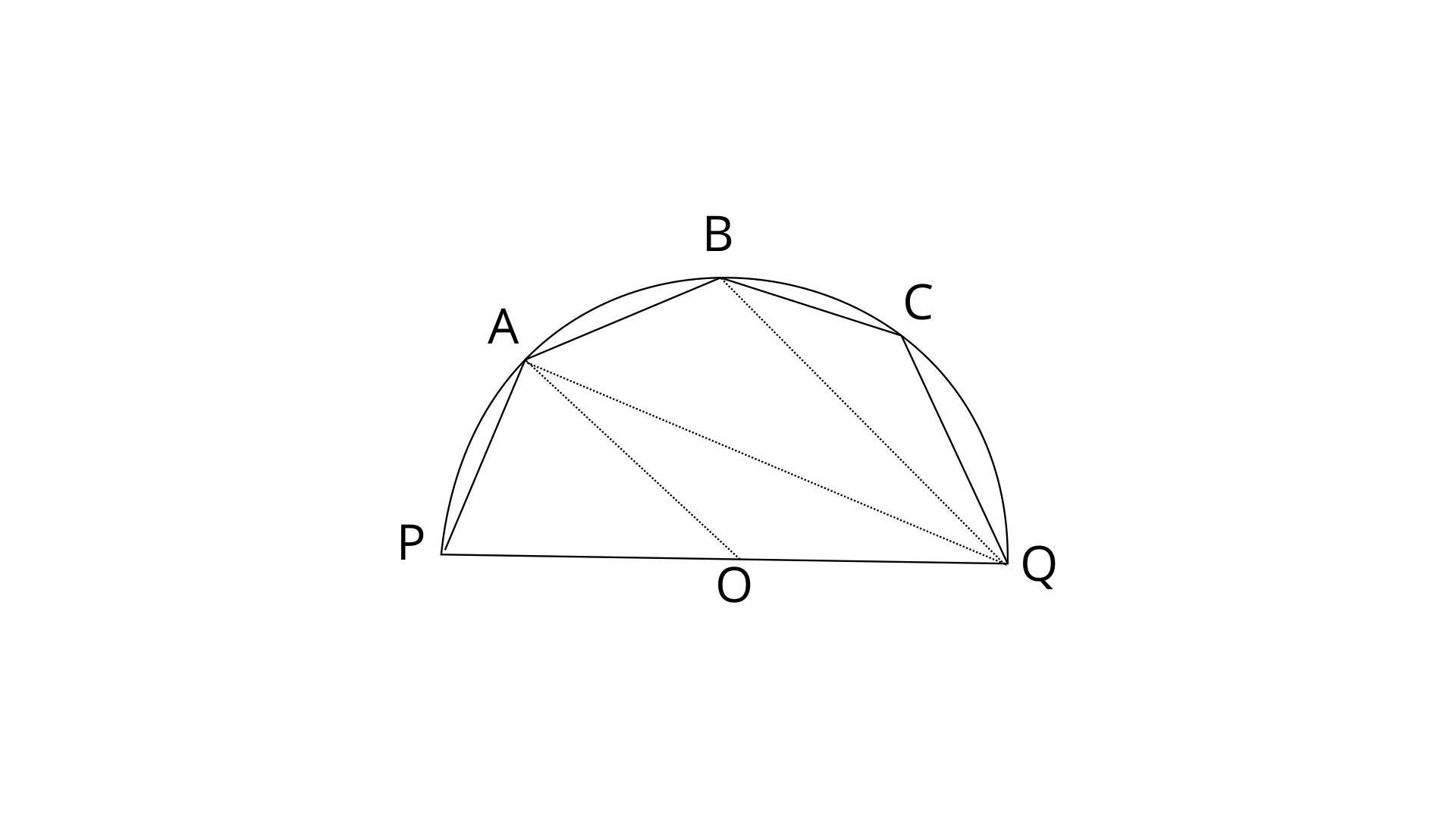
Ans: Join PB.

In cyclic quadrilateral PBCQ,

$\angle BCQ+\angle BPQ={{180}^{\circ }}$

$\angle BPQ={{180}^{\circ }}-{{140}^{\circ }}={{40}^{\circ }}$---------(1)

By using angle sum property in $\Delta PBQ$

$\angle BPQ+\angle PBQ+\angle PQB={{180}^{\circ }}$

$\Rightarrow \angle PQB={{180}^{\circ }}-{{90}^{\circ }}-{{40}^{\circ }}={{50}^{\circ }}$

In cyclic quadrilateral PABQ,

$\angle PAB+\angle PQB={{180}^{\circ }}$

$\Rightarrow \angle PAB={{180}^{\circ }}-{{50}^{\circ }}={{130}^{\circ }}$

Thus the value of $\angle PAB$is${{130}^{\circ }}$.

By using angle sum property in $\Delta PAB$

$\angle PAB+\angle APB+\angle ABP={{180}^{\circ }}$

$\angle APB+\angle ABP={{180}^{\circ }}-{{130}^{\circ }}={{50}^{\circ }}$

Given PA=AB

$\Rightarrow \angle APB=\angle ABP$(opposite angles of equal sides)

Therefore, $2\angle APB={{50}^{\circ }}\Rightarrow \angle APB={{25}^{\circ }}$

$\Rightarrow \angle APB=\angle ABP={{25}^{\circ }}$

Angles at the circumference subtended by the same chord are equal.

$\angle APB=\angle AQB={{25}^{\circ }}$(Angles subtended by the chord AB) -----(2)

$\angle BPQ=\angle BAQ={{40}^{\circ }}$(Angles subtended by the chord BQ)

Now, 

$\angle APQ=\angle APB+\angle BPQ$------(3)

From eq. (1), (2), and (3)

$\angle APQ={{25}^{\circ }}+{{40}^{\circ }}={{65}^{\circ }}$

Arc AQ subtends the angle $\angle AOQ$at the centre and $\angle APQ$at the circumference. 

$\angle AOQ=2\angle APQ=2\times {{65}^{\circ }}={{130}^{\circ }}$

By using angle sum property in $\Delta AOQ$

$\angle AOQ+\angle OAQ+\angle OQA={{180}^{\circ }}^{{}}$

$\Rightarrow \angle OAQ+\angle OQA={{180}^{\circ }}^{{}}-{{130}^{\circ }}$

$\Rightarrow 2\angle OAQ={{50}^{\circ }}$(∵OA=OQ)

$\angle OAQ={{25}^{\circ }}$------(4)

From eq.(2) and (4)

$\angle OAQ=\angle AQB$

But these are alternate angles.

Therefore, AO||BQ

24. The given figure shows a circle with centre O such that chord RS is parallel to chord QT. angle $PRT={{20}^{\circ }}$and angle $POQ={{100}^{\circ }}$, Calculate :

(i). Angle QTR

(ii). Angle QRP

(iii). Angle QRS

(iv). Angle STR

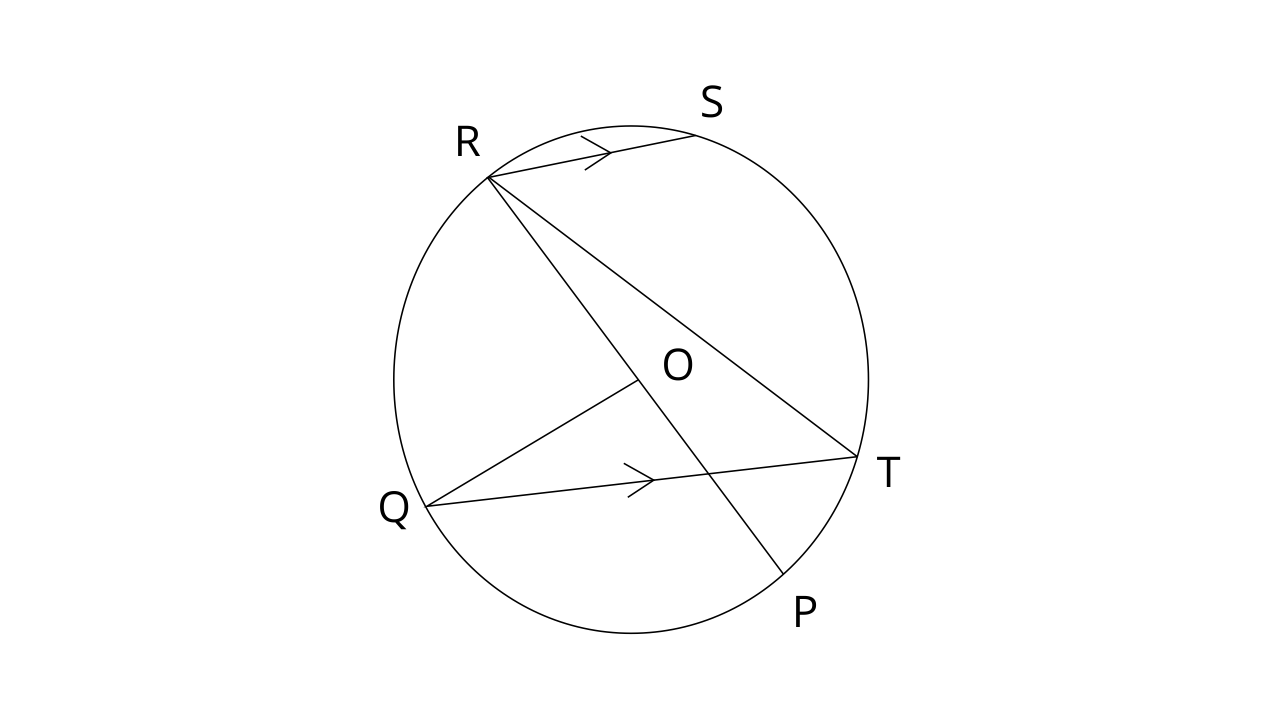
Ans: Join PQ, QR and ST

(i). Angle QTR

Ans: PR is a straight line.

$\angle POQ+\angle QOR={{180}^{\circ }}$

$\Rightarrow \angle QOR={{180}^{\circ }}-{{100}^{\circ }}={{80}^{\circ }}$

In a circle, Angle at the centre is doubled the angle at the circumference subtended by the same chord.

$\angle QOR=2\angle QTR$

$\Rightarrow \angle QTR=\frac{\angle QOR}{2}=\frac{{{80}^{\circ }}}{2}={{40}^{\circ }}$

(ii). Angle QRP

Ans: In a circle, Angle at the centre is doubled the angle at the circumference subtended by the same chord.

$\angle POQ=2\angle QRP$

$\angle QRP=\frac{\angle POQ}{2}=\frac{{{100}^{\circ }}}{2}={{50}^{\circ }}$

(iii). Angle QRS

Ans: Given, RS||QT

Therefore, $\angle SRT=\angle QTR$(Alternate angles)

$\angle SRT={{40}^{\circ }}$(from ii part $\angle QTR={{40}^{\circ }}$)

Now,  $\angle QRS=\angle QRP+\angle PRT+\angle SRT$

$\angle QRS={{50}^{\circ }}+{{20}^{\circ }}+{{40}^{\circ }}={{110}^{\circ }}$

(iv). Angle STR

Ans: QRST is a cyclic quadrilateral.

$\angle QRS+\angle QTS={{180}^{\circ }}$(Sum of opposite angles)

$\angle QRS+\angle QTR+\angle STR={{180}^{\circ }}$

$\Rightarrow {{110}^{\circ }}+{{40}^{\circ }}+\angle STR={{180}^{\circ }}$

$\Rightarrow \angle STR={{180}^{\circ }}-{{150}^{\circ }}={{30}^{\circ }}$

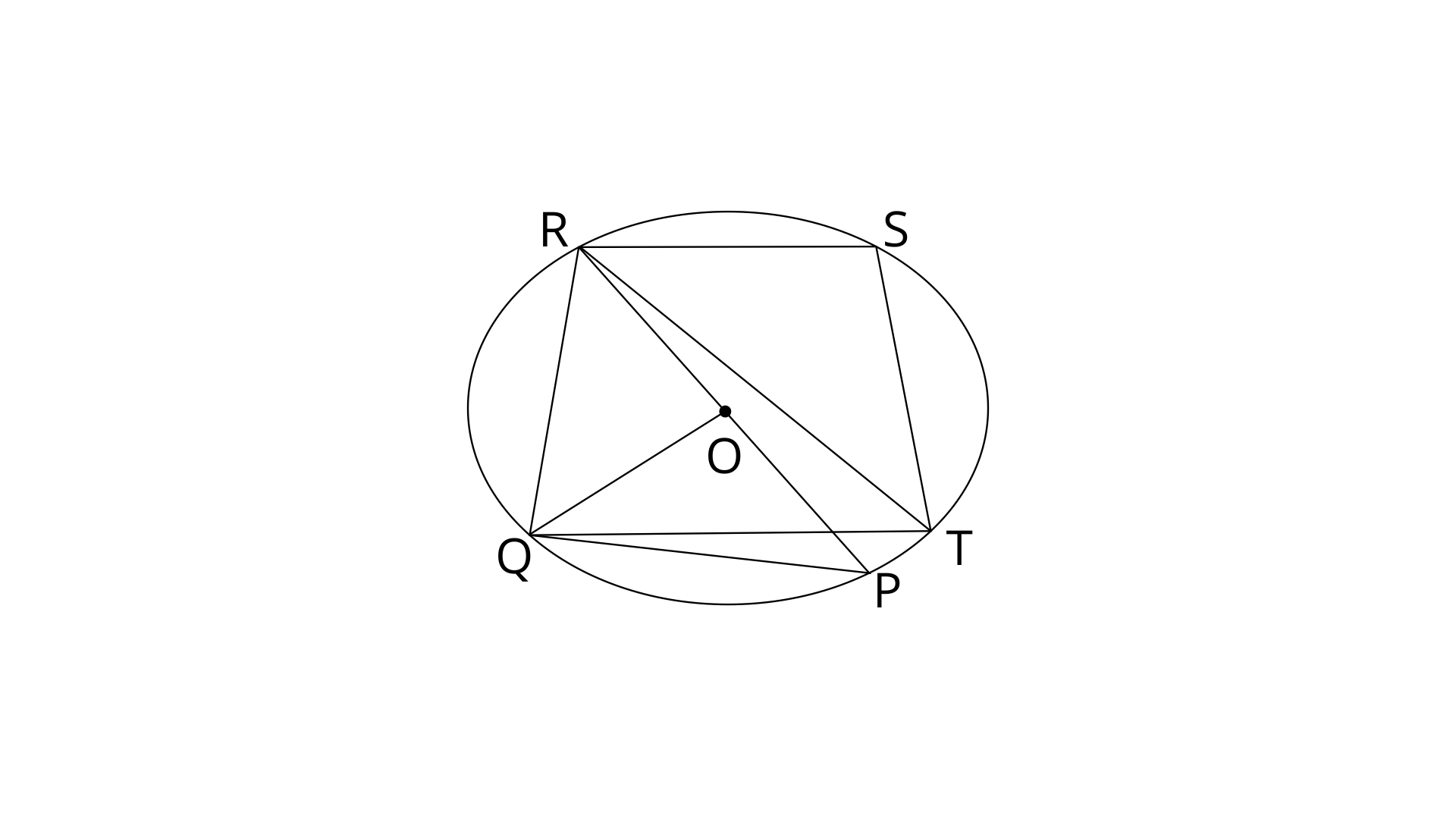
25. In the given figure, PAT is tangent to the circle with centre O, at point A on its circumference and is parallel to chord BC. If CDQ is a line segment, show that :

(i). $\angle BAP=\angle ADQ$

(ii). $\angle AOB=2\angle ADQ$

(iii). $\angle ADQ=\angle ADB.$

(i).$\angle BAP=\angle ADQ$

Ans: PAT||BC

Therefore, $\angle PAB=\angle ABC$ (Alternate angles)  -----------(1)

ABCD is a cyclic quadrilateral. By using exterior angle property.

Ext. $\angle ADQ=\angle ABC$ ----------(2)

From eq. (1) and (2)

$\angle PAB=\angle ADQ$

(ii). $\angle AOB=2\angle ADQ$

Ans: In a circle, Angle at the centre is doubled the angle at the circumference subtended  by the same chord.

$\angle AOB=2\angle ADB$

PAT is tangent and AB is chord.

Hence, $\angle PAB=\angle ADB$(Angles in alternate segments)

From (i) part, $\angle PAB=\angle ADQ$

$\Rightarrow \angle ADQ=\angle ADB$

Therefore, $\angle AOB=2\angle ADQ$

(iii). $\angle ADQ=\angle ADB$

Ans: ∵$\angle PAB=\angle ADB$(Angles in alternate segments)

$\angle PAB=\angle ADQ$ (From i part)

Therefore, $\angle ADQ=\angle ADB$

26. AB is a line segment and M is its mid-point. Three semi-circle are drawn with AM, MB and AB as diameters on the same side of the line AB. A circle with radius r unit is drawn so that it touches all the three semi-circles. Show that :$AB=6\times r$.

Ans: Join OP and OQ.

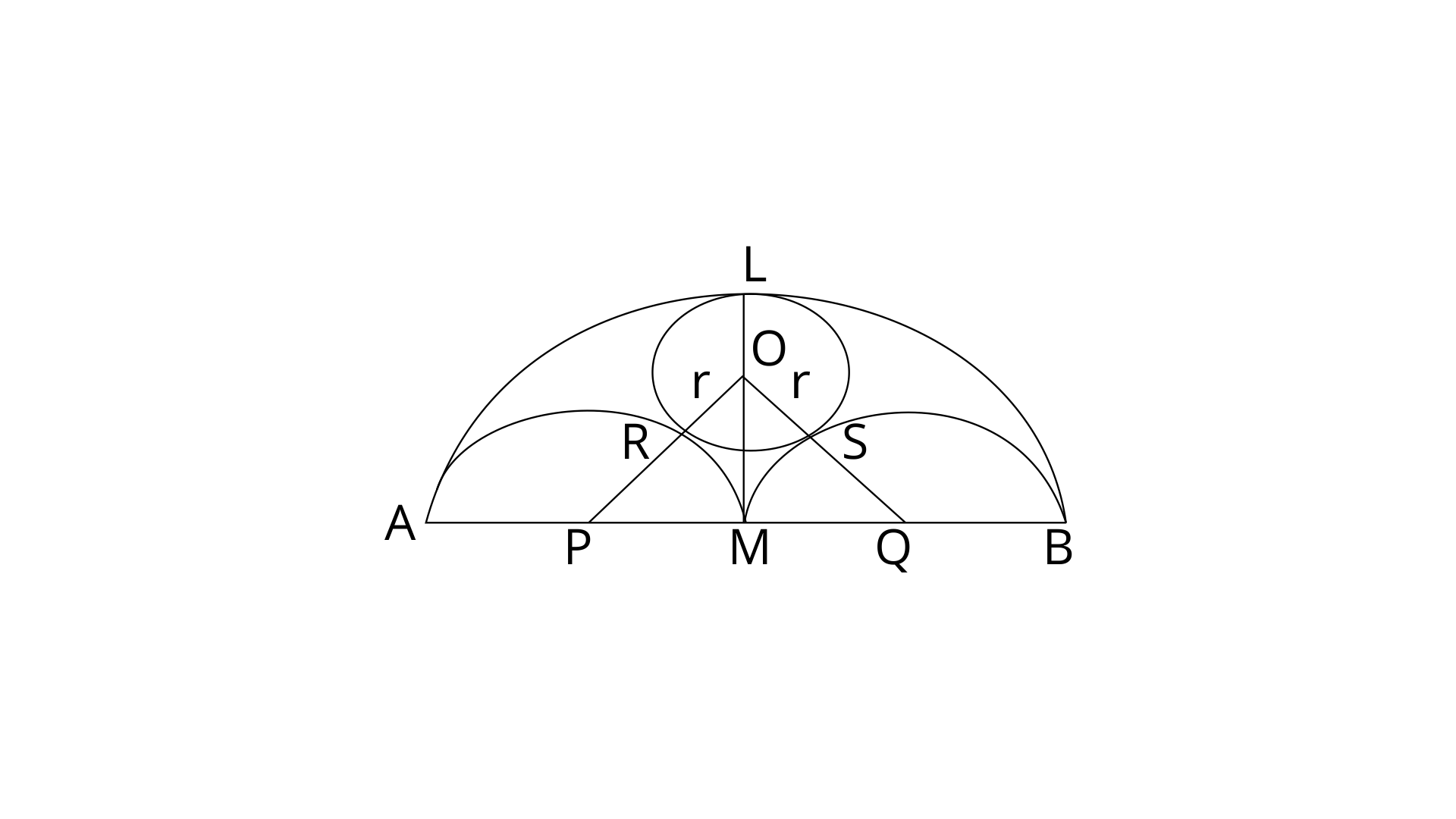
OR=OS=r

And, $AP=PM=MQ=QB=\frac{AB}{4}$

Now, $OP=r+PR=r+\frac{AB}{4}$ ($\because PR=PM=AP$radii of same circle)

Similarly, $OP=r+SQ=r+\frac{AB}{4}$

Now, $OM=LM-OL=\frac{AB}{2}-r$

In $\angle OPM$by using pythagoras theorem,

$O{{P}^{2}}=P{{M}^{2}}+O{{M}^{2}}$

$\Rightarrow {{\left( r+{{\frac{AB}{4}}_{{}}} \right)}^{2}}={{\left( \frac{AB}{4} \right)}^{2}}+{{\left( \frac{AB}{2}-r \right)}^{2}}$

$\Rightarrow 

{{r}^{2}}+\frac{A{{B}^{2}}}{16}+\frac{rAB}{2}=\frac{A{{B}^{2}}}{16}+\frac{A{{B}^{2}}}{4}+{{r}^{2}}-rAB$

$\Rightarrow \frac{rAB}{2}=\frac{A{{B}^{2}}}{4}-rAB$

$\Rightarrow \frac{A{{B}^{2}}}{4}=\frac{rAB}{2}+rAB$

$\Rightarrow \frac{A{{B}^{2}}}{4}=\frac{3rAB}{2}$

$\Rightarrow \frac{AB}{2}=3r$

$\Rightarrow AB=6r$

Hence, $AB=6\times r$

27. TA and TB are tangents to a circle with centre O from an external point T. OT intersects the circle at point P. Prove that AP bisects the angle TAB.

Ans: Join PB.

In $\Delta TAP$and $\Delta TBP$

TA=TB (Given)

PT=PT (common sides)

$\angle ATP=\angle BTP$(OT inclined equally with AT and BT)

$\Delta ATP\Delta BTP$(SAS congruence criterion)

Therefore, $\angle TAP=\angle TBP$(C.P.C.T) ------(1)

And, $\angle TBP=\angle BAP$(Angles in alternate segments) ------(2)

From eq. (1) and (2)

$\angle TAP=\angle BAP$

Thus AP bisects the angle TAB.

28. Two circles intersect in points P and Q. A secant passing through P intersects the circles in A and B respectively. Tangents to the circles at A and B intersect at T. Prove that A, Q, B and T lie on a circle. 

Ans: Join PQ.

TA is a tangent and AP is a chord.

$\therefore \angle TAP=\angle AQP$(Angles in alternate segments) ---------(1)

Similarly, $\angle TBP=\angle PQB$(Angles in alternate segments) -------(2)

On adding eq.(1) and (2)

$\angle TAP+\angle TBP=\angle AQP+\angle PQB$

$\Rightarrow \angle TAP+\angle TBP=\angle AQB$

Now by using angle sum property in $\Delta TAB$

$\angle TAP+\angle TBP+\angle ATB={{180}^{\circ }}$

$\angle ATB+\angle AQB={{180}^{\circ }}$

The sum of opposite angles is ${{180}^{\circ }}$. Therefore, ATBQ is a cyclic quadrilateral.

Thus A, Q, B and T lie on a circle.

29. Prove that any four vertices of a regular pentagon are concyclic (lie on the same circle).

Ans: 

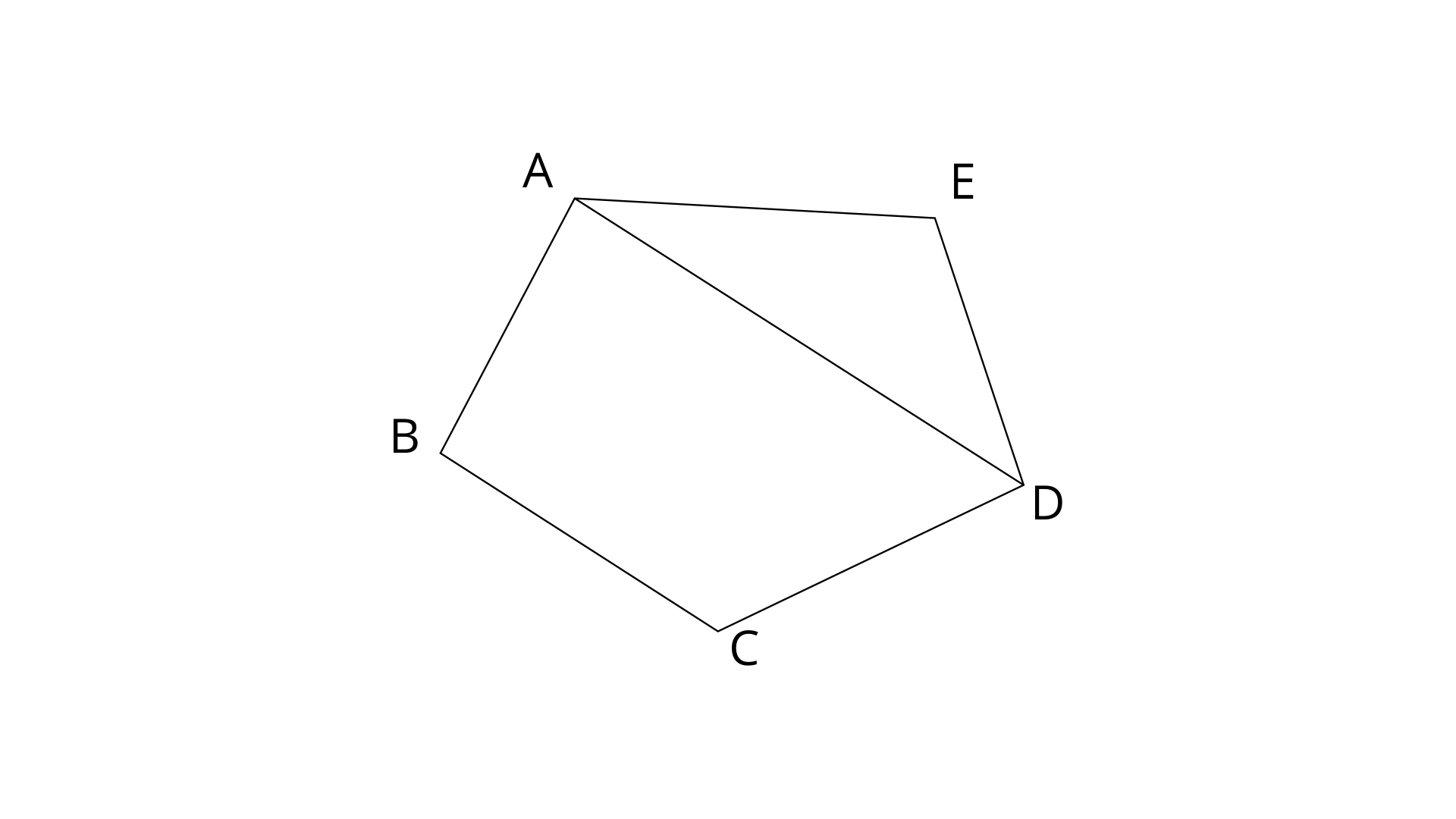
ABCDE is a regular pentagon.

Therefore, $\angle ABC=\angle BCD=\angle CDE=\angle DEA=\angle BAE=\frac{n-2}{n}\left( {{180}^{\circ }} \right)=\frac{3}{2}\times {{180}^{\circ }}={{108}^{\circ }}$

In $\Delta AED$

AE=ED (Sides of a regular pentagon)

$\therefore \angle EAD=\angle EDA$

By using angle sum property in $\Delta EAD$

$\angle EAD+\angle EDA+\angle AED={{180}^{\circ }}$

$\Rightarrow 2\angle EAD+{{108}^{\circ }}={{180}^{\circ }}$

$\Rightarrow 2\angle EAD={{72}^{\circ }}$

$\Rightarrow \angle EAD={{36}^{\circ }}$

Since, $\angle EAD=\angle EDA$

$\therefore \angle EDA={{36}^{\circ }}$

Now, $\angle BAD=\angle BAE-\angle DAE={{108}^{\circ }}-{{36}^{\circ }}={{72}^{\circ }}$

In quadrilateral ABCD,

$\angle BAD+\angle BCD={{108}^{\circ }}+{{72}^{\circ }}={{180}^{\circ }}$

The sum of opposite angles is ${{180}^{\circ }}$. Therefore ABCD is cyclic quadrilateral.

30. Chords AB and CD of a circle when extended meet at point X. Given AB=4cm, BX=6cm and XD=5cm, calculate the length of the CD. 

Ans: 

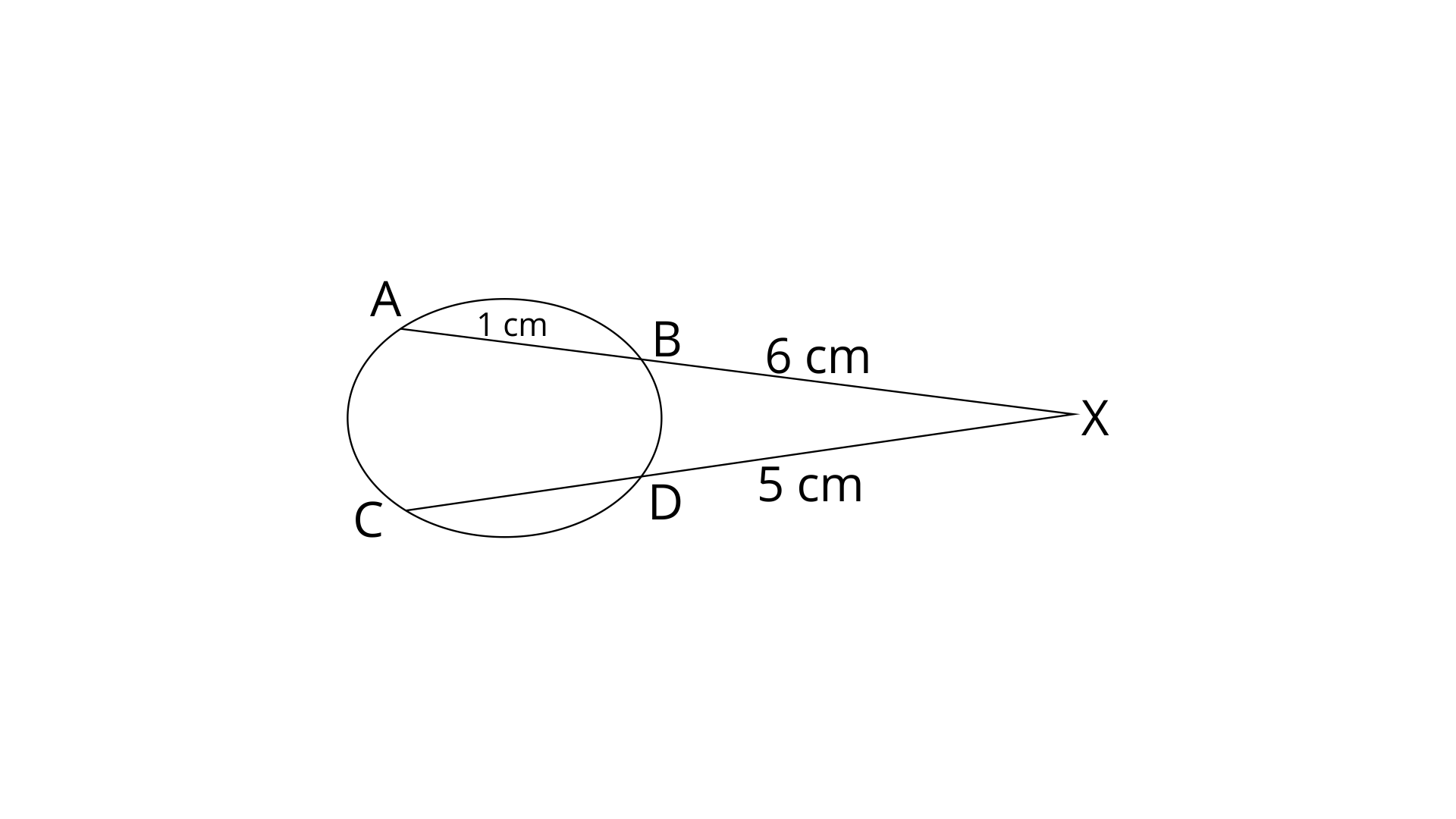
We know that, 

$XA.~XB=XC.~XD$

$\Rightarrow \left( XB+AB \right).~XB=\left( CD+XD \right).XD$

$\Rightarrow \left( 6+4 \right).~6=\left( CD+5 \right).5$

$\Rightarrow 60=5.\left( CD+5 \right)$

$\Rightarrow 12=\left( CD+5 \right)$

$\Rightarrow CD=7cm$

Therefore the length of the CD is 7cm.

31. In the given figure, find TP if AT=16cm and AB=12cm.

Ans: TP is tangent and ABT is secant.

$T{{P}^{2}}=TA\times TB$

$\Rightarrow T{{P}^{2}}=16\left( 16-12 \right)$ (∵TB=TA-AB)

$\Rightarrow T{{P}^{2}}=16\times 4=64$

$\Rightarrow T{{P}^{2}}={{\left( 8 \right)}^{2}}$

$\Rightarrow TP=8cm$

Therefore, TP=8cm.

32. In the following figure, a circle is inscribed in the quadrilateral ABCD.

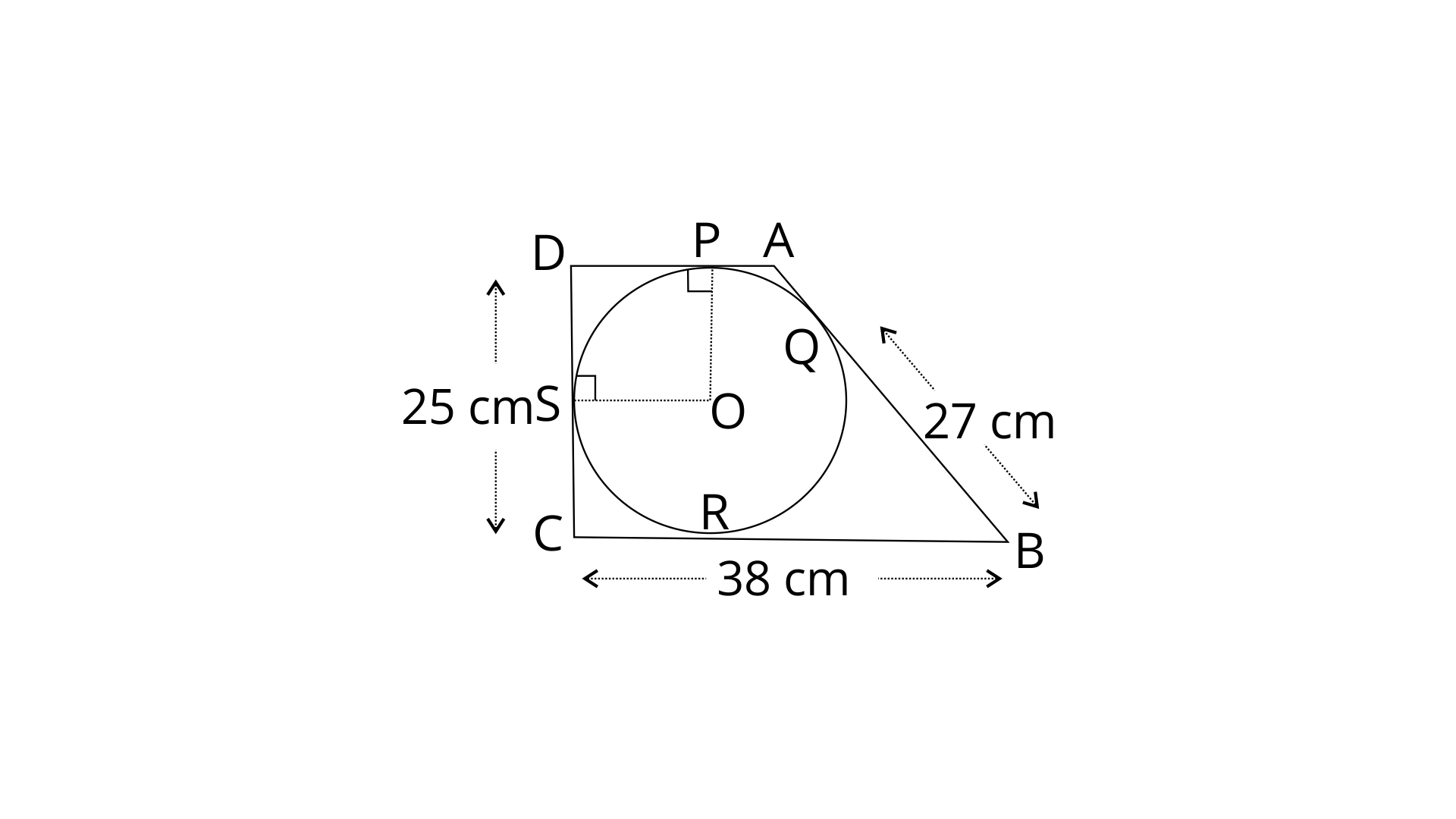
If BC=38cm, QB=27cm, DC=25cm and that AD is perpendicular to DC, find the radius of the circle.

Ans: From figure, BQ=BR (tangents to the circle from external point)

Since, $BR=BQ=27cm$

∴ CR=BC-BR=38-27=11cm

Again, CR=CS=11cm (tangents to the circle from external point)

Since, DC=25cm

∴ DS=DC-CS=25-11=14cm

Similarly, DP=DS=14cm (tangents to the circle from external point)

Now, In quadrilateral DSOP,

$\angle PDS={{90}^{\circ }}$

$\angle OPD={{90}^{\circ }}$(Radius is perpendicular to tangent through the point of contact)

Therefore, DSOP is a parallelogram

Thus OP||DS and DP||OS

Now, OP=OS (Radii of same circle)

Therefore, OPDS is a square.

So, DS=OP=14cm.

Hence the radius of the circle is 14cm.

33. In the given figure, XY is the diameter of the circle and PQ is a tangent to the circle Y.

If $\angle AXB={{50}^{\circ }}$and $\angle ABX={{70}^{\circ }}$, find $\angle BAY$and $\angle APY$.

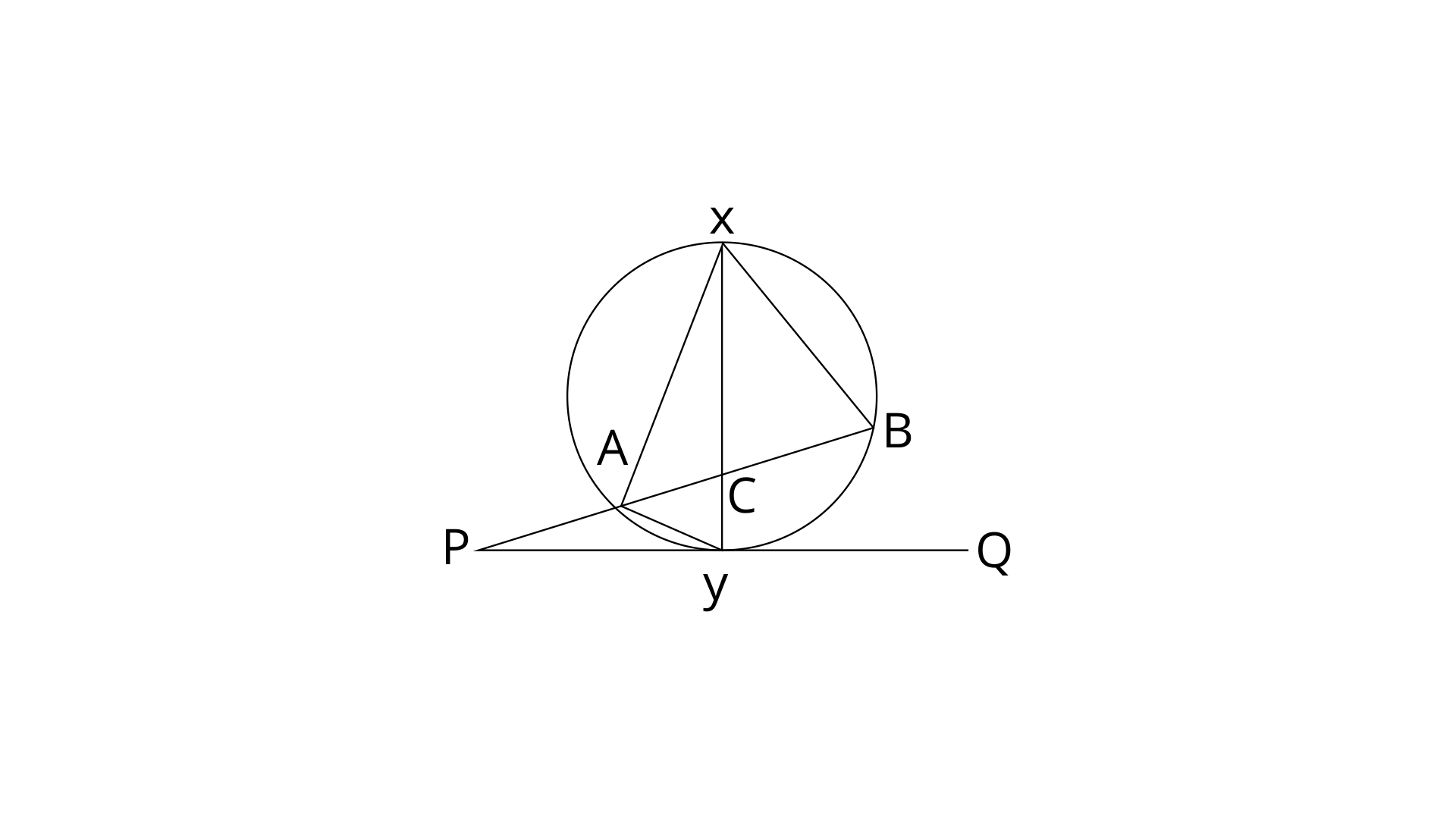
Ans: By using angle sum property in $\Delta AXB$

$\angle AXB+\angle XAB+\angle XBA={{180}^{\circ }}$

$\Rightarrow \angle XAB={{180}^{\circ }}-{{50}^{\circ }}-{{70}^{\circ }}={{60}^{\circ }}$

Angle at the circumference subtended by the diameter is the right angle.

Therefore, $\angle XAY={{90}^{\circ }}$

Since, $\angle XAY=\angle XAB+\angle BAY$

$\Rightarrow \angle XAB+\angle BAY={{90}^{\circ }}$

$\Rightarrow \angle BAY={{90}^{\circ }}-{{60}^{\circ }}={{30}^{\circ }}$

Angles subtended by the same chord on circumference are equal.

Therefore, $\angle BXY=\angle BAY={{30}^{\circ }}$ (Subtended by same chord BY)

By using external angle property, 

$\angle ACX=\angle BXC+\angle CBX$ 

$\Rightarrow \angle ACX=\angle BXY+\angle ABX={{30}^{\circ }}+{{70}^{\circ }}={{100}^{\circ }}$ [$\angle BXC=\angle BXY$and $\angle CBX=\angle ABX$angles b/w same line segments are equal]

$\Rightarrow \angle ACX={{100}^{\circ }}$

And, $\angle XYP={{90}^{\circ }}$(Diameter⊥tangent)

By using exterior angle property,

$\angle ACX=\angle CPY+\angle CYP$

$\Rightarrow {{100}^{\circ }}=\angle CPY+{{90}^{\circ }}$

$\Rightarrow \angle CPY={{100}^{\circ }}-{{90}^{\circ }}={{10}^{\circ }}$

$\angle CPY=\angle APY$(Angles b/w same line segments)

Therefore, $\angle APY={{10}^{\circ }}$.

34. In the given figure, QAP is the tangent at point A and PBD is a straight line.

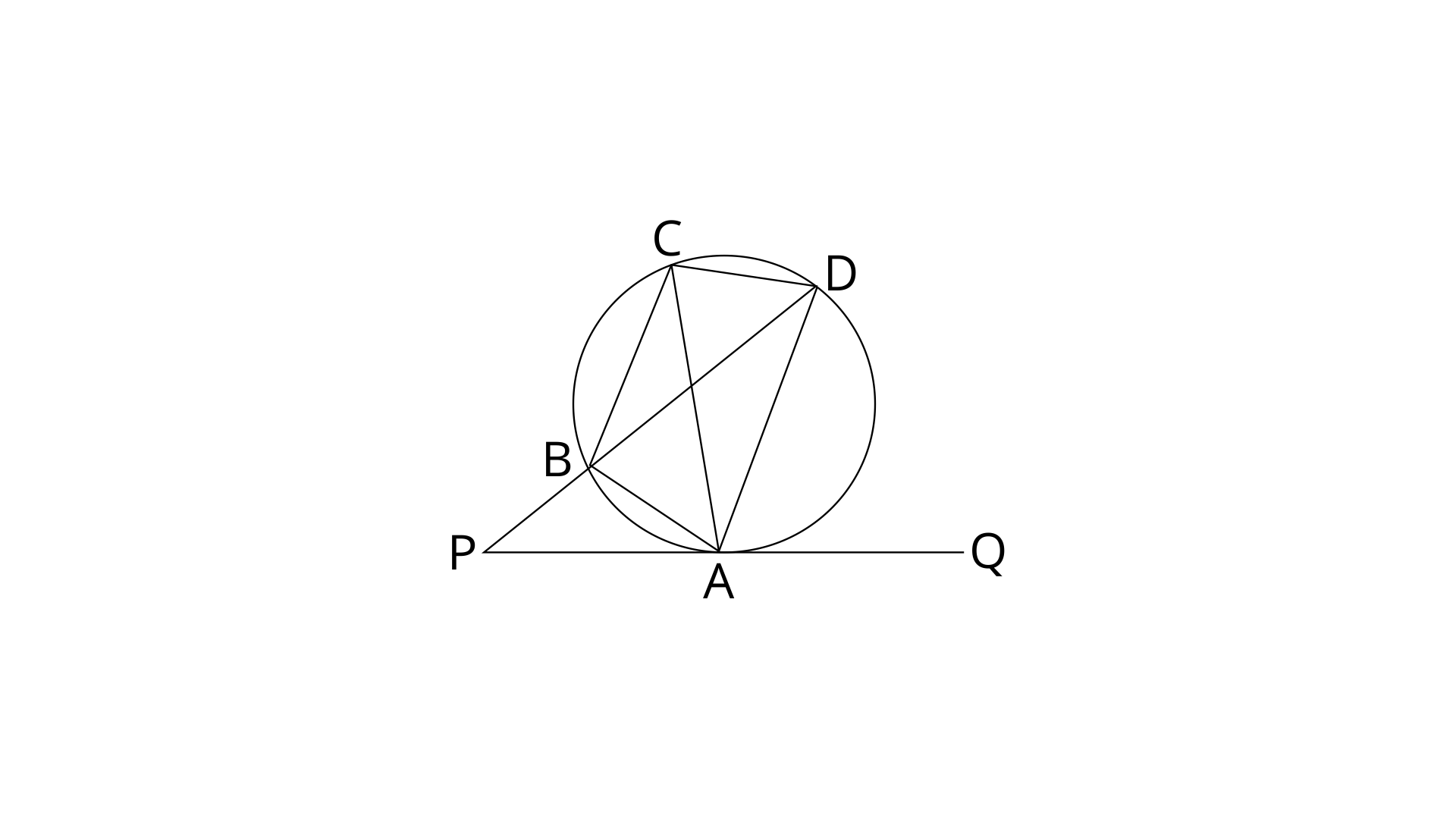
If $\angle ACB={{36}^{\circ }}$and $\angle APB={{42}^{\circ }}$, find :

(i). $\angle BAP$

Ans: PAQ is a tangent and AB is a chord of the circle.

Therefore, $\angle BAP=\angle ACB={{36}^{\circ }}$(Angles in alternate segments)

Thus the value of $\angle BAP$is ${{36}^{\circ }}$.

(ii). $\angle ABD$

Ans: By using exterior angle property in $\Delta APB$

ext.$\angle ABD=\angle BAP+\angle APB$

$\Rightarrow \angle ABD={{36}^{\circ }}+{{42}^{\circ }}={{78}^{\circ }}$

Thus the value of $\angle ABD$is ${{78}^{\circ }}$

(iii). $\angle QAD$

Ans: PAQ is a straight line and AD is the chord of the circle.

$\angle QAD=\angle ABD={{78}^{\circ }}$(Angles in alternate segments)

Thus the value of $\angle QAD$is ${{78}^{\circ }}$

(iv). $\angle BCD$

Ans: $\angle ACD=\angle ABD={{78}^{\circ }}$(Angles subtended by same chord AD)

Now, $\angle BCD=\angle ACB+\angle ACD={{36}^{\circ }}+{{78}^{\circ }}={{114}^{\circ }}$

Thus the value of $\angle BCD$is ${{114}^{\circ }}$

35. In the given figure, AB is the diameter. The tangent at C meets AB produced at Q.

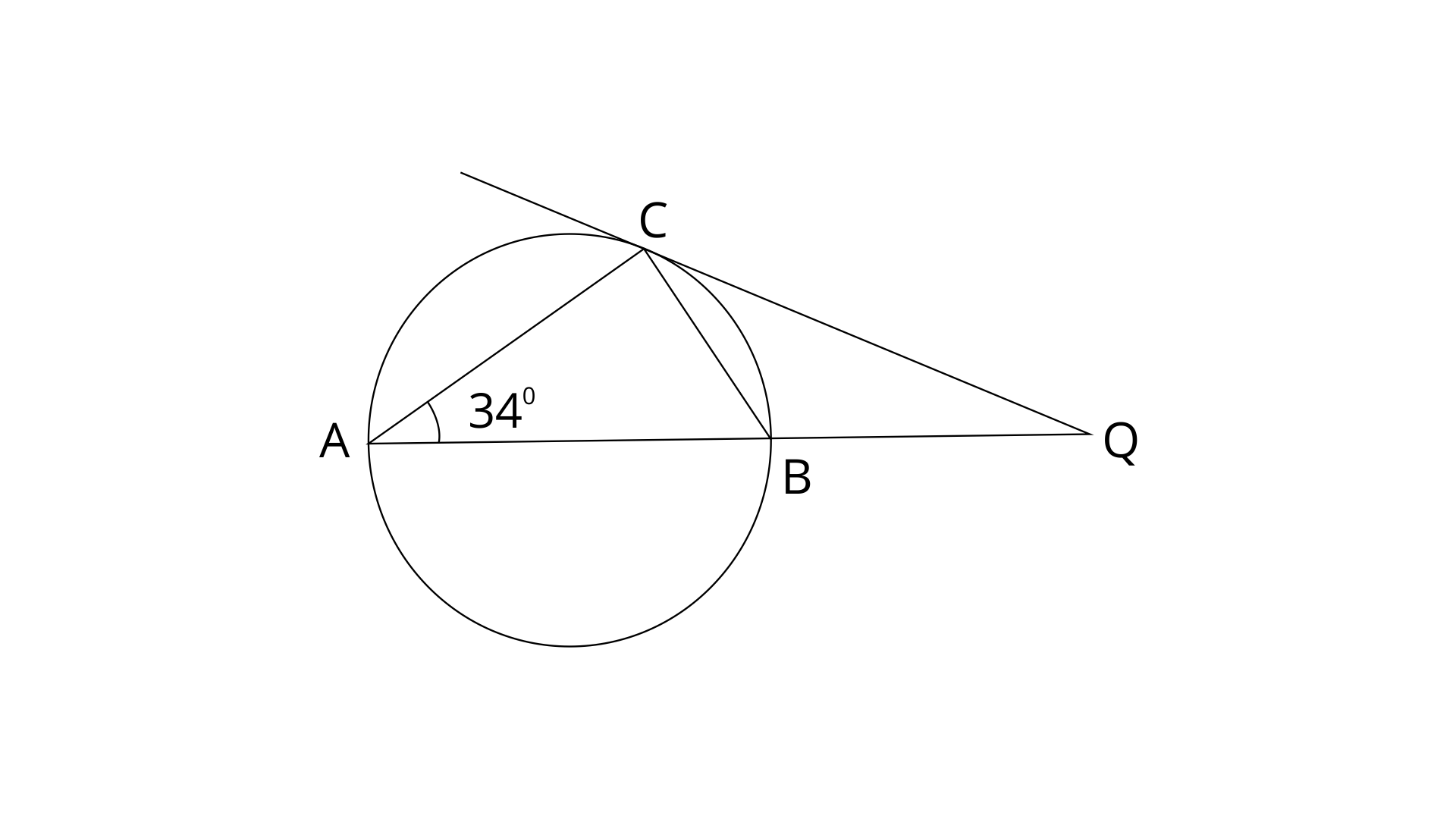
If $\angle CAB={{34}^{\circ }}$, find :

(i).$\angle CBA$

Ans: In a circle, Angle at the circumference subtended by the diameter is right angle. Here angle $\angle ACB~$is subtended by the diameter of the circle. Hence, $\angle ACB={{90}^{\circ }}$

Now by using angle sum property in $\Delta ABC$

$\angle ACB+\angle CBA+\angle BAC={{180}^{\circ }}$

$\angle CBA={{180}^{\circ }}-{{34}^{\circ }}-{{90}^{\circ }}={{56}^{\circ }}$

(ii). $\angle CQB$

Ans: QC is a tangent and BC is a chord.

Therefore, $\angle QCB=\angle CAB$(Angles in alternate segments)

$\Rightarrow \angle QCB={{34}^{\circ }}$

ABC is a straight line, Hence $\angle CBA+\angle CBQ={{180}^{\circ }}$(linear pairs)

$\Rightarrow \angle CBQ={{180}^{\circ }}-{{56}^{\circ }}={{124}^{\circ }}$(from i part $\angle CBA={{56}^{\circ }}$)

By using angle sum property in $\Delta BCQ$,

$\angle CQB+\angle QCB+\angle CBQ={{180}^{\circ }}$

$\angle CQB={{180}^{\circ }}-{{124}^{\circ }}-{{34}^{\circ }}={{22}^{\circ }}$

36. In the given figure, O is the centre of the circle. The tangents at B and D intersect at B and D intersect each other at point P.

If AB is parallel to CD and $\angle ABC={{55}^{\circ }}$, find:

(i). $\angle BOD$

Ans: Since, AB||CD$\Rightarrow \angle ABC=\angle BCD={{55}^{\circ }}$(Alternate angles)

In a circle, Angle at the centre is twice the angle at the circumference subtended by the same chord. 

Therefore, $\angle BOD=2\angle BCD=2\times {{55}^{\circ }}={{110}^{\circ }}$(Angles subtended by chord BD)

(ii). $\angle BPD$

Ans: Here PB and PD are the tangents drawn from point P and OB and OD are radius which are perpendicular to the tangents. Hence, $\angle OBP=\angle ODP={{90}^{\circ }}$

BPDO is a quadrilateral, 

Therefore, $\angle OBP+\angle ODP+\angle BOD+\angle BPD={{360}^{\circ }}$

$\angle BPD={{360}^{\circ }}-{{180}^{\circ }}-{{110}^{\circ }}={{70}^{\circ }}$

37. In the following figure, PQ=QR, $\angle RQP={{68}^{\circ }}$ , PC and CQ are tangents to the circle with centre O.

Calculate the values of :

(i).$\angle \text{QOP}$

Ans: Since, PQ=PR

$\Rightarrow \angle PRQ=\angle QPR$(Opposite angles of equal sides)

By using angle sum property in $\Delta PQR$

$\angle PRQ+\angle QPR+\angle PQR={{180}^{\circ }}$

$\Rightarrow 2\angle PRQ={{180}^{\circ }}-{{68}^{\circ }}={{112}^{\circ }}$

$\Rightarrow \angle PRQ={{56}^{\circ }}$

In a circle, Angle at centre is doubled the angle at the circumference subtended by the same chord.

Now, $\angle QOP=2.\angle PRQ=2\times {{56}^{\circ }}={{112}^{\circ }}$

(ii). $\angle QCP$

Ans: PC is a tangent and PQ is a chord.

Therefore, $\angle PQC=\angle PRQ={{56}^{\circ }}$(Angles in alternate segments)

Similarly, $\angle QPC=\angle PRQ={{56}^{\circ }}$(Angles in alternate segments)

By using angle sum property in $\Delta QPC$,

$\angle QCP+\angle QPC+\angle PQC={{180}^{\circ }}$

$\Rightarrow \angle QCP={{180}^{\circ }}-{{56}^{\circ }}-{{56}^{\circ }}={{68}^{\circ }}$

38. In two circles, prove that all the chords of the outer circle, which touch the inner circle, are of equal length.

Ans:

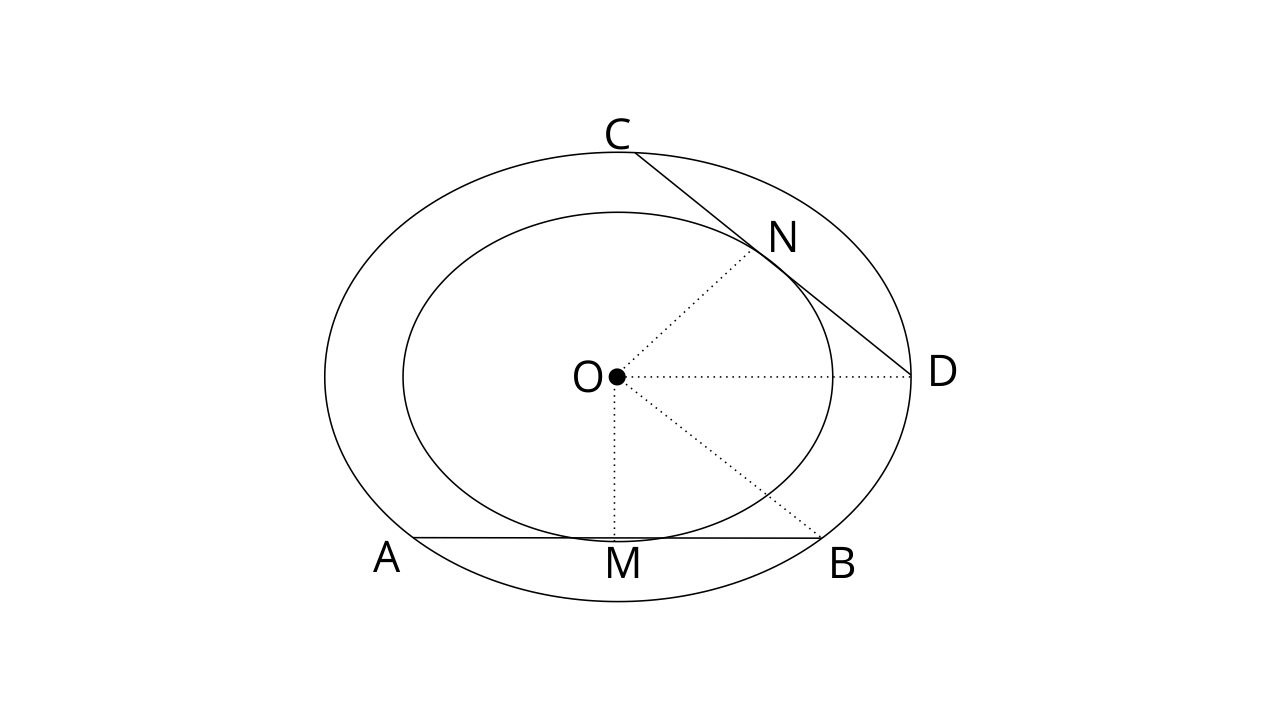
Consider the following two concentric circles with centres o.

Let AB and CD are two chords of the outer circle and touch the inner circle at point M and N respectively.

We have to prove the chords are equal, that means AB=CD.

We join points OM, OB, ON, OD.

Here OM and ON are the radius of the inner circle. Let the radius is r.

So, OM=ON=r

Similarly , Let R is the radius of the outer circle. OB=OD=R

AB is tangent to the inner circle and OM is radius and we know that the radius is perpendicular to the tangent.

$OM\bot AB$

$\Rightarrow BM=\frac{1}{2}\times AB$

$\Rightarrow AB=2BM$

Similarly, $ON\bot CD$

$\Rightarrow CD=2DN$

By using pythagoras theorem in $\Delta OBM$and$\Delta ODN$

$O{{B}^{2}}=O{{M}^{2}}+B{{M}^{2}}$

$\Rightarrow BM=\sqrt{{{R}^{2}}-{{r}^{2}}}$

$\Rightarrow AB=2BM=2\sqrt{{{R}^{2}}-{{r}^{2}}}$------------(1)

And, $O{{D}^{2}}=O{{N}^{2}}+N{{D}^{2}}$

$\Rightarrow N{{D}^{2}}=O{{D}^{2}}-O{{N}^{2}}$

$\Rightarrow ND=\sqrt{{{R}^{2}}-{{r}^{2}}}$

$\Rightarrow CD=2ND=2\sqrt{{{R}^{2}}-{{r}^{2}}}$----------(2)

From eq. (1) and (2)

AB=CD (Hence proved)

39. In the figure, given below, AC is a transverse common tangent to two circles with centres P and Q and of radii 6cm and 3cm respectively.

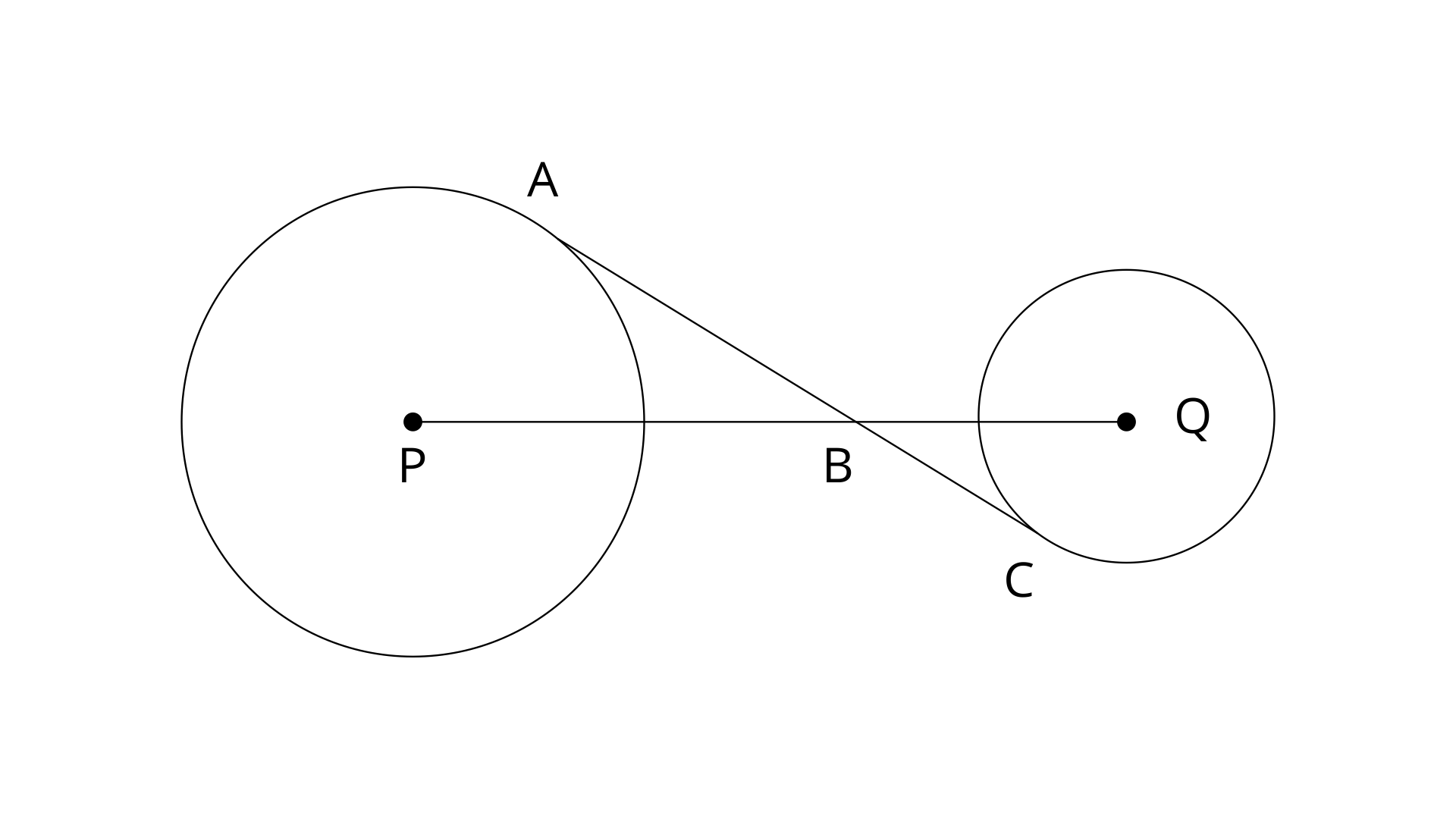
Given that AB=8cm, calculate PQ.

Ans:

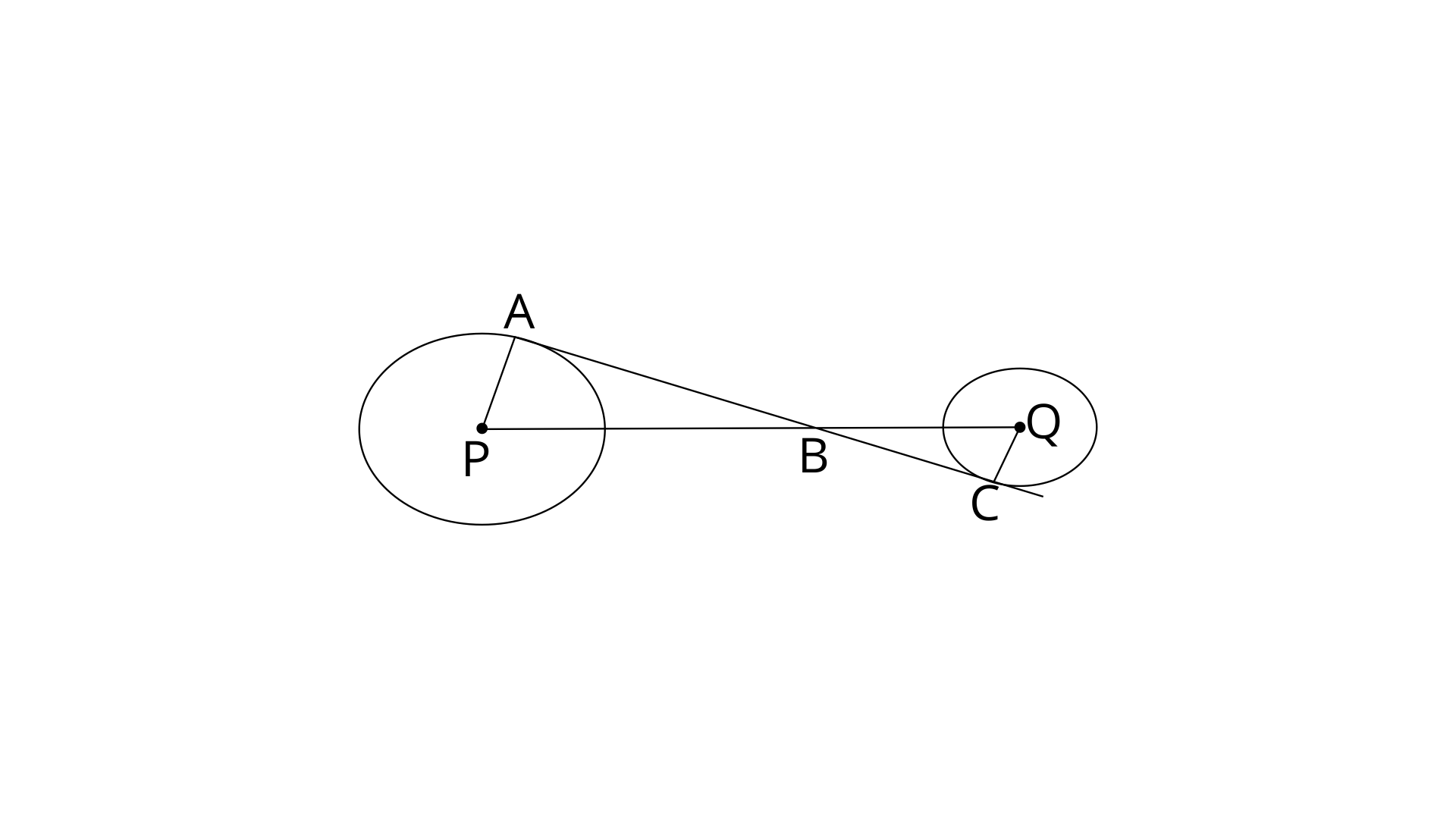
Since AC is tangent to the circle with centre P at point A.

Therefore, $\angle PAB={{90}^{\circ }}$

Similarly, $\angle QCB={{90}^{\circ }}$

In$\Delta PAB~and~\Delta BCQ$

$\angle PAB=\angle QCB={{90}^{\circ }}$

$\angle PBA=\angle QBC$(Vertically opposite angles)

Therefore, $\Delta PAB\sim \Delta BCQ$

$\frac{PA}{QC}=\frac{PB}{QB}$--------------(i)

By using pythagoras theorem in $\Delta PAB$and $\Delta BCQ$

$P{{B}^{2}}=A{{B}^{2}}+A{{P}^{2}}={{6}^{2}}+{{8}^{2}}$

$PB=\sqrt{{{8}^{2}}+{{6}^{2}}}=\sqrt{64+36}=10$cm -------------(ii)

From (i) and (ii)

$\frac{6}{3}=\frac{10}{QB}$

$QB=5cm$

Now, $PQ=PB+BQ=10+5=15cm$

40. In the figure, given below, O is the centre of the circumcircle of triangle XYZ.

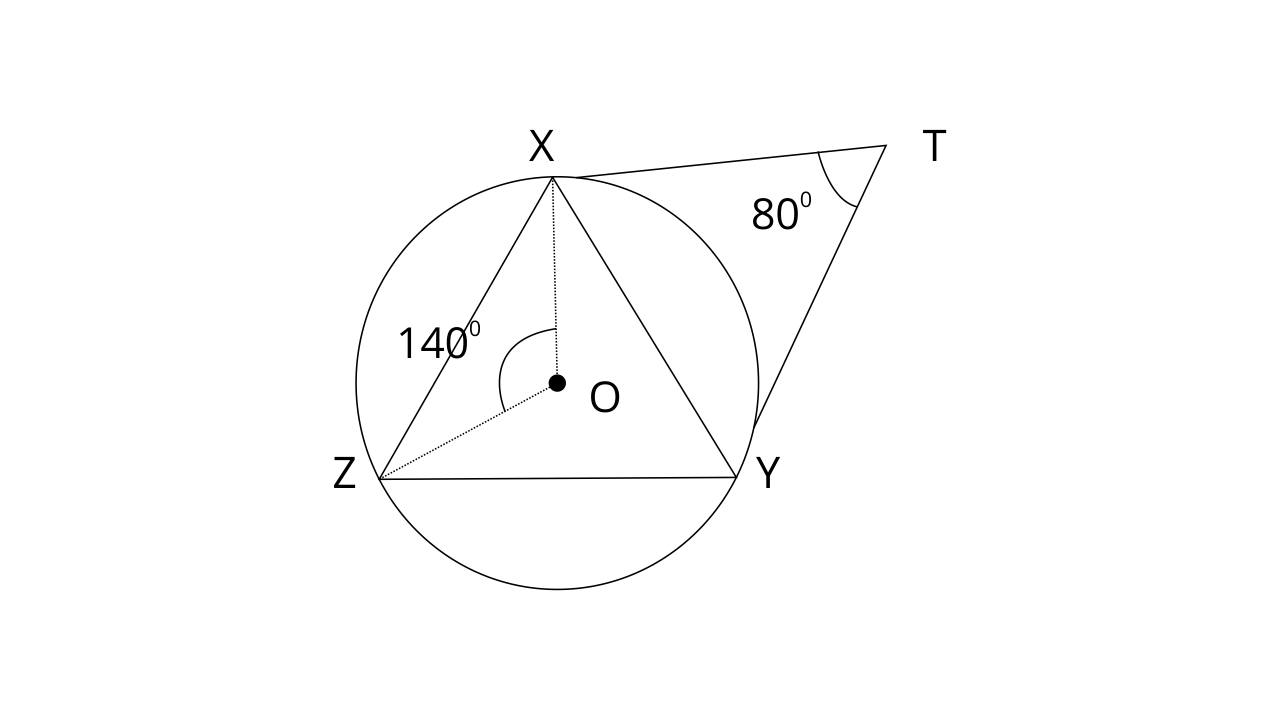
Tangents at X and Y intersect at point T. Given $\angle XTY={{80}^{\circ }}$and $\angle XOZ={{140}^{\circ }}$, calculate the value of $\angle ZXY$.

Ans: Join the points OY.

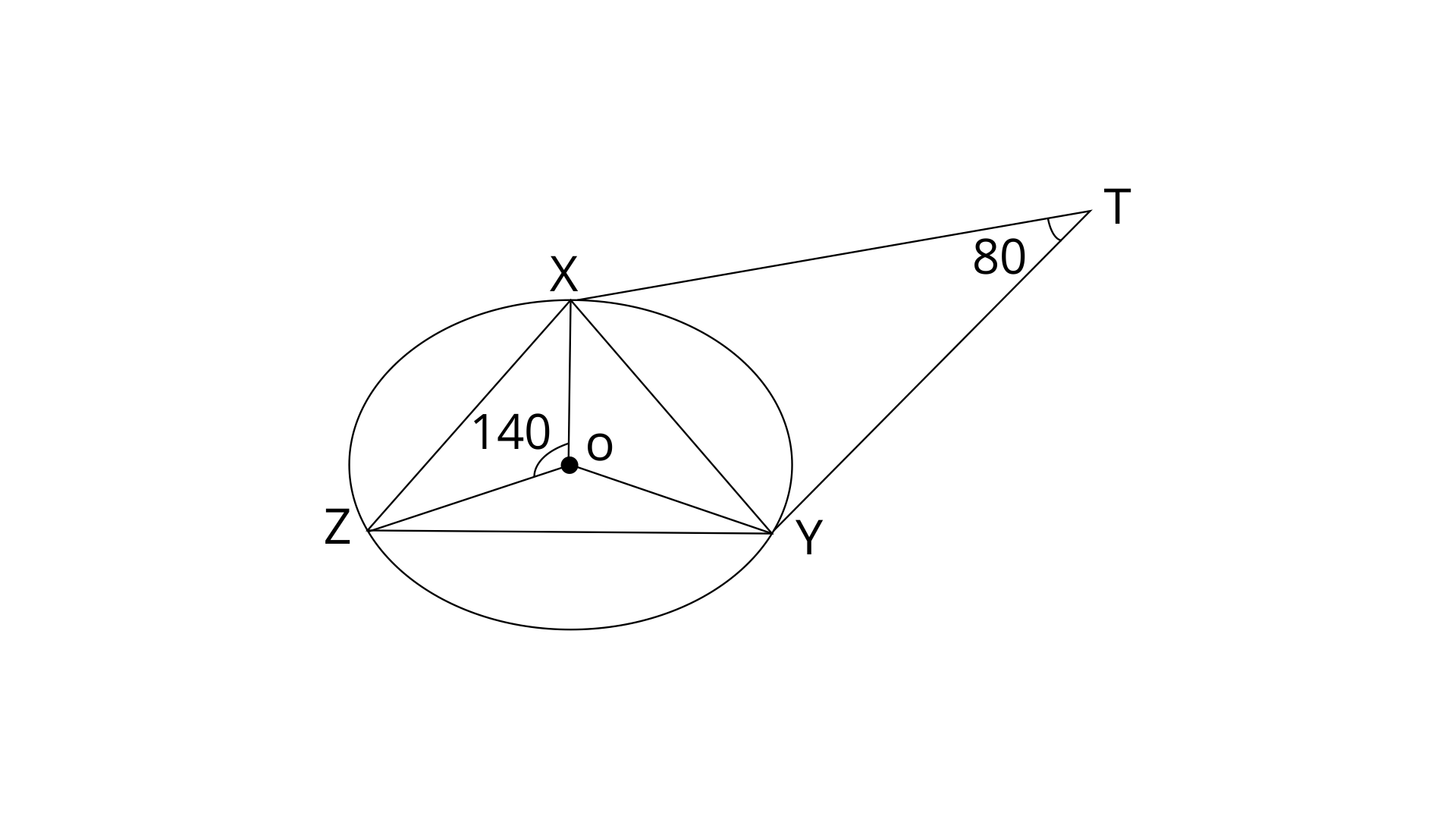
In the above figure, the circle is the circumcircle of triangle XYZ.

Given, $\angle XOZ={{140}^{\circ }}$

Tangents X and Y are intersecting at point T such that $\angle XTY={{80}^{\circ }}$

$\angle XOY+\angle XTY={{180}^{\circ }}$

$\angle XOY={{180}^{\circ }}-{{80}^{\circ }}={{100}^{\circ }}$

Now, $\angle XOY+\angle YOZ+\angle XOZ={{360}^{\circ }}$(Angles at the centre)

$\Rightarrow \angle YOZ={{360}^{\circ }}-{{140}^{\circ }}-{{100}^{\circ }}={{120}^{\circ }}$

In a circle, Angle at the centre is doubled the angle at the circumference subtended by the same chord.

Therefore, $\angle ZOY=2.\angle ZXY$

$\angle ZXY=\frac{\angle ZOY}{2}=\frac{{{120}^{\circ }}}{2}={{60}^{\circ }}$

41. In the given figure, AE and BC intersect each other at point D.

If $\angle CDE={{90}^{\circ }}$, AB=5cm, BD=4cm and CD=9cm, find AE.

Ans: Since ADB is a right angle triangle, 

By using pythagoras theorem.

$AD=\sqrt{A{{B}^{2}}-B{{D}^{2}}}$

$\Rightarrow AD=\sqrt{{{5}^{2}}-{{4}^{2}}}=3cm$

Since, two chords AE and BC intersect at point D.

$AD\times DE=BD\times CD$

$\Rightarrow 3\times DE=4\times 9$

$\Rightarrow DE=12$

Hence, $AE=AD+DE=3+12=15cm$

42. In the given circle with centre O, angle ABC=${{100}^{\circ }}$, $\angle ACD={{40}^{\circ }}$and CT is a tangent to the circle at C. Find $\angle ADC$and $\angle DCT$.

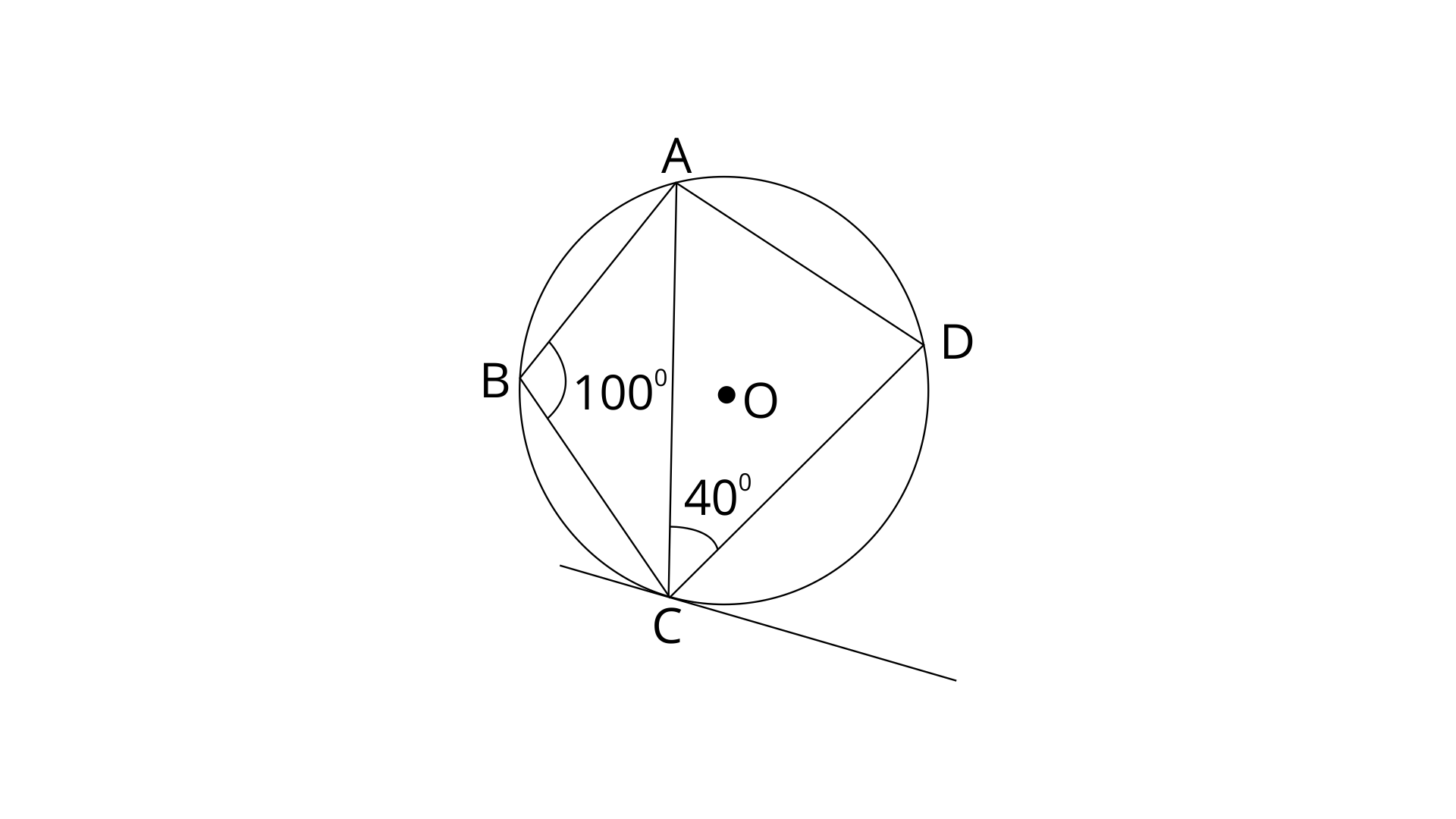
Ans: ABCD is a cyclic quadrilateral and in a cyclic quadrilateral the sum of opposite angles is ${{180}^{\circ }}$.

$\angle ABC+\angle ADC={{180}^{\circ }}$

$\angle ADC={{180}^{\circ }}-{{100}^{\circ }}={{80}^{\circ }}$

By using angle sum property in $\Delta ADC$

$\angle ADC+\angle ACD+\angle CAD={{180}^{\circ }}$

$\angle CAD={{180}^{\circ }}-{{80}^{\circ }}-{{40}^{\circ }}={{60}^{\circ }}$

CT is a tangent and AC is diameter,

Therefore, $\angle DCT=\angle CAD={{60}^{\circ }}$(Angles in alternate segments)

Thus the value of $\angle CAD={{60}^{\circ }}$and $\angle DCT={{60}^{\circ }}$.

43. In the figure given below, O is the centre of the circle and SP is a tangent. If $\angle SRT={{65}^{\circ }}$, find the values of x, y and z.

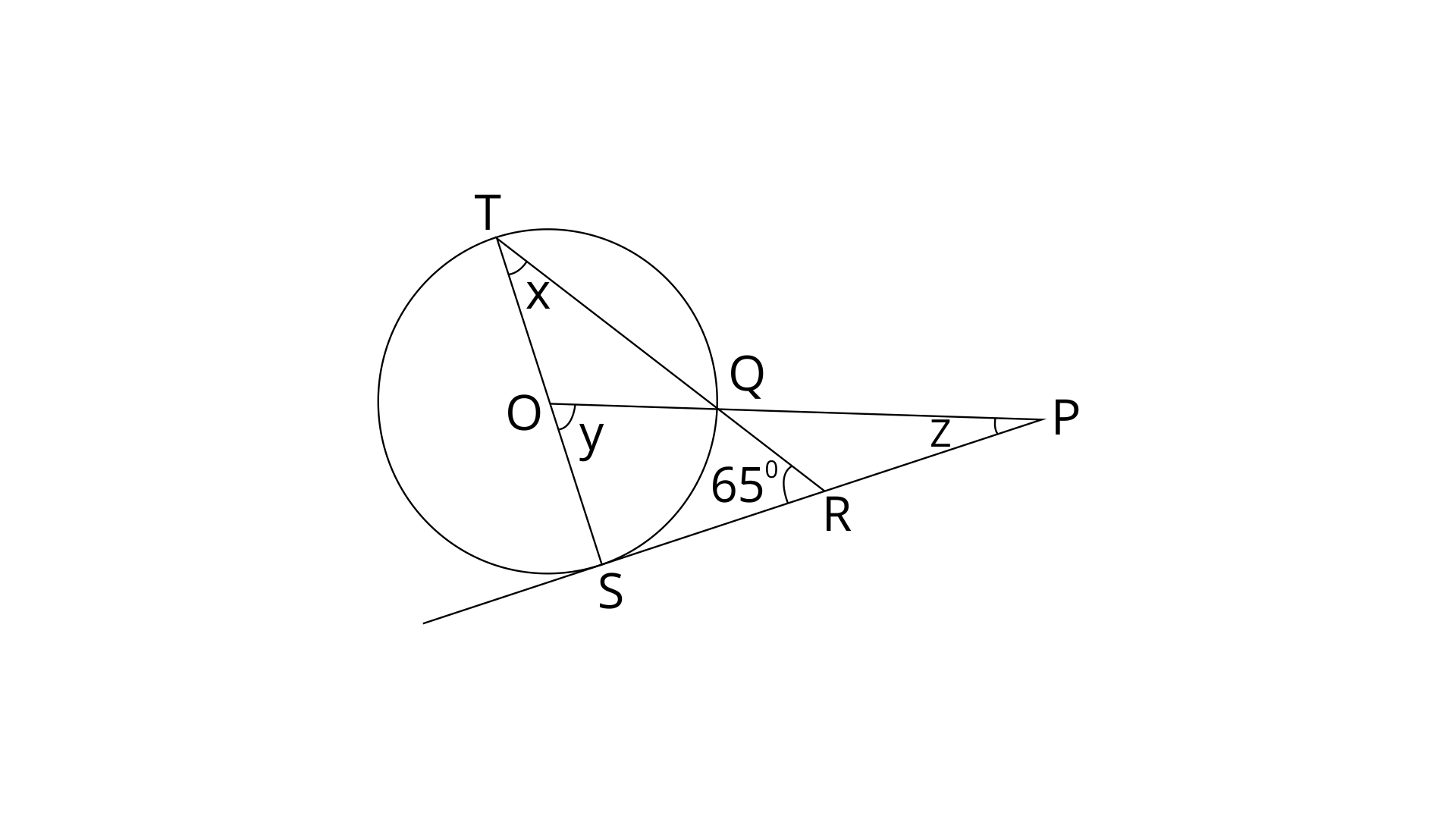
Ans: TS is diameter and PS is tangent.

Therefore, $\angle TSR={{90}^{\circ }}$

By using angle sum property in $\Delta TSR$,

$\angle TSR+\angle TRS+x={{180}^{\circ }}$

$x={{180}^{\circ }}-{{65}^{\circ }}-{{90}^{\circ }}={{25}^{\circ }}$

Angle at the centre is doubled the angle at the circumference subtended by the same Arc.

Therefore, y=2x

$y=2\times {{25}^{\circ }}={{50}^{\circ }}$

By using angle sum property in $\Delta POS$

${{90}^{\circ }}+y+z={{180}^{\circ }}$

$\Rightarrow z={{180}^{\circ }}-{{90}^{\circ }}-{{50}^{\circ '}}={{40}^{\circ }}$

Hence, $x={{25}^{\circ }},~y={{50}^{\circ }},~z={{40}^{\circ }}$.

ICSE Class 10 Mathematics Chapter 18 Selina Concise Solutions

Our Vedantu Selina ICSE Solutions work as a step-by-step guide and are essential when preparing for the examination. Selina ICSE Chapter 18 Tangents and Intersecting Chords Solutions for Class 10 help you to know concepts better so that you can study anytime, anywhere.Vedantu Selina Maths ICSE Solutions Class 10 are available free of cost and include all the chapters in detail. Our Vedantu Selina ICSE Maths solutions for Class 10 act as a free guide to jump ahead in your academic life.

In order to help most students prepare for this subject and score good marks in the Class 10 ICSE  board examinations, the Vedantu Selina solutions for Class 10 can be downloaded free of cost from our website. The pdf is also available for all chapters.

Vedantu Selina Solutions will help to get through the problems with proper and step wise understanding. 

Let us revise the basis of tangents and intersecting chords

Introduction to the Chapter

The chapter is all about the tangents and the intersecting chords. There are many theorems related to the topics which are specified in this chapter. Students can go through the topics and in case of doubts or difficulty, they can refer to Vedantu's NCERT solution where the solutions are exactly as per the chapter and with easy explanations of the topics.

The solutions are curated to boost confidence in their ICSE preparations. The solutions also help in the problem-solving skills of the students, which are essential from an examination standpoint. 

Some of the methods to be followed for the subject so that it becomes easy and you will have an organized study preparation are:

• Time Table

Students should prepare an organized study schedule. Before doing this they should know the topics to be studied for each day. Once the schedule is prepared it is very important to be followed regularly. A consistent study will always be helpful to learn in a better way.

• Dedicate Specific Time

Know your strengths and weaknesses. Analyze the area which needs to be given more time and dedicate the time accordingly. Maths is a challenging subject at the same time it can be easy if you practice. It is the easiest subject. The main element of getting the grip of the subject is understanding the concepts and practice.

• Revise

Revision is very important and it is a continuous process so has to be done every day and not just during the exams.

• Preparation of Notes

An organized note is very useful. This should contain the key points and the important topics. List of formulas and theorems that can give you a quick revision of the whole chapter.

• Mock Test and the Previous Year’s Question Paper

Once the completion of the syllabus or even the chapter, you can take a mock test to see the progress of the preparation level. This will help in rectifying the mistakes as well.

Once you have completed the whole syllabus you can solve the previous year’s question paper and you will get an idea of the exact exam pattern and the types of different questions. Practicing the question's paper will give the thorough practice of the whole syllabus and the various problems.

This will also help in time management and enhance the speed while solving the problem.

Definition of Tangent

If a segment touches a circle at only one point, it is said to as Tangent to the circle.

If the point is touching the circle at more than one point, then it cannot be a tangent. The tangents of a circle have the following properties.

• A tangent touches the boundary of the circle at only one point. 

• A tangent is perpendicular to the radius of the circle at the point of contact.

• Tangents cannot be drawn through a point which lies in the interior of the circle. 

• Two tangents can be drawn to the circle from the point outside a circle.

• Every point on the circle has one and only one tangent passing through it.

(Image will be Uploaded soon)

Length of Tangent Theorem

Only two tangents can be drawn to a circle from a point external to the circle. These two tangents will have the same length. Length of tangent to the circle from an external point can be calculated as given

l = \[\sqrt{d^2 - r^2}\]

In the above equation,

‘l’ is the length of the tangent 

d is the distance between the center of the circle and the external point from which the tangents are drawn

‘r’ is the radius of the circle

Key Features of Vedantu Solutions 

• Easy & reliable solutions

• Available free of cost

• Highlight important examination questions

• Step-by-step solutions for all questions

• Prepared by subject experts

• Designed according to the latest ICSE syllabus pattern

• Available online 24/7

• In-depth knowledge of the chapter is provided in easy language.

• Is based on the latest ICSE syllabus.

• Best study material to prepare for the Class 10 ICSE board exam.

• All the doubts that might arise while solving the exercise questions will vanish by going through these Vedantu Selina solutions

The Vedantu Selina Maths Solutions are designed to give maximum information to students preparing for the board examinations. By referring to Vedantu Solutions, students can grasp the concepts behind the solutions very quickly. This will not only aid students by giving them the confidence to answer all sorts of questions in the examinations but will also ensure that they learn the concepts in the long run as well. The Vedantu Selina Maths Chapter 18 Tangents and Intersecting Chords Solutions Class 10 focuses on answering the questions in the right way to encourage students to fetch full marks in the examination.

In ICSE Class 10, Maths is one of the challenging subjects of all as it includes all chapters. The chapters presented in the textbook are hard, and students often get confused about what is the right solution. Vedantu provides Selina Maths Chapter 18 Tangents and Intersecting Chords Solutions for ICSE Class 10 so that they can study well in their examination. All the Selina Maths Solutions are created by our experts who have vast academic experience.

Vedantu Selina Concise Maths Solutions for Class 10 is perfect for preparing for the board examinations. ICSE Class 10 syllabus is vast and needs concentrated efforts on the part of the students to face the examinations and shine out successful. The Vedantu Selina Concise Maths Class 10 ICSE solutions pdf includes details for all chapters from the subject. This will allow the students to examine and understand every concept even if practising for the first time.

Vedantu Selina ICSE Chapter 18 Tangents and Intersecting Chords Solutions for Class 10 all questions are answered and explained by expert teachers as per ICSE board guidelines. By referring these Vedantu Selina ICSE Solutions for Class 10 you can easily get high marks in ICSE Class 10 Board Examinations.