Courses

Courses for Kids

Free study material

Store

Talk to our experts

1800-120-456-456

Textbook Solutions

NCERT Exemplar for Class 11 Maths Chapter 7 - Permutations and Combinations (Book Solutions)

NCERT Exemplar for Class 11 Maths Chapter 7 - Permutations and Combinations (Book Solutions)

NCERT Exemplar for Class 11 Maths - Permutations and Combinations - Free PDF Download

Free PDF download of NCERT Exemplar for Class 11 Math Chapter 7 - Permutations and Combinations solved by expert Math teachers on Vedantu as per NCERT (CBSE) Book guidelines. All Chapter 7 - Permutations and Combinations exercise questions with solutions to help you to revise the complete syllabus and score more marks in your examinations.

Looking for study material like NCERT Exemplar for Class 11 Maths - Permutations and Combinations? Then trust Vedantu and the outcomes will please you. Both easy and hard topics are covered with ease and your understanding of the subject and the concepts will be stronger with every passing day. This chapter is quite complex, Vedantu's experts organize the entire set of questions in an advanced manner.

Popular Vedantu Learning Centres Near You

Sharjah, Sharjah

King Abdul Aziz St - Al Mahatta - Al Qasimia - Sharjah - United Arab Emirates

Visit Centre

Abu Dhabi, Abu-Dhabi

Mohammed Al Otaiba Tower - 1401, 14th Floor - opposite to Nissan Showroom West Zone building - Al Danah - Zone 1 - Abu Dhabi - United Arab Emirates

Visit Centre

22 No Phatak, Patiala

#2, Guhman Road, Near Punjabi Bagh, 22 No Phatak-Patiala

Visit Centre

Chhoti Baradari, Patiala

Vedantu Learning Centre, SCO-144 -145, 1st & 2nd Floor, Chotti Baradari Scheme Improvement Trust, Patiala-147001

Visit Centre

Janakpuri, Delhi

Vedantu Learning Centre, A-1/173A, Najafgarh Road, Opposite Metro Pillar 613, Block A1, Janakpuri, New Delhi 110058

Visit Centre

Tagore School, Gudha-Gorji

Tagore Public School Todi, Gudha Gorji, Jhunjhunu, Rajasthan 333022

Visit Centre

Courses

Access NCERT Exemplar Solutions for Class 11 Mathematics Chapter 7 - Permutations and Combinations (Examples, Easy Methods and Step by Step Solutions)

Examples:

Short Answer Type Questions:

Example 1: In a class, there are $27$ boys and $14$ girls. The teacher wants to select $1$ boy and $1$ girl to represent the class for a function. In how many ways can the teacher make this selection?

Ans : Given: Total number of boys $= 27$

Total number of girls $= 14$

Number of boys to be selected $= 1$

Number of girls to be selected $= 1$

Use the formula $^{27} C_{1}$ for the selection of $1$ boy and $^{14} C_{1}$ for the selection of $1$ girl.

To select $1$ boy out of a total of $27$ boys the number of ways is $^{27} C_{1} = 27$ .

To select $1$ girl out of a total of $14$ girls the number of ways is $^{14} C_{1} = 14$ .

Hence, the total number of ways for the teacher to make the selection $= 27 \times 14 = 378$ .

Example 2: (i) How many numbers are there between $99$ and $1000$ having $7$ in the units place?

Ans: Given: The numbers between $99$ and $1000$ .

fix the digit $7$ at the unit place and form all the three digit numbers

To find the numbers between $99$ and $1000$ that means to focus only on the three digit numbers. Fixing $7$ at the unit place, the tens and hundreds place is left to fill.

The hundreds of places can be filled with any digit other than $0$ , so there are $9$ choices. The tens place can be filled with any of the $10$ digits, so there are $10$ choices.

Hence, the total numbers of numbers $= 9 \times 10 = 90$ .

(ii) How many numbers are there between $99$ and $1000$ having at least one of their digits $7$ ?

Ans: Given: The numbers between $99$ and $1000$ .

form all the three digit numbers that can have $7$ at any place.

Number of numbers where at least one of digits is $7$ will be the difference of the total number of three digit numbers and the number of numbers where none of the digits is $7$ .

Total number of three digit numbers $= 900$ .

Now, in the numbers having no digit as $7$ , the hundreds place can be filled in $8$ ways (other than $0$ and $7$ ). Similarly, the tens place can be filled in $9$ ways (other than $7$ ) and the units place can also be filled in $9$ ways (other than $7$ ). Therefore, total numbers $= 8 \times 9 \times 9 = 648$ .

Hence, the total number of numbers $= 900 - 648 = 252$ .

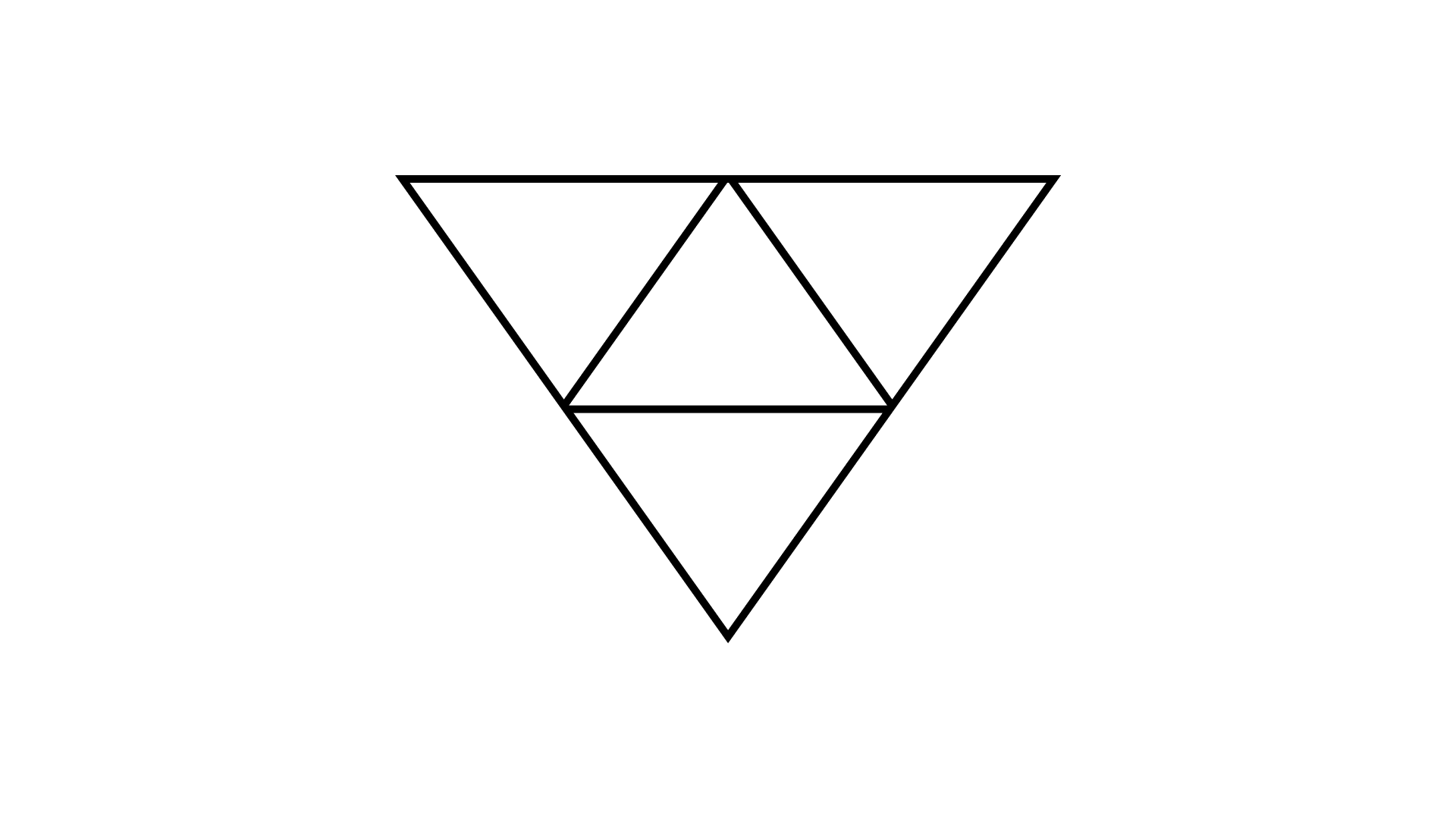
Example 3: In how many ways can this diagram be coloured subject to the following two conditions?

Each of the smaller triangles is to be painted with one of three colours: red, blue or green And No two adjacent regions have the same colour.

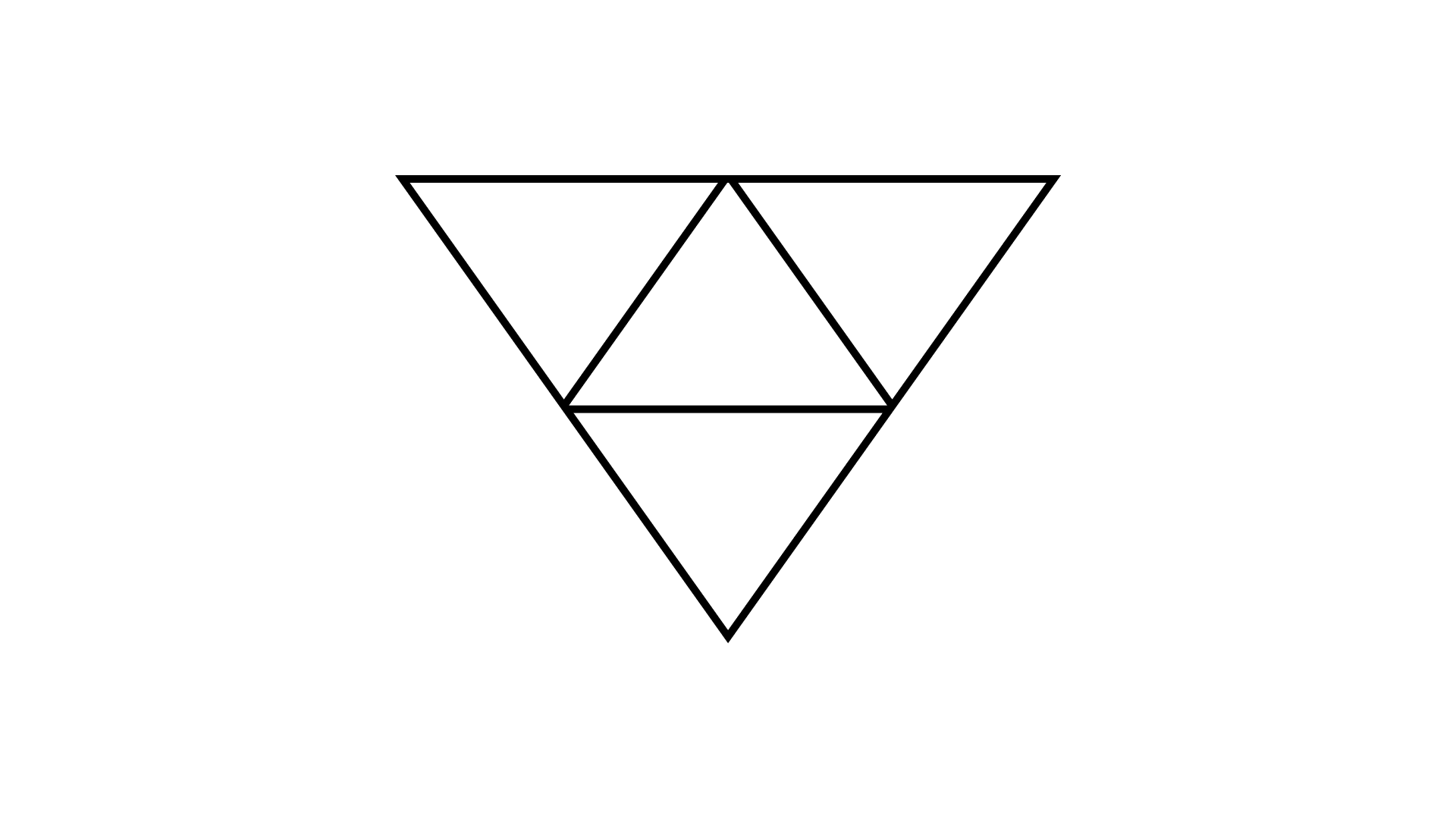
Ans : Given: The diagram: -

Total number of different colours $= 3$ (red, blue and green)

Fill the middle triangle with any of the three colours. Now, fill the three other triangles with the other two colours (may be same or different).

First let us fill the smaller triangle in the middle. There are $3$ choices to fill the middle triangle. Now, the other three triangles must be filled with the colour other than that is used to fill the middle triangle.

So there are $2$ choices of colours left for each of the three triangles to be filled. So these triangles can be filled in $2 \times 2 \times 2 = 8$ ways.

Hence, the total number of ways to colour all the smaller triangles $= 3 \times 8 = 24$ .

Example 4: In how many ways can $5$ children be arranged in a line such that:

(i) two particular children of them are always together.

Ans: Given: Total number of children $= 5$

assume the two particular children as one unit and arrange them among themselves. Now, arrange them will the remaining three children.

Since two particular children are always together so they can be arranged among themselves in $2!$ ways.

Now, they can be considered as one unit so the other three children and this one unit can be considered as $4$ beings and the number of ways to arrange them is $4!$ .

Hence, the total number of arrangements $= 2! \times 4! = 48$ .

(ii) two particular children of them are never together.

Ans: Given: Total number of children $= 5$

find the number of possible arrangements if no conditions are applied and subtract the arrangements obtained in (i) from this.

If there are no conditions then the $5$ children can be arranged in $5!$ ways. So the number of ways in which two particular children are never together will be the difference between the total number of arrangements with no condition and the number of arrangements where they are always together.

Hence, the total number of arrangements $= 5! - 48 = 72$ .

Example 5: If all permutations of the letters of the word AGAIN are arranged in the order as in a dictionary. What is the $4 9^{t h}$ word?

Ans : Given: The word AGAIN.

Arrange the letters in alphabetical order and find the number of words starting with the letters A, G and I. Check the sum and accordingly find the $49^{t h}$ word.

The alphabetical order of letters in the word AGAIN is A (repeated twice), G, I, N.

If A is chosen as the first letter of the word then the remaining four letters can be arranged in $4! = 24$ ways.

If G is fixed as the first letter then the remaining four letters can be arranged in $4!$ ways, however A is repeated twice so the effective different number of words formed $= \frac{4!}{2!} = 12$ .

Similarly, if I is the first letter then also the effective different number of words formed $= \frac{4!}{2!} = 12$

So a total of $(24 + 12 + 12) = 48$ words are formed. The next word will start with the letter N and the remaining letters will be arranged in alphabetical order.

Hence, the $49^{t h}$ word is NAAGI.

Example 6: In how many ways $3$ mathematics books, $4$ history books, $3$ chemistry books and $2$ biology books can be arranged on a shelf so that all books of the same subjects are together.

Ans : Given: Number of mathematics books $= 3$

Number of history books $= 4$

Number of chemistry books $= 3$

Number of biology books $= 2$

Arrange all the different subject books individually among themselves. Now, consider the similar subject books as a single unit and arrange them.

$3$ mathematics books can be arranged among themselves in $3!$ ways. Similarly, $4$ history books can be arranged among themselves in $4!$ ways, $3$ chemistry books in $3!$ ways and $2$ biology books in $2!$ ways.

Now, the group of similar subject books can be assumed to be as a single unit each, so consider each single unit of different subject books as a single object. Therefore, there are $4$ objects that can be arranged on the shelf in $4!$ ways.

Hence, the total number of arrangements possible $= 3! \times 4! \times 3! \times 2! \times 4! = 41472$ .

Exercise 7: A student has to answer $10$ questions choosing at least $4$ questions from each of Parts A and B. If there are $6$ questions in Part A and $7$ in Part B, in how many ways can the student choose $10$ questions?

Ans : Given: Total number of questions in part A $= 6$ .

Total number of questions in part B $= 7$ .

Number of questions to be answered $= 10$ .

Minimum number of questions that must be attempted from each part $= 4$ .

Consider different cases for the number of questions he can do from the two parts such that the sum of the attempted number of questions is $10$ . Add the number of arrangements of all the cases.

At least $4$ questions must be attempted from both the parts A and B. So the following cases arise: -

(1) From part A he can attempt $4$ questions and from part B $6$ questions.

Therefore, number of possible ways $=^{6} C_{4} \times^{7} C_{6} = 105$ .

(2) From part A he can attempt $5$ questions and from part B also $5$ questions.

Therefore, number of possible ways $=^{6} C_{5} \times^{7} C_{5} = 126$ .

(3) From part A he can attempt $6$ questions and from part B $4$ questions.

Therefore, number of possible ways $=^{6} C_{6} \times^{7} C_{4} = 35$ .

Hence, the total number of ways to choose questions $= 105 + 126 + 35 = 266$ .

Example 8: Suppose $m$ men and $n$ women are to be seated in a row so that no two women sit together. If $m > n$ , show that the number of ways in which they can be seated is $\frac{m! (m + 1)!}{(m - n + 1)!}$ ?

Ans : Given: Total number of men $= m$

Total number of women $= n$

No two women are to be seated together.

First arrange all the men and then arrange all the women between them such that any two women are not adjacent to each other.

First let us arrange all the men.

_ M _ M _ M _ M _ M _ M … M _ (total $m$ men)

Now, when the number of men is $m$ then there will be $(m + 1)$ spaces where $n$ women can be seated. Since $(m + 1) > n$ that means $n$ spaces are to be selected from a total of $(m + 1)$ which can be done in $^{m + 1} C_{n}$ ways.

Also, $m$ men can be arranged among themselves in $m!$ ways and $n$ women can be arranged among themselves in $n!$ .

Therefore, the total number of arrangements $=^{m + 1} C_{n} \times m! \times n! = \frac{m! (m + 1)!}{(m - n + 1)!}$ .

Example 9: Three married couples are to be seated in a row having six seats in a cinema hall. If spouses are to be seated next to each other, in how many ways can they be seated? Find also the number of ways of their seating if all the ladies sit together.

Ans : Given: Total number of married couples $= 3$

Total number of seats $= 6$

For (i) consider that the three pairs can be arranged among themselves in $3!$ ways and the two persons in each couple can be arranged within them in $2!$ ways.

For (ii) select the combination of seats such that the three ladies will always be sitting together and arrange them among themselves. Now, arrange the three men at three places.

Let us assume the couples as $M_{1} F_{1}, M_{2} F_{2}$ and $M_{3} F_{3}$ , where $M$ represents the male person and $F$ represents the female person.

(i) When the spouses will be sitting next to each other then there will be three pairs to be arranged at six seats. So the number of ways to arrange the couples is $3!$ . The two persons in each couple can be arranged among them in $2!$ ways.

Therefore, the total number of arrangements $= 3! \times 2! \times 2! \times 2! = 48$ .

(ii) In case all the ladies will be sitting together, let us assume the seats are numbered as $1, 2, 3, 4, 5, 6$ . The combinations in which all the ladies will always be together are $(1, 2, 3), (2, 3, 4), (3, 4, 5)$ and $(4, 5, 6)$ . So there are $4$ combinations.

The three ladies can be arranged among themselves in $3!$ ways. Now, the three men are to be seated at the remaining three seats, so the number of ways to arrange the men is $3!$ .

Therefore, the total number of arrangements $= 4 \times 3! \times 3! = 144$ .

Example 10: In a small village, there are $87$ families, of which $52$ families have at most $2$ children. In a rural development programme $20$ families are to be chosen for assistance, of which at least $18$ families must have at most $2$ children. In how many ways can the choice be made?

Ans : Given: Total number of families in the village $= 87$

Number of families having at most $2$ children $= 52$

Number of families to be chosen for assistance $= 20$

Consider different case: -

In the first case assume that $18$ families chosen have at most $2$ children.

In the second case assume that $19$ families chosen have at most $2$ children.

In the third case assume that all the $20$ families chosen have at most $2$ children.

Since, at least $18$ of the $20$ families chosen must have at most $2$ children, so the following cases can be taken: -

(1) $18$ families chosen have at most $2$ children.

In this case $18$ families must be chosen from a total of $52$ families and the remaining $2$ families from the remaining $35$ families which do not have at most $2$ children.

Therefore, the total number of ways to make the choice $=^{52} C_{18} \times^{35} C_{2}$ .

(2) $19$ families chosen have at most $2$ children.

In this case $19$ families must be chosen from a total of $52$ families and the remaining $1$ families from the remaining $35$ families.

Therefore, the total number of ways to make the choice $=^{52} C_{19} \times^{35} C_{1}$ .

(3) All the $20$ families chosen have at most $2$ children.

In this case all the $20$ families must be chosen from a total of $52$ families.

Therefore, the total number of ways to make the choice $=^{52} C_{20}$ .

Hence, the total number of ways to make the choice $=^{52} C_{18} \times^{35} C_{2} +^{52} C_{19} \times^{35} C_{1} +^{52} C_{20}$ .

Example 11: A boy has $3$ library tickets and $8$ books of his interest in the library. Of these $8$ , he does not want to borrow Mathematics Part II, unless Mathematics Part I is also borrowed. In how many ways can he choose the three books to be borrowed?

Ans : Given: Total number of books of interest $= 8$ .

Number of library tickets $= 3$ .

Consider three cases: -

In the first case assume that Mathematics Part I is not borrowed.

In the second case assume that both Mathematics Part I and II are borrowed.

In the third case assume that Mathematics Part I is borrowed but not II.

The following three cases are possible: -

(1) Mathematics Part I is not borrowed.

So, in this case Mathematics Part II will also not be borrowed. That means the three books can be borrowed from the remaining $6$ books in $^{6} C_{3}$ ways.

(2) Both Mathematics Part I and II are borrowed.

So, in this case the remaining one book can be borrowed from the remaining $6$ books in $^{6} C_{1}$ ways.

(3) Mathematics Part I is borrowed but II is not borrowed.

So, in this case the remaining two books can be borrowed from the remaining $6$ books in $^{6} C_{2}$ ways.

Hence, the total number of ways to borrow the books $=^{6} C_{3} +^{6} C_{1} +^{6} C_{2} = 41$ .

Example 12: Find the number of permutations of $n$ different things taken $r$ at a time such that two specific things occur together.

Ans : Given: Total number of distinct things $= n$

Number of things selected $= r$

$2$ specific things occur together.

First select $(r - 2)$ things from a total of $(n - 2)$ things. Now, arrange $2$ things which occur together and then consider them as a single unit and arrange $(r - 1)$ things.

Since from a total of $n$ things, $r$ things are to be taken at a time and $2$ things are always together. That means those $2$ things will always be selected.

Therefore, now $(r - 2)$ things are left to be selected from a total of $(n - 2)$ things. So the number of ways of selection $=^{n - 2} C_{r - 2}$ .

The $2$ things which occur together can be arranged among themselves in $2!$ ways.

Now, these $2$ things can be considered as a single unit and so $(r - 2 + 1) = (r - 1)$ things are left to be arranged. So the number of ways of arrangement $= (r - 1)!$ .

Hence, the total number of permutations $=^{n - 3} C_{r - 3} \times 2! \times (r - 1)! = 2! (r - 1)^{n - 3} P_{r - 3}$ .

Example 13: There are four bus routes between A and B; and three bus routes between B and C. A man can travel round – trip in number of ways by bus from A to C via B. If he does not want to use a bus route more than once, in how many ways can he make round trip?

(A) $72$

(B) $144$

(D) $19$

Ans : The correct option is A.

Given: Total number of bus routes from A to B $= 4$

Total number of bus routes from B to C $= 3$

First choose one of the paths from A to B then choose one of the paths from B to C. Now, in returning choose a different path from C to B and then from B to A.

Since, there are four routes from A to B, there are $^{4} C_{1}$ ways to choose one of the routes. Now, from B to C there are three routes and one of them can be chosen in $^{3} C_{1}$ ways.

On returning from C to B a route is to be chosen other than the route chosen to travel from B to C. So there are two routes left and one of them can be chosen in $^{2} C_{1}$ . Similarly, from B to A there are three routes left and one of them can be chosen in $^{3} C_{1}$ ways.

Hence, the total number of ways to make a round – trip $=^{4} C_{1} \times^{3} C_{1} \times^{2} C_{1} \times^{3} C_{1} = 72$ .

Correct Answer: A

Example 14: In how many ways a committee consisting of $3$ men and $2$ women, can be chosen from $7$ men and $5$ women?

(A) $45$

(B) $350$

(D) $230$

Ans : The correct option is B.

Given: Total number of men $= 7$

Total number women $= 5$

Number of men to be chosen $= 3$

Number of women to be chosen $= 2$

Use the formula $^{7} C_{3}$ to select $3$ men from a total of $7$ and the formula $^{5} C_{2}$ to select $2$ women from a total of $5$ . Multiply both the number of ways.

To choose $3$ men out a total of $7$ men there are $^{7} C_{3}$ ways. Similarly, to choose $2$ women out of a total of $5$ women there are $^{5} C_{2}$ .

Therefore, the total number of ways to form the committee $=^{7} C_{3} \times^{5} C_{2} = 350$ .

Correct Answer: B

Example 15: All the letters of the word ‘EAMCOT’ are arranged in different possible ways. The number of such arrangements in which no two vowels are adjacent to each other is

(A) $360$

(B) $144$

(D) $54$

Ans: The correct option is B.

Given: The word ‘EAMCOT’.

First arrange all the consonants of the word and then arrange the vowels between them such that any two vowels are not side by side.

There are three vowels (A, E, O) and three consonants (C, M, T) in the word ‘EAMCOT’. Let us first arrange the consonants.

_ C _ M _ T _

Now, the three consonants can be arranged among themselves in $3!$ ways. There are $4$ blank spaces where the $3$ vowels can be placed and no two of them will be adjacent. So the number of ways to select the three positions and arrange the vowels there is given as $^{4} P_{3}$ .

Hence, the total number of ways $= 3! \times^{4} P_{3} = 144$ .

Correct Answer: B

Example 16: Ten different letters of the alphabet are given. Words with five letters are formed from these given letters. Then the number of words which have at least one letter repeated is

(A) $69760$

(B) $30240$

(D) $99784$

Ans : The correct option is A.

Given: Total number of letters given $= 10$

Number of letters to be chosen $= 5$

Find the number of five letter words that can be formed if repetitions of letters are allowed. Now, find the number of five letter words that can be formed by five different letters given.

Consider the difference of the number of words formed in the two conditions.

Since there are $10$ letters already given and five letter words are to be formed. Suppose there are five boxes and letters are to be filled in them.

In case the letters are allowed to repeat then each box can be filled in $10$ ways because there are $10$ choices of letters, so the number of words formed $= 10 \times 10 \times 10 \times 10 \times 10 = 10^{5}$ .

If none of the letters are repeated then the five letters can be selected and arranged in five boxes in $^{10} P_{5}$ ways.

Therefore, the number of words formed which have at least one letter repeated will be the difference of the number of words formed if the letters can be repeated and the number of words formed if no letter is repeated.

Hence, total number of words formed $= 10^{5} -^{10} P_{5} = 69760$ .

Correct Answer: A

Example 17: The number of signals that can be sent by $6$ flags of different colours taking one or more at a time is

(A) $63$

(B) $1956$

(D) $21$

Ans : The correct option is B.

Given: Total number of flags of different colours $= 6$

Consider different cases where the number of flags used to send the signals will vary. For more than one flag taken at a time consider their order of sending the signals also.

One or more flags are being used to send the signals so the following cases arise: -

(1) Only one flag is used.

So, one flag can be selected in $^{6} C_{1}$ ways.

(2) Two flags are used.

So, two flags can be selected in $^{6} C_{2}$ ways. Also, any of the two flags can be used to send the signal first. That means the two flags is to be arranged among them which can be done in $2!$ ways. So the total number of ways will be $^{6} C_{2} \times 2! =^{6} P_{2}$ .

(3) Three flags are used.

The total number of ways will be $^{6} C_{3} \times 3! =^{6} P_{3}$ .

(4) Four flags are used.

The total number of ways will be $^{6} C_{4} \times 4! =^{6} P_{4}$ .

(5) Five flags are used.

The total number of ways will be $^{6} C_{5} \times 5! =^{6} P_{5}$ .

(6) All the six flags are used.

The total number of ways will be $^{6} C_{6} \times 6! =^{6} P_{6}$ .

Hence, the total number of ways to send the signals $=^{6} C_{1} +^{6} P_{2} +^{6} P_{3} +^{6} P_{4} +^{6} P_{5} +^{6} P_{6} = 1956$ .

Correct Answer: B

Example 18: In an examination there are three multiple choice questions and each question has $4$ choices. Number of ways in which a student can fail to get all answer correct is

(A) $11$

(B) $12$

(D) $63$

Ans : The correct option is D.

Given: Number of multiple choice questions $= 3$

Number of choices in each question $= 4$

Find the number of ways he can answer all the questions. Now, subtract $1$ from this which is the case that he gets all the choices correct.

Each of the three multiple choice questions has $4$ choices. So, the number of ways the combination of choices he can make will be $4 \times 4 \times 4 = 64$ .

Since, only one choice among the four choices in each of the questions will be correct, there will be only a $1$ combination where he can answer all the questions correctly.

Therefore, the number of ways in which the student can fail to get all answer correct $= 64 - 1 = 63$ .

Correct Answer: D

Example 19: The straight lines $l_{1}, l_{2}$ and $l_{3}$ are parallel and lie in the same plane. A total number of $m$ points are taken on $l_{1}$ , $n$ points on $l_{2}$ , $k$ points on $l_{3}$ . The maximum number of triangles formed with vertices at these points are

(A) $^{(m + n + k)} C_{3}$

(B) $^{(m + n + k)} C_{3} -^{m} C_{3} -^{n} C_{3} -^{k} C_{3}$

(D) $^{m} C_{3} \times^{n} C_{3} \times^{k} C_{3}$

Ans : The correct option is B.

Given: Total number parallel lines $= 3 (l_{1}, l_{2}, l_{3})$

Number of points on line $l_{1} = m$

Number of points on line $l_{2} = n$

Number of points on line $l_{3} = k$

Find the total number of points on the three lines and select any three of them. Subtract the number of cases where all the points lie on the same line.

Three no – collinear points are required to form a triangle. Since the total number of points on the three lines are $(m + n + k)$ , so any $3$ points can be selected in $^{(m + n + k)} C_{3}$ ways.

Now, in case all the points lie on the same line then they will not form a triangle. So, if all the three points are chosen from amongst the $m$ points lying on the line $l_{1}$ in $^{m} C_{3}$ ways then they will not form a triangle.

Similarly, $^{n} C_{3}$ and $^{k} C_{3}$ ways of selection of the three points will also not form any triangle.

Hence, the total number of triangles formed $=^{(m + n + k)} C_{3} -^{m} C_{3} -^{n} C_{3} -^{k} C_{3}$ .

Correct Answer: B

Exercise

Short Answer Type Questions:

1: Eight chairs are numbered $1$ to $8$ . Two women and $3$ men wish to occupy one chair each. First the women choose the chairs from amongst the chairs $1$ to $4$ and then men select from the remaining chairs. Find the total number of possible arrangements.

Ans: Given: Number of chairs $= 8$

Number of women $= 2$

Number of chairs $= 3$

Women choose the chairs from amongst the chairs $1$ to $4$ .

Use the formula $^{4} P_{2}$ for the number of arrangements of women and $^{6} P_{3}$ for the number of arrangements of men. Multiply the number of arrangements of women and men.

First $2$ women will select $2$ chairs from amongst the chairs $1$ to $4$ (total $4$ chairs), so the number of arrangements of women $=^{4} P_{2} = 12$ .

Now, $6$ chairs will be remaining for $3$ men to select, so the number of arrangements of men $=^{6} P_{3} = 120$ .

Since both the activities are to be performed, so the total number of possible arrangements $= 12 \times 120 = 1440$ .

2: If the letters of the word RACHIT are arranged in all possible ways as listed in the dictionary. Then what is the rank of the word RACHIT?

Ans: Given: The word RACHIT.

Find the number of words that can be formed with the starting letters as A, C, H and I. Take the sum of the number of words formed in each case and add $1$ to get the rank.

In the word RACHIT there are $6$ different alphabets A, C, H, I, R and T (arranged in alphabetical order).

Total number of words that can be formed with the starting letter as A $= 5! = 120$ .

Total number of words that can be formed with the starting letter as C $= 5! = 120$ .

Total number of words that can be formed with the starting letter as H $= 5! = 120$ .

Total number of words that can be formed with the starting letter as I $= 5! = 120$ .

Now, the next word will be RACHIT, so its rank $= 120 + 120 + 120 + 120 + 1 = 481$ .

3: A candidate is required to answer $7$ questions out of $12$ questions, which are divided into two groups, each containing $6$ questions. He is not permitted to attempt more than $5$ questions from either group. Find the number of different ways of doing questions.

Ans: Given: Total number of questions $= 12$ .

Number of questions to be answered $= 7$ .

Number of questions in the $2$ groups is $6$ each.

Maximum number of questions that can be attempted from each group $= 5$ .

Consider different cases for the number of questions he can do from the two groups such that the sum of the attempted number of questions is $7$ . Add the number of arrangements of all the cases.

Since the candidate has to attempt $7$ questions out of $12$ questions which are divided into two groups of $6$ questions each. He cannot attempt more than $5$ questions from each group. So the following cases arise: -

(1) From the first group he can attempt $2$ questions and from the second group $5$ questions.

Therefore, number of possible ways $=^{6} C_{2} \times^{6} C_{5} = 90$ .

(2) From the first group he can attempt $3$ questions and from the second group $4$ questions.

Therefore, number of possible ways $=^{6} C_{3} \times^{6} C_{4} = 300$ .

(3) From the first group he can attempt $4$ questions and from the second group $3$ questions.

Therefore, number of possible ways $=^{6} C_{4} \times^{6} C_{3} = 300$ .

(4) From the first group he can attempt $5$ questions and from the second group $2$ questions.

Therefore, number of possible ways $=^{6} C_{5} \times^{6} C_{2} = 90$ .

Hence, the total number of ways to attempt questions $= 90 + 300 + 300 + 90 = 780$ .

4: Out of $18$ points in a plane, no three are in the same line except five points which are collinear. Find the number of lines that can be formed joining the point.

Ans: Given: Total number of points in a plane $= 18$

Number of collinear points $= 5$ .

Use the formula $^{18} C_{2}$ to find the number of lines formed by $18$ non – collinear points.

Use the formula $^{5} C_{2}$ to find the number of lines formed by $5$ collinear points.

Subtract $^{5} C_{2}$ from $^{18} C_{2}$ and add $1$ to get the answer.

To form a straight line segment $2$ points are required, so the number of straight lines that would have been formed if all the $18$ points were non – collinear $=^{18} C_{2}$ .

Since $5$ points among $18$ points are collinear, that means they lie on a single straight line, so those $5$ points will form the $^{5} C_{2}$ number of the same straight lines. Overall the number of distinct straight lines formed by $5$ collinear points will be $1$ .

Therefore, the total number of distinct straight lines that will be formed by the given $18$ points $=^{18} C_{2} -^{5} C_{2} + 1 = 144$ .

5: We wish to select $6$ persons from $8$ , but if person A is chosen, then B must be chosen. In how many ways can selection be made?

Ans: Given: Total number of persons $= 8$

Number of persons to be selected $= 6$

If person A is chosen then person B must be chosen.

Consider three cases: -

In the first case assume that A is selected and find the possible ways.

In the second case consider that A is not selected and find the number of ways.

In the third case consider that B is selected but A is not and find the number of ways.

Add the number of ways obtained in all the cases to get the answer.

Since, $6$ persons are to be selected from a total of $8$ persons and if A is selected then B is also selected. So the following cases arise: -

Case (1): - Considering that person A is selected. That means person B is also selected.

Therefore, number of ways to select the remaining $4$ persons from the remaining total of $6$ persons $=^{6} C_{4} = 15$ .

Case (2): - Considering that person A is not selected. That means person B is also not selected.

Therefore, number of ways to select the remaining $6$ persons from the total of $6$ persons $=^{6} C_{6} = 1$ .

Case (3): - It may also be possible that B is selected and not A because there is no such given condition that if B is selected then A must also be selected.

Therefore, number of ways to select the remaining $5$ persons from the total of $6$ persons $=^{6} C_{5} = 6$ .

Hence, the total number of ways of selection $= 15 + 1 + 6 = 22$ .

6: How many committees of five persons with a chairperson can be selected from $12$ persons?

Ans: Given: Total number of persons $= 12$

Number of persons to be selected $= 5$

Number of chairperson $= 1$

Use the formula $^{12} C_{5}$ to select five persons for the committee and then $^{5} C_{1}$ to select the chairperson. Multiply both the expressions to get the answer.

Since $5$ persons are to be selected from a total of $12$ persons, so the number of ways of selection $=^{12} C_{5} = 792$ .

Now, $1$ person among these $5$ persons will be the chairperson of the committee, so the number of ways of selection $=^{5} C_{1} = 5$ .

Hence, the total number of ways to form the committee $= 792 \times 5 = 3960$ .

7: How many automobile license plates can be made if each plate contains two different letters followed by three different digits?

Ans: Given: Count of different letters on the number plate $= 2$

Count of different digits on the number plate $= 3$

Use the formula $^{26} P_{2}$ to select and arrange two different alphabets and then $^{10} P_{3}$ to select three different digits. Multiply both the expressions to get the answer.

First two different letters are to be selected and arranged among themselves and there are $26$ different alphabets, so the number of ways of arrangements $=^{26} P_{2} = 650$ .

Now, three different digits are to be selected and arranged among themselves and there are $10$ different digits, so the number of ways of arrangements $=^{10} P_{3} = 720$ .

Hence, the total number of license plates that can be made $= 650 \times 720 = 468000$ .

8: A bag contains $5$ black and $6$ red balls. Determine the number of ways in which $2$ black and $3$ red balls can be selected from the lot.

Ans: Given: Total number of black balls $= 5$

Total number of red balls $= 6$

Number of black balls to be selected $= 2$

Number of red balls to be selected $= 3$

Use the formula $^{5} C_{2}$ to select $2$ black balls and then $^{6} C_{3}$ to select $3$ red balls. Multiply both the expressions to get the answer.

Since there are $5$ black balls from which only $2$ are to be selected, so the number of ways of selection $=^{5} C_{2} = 10$ .

Now, there are $6$ red balls from which only $3$ are to be selected, so the number of ways of selection $=^{6} C_{3} = 20$ .

Hence, the total number of ways in which $2$ black and $3$ red balls can be selected $= 20 \times 10 = 200$ .

9: Find the number of permutations of $n$ distinct things taken $r$ together, in which $3$ particular things must be together.

Ans: Given: Total number of distinct things $= n$

Number of things selected $= r$

$3$ particular things are always together.

First select $(r - 3)$ things from a total of $(n - 3)$ things. Now, arrange $3$ things which are always together and then consider them as a single unit and arrange $(r - 2)$ things.

Since from a total of $n$ things, $r$ things are to be taken and $3$ things are always together. That means those $3$ things will always be selected.

Therefore, now $(r - 3)$ things are left to be selected from a total of $(n - 3)$ things. So the number of ways of selection $=^{n - 3} C_{r - 3}$ .

The $3$ things which are together can be arranged among themselves in $3!$ ways.

Now, these $3$ things can be considered as a single unit and so $(r - 3 + 1) = (r - 2)$ things are left to be arranged. So the number of ways of arrangement $= (r - 2)!$ .

Hence, the total number of permutations $=^{n - 3} C_{r - 3} \times 3! \times (r - 2)!$ .

10: Find the number of different words that can be formed from the letters of the word ‘TRIANGLE’ so that no vowels are together.

Ans: Given: The letters of the word ‘TRIANGLE’.

First arrange all the consonants of the given word and then arrange the vowels between them such that two vowels must not occur adjacently.

In the word ‘TRIANGLE’, the three vowels are A, E, I and the five consonants are G, L, N, R, T.

$5$ consonants can be arranged among themselves in $5!$ ways.

_ G _ L _ N _ R _ T _

Now, the $3$ vowels can be filled at $6$ different places so that no two vowels are together. The $3$ places out of $6$ places and are to be selected and the vowels are arranged.

So the total number of arrangements $=^{6} C_{3} \times 3!$

Hence, the total number of words that can be formed $= 5! \times^{6} C_{3} \times 3! = 14400$ .

11: Find the number of possible integers greater than $6000$ and less than $7000$ which are divisible by $5$ , provided that no digit is to be repeated.

Ans: Given: Numbers $6000$ and $7000$ .

Consider two cases: -

In the first case fill the unit place digit as $0$ and find the number of ways to fill the tens and hundreds place.

In the second case fill the unit place digit as $5$ and find the number of ways to fill the tens and hundreds place.

Add the total numbers of ways obtained in the two cases.

Since the integer is to lie between $6000$ and $7000$ that means the thousands place digit of the possible integers will always be $6$ . Also the integer should be divisible by $5$ that means the unit place digit will be either $0$ or $5$ . The following cases arise: -

(1) The unit's place digit is $0$ so the number will be of the form 6 _ _ 0. The digits cannot be repeated than means the remaining two places can be filled in $=^{8} P_{2} = 56$ ways.

(2) The unit's place digit is $5$ so the number will be of the form 6 _ _ 5. Again the digits cannot be repeated than means the remaining two places can be filled in $=^{8} P_{2} = 56$ ways.

Hence, the total number of integers that can be formed $= 56 + 56 = 112$ .

12: There are $10$ persons named $P_{1}, P_{2}, P_{3}, . . ., P_{10}$ . Out of $10$ persons, $5$ persons are to be arranged in a line such that in each arrangement $P_{1}$ must occur whereas $P_{4}$ and $P_{5}$ does not occur. Find the number of such possible arrangements.

Ans : Given: Total number of persons $= 10 (P_{1}, P_{2}, P_{3}, . . ., P_{10})$

Number of persons to be selected $= 5$

Use the formula $^{7} P_{4}$ to select the remaining $4$ persons out of $7$ persons. Now, arrange $5$ persons in $5!$ ways.

Since $5$ persons are to be selected where $P_{1}$ must occur whereas $P_{4}$ and $P_{5}$ does not occur. That means the remaining $4$ persons are to be selected from the remaining $7$ persons $(P_{2}, P_{3}, P_{6}, P_{7}, P_{8}, P_{9}, P_{10})$ .

Therefore, the number of ways to select $=^{7} C_{4} = 35$ .

Now, $5$ persons selected can be arranged in $5! = 120$ ways.

Therefore, total number of arrangements $= 35 \times 120 = 4200$ .

13: There are $10$ lamps in the hall. Each of them can be switched on independently. Find the number of ways in which the hall can be illuminated.

Ans : Given: Total number of lamps $= 10$

Their switches are independent of each other.

Use the formula $2^{10}$ to find the number of ways in which the combination of switches (on or off) can be made. Subtract one of the combinations where all the switches are off.

Since there are $10$ lamps and each lamp may be either switched on or off, i.e. there are $2$ possible options, so the number of combinations that can be made for all the switches $= 2^{10}$ .

Now, one of the combinations will be such that all the switches are off and therefore the hall cannot be illuminated. To illuminate the hall at least one of the switches must be on.

Therefore, the total number of ways to illuminate the hall $= 2^{10} - 1 = 1023$ .

14: A box contains two white, three black and four red balls. In how many ways can three balls be drawn from the box, if at least one black ball is to be included in the draw?

Ans : Given: Total number of white balls $= 2$

Total number of black balls $= 3$

Total number of red balls $= 4$

Number of balls to be drawn $= 3$

Consider three cases: -

In the first case, draw one black ball and find the ways to draw the other two balls.

In the second case draw two black balls and find the ways to draw the remaining one ball.

In the third case draw all the three black balls.

Since at least one black ball is to be drawn, so the following three cases arise: -

(1) One black ball is drawn from a total of three black balls and the other two balls are drawn from the remaining six balls.

Therefore, the number of ways to select $=^{3} C_{1} \times^{6} C_{2} = 45$

(2) Two black balls are drawn from a total of three black balls and the remaining one ball is drawn from the remaining six balls.

Therefore, the number of ways to select $=^{3} C_{2} \times^{6} C_{1} = 18$

(3) All the three black balls are drawn.

Therefore, the number of ways to select $=^{3} C_{3} = 1$

Hence, the total number of ways to draw at least one black ball $= 45 + 18 + 1 = 64$ .

15: If $^{n} C_{r - 1} = 36$ , $^{n} C_{r} = 84$ and $^{n} C_{r + 1} = 126$ , then find $^{r} C_{2}$ .

Ans : Given: $^{n} C_{r - 1} = 36$

$^{n} C_{r - 1} = 36$

$^{n} C_{r + 1} = 126$

Use the formula $^{n} C_{r} = \frac{n!}{r! (n - r)!}$ and evaluate the relations $\frac{^{n} C_{r}}{^{n} C_{r - 1}}$ and $\frac{^{n} C_{r + 1}}{^{n} C_{r}}$ to find the value of $r$ . Now, substitute the value in $^{r} C_{2}$ to get the answer.

Using the formulas $^{n} C_{r} = \frac{n!}{r! (n - r)!}$ , $^{n} C_{r - 1} = \frac{n!}{(r - 1)! (n - r + 1)!}$ and $^{n} C_{r + 1} = \frac{n!}{(r + 1)! (n - r - 1)!}$ ,

$\Rightarrow \frac{^{n} C_{r}}{^{n} C_{r - 1}} = \frac{84}{36}$

$\Rightarrow \frac{n - r + 1}{r} = \frac{7}{3}$

$\Rightarrow 3 n - 3 r + 3 = 7 r$

$\Rightarrow 3 n + 3 = 10 r . . . . . . . . . . . . . . . (i)$

Similarly,

$\Rightarrow \frac{^{n} C_{r + 1}}{^{n} C_{r}} = \frac{126}{84}$

$\Rightarrow \frac{n - r}{r + 1} = \frac{3}{2}$

$\Rightarrow 2 n - 2 r = 3 r + 3$

$\Rightarrow 2 n = 5 r + 3. . . . . . . . . . . . . . . . (i i)$

Solving equations $(i)$ and $(i i)$ ,

$\Rightarrow r = 3$

Therefore, the value of $^{r} C_{2} =^{3} C_{2} = 3$ .

16: Find the number of integers greater than $7000$ that can be formed with the digits $3, 5, 7, 8$ and $9$ where no digits are repeated.

Ans: Given: The digits $3, 5, 7, 8$ and $9$ .

The integer $7000$ .

Consider two cases: -

In the first case form all the five digit integers using the formula $5!$ .

In the second case form all the four digits integers that are greater than $7000$ .

Add the number of arrangements of the two cases.

Since the number to be formed should be greater than $7000$ , so the following two cases are possible: -

(1) All the five digit integers will be greater than $7000$ , therefore the number of $5$ digit integers that can be formed $= 5! = 120$ (no digit repeated).

(2) The four digit integers in which the thousands place digit is either $7, 8$ or $9$ will be greater than $7000$ . So there are $3$ ways to fill the thousands place digit.

Now, the other three places can be filled (with the remaining four digits) in $4 \times 3 \times 2 = 24$ ways (no digits repeated).

Therefore, the number of four digit integers which are greater than $7000$ is $24 \times 3 = 72$ .

Hence, in overall the number of integers greater than $7000$ is $120 + 72 = 192$ .

17: If $20$ lines are drawn in a plane such that no two of them are parallel and no three are concurrent, how many points will they intersect each other?

Ans: Given: Number of lines $= 20$

No two lines are parallel and no three lines are concurrent (meet at the same point).

Use the formula $^{20} C_{2}$ to select any two lines which intersect at a point.

Since two non – parallel lines intersect at only one point so the total number of points of intersection of $20$ no – parallel lines will be equal to the number of ways to select any $2$ lines out of those $20$ lines.

Therefore, the number of ways to select $2$ lines out of a total of $20$ lines $=^{20} C_{2} = 190$ .

18: If a certain city, all telephone numbers have six digits, the first two digits always being $41$ or $42$ or $46$ or $62$ or $64$ . How many telephone numbers have all six digits distinct?

Ans: Given: Number of digits in a telephone number $= 6$ .

The first two digits are either $41$ or $42$ or $46$ or $62$ or $64$ .

Use the formula $^{8} P_{4}$ for the selection and arrangement of $4$ remaining distinct digits of the telephone number. Multiply it with $5$ to get the answer.

There are $5$ choices for the starting two digits of a telephone number. Now, the next four digits can be selected and arranged among themselves from the remaining $8$ digits in $^{8} P_{4}$ ways (without repetition of digits).

Therefore, the number of ways to form the telephone numbers $= 5 \times^{8} P_{4} = 8400$ .

19: If an examination, a student has to answer $4$ questions out of $5$ questions: questions $1$ and $2$ are however compulsory. Determine the number of ways in which the student can make the choice.

Ans: Given: Total number of questions $= 5$ .

Number of questions to be answered $= 4$ .

Questions $1$ and $2$ are compulsory.

Use the formula $^{3} C_{2}$ to select the remaining two non compulsory questions which are questions $3, 4$ and $5$ .

Since two out of five questions are compulsory and the student has to do any four questions. That means the remaining two questions are to be selected from questions $3, 4$ or $5$ .

Therefore, the number of ways to select any two questions out of the remaining three $=^{3} C_{2} = 3$ .

Hence, the number of ways in which the student can make the choice is $3$ .

20: A convex polygon has $44$ diagonals. Find the number of its sides.

Ans : Given: Total number of diagonals of the convex polygon $= 44$ .

Use the formula, number of diagonals $= (^{n} C_{2} - n)$ , where $n$ is the number of sides, to calculate the value of $n$ .

The diagonals of an $n$ sided polygon can be formed by joining any two non – adjacent vertices of the polygon. If the adjacent points are joined then they form a side and not a diagonal.

An $n$ sided polygon has $n$ vertices and $n$ sides, so the number of ways to select any two points $=^{n} C_{2}$ . Here $n$ pairs of vertices are adjacent to each other, so among $^{n} C_{2}$ number of line segments there are $n$ line segments which are not diagonals.

Therefore, the number of diagonals $=^{n} C_{2} - n$

$\Rightarrow^{n} C_{2} - n = 44$

$\Rightarrow \frac{n (n - 1)}{2} - n = 44$

$\Rightarrow n^{2} - 3 n - 88 = 0$

Using the middle term split method,

$\Rightarrow (n - 11) (n + 8) = 0$

$\Rightarrow n = 11$ or $- 8$

Here $n = - 8$ is not possible because the number of sides is not negative, therefore $n = 11$ .

21: 18 mice were placed in two experimental groups and one control group, with all groups equally large. In how many ways can the mice be placed into three groups?

Ans : Given: Total number of mice $= 18$

Number of experimental groups $= 2$

Number of control group $= 1$

All the groups are equally large.

Use the formula $^{18} C_{12}$ to select $12$ mice out of $18$ to be placed in two experimental groups. Now, use the formula $^{12} C_{6}$ to select $6$ mice out of $12$ to be placed in one of the experimental groups.

Multiply both the expressions.

Since all groups are equally large that means they will contain $6$ mice each.

$12$ mice will be placed in the two experimental groups combined, so the number of ways to select $12$ out of $18$ mice $=^{18} C_{12}$ .

The remaining $6$ mice will automatically gets selected and placed in the control group because only $6$ will be left and the number of ways will be $^{6} C_{6} = 1$ .

Now, among $12$ mice $6$ will be placed in one experimental group and $6$ in other, so the number of ways to select $6$ out of $12$ mice $=^{12} C_{6}$ .

Since nothing is given that the two experimental groups are different so they are assumed to be similar. Therefore, there is no need to select any one of the two experimental groups to place $6$ particular mice among $12$ . This is because the number of ways to select one group will be $2$ and since the two groups are similar so it will be divided by $2!$ which results in $1$ .

Hence, the total number of ways $=^{18} C_{12} \times^{12} C_{6} = \frac{18!}{6! \times 6! \times 6!}$ .

22: A bag contains six white marbles and five red marbles. Find the number of ways in which four marbles can be drawn from the bag if

(a) they can be of any colour

Ans: Given: Total number of white marbles $= 6$

Total number of red marbles $= 5$

Number of marbles drawn $= 4$

use the formula $^{11} C_{4}$ to select any $4$ marbles out of a total of $11$ .

The number of ways to select any $4$ marbles out of $11$ marbles (can be of any colour) $=^{11} C_{4} = 330$ .

(b) two must be white and two red and

Ans: Given: Total number of white marbles $= 6$

Total number of red marbles $= 5$

Number of marbles drawn $= 4$

use the formula $^{6} C_{2} \times^{5} C_{2}$ to select two white and two red marbles.

Two white marbles can be selected out of a total of six white marbles in $^{6} C_{2}$ ways and two red marbles can be selected out of a total of five red marbles in $^{5} C_{2}$ .

Therefore, the total number of ways of selection of two white and two red marbles $=^{6} C_{2} \times^{5} C_{2} = 150$ .

Ans: Given: Total number of white marbles $= 6$

Total number of red marbles $= 5$

Number of marbles drawn $= 4$

consider two cases. In the first case assume all the selected marbles are white and in the second case assume all are red. Add the number of ways obtained in the two cases.

To select all the marbles of the same colour, there can be two cases: -

(i) All the marbles are white, so the number of ways of selection $=^{6} C_{4} = 15$ .

(i) All the marbles are red, so the number of ways of selection $=^{5} C_{4} = 5$ .

Therefore, the total number of ways of selection of marbles (all of the same colour) $= 15 + 5 = 20$ .

23: In how many ways can a football team of $11$ players be selected from $16$ players? How many of them will:

(i) Include $2$ particular players.

Ans: Given: Total number of players $= 16$ .

Number of players to be selected $= 11$ .

To select any $11$ players out of a total of $16$ , use the formula $^{16} C_{11}$ .

use the formula $^{14} C_{9}$ to select the remaining $9$ players out of the remaining total of $14$ players.

The total number of ways to select any $11$ players out of a total of $16$ players $=^{16} C_{11} = 4368$ .

Since $2$ particular players are always included that means there are $9$ players left to be selected from a total of $14$ players as options.

Therefore, the number of ways of selection $=^{14} C_{9} = 2002$ .

(ii) Exclude $2$ particular players.

Ans: Given: Total number of players $= 16$ .

Number of players to be selected $= 11$ .

To select any $11$ players out of a total of $16$ , use the formula $^{16} C_{11}$ .

use the formula $^{14} C_{11}$ to select the $11$ players out of the remaining total of $14$ players.

The total number of ways to select any $11$ players out of a total of $16$ players $=^{16} C_{11} = 4368$ .

Since $2$ particular players are always excluded that means all the $11$ players are to be selected from a total of $14$ remaining players as options.

Therefore, the number of ways of selection $=^{14} C_{11} = 364$ .

24: A sports team of $11$ students is to be constituted, choosing at least $5$ from class XI and $5$ from class XII. If there are $20$ students in each of these classes, in how many ways can the team be constituted?

Ans : Given: Total number of students in class XI and XII each $= 20$ .

Number of students in the sports team $= 11$ .

At least $5$ students are to be selected from both the classes XI and XII.

Consider two cases: -

In the first case consider that $5$ players are chosen from class XI and $6$ from class XII.

In the second case consider that $5$ players are chosen from class XII and $6$ from class XI.

Since $11$ students are to be selected in combined from both the classes XI and XII and at least $5$ from each class, so the following two cases arise: -

(1) $5$ players are chosen from class XI and $6$ from class XII, so the total number of ways of selection $=^{20} C_{5} \times^{20} C_{6}$ .

(2) $5$ players are chosen from class XII and $6$ from class XI, so the total number of ways of selection $=^{20} C_{5} \times^{20} C_{6}$ .

Hence, the total number of ways of forming the team $=^{20} C_{5} \times^{20} C_{6} +^{20} C_{5} \times^{20} C_{6} = 2 (^{20} C_{5} \times^{20} C_{6})$ .

25: A group consists of $4$ girls and $7$ boys. In how many ways can a team of $5$ members be selected if the team has: -

(i) No girls.

Ans: Given: Total number of girls in the group $= 4$

Total number of boys in the group $= 7$

Number of persons to be selected $= 5$

use the formula $^{7} C_{5}$ to select all the boys.

Here all the members in the team should be a boy, so the total number of ways of selection $=^{7} C_{5} = 21$ .

(ii) At least one boy and one girl.

Ans: Given: Total number of girls in the group $= 4$

Total number of boys in the group $= 7$

Number of persons to be selected $= 5$

consider different cases where there is at least one boy and one girl present in the team in each case.

Here at least one boy and one girl should be present in the team, so the following cases can be considered: -

(a) One boy and four girls are selected, so the number of ways $=^{7} C_{1} \times^{4} C_{4} = 7$ .

(b) Two boys and three girls are selected, so the number of ways $=^{7} C_{2} \times^{4} C_{3} = 84$ .

(d) Four boys and one girl are selected, so the number of ways $=^{7} C_{4} \times^{4} C_{1} = 140$ .

Hence, the total number of ways of selection of the team $= 7 + 84 + 210 + 140 = 441$ .

(iii) At least three girls.

Ans: Given: Total number of girls in the group $= 4$

Total number of boys in the group $= 7$

Number of persons to be selected $= 5$

consider different cases where there is a minimum of three girls in the team in each case.

Here at least three girls should be present in the team, so the following cases can be considered: -

(a) Two boys and three girls are selected, so the number of ways $=^{7} C_{2} \times^{4} C_{3} = 84$ .

(b) One boy and four girls are selected, so the number of ways $=^{7} C_{1} \times^{4} C_{4} = 7$ .

Hence, the total number of ways of selection of the team $= 7 + 84 = 91$ .

26: If $^{n} C_{12} =^{n} C_{8}$ , then $n$ is equal to

(A) $20$

(B) $12$

(D) $30$

Ans: The correct answer is A.

Given: The expression $^{n} C_{12} =^{n} C_{8}$ .

Use the formula $^{n} C_{r} =^{n} C_{n - r}$ and substitute $r = 12$ to solve for the value of $n$ .

The given expression is $^{n} C_{12} =^{n} C_{8}$ . Using the formula $^{n} C_{r} =^{n} C_{n - r}$ in the R.H.S,

$\Rightarrow^{n} C_{12} =^{n} C_{n - 8}$

Comparing the expression on both the sides,

$\Rightarrow 12 = n - 8$

$\Rightarrow n = 20$

Correct Answer: A

27: The number of possible outcomes when a coin is tossed $6$ is

(A) $36$

(B) $64$

(D) $32$

Ans: The correct answer is B.

The correct answer is option B.

The possible outcomes when a coin is tossed one time are $2$ (heads or tails).

When a coin is tossed $n$ times then the total number of possible outcomes is given as $2^{n}$ , so here $n = 6$ .

Therefore, the total number of possible outcomes is $2^{6} = 64$ .

Correct Answer: B

28: The number of different four digit numbers that can be formed with the digits $2, 3, 4, 7$ and using each digit only once is

(A) $120$

(B) $96$

(D) $100$

Ans: The correct answer is C.

Given: The digits $2, 3, 4$ and $7$ .

Consider four boxes where four digits are to be filled with four different digits and find the number of ways to fill each box. Multiply all the number of ways.

Let us consider that there are four boxes and each box is to be filled with the digits $2, 3, 4$ and $7$ (no digits being repeated) to form a four digit number.

The first box can be filled in $4$ ways because there are four choices. Similarly, the second box can be filled in $3$ ways, the third box in $2$ ways and the last box in only $1$ ways because one digit will be left.

Therefore, the total number of different four digit numbers that can be formed $= 4 \times 3 \times 2 \times 1 = 24$ .

Correct Answer: C

29: The sum of the digits in unit place of all the numbers found with the help of $3, 4, 5$ and $6$ taken all at a time is

(A) $432$

(B) $108$

(D) $18$

Ans : The correct answer is B.

Given: The digits $3, 4, 5$ and $6$ .

Consider different cases where fix the unit place digit and find the number of ways to form different numbers. Take the sum of the unit place digits of all the numbers in all the cases.

The provided digits are $3, 4, 5$ and $6$ . So the following cases regarding the unit place digit can be considered: -

(1) When the unit place digit is fixed to be $3$ , so the tens, hundreds and thousands place digits can be filled in $3!$ ways.

Since the unit place of each digit is $3$ and the total number of different numbers formed is $3!$ , therefore the sum of the unit place digits $= 3! \times 3 = 18$ .

(2) When the unit place digit is fixed to be $4$ , so the tens, hundreds and thousands place digits can be filled in $3!$ ways.

Therefore the sum of the unit place digits $= 3! \times 4 = 24$ .

(3) When the unit place digit is fixed to be $5$ , so the tens, hundreds and thousands place digits can be filled in $3!$ ways.

Therefore the sum of the unit place digits $= 3! \times 5 = 30$ .

(4) When the unit place digit is fixed to be $6$ , so the tens, hundreds and thousands place digits can be filled in $3!$ ways.

Therefore the sum of the unit place digits $= 3! \times 6 = 36$ .

Hence, the sum of unit digits of all the different numbers formed $= 18 + 24 + 30 + 36 = 108$

Correct Answer: B

30: The number of words formed by $2$ vowels and $3$ consonants taken from $4$ vowels and $5$ consonants is equal to

(A) $60$

(B) $120$

(D) $720$

Ans: The correct answer is C.

Given: Total number of vowels $= 4$

Total number of consonants $= 5$

Number of vowels to be selected $= 2$

Number of consonants to be selected $= 2$

Use the formula $^{4} C_{2}$ to select $2$ vowels out of a total of $4$ and $^{5} C_{3}$ to select $3$ consonants out of a total of $5$ .

Now, arrange the $5$ letters in $5!$ ways.

Since $2$ vowels are to be selected out of a total of $4$ , so the total number of ways of selection $=^{4} C_{2}$ .

Also, $3$ consonants are to be selected out of a total of $5$ , so the total number of ways of selection $=^{5} C_{3}$ .

Now, the $5$ letters selected can be arranged among themselves in $5!$ ways.

Hence, the total number of different words that can be formed $=^{4} C_{2} \times^{5} C_{3} \times 5! = 7200$ .

Correct Answer: C

31: A five digit number divisible by $3$ is to be formed using the numbers $0, 1, 2, 3, 4$ and $5$ without repetitions. The total number of ways this can be done is

(A) $216$

(B) $600$

(D) $3125$

Ans : The correct answer is A.

Given: The digits $0, 1, 2, 3, 4$ and $5$ .

Consider different cases where the sum of the selected digits is divisible by $3$ so that the five digit number is divisible by $3$ .

Add the total number of five digit numbers obtained in all cases.

For a number to be divisible by $3$ , the sum of all the digits of that number must be divisible by $3$ . So the following cases where the sum of digits is $3$ can be considered:

(1) The digits selected are $0, 1, 2, 4$ and $5$ . Since $0$ cannot be placed as the leftmost digit because if that happens then the number will be of four digits only.

So the leftmost digit can be chosen amongst the four digits $1, 2, 4$ or $5$ . Now, the next four digits can be chosen and arranged in $4!$ ways.

Therefore, the total number of five digit numbers $= 4 \times 4!$ .

(2) The digits selected are $1, 2, 3, 4$ and $5$ . Here the five selected digits can be arranged among themselves in $5!$ ways.

Hence, the total number of five digit numbers (without repetition), divisible by $3$ , that can be formed $= (4 \times 4!) + 5! = 216$ .

Correct Answer: A

32: Everybody in a room shakes hands with everybody else. The total number of handshakes is $66$ . The total number of persons in the room is

(A) $11$

(B) $12$

(D) $14$

Ans: The correct answer is B.

Given: Total number of handshakes $= 66$ .

Assume the total number of persons as $n$ . Use the formula, total number of handshakes $=^{n} C_{2}$ to solve for the value of $n$ .

For a handshake to a person there must be two persons, so the total number of handshakes will be equal to the total number of ways of selecting any two persons.

Let us assume the total number of persons in the room is $n$ so no of ways to select any two of them will be $^{n} C_{2}$ .

$\Rightarrow$ Number of handshakes $=^{n} C_{2}$

$\Rightarrow 66 = \frac{n (n - 1)}{2}$

$\Rightarrow n^{2} - n - 132 = 0$

Using the middle term split method,

$\Rightarrow (n - 12) (n + 11) = 0$

$\Rightarrow n = 12$ or $- 11$

Since the total number of persons cannot be negative so $n = - 11$ is not possible. Hence, $n = 12$ .

Correct Answer: B

33: The number of triangles that are formed by choosing the vertices from a set of $12$ points, seven of which lie on the same line is

(A) $105$

(B) $15$

(D) $185$

Ans : The correct answer is D.

Given: Total number of points $= 12$

Total number of collinear points $= 7$ .

Form three cases: -

In the first case assume that all the three vertices are taken from the set of non – collinear points.

In the second case assume that two vertices are taken from the set of non – collinear points and one vertex from the set of collinear points.

In the third case assume that one vertex is taken from the set of non – collinear points and two vertices from the set of collinear points.

There are $12$ points and $7$ are collinear that means $5$ are non – collinear. To form a triangle three non – collinear points are required, so the following three cases can arise: -

(1) All the three vertices are taken from the set of non – collinear points, so the number of triangles $^{5} C_{3} = 10$ .

(2) Two vertices are taken from the set of non – collinear points and one vertex from the set of collinear points. So the number of triangles $=^{5} C_{2} \times^{7} C_{1} = 70$ .

(3) One vertex is taken from the set of non – collinear points and two vertices from the set of collinear points. So the number of triangles $=^{5} C_{1} \times^{7} C_{2} = 105$ .

Hence, the total number of triangles that can be formed $= 10 + 70 + 105 = 185$ .

Correct Answer: D

34: The number of parallelograms that can be formed from a set of four parallel lines intersecting another set of three parallel lines is

(A) $6$

(B) $18$

(D) $9$

Ans : The correct answer is D.

Given: Set of four parallel lines intersecting another set of three parallel lines.

Select two parallel lines from each set of intersecting parallel lines and multiply the number of selections.

To form a parallelogram two sets of parallel intersecting another two sets of parallel lines is required. So the total number of parallelograms that can be formed will be equal to the number of ways to select two parallel lines from each set.

From the set of four parallel lines two lines can be selected in $=^{4} C_{2} = 6$ ways.

From the set of three parallel lines two lines can be selected in $=^{3} C_{2} = 3$ ways.

Therefore, the number of parallelograms that can be formed $= 6 \times 3 = 18$ .

Correct Answer: D

35: The number of ways in which a team of eleven players can be selected from $22$ players always including $2$ of them and excluding $4$ of them is

(A) $^{16} C_{11}$

(B) $^{16} C_{5}$

(D) $^{20} C_{9}$

Ans : The correct answer is C.

Given: Total number of players available $= 22$

Number of payers to be selected $= 11$

Number of players always included $= 2$

Number of players always excluded $= 4$

Use the formula $^{16} C_{9}$ to select the remaining $9$ players out of a total of $16$ players.

Since there are $22$ players and $11$ are to be selected and in that $2$ particular players are already included and $4$ excluded. That means the remaining $9$ players are left to be chosen from a total of $(22 - 2 - 4) = 16$ available players as options.

Therefore, the total number of ways of selection of the team $=^{16} C_{9}$ .

Correct Answer: C

36: The number of $5 -$ digit telephone numbers having at least one of their digits repeated is

(A) $90000$

(B) $10000$

(D) $69760$

Ans : The correct answer is D.

Given: A telephone number made up of five digits.

Consider that all the digits can be repeated and find the number of telephone numbers. Now, assume that no digit can be repeated and find the number of telephone numbers that can be formed.

Subtract the number obtained in the later assumption from the former one.

There are only five digits in the telephone number. In case all the digits can be repeated then the number of telephone numbers that can be formed is $= 10^{5}$ . This is because there are ten digits from $0$ to $9$ and each digit can be repeated five times.

If no digits are repeated then the number of telephone numbers that can be formed $=^{10} P_{5}$ . This is because five different digits are to be selected and arranged.

Therefore, the number of telephone numbers having at least one of its digit repeated $= (10^{5} -^{10} P_{5}) = 69760$ .

Correct Answer: D

37: The number of ways in which we can choose a committee from four men and six women so that the committee includes at least two men and exactly twice as many women as men is

(A) $94$

(B) $126$

(D) None

Ans: The correct answer is A.

Given: Total number of men $= 4$ .

Total number of women $= 6$ .

Consider two different cases: -

In the first case assume there are two men and four women.

In the first case assume there are three men and six women.

The number of men should be at least two and the number of women must be twice the number of men. So the following cases arise: -

(1) There are two men (out of four) and four women (out of six) selected. Therefore, the number of ways to select them $=^{4} C_{2} \times^{6} C_{4} = 90$ .

(2) There are three men (out of four) and six women (out of six) selected. Therefore, the number of ways to select them $=^{4} C_{3} \times^{6} C_{6} = 4$ .

Hence, the number of ways to form the committee $= 90 + 4 = 94$ .

Correct Answer: A

38: The total number of $9$ digit numbers which have all different digits is

(A) $10!$

(B) $9!$

(D) $10 \times 10!$

Ans : The correct answer is C.

Given: The $9$ digit numbers with all their digits different.

Consider that there are nine boxes to be filled with nine different digits. Using the condition that the leftmost place cannot be filled with the digit $0$ , find the number of ways to fill the remaining boxes.

Since $9$ digit numbers (no repetition) are formed, any nine digits can be chosen out of the available ten digits from $0$ to $9$ . Assume that there are nine boxes to be filled with nine different digits.

Now, the leftmost place (box) cannot be filled with $0$ otherwise the number would be of only $8$ digits. So there are $9$ choices to fill the leftmost box.

After the leftmost box is filled we still have $9$ choices to fill the next box because now $0$ can be filled at any place. So the remaining eight boxes can be filled in $9 \times 8 \times 7 \times 6 \times 5 \times 4 \times 3 \times 2$ ways. This can be written as $9 \times 8 \times 7 \times 6 \times 5 \times 4 \times 3 \times 2 = 9!$ .

Hence, the total number of $9$ digit numbers that can be formed is $= 9 \times 9!$ .

Correct Answer: C

39: The number of words which can be formed out of the letters of the word ARTICLE, so that vowels occupy the even place is

(A) $1440$

(B) $144$

(D) $^{4} C_{4} \times^{3} C_{3}$

Ans : The correct answer is B.

Given: The word ARTICLE.

First arrange all the consonants of the given word. Now, arrange the vowels between these consonants.

There are three vowels (A, E, I) and four consonants (C, L, R, T) in the word article. Since vowels have to occupy the even places so the word will start with a consonant.

Seven places are to be filled and at positions $1, 3, 5$ and $7$ there will be consonants. Therefore, the number of ways to arrange four consonants at four places $= 4! = 24$ .

Now, the positions $2, 4$ and $6$ will be filled with vowels. Therefore, the number of ways to arrange four consonants at four places $= 3! = 6$ .

Hence, the total number of words that can be formed $= 24 \times 6 = 144$ .

Correct Answer: B

40: Given $5$ different green dyes, four different blue dyes and three different red dyes, the number of combination of dyes which can be chosen taking at least one green and one blue dye is

(A) $3600$

(B) $3720$

(D) $3700$

Ans : The correct answer is B.

Given: Total number of green dyes $= 5$

Total number of blue dyes $= 4$

Total number of red dyes $= 3$

Consider $2^{5}$ , $2^{4}$ and $2^{3}$ as the number of ways of choosing or not choosing a green dye, blue dye and red dye respectively. Now, subtract $1$ from $2^{5}$ and $2^{4}$ each to find the number of ways of choosing at least one green and blue dye respectively.

Since there are $2$ choices either choosing or not choosing a particular dye. So there are the following conclusions: -

Number of ways of choosing $5$ green dyes $= 2 \times 2 \times 2 \times 2 \times 2 = 2^{5}$

Number of ways of choosing $4$ blue dyes $= 2 \times 2 \times 2 \times 2 = 2^{4}$

Number of ways of choosing $3$ red dyes $= 2 \times 2 \times 2 = 2^{3}$

At least one green and one blue dye are to be selected. The number of ways in which no green dye will be selected will be $1$ and similarly the number of ways in which no blue dye will be selected will also be $1$ .

Therefore, the number of ways in which at least one green dye is selected will be $(2^{5} - 1)$ and the number of ways in which at least one blue dye is selected will be $(2^{4} - 1)$ .

Hence, the number of combinations of dyes that can be chosen $= 2^{3} \times (2^{5} - 1) \times (2^{4} - 1) = 3720$ .

Correct Answer: B

Fill in the Blanks in Exercises $41$ to $50$ :

41: If $^{n} P_{r} = 840$ and $^{n} C_{r} = 35$ , then $r =$ _____.

Ans : Given: $^{n} P_{r} = 840$

$^{n} C_{r} = 35$

Divide $^{n} P_{r}$ by $^{n} C_{r}$ and use the formulas $^{n} P_{r} = \frac{n!}{(n - r)!}$ and $^{n} C_{r} = \frac{n!}{r! (n - r)!}$ to evaluate.

Dividing $^{n} P_{r}$ by $^{n} C_{r}$ ,

$\Rightarrow \frac{^{n} P_{r}}{^{n} C_{r}} = \frac{840}{35}$

$\Rightarrow \frac{^{n} P_{r}}{^{n} C_{r}} = 24$

Using the formulas $^{n} P_{r} = \frac{n!}{(n - r)!}$ and $^{n} C_{r} = \frac{n!}{r! (n - r)!}$ ,

$\Rightarrow \frac{\frac{n!}{(n - r)!}}{\frac{n!}{r! (n - r)!}} = 24$

$\Rightarrow r! = 4!$

On comparing both the sides,

$r = 4$

42: $^{15} C_{8} +^{15} C_{9} -^{15} C_{6} -^{15} C_{7} =$ _______.

Ans : Given: The expression $^{15} C_{8} +^{15} C_{9} -^{15} C_{6} -^{15} C_{7}$ .

Take the terms $(^{15} C_{8} +^{15} C_{9})$ together and $(^{15} C_{6} +^{15} C_{7})$ , use the formula $^{n} C_{r - 1} +^{n} C_{r} =^{n + 1} C_{r}$ to evaluate. Now, use the relation $^{n} C_{r} =^{n} C_{n - r}$ to simplify.

The provided expression is $^{15} C_{8} +^{15} C_{9} -^{15} C_{6} -^{15} C_{7}$ .

$\Rightarrow^{15} C_{8} +^{15} C_{9} -^{15} C_{6} -^{15} C_{7} = (^{15} C_{8} +^{15} C_{9}) - (^{15} C_{6} +^{15} C_{7})$

Using the formula $^{n} C_{r - 1} +^{n} C_{r} =^{n + 1} C_{r}$ ,

$\Rightarrow^{15} C_{8} +^{15} C_{9} -^{15} C_{6} -^{15} C_{7} = (^{16} C_{9}) - (^{16} C_{7})$

Now, using the relation $^{n} C_{r} =^{n} C_{n - r}$ for the term $^{16} C_{7}$ ,

$\Rightarrow^{15} C_{8} +^{15} C_{9} -^{15} C_{6} -^{15} C_{7} = (^{16} C_{9}) - (^{16} C_{16 - 7})$

$\Rightarrow^{15} C_{8} +^{15} C_{9} -^{15} C_{6} -^{15} C_{7} = (^{16} C_{9}) - (^{16} C_{9})$

$\Rightarrow^{15} C_{8} +^{15} C_{9} -^{15} C_{6} -^{15} C_{7} = 0$

43: The number of permutations of $n$ different objects, taken $r$ at a time, when repetitions are allowed, is _____.

Ans : Given: Total number of objects $= n$

Number of objects taken at a time $= r$

Repetitions are allowed.

The total number of permutations of $n$ different objects taken $r$ at a time when repetitions are allowed is given as $n^{r}$ .

Think that there $r$ spaces which are to be filled with $n$ different letters and repetitions are allowed. So there are $n$ choices to fill each of the spaces.

Therefore, the total number of permutations will be the product $n \times n \times n . . .$ where $n$ appears $r$ times. So, the product is $n^{r}$ .

Hence, the total number of permutations is $n^{r}$ .

44: The number of different words that can be formed from the letters of the word INTERMEDIATE such that two vowels never come together is _____.

Ans : Given: The word INTERMEDIATE.

First arrange all the consonants and then place the vowels between them such that no two vowels are placed adjacent to each other.

The consonants in the word INTERMEDIATE are D, M, N, R and T where T is repeated twice. Now, the vowels are A, E and I where E is repeated thrice and I is repeated twice.

First arranging the consonants,

_ D _ M _ N _ R _ T _ T _

The six consonants can be arranged among themselves in $6!$ ways but since T is repeated twice so the effective number of arrangements will be $\frac{6!}{2!}$ .Now, the six vowels can be arranged at seven blank spaces in $^{7} P_{6}$ ways, however E is repeated thrice and I is repeated twice, so effective number of arrangements $= \frac{^{7} P_{6}}{2! \times 3!}$ .

Hence, the total number of words that can be formed $= \frac{^{7} P_{6}}{2! \times 3!} \times \frac{6!}{2!} = 151200$ .

45: Three balls are drawn from a bag containing $5$ red, $4$ white and $3$ black balls. The number of ways in which this can be done if at least $2$ are red is _____.

Ans : Given: Total number of red balls $= 5$

Total number of white balls $= 4$

Total number of black balls $= 3$

Number of balls drawn $= 3$

Consider two different cases: -

In the first case assume two red balls are drawn and the remaining one ball drawn is of any colour (white or black).

In the second case assume that all the three balls drawn are red.

Since three balls are drawn in which at least two balls will be red. So the following two cases arise: -

(1) Two balls drawn are red and one ball drawn is either white or black. There are $5$ red balls and the sum total of white and black balls is $7$ .

Therefore, the number of ways to draw the balls $=^{5} C_{2} \times^{7} C_{1} = 70$ .

(2) All the three balls drawn are red.

Therefore, the number of ways to draw the balls $=^{5} C_{3} = 10$ .

Hence, the total number of ways to draw at least two red balls $= 70 + 10 = 80$ .

46: The number of six digit numbers, all digits of which are odd is _____.

Ans : Given: A six digit number is to be formed and the digits are to be odd.

Assume that there are six boxes to be filled with the digits $1, 3, 5, 7$ or $9$ and repetitions are allowed.

Since a six digit number is to be formed whose all the digits should be odd that means the choices are $1, 3, 5, 7$ or $9$ .

Suppose there are six boxes to be filled with $5$ digits and repetitions are allowed, so to fill each box there are $6$ choices. Therefore, the number of ways in which all the boxes can be filled $= 5^{6}$ .

Hence, the total number of six digit numbers that can be formed is $= 5^{6} = 15625$ .

47: In a football championship, $153$ matches were played. Every two teams played one match with each other. The number of teams participating in the championship is ______.

Ans : Given: Total number of matches played $= 153$

Assume that there are $n$ teams. Use the formula, the total number of matches played $=^{n} C_{2}$ to solve for the value of $n$ .

Let us assume that there are $n$ teams participating in the championship.

For a match to occur there must be two teams, so the total number of matches played will be equal to the number of ways in which any two teams can be selected which is given as $^{n} C_{2}$ .

$\Rightarrow$ Total number of matches played $=^{n} C_{2}$

$\Rightarrow 153 = \frac{n (n - 1)}{2}$

$\Rightarrow n^{2} - n - 306 = 0$

Using the middle term split method,

$\Rightarrow (n - 18) (n + 17) = 0$

$\Rightarrow n = 18$ or $- 17$ .

Here $n = - 17$ is not possible because the number of teams cannot be negative. Hence, $n = 18$ .

48: The total number of ways in which six $^{'} +^{'}$ and four $^{'} -^{'}$ signs can be arranged in a line such that no two $^{'} -^{'}$ signs occur together is _____.

Ans : Given: Total number of $^{'} +^{'}$ signs $= 6$

Total number of $^{'} -^{'}$ signs $= 4$

First arrange all the $^{'} +^{'}$ signs and then arrange the $^{'} -^{'}$ signs such that no two of them are present side by side.

Since no two $^{'} -^{'}$ signs should be present side by side, so first let us arrange all the $^{'} +^{'}$ signs.

_ $+$ _ $+$ _ $+$ _ $+$ _ $+$ _ $+$ _

As it can be seen that there are seven blank spaces left where $^{'} -^{'}$ signs so that they will not occur together. Therefore, any of the four blank spaces is to be selected.

The total number of selections of four spaces out of seven $=^{7} C_{4} = 35$ .

49: A committee of $6$ is to be chosen from $10$ men and $7$ women so as to contain at least $3$ men and $2$ women. In how many different ways can this be done if two particular women refuse to serve on the same committee.

Ans: Given: Total number of men $= 10$

Total number of women $= 7$

Number of member to be chosen $= 6$

Minimum number of men $= 3$

Minimum number of women $= 2$

Consider two cases: -

In the first case assume that $3$ men and $3$ women are selected.

In the second case assume that $4$ men and $2$ women are selected.

Since at least $3$ men (out of $10$ ) and $2$ women (out of $7$ ) are to be selected in a committee of $6$ persons. So the following two cases arise: -

(1) $3$ men and $3$ women are selected. Men can be selected in $^{10} C_{3}$ ways. Since out of $7$ women, $2$ women refuse to serve in the same committee, two sub cases may arise: -

(a) It may be possible that those two women are not selected, so the number of ways to select the three women out of the remaining five is $=^{5} C_{3}$ .

(b) One of them is selected in $^{2} C_{1}$ ways and the remaining two women are selected amongst the remaining five in $^{5} C_{2}$ ways. Therefore, the total number of ways is $^{2} C_{1} \times^{5} C_{2}$ .

So, the overall ways to form the committee for case (1) $=^{10} C_{3} (^{5} C_{3} +^{2} C_{1} \times^{5} C_{2}) = 3600$ .

(2) $4$ men and $2$ women are selected. Here also two sub cases may arise: -

(a) Those two women are not selected, so the number of ways to select the two women out of the remaining five is $=^{5} C_{2}$ .

(b) One of them is selected in $^{2} C_{1}$ ways and the remaining one woman is selected amongst the remaining five in $^{5} C_{1}$ ways. Therefore, the total number of ways is $^{2} C_{1} \times^{5} C_{1}$ .

So, the overall ways to form the committee for case (2) $=^{10} C_{3} (^{5} C_{2} +^{2} C_{1} \times^{5} C_{1}) = 4200$ .

Hence, the total number of ways to form the committee $= 3600 + 4200 = 7800$ .

50: A box contains $2$ white balls, $3$ black balls and $4$ red balls. The number of ways three balls can be drawn from the box if at least one black is to be included in the draw is _____.

Ans : Given: Total number of white balls $= 2$

Total number of black balls $= 3$

Total number of red balls $= 4$

Number of balls drawn $= 3$

Consider three different cases: -

In the first case assume one black ball is drawn and the remaining two balls drawn are of any colour (white or red).

In the second case assume two black balls are drawn and the remaining one ball drawn is of any colour (white or red).

In the third case assume that all the three balls drawn are black.

Since three balls are drawn in which at least one will be black. So the following three cases arise: -

(1) One ball drawn is black and the remaining two balls drawn are either white or red. There are $3$ black balls and the sum total of white and red balls is $6$ .

Therefore, the number of ways to draw the balls $=^{3} C_{1} \times^{6} C_{2} = 45$ .

(2) Two balls drawn are black and the remaining two balls drawn are either white or red.

Therefore, the number of ways to draw the balls $=^{3} C_{2} \times^{6} C_{1} = 18$ .

(3) All the three balls drawn are black.

Therefore, the number of ways to draw the balls $=^{3} C_{3} = 1$ .

Hence, the total number of ways to draw at least one black ball $= 45 + 18 + 1 = 64$ .

State whether the statements in Exercises from $51$ to $59$ True or False? Also given justification:

51: There are $12$ points in a plane of which $5$ points are collinear, then the number of lines obtained by joining these points in pairs is $^{12} C_{2} -^{5} C_{2}$ .

Ans : The given statement is false.

To form a straight line $2$ points are required, so the number of straight lines that would have been formed if all the $12$ points were non – collinear $=^{12} C_{2}$ .

Since $5$ points among $12$ points are collinear, that means they lie on a single straight line, so those $5$ points will form the $^{5} C_{2}$ number of the same straight lines. Overall the number of distinct straight lines formed by $5$ collinear points will be $1$ .

Therefore, the total number of distinct straight lines that will be formed by the given $12$ points will be $^{12} C_{2} -^{5} C_{2} + 1$ .

52: Three letters can be posted in five letterboxes in $3^{5}$ ways.

Ans : The given statement is false.

Here it is possible that all the three letters can be posted in the same letterbox. So there are $5$ choices in which the first letter can be posted.

Similarly, there are $5$ choices for each of the first, second and third letters to be posted. Therefore, the total number of ways to post the letters are $5 \times 5 \times 5 = 5^{3}$ .

53: In the permutations of $n$ distinct things, $r$ taken together, the number of permutations in which $m$ particular things occur together is $^{n - m} P_{r - m} \times^{r} P_{m}$ .

Ans : The given statement is false.

Since from a total of $n$ things, $r$ things are to be taken and $m$ things are always together. That means those $m$ things will always be selected.

Therefore, now $(r - m)$ things are left to be selected from a total of $(n - m)$ things. So the number of ways of selection $=^{n - m} C_{r - m}$ .

The $m$ things which are together can be arranged among themselves in $m!$ ways.

Now, these $m$ things can be considered as a single unit and so $(r - m + 1)$ things are left to be arranged. So the number of ways of arrangement $= (r - m + 1)!$ .

Hence, the total number of permutations $=^{n - m} C_{r - m} \times m! \times (r - m + 1)!$ .

54: In a steamer there are stalls for $12$ animals, and there are horses, cows and calves (not less than $12$ each) ready to be shipped. They can be loaded in $3^{12}$ ways.

Ans : The given statement is true.

It can be assumed that there are $12$ spaces to be filled and each space can be filled in $3$ ways (either with horses, cows or calves).

All the animals cannot be filled in one stall. So the total number of ways to fill the $12$ spaces $= 3^{12}$ .

55: If some or all of $n$ objects are taken at a time, the number of combinations is $2^{n} - 1$ .

Ans : The given statement is true.

There are two choices for each object, either it gets selected or not selected. So the total number of combinations for $n$ object of either getting selected or not will be $2 \times 2 \times 2 . . . .$ where $2$ occurs $n$ times, i.e. the product is $2^{n}$ .

Now, one combination will be such that none of the objects got selected, so the total number of ways to select some or all of $n$ objects will be $2^{n} - 1$ .

56: There will be only $24$ selections containing at least one red ball out of a bag containing $4$ red balls and $5$ black balls. It is being given that the balls of the same colour are identical.

Ans : The given statement is true.

Since at least one red ball is to be selected that means either $1$ or $2$ or $3$ or all the $4$ red balls can be selected. So the number of choices to select at least one red ball $= 4$ .

Now, nothing is given about the black balls, so it may be possible that $0$ or $1$ or $2$ or $3$ or $4$ or all the $5$ black balls are selected. So the number of choices to select or not select the black balls is $= 6$ .

Hence, the total number of ways $= 4 \times 6 = 24$ .

57: Eighteen guests are to be seated, half on each side of a long table. Four particular guests desire to sit on one particular side and three others on the other side of the table. The number of ways in which the seating arrangements can be made is $\frac{11!}{5! 6!} (9!) (9!)$ .

Ans: The given statement is true.

$9$ guests are to be seated on each side of the table so the number of arrangements of $9$ persons on both sides will be $9! \times 9!$ .

Since $4$ particular guests desired to sit on one side and $3$ particular guests on the other side that means $11$ guests are yet to be arranged on either side. So the remaining $5$ guests on the former side can be chosen among $11$ remaining guests in $^{11} C_{5}$ . The remaining $6$ guests will automatically get shifted to the later side.

Therefore, the number of arrangements $=^{11} C_{5} \times 9! \times 9! = \frac{11!}{5! 6!} (9!) (9!)$ .

Exercise 58: A candidate is required to answer $7$ questions out of $12$ questions which are divided into two groups, each containing $6$ questions. He is not permitted to attempt more than $5$ questions from either group. He can choose the $7$ questions in $650$ ways.

Ans : The given statement is false.

The following cases for attempting the questions from the two groups are possible: -

(1) $2$ questions from one of the groups and $5$ questions from the other.

The number of ways in which one of the groups can be selected is $^{2} C_{1}$ and the questions can be selected in $^{6} C_{2} \times^{6} C_{5}$ ways.

Therefore, total number of ways $=^{2} C_{1} \times^{6} C_{2} \times^{6} C_{5} = 180$ .

(2) $3$ questions from one of the groups and $4$ questions from the other.

Here, the number of ways to select one of the groups is $^{2} C_{1}$ and the questions can be selected in $^{6} C_{3} \times^{6} C_{4}$ ways.

Therefore, total number of ways $=^{2} C_{1} \times^{6} C_{3} \times^{6} C_{4} = 600$ .

Hence, the total number of ways $= 180 + 600 = 780$ .

59: To fill $12$ vacancies there are $25$ candidates of which $5$ are from scheduled castes. If $3$ of the vacancies are reserved for scheduled caste candidates while the rest are open to all, the number of ways in which the selection can be made is $^{5} C_{3} \times^{20} C_{9}$ .

Ans : The given statement is true.

Since $5$ candidates are from scheduled castes and $3$ vacancies are reserved for then so for $3$ vacancies the number of ways in which $5$ candidates can be selected $=^{5} C_{3}$ .

Now, for the remaining $9$ vacancies, $20$ candidates are eligible, so they can be selected in $^{20} C_{9}$ .

Hence, the total number of ways $=^{5} C_{3} \times^{20} C_{9}$ .

In each of the Exercises from $60$ to $64$ match each item given under the column $C_{1}$ to its correct answer given under the column $C_{2}$ :

60: There are $3$ books of Mathematics, $4$ of Physics and $5$ of English. How many different collections can be made such that each collection consists of: -

$C_{1}$	$C_{2}$
(a) One book of each subject :	(i) $3968$
(b) At least one book of each subject :	(ii) $60$
(c) At least on book of English :	(iii) $3255$

Ans: (a) Any one of the three Mathematics books, any one of the four Physics books and any one of the five English books can be selected. So, one book of each subject can be selected in $^{3} C_{1} \times^{4} C_{1} \times^{5} C_{1} = 60$ ways.

(b) There are $2^{3}$ , $2^{4}$ and $2^{5}$ combinations to either select or not select the Mathematics, Physics and English books respectively. For each of the books there will be one such combination where no book is selected.

So, there are $(2^{3} - 1)$ , $(2^{4} - 1)$ and $(2^{5} - 1)$ combinations to select at least one book of Mathematics, Physics and English books respectively.

Therefore, the total number of ways to select at least one book of each subject $= (2^{3} - 1) (2^{4} - 1) (2^{5} - 1) = 3255$ .

(c) Here Mathematics and Physics books may or may not be selected but at least one English book is to be selected, so the total number of ways $= (2^{3}) (2^{4}) (2^{5} - 1) = 3968$ .

Hence, the correct match is (a) – (ii), (b) – (iii), (c) – (i).

61: Five boys and five girls form a line. Find the number of ways of making the seating arrangement under the following condition: -

$C_{1}$	$C_{2}$
(a) Boys and girls alternate :	(i) $5! \times 6!$
(b) No two girls sit together :	(ii) $10! - 5! 6!$
(c) All the girls sit together :	(iii) ${(5!)}^{2} + {(5!)}^{2}$
(d) All the girls are never together :	(iv) $2! 5! 5!$

Ans : (a) Let us first arrange the five boys.

_ B _ B _ B _ B _ B _

Since the boys and girls are to be alternate, either the first five positions will be filled or the last five positions. So there are $2$ ways to select the five positions. Now, the five girls and five boys can be arranged in $5!$ ways each.

Therefore, the total number of arrangements $= 2 \times 5! \times 5! = 2 \times {(5!)}^{2} = {(5!)}^{2} + {(5!)}^{2}$ .

(b) In this condition no two girls sit together that means the five girls can be arranged at any $5$ of the $6$ positions shown. There are $^{6} P_{5}$ ways to select the $5$ positions and arrange all the girls. Now, the five boys can be arranged among themselves in $5!$ ways.

Therefore, the total number of arrangements $=^{6} P_{5} \times 5! = 6! \times 5!$ .

(c) All the five girls must sit together, which means they can be considered as one unit. They can be arranged among themselves in $5!$ ways. Now, the five boys and the single unit of girls can be counted as six beings which can be arranged in $6!$ ways.

Therefore, the total number of arrangements $= 5! \times 6!$ .

(d) The number of arrangements where all the girls are never together will be the difference between the total number of arrangements (without any condition) and the number of arrangements where all the girls are together.

Since there are $10$ persons in total (five boys and five girls) , they can be arranged in $10!$ ways without any conditions.

Therefore, the number of arrangements where all the girls are never together $= 10! - 5! 6!$ .

Hence, the correct match is (a) – (iii), (b) – (i), (c) – (i), (d) – (ii).

62: There are $10$ professors and $20$ lecturers out of whom a committee of $2$ professors and $3$ lecturer is to be formed. Find: -

$C_{1}$	$C_{2}$
(a) In how many ways committee can be formed	(i) $^{10} C_{2} \times^{19} C_{3}$
(b) In how many ways a particular professor is included	(ii) $^{10} C_{2} \times^{19} C_{2}$
(c) In how many ways a particular lecturer is included	(iii) $^{9} C_{1} \times^{20} C_{3}$
(d) In how many ways a particular lecturer is excluded	(iv) $^{10} C_{2} \times^{20} C_{3}$

Ans: (a) $2$ professors out of a total of $10$ can be selected in $^{10} C_{2}$ ways and $3$ lecturer out of a total of $20$ lecturers can be selected in $^{20} C_{3}$ ways.

Therefore, the total number of ways $=^{10} C_{2} \times^{20} C_{3}$ .

(b) $1$ particular professor is already included, that means the remaining $1$ professors out of a total of remaining $9$ can be selected in $^{9} C_{1}$ ways. The number of ways to select the $3$ lecturer will be $^{20} C_{3}$ .

Therefore, the total number of ways $=^{9} C_{1} \times^{20} C_{3}$ .

(c) $1$ particular lecturer is already included, which means the remaining $2$ lecturer out of a total of remaining $19$ can be selected in $^{19} C_{2}$ ways. The number of ways to select the $2$ professors will be $^{10} C_{2}$ .

Therefore, the total number of ways $=^{19} C_{2} \times^{10} C_{2}$ .

(d) $1$ particular lecturer is already excluded, that means the $3$ lecturer out of a total of remaining $19$ can be selected in $^{19} C_{3}$ ways. The number of ways to select the $2$ professors will be $^{10} C_{2}$ .

Therefore, the total number of ways $=^{10} C_{2} \times^{19} C_{3}$ .

Hence, the correct match is (a) – (iv), (b) – (iii), (c) – (ii), (d) – (i).

63: Using the digits $1, 2, 3, 4, 5, 6, 7$ , a number of $4$ different digits is formed. Find: -

$C_{1}$	$C_{2}$
(a) how many numbers are formed?	(i) $840$
(b) how many numbers are exactly divisible by $2$ ?	(ii) $200$
(c) how many numbers are exactly divisible by $25$ ?	(iii) $360$
(d) how many numbers are exactly divisible by $4$ ?	(iv) $40$

Ans: (a) $4$ different digits out of a total of seven given digits can be selected and arranged in $^{7} P_{4} = 840$ ways.

(b) The numbers that are divisible by $2$ must contain $2, 4$ or $6$ at the unit place. So there are three choices for the unit place digit. Now, the remaining three places can be filled by the remaining six digits in $^{6} P_{3}$ ways.

Therefore, the total number of ways $= 3 \times^{6} P_{3} = 360$ .

(c) The numbers that are divisible by $25$ must have their last two digits, a multiple of $25$ . So the suitable combinations are $25$ and $75$ . Now, the remaining two places can be filled by the remaining five digits in $^{5} P_{2}$ ways.

Therefore, the total number of ways $= 2 \times^{5} P_{2} = 40$ .

(d) The numbers that are divisible by $4$ must have their last two digits a multiple of $4$ . So the suitable combinations are $12, 16, 24, 32, 36, 52, 56, 64, 72$ and $76$ . The remaining two places can be filled by the remaining five digits in $^{5} P_{2}$ ways.

Therefore, the total number of ways $= 10 \times^{5} P_{2} = 200$ .

Hence, the correct match is (a) – (i), (b) – (iii), (c) – (iv), (d) – (ii).

64: How many words (with or without dictionary meaning) can be made from the letters of the word MONDAY, assuming that no letter is repeated, if: -

$C_{1}$	$C_{2}$
(a) $4$ letters are used at a time	(i) $720$

(b) All letters are used at a time	(ii) $240$
(c) At letters are used but the first is a vowel	(iii) $360$

Ans: (a) There are $^{6} P_{4}$ ways to select and arrange the four different letters of the MONDAY. So the total number of words formed $=^{6} P_{4} = 360$ .

(b) $6$ letters can be arranged among themselves in $6!$ ways. Therefore, the number of words $= 6! = 720$ .

(c) The vowels in the given word are A and O, so there are two choices to fill the first letter. Now, the remaining $5$ places can be filled in $5!$ ways. So the total number of words formed $= 2 \times 5! = 240$ .

Hence, the correct match is (a) – (iii), (b) – (i), (c) – (ii).

Learn Chapter 7 with Vedantu

The chapter 7 of Class 11 Maths discusses the concepts of permutation and combination. The topics included in the chapter are easy but it is only with proper guidance that students can ace the questions asked in the exam from this chapter. Vedantu provides exemplary solutions for the same reason.

The aim of the Vedantu's experts is to keep the students at ease and help them comprehend the concepts of combinations and permutation, the properties, and a few other crucial formulas. The provided solutions are not only useful in clearing the doubts of the students but they are also able to prepare the subject more efficiently.

Vedantu is ready to help students learn permutations of both types with repetition and without repetition. Similarly, you will get a detailed guide for both types of combinations, with repetition and without repetition.

FAQs on NCERT Exemplar for Class 11 Maths Chapter 7 - Permutations and Combinations (Book Solutions)

1. How to search for the integers number which is greater than 7000? Also, this could be formed with the digits 5, 3, 8, 7, and 9? Here any digit does not repeat!

The integers number, which is greater than 7000 could be solved in the following way: We need to ensure that we can create a maximum 5 digit number as we are not allowed to repeat any digit. Because all the provided 5 digits are greater than 7000, so we will get a 5 digit integers number that will be = 5x4x3x2x1 = 120. Similarly, a 4 digit integer will be greater than 7000 in case a thousand places contains any one of 9, 7, or 8. Hence, the place of the thousandth could be filled in 3 different ways. The three places that remained could be filled with the other four digits in a 4P3 manner. Thus, the total of four digit integers can be calculated as 3x 4P3 = 3x4x3x2 = 72 Total number of integers = 120 + 72 = 192.

2. What topics am I going to learn in NCERT Exemplar for Class 11 Math - Permutations and Combinations?

In the chapter, NCERT Exemplar for Class 11 Math - Permutations and Combinations, you are going to learn four different topics. The first one 7.1 is all about the introduction of permutations and combinations, the second one is 7.2 that comprises fundamental principles of counting, the third one 7.3 defines permutations and related aspects, and the last one 7.4 explains everything about combinations. So, this is all about the topics covered in this specific chapter of class 11 mathematics.

3. How to be exam ready while preparing NCERT Exemplar for Class 11 Math - Permutations and Combinations?

If you are preparing chapter 7 of NCERT Exemplar for Class 11 Math - Permutations and Combinations, then it takes a few steps to be fully exam ready. Your first step while preparing for the subject is to learn the formulas and equations. Once your mind is clear in terms of formulas, then it is time to cover topic-wise study. Then, the next step is to plan a timetable for regular study. And, once the syllabus is complete, you should start revising all the chapters immediately.

4. Will Vedantu prove to be a complete guide for preparing NCERT Exemplar for Class 11 Math Chapter 7 - Permutations and Combinations (Book Solutions)?

You can call Vedantu a complete package for preparing NCERT Exemplar for Class 11 Math Chapter 7 - Permutations and Combinations (Book Solutions). Vedantu works on a unique approach to guide students with basic concepts and further take them to complex ones. This way, students get habitual to the syllabus gradually and consider working on their lacking points during this process. From helping you resolve the questions to the revision procedure, Vedantu experts create a foolproof strategy to ensure your success.

5. What are the key pointers to ensure a good score in NCERT Exemplar for Class 11 Math - Permutations and Combinations?

If you want to score well in NCERT Exemplar for Class 11 Math - Permutations and Combinations, then you only need to follow a few simple steps:

The first one is to download study material from the Vedantu.
The second one is to map out a dedicated schedule for studying the downloaded material, or if you want to study in online mode, that is also possible.
The third one is to stick to the schedule you have prepared. Ensure that you follow this routine till your exam completes and leave enough time for revision.
The fourth one is to keep time for relaxation therapies in between otherwise learning will turn monotonous and you will avoid it unconsciously.