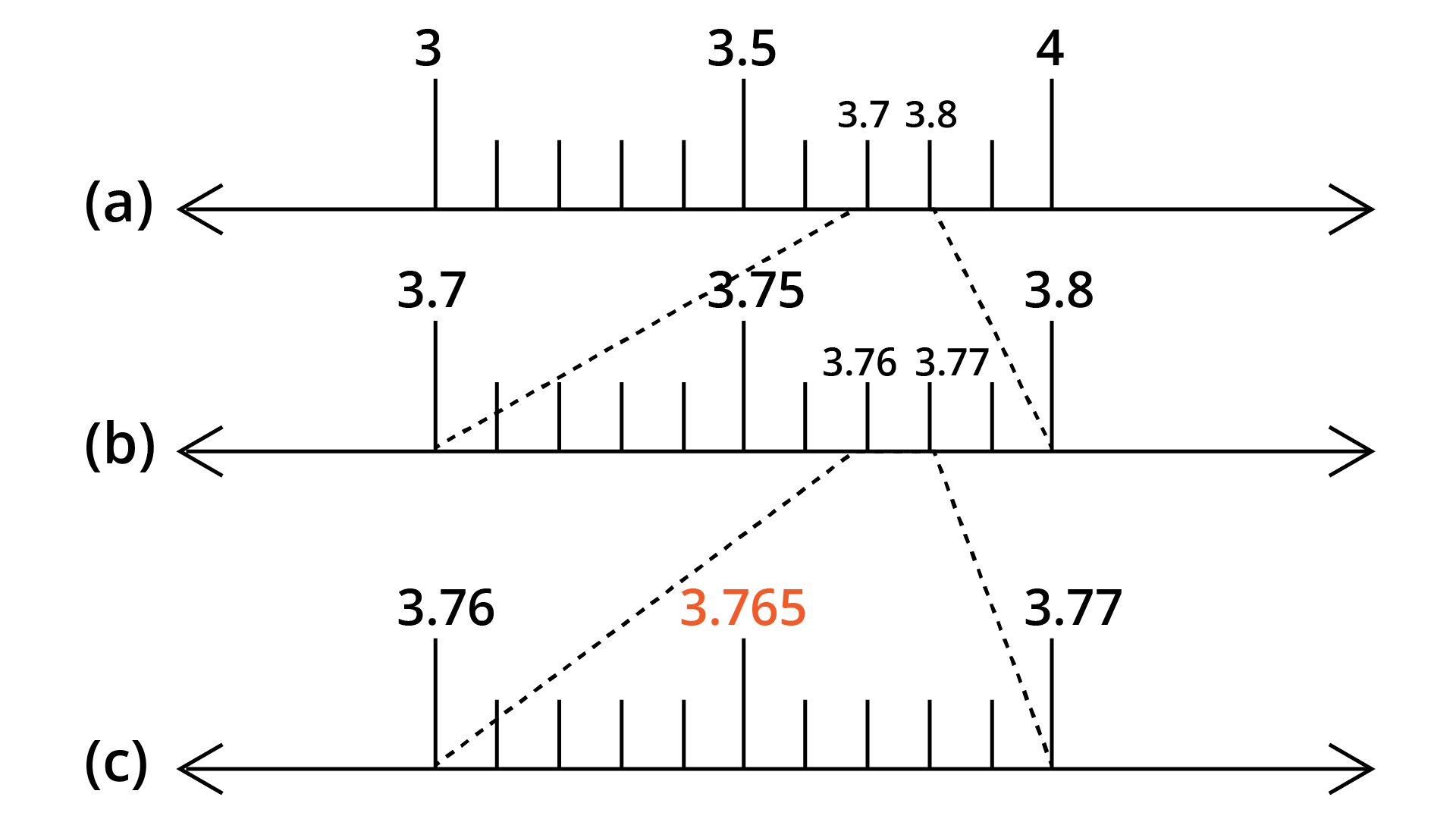NCERT Solutions for Class 9 Maths Chapter 1 Number System In Hindi pdf download
NCERT Solutions for Class 9 Maths In Hindi Chapter 1 Number System
FAQs on NCERT Solutions for Class 9 Maths In Hindi Chapter 1 Number System
1. How many questions in each exercise are given in Chapter 1 Number Systems of Class 9 Maths?
In Chapter 1 of Class 9 Maths, there are six exercises. These exercises are based upon the number system with a special focus on rational and irrational numbers. The first exercise contains four questions, the second contains four, the third contains nine, the fourth contains two, the fifth contains four, and the sixth contains three. Number Systems, Chapter 1 of Class 9 Mathematics, has a total of 35 questions, 30 of which are simple, two intermediate, and three of which are extended answer-type problems.
2. What are the core topics to study in Chapter 1 Number Systems of Class 9 Mathematics?
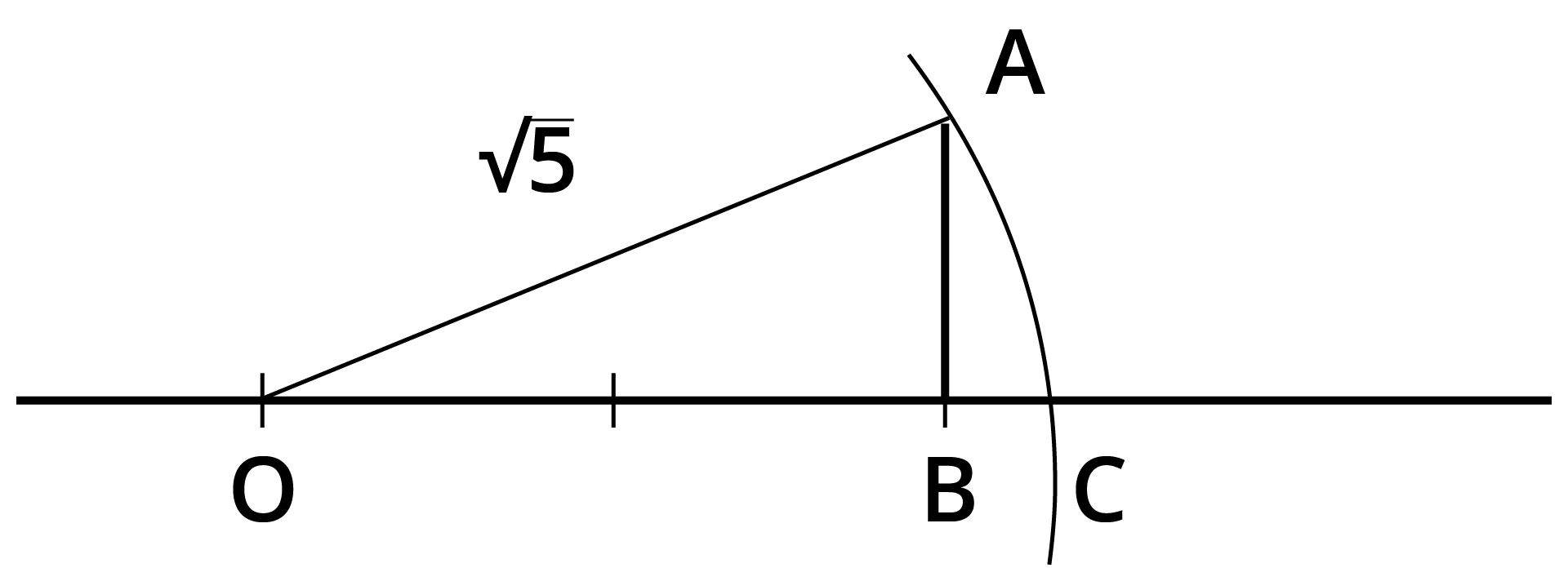
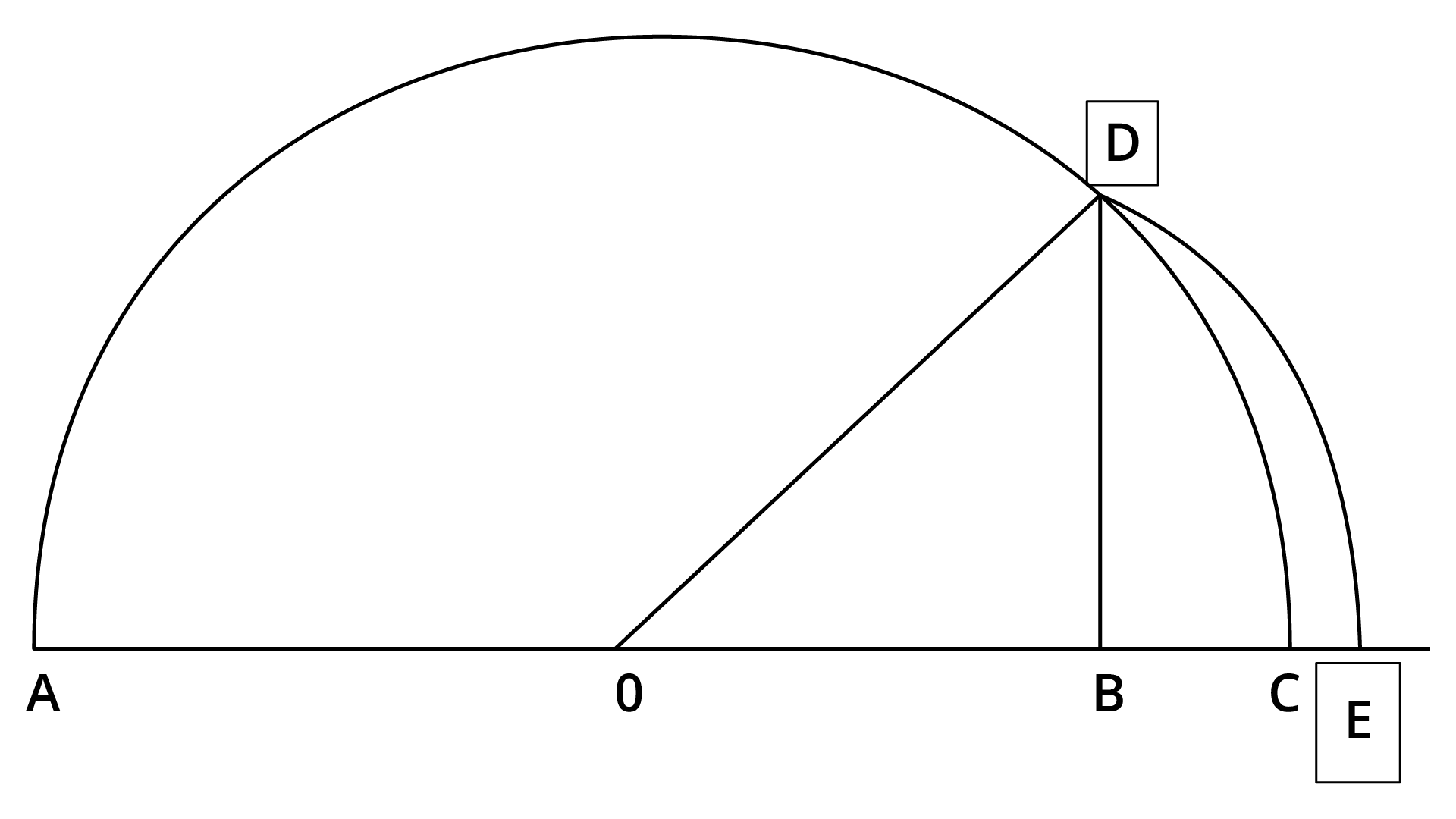
There are a lot of core topics to study in Chapter 1 Number Systems of Class 9 Mathematics. They are irrational numbers, real numbers, and real numbers extended in decimal form are among the major subjects covered. The representation of real numbers on a number line, techniques for performing operations on real numbers, and rules of exponents when working with real numbers are all covered in Chapter 1 of the NCERT answers for Class 9 maths.
3. Is Chapter 1 Number Systems of Class 9 Maths difficult to solve?
Chapter 1 of Class 9 Maths is very new to the students. The concept of rational and irrational numbers is introduced in Class 9 Maths hence it comes across as a bit difficult to students. Following the right approach to study can help the students study this chapter and get good marks in the long run. Students must complete all the NCERT exercises to get a comprehensive idea of the chapter and this will help them gain clarity of the concepts in the chapter.
These solutions are available on Vedantu's official website(vedantu.com) and mobile app free of cost.
4. How many chapters are there in NCERT Class 9 Maths apart from Chapter 1?
In the Class 9 Maths NCERT, there are a total of 15 chapters. These chapters are based upon a variety of topics that are covered in the Maths of higher studies. Class 9 Maths consists of a lot of new topics that were not essentially covered in the previous classes. So to accommodate all the new concepts and to make sure that you are not intimidated by the sheer longevity of the subject, you must study from the NCERT and work extensively on the NCERT Solutions.
5. Is Chapter 1 Number Systems of Class 9 Maths tough?
Students in the ninth grade require thorough preparation for their impending exams. Mathematics is one of those subjects that requires practice from the start. The majority of pupils are scared of this topic and have little understanding of the mathematics chapters. Finally, they receive unsatisfactory grades, lowering their aggregate during the finals. Some pupils struggle with Geometry, while others struggle with Surface Area and Volume, Constructions, and Statistics. Usually, geometry is considered the most difficult. However, proper practice can solve anything. When it comes to Chapter 1, it is an important chapter as it is the foundation for other chapters you will encounter in the future. Once you understand the concept, it is quite easy.




















 Watch Video
Watch Video