



What is the Intercept of a Line?
The x-intercept and the y-intercept are the two different types of intercepts. The line's actual point of intersection with the x-axis is known as the x-intercept, while its actual point of intersection with the y-axis is known as the y-intercept. In this article, we will define the intercept, show how to obtain the intercept for a given line, and demonstrate how to graph intercepts.
What is x-Intercept?
The general form of the linear equation is written as \[y = mx + c\], where m and c are constants. The line's crossing point, which is located at the x-axis of the plane, is known as the x-intercept. This indicates that anytime a linear equation crosses the x-axis, its y-coordinate value will always be equal to 0. The y-coordinate is zero for the x-intercept and the x-coordinate is zero for the y-intercept. The term "horizontal intercept" also applies to the x-intercept.
X-Intercept
What is y- Intercept?
The graph's intersection with the y-axis is known as the y-intercept. Finding the intercepts for any function with the formula \[y = f\left( x \right)\] is crucial when graphing the function. An intercept can be one of two different forms for a function. The x-intercept and the y-intercept are what they are. A function's intercept is the location on the axis where the function's graph crosses it.
Y-Intercept
Equation of a Line with Intercepts
How to find an intercept of a straight line? Intercepts are subject to various equations and formulas. By solving for x and putting \[y = 0\] in the equation, all of the formulas are obtained.
Following are the steps to determine the y intercept of a function, where\[y = f\left( x \right)\], where \[x = 0\] is simply substituted.
Resolve for y.
Put the y-point intercepts in place (0, y).
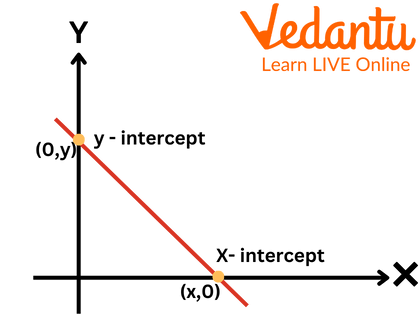
Intercepts
Conclusion
The two different kinds of intercepts are the x-intercept and the y-intercept. The x-intercept of a line is its actual point of intersection with the x-axis, and the y-intercept is its true point of intersection with the y-axis.
Solved Examples
Example 1:Find the value of "a" if the y-intercept of a function \[y = a(x - 1)\left( {x - 2} \right)\left( {x - 3} \right)\] is \[\left( {0,12} \right)\] .
Ans: The given function's equation is:
\[y = a(x - 1)\left( {x - 2} \right)\left( {x - 3} \right)\]
By adding \[x = 0\] to the y-intercept formula, it can be calculated.
\[y = a \left( {0 - 1} \right]\left[ {0 - 2} \right]\left[ {0 - 3} \right]\] \[ = - 6a\]
The y-intercept is therefore \[\left( {0, - 6a} \right)\]
However, the issue claims that the provided function's y-intercept is \[\left( {0,12} \right)\]. Thus,
\[ - 6a = 12\]
Using -6 to divide both sides,
\[a = - 2\]
Example 2: Find the x and y intercept in the given graph.
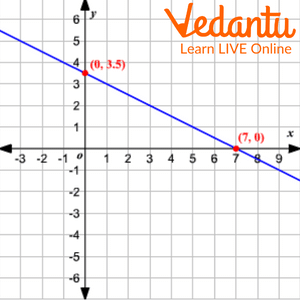
Ans: The x-intercept is 7 and y – intercept is 3.5 in the given graph.
Example 3: Find the x-intercept of \[y = 4x - 8\].
Ans: For finding the x – intercept we must put y coordinate as 0.
Thus, putting\[y = 0\] ,we will get:
\[\begin{array}{l}0 = 4x - 8\\8 = 4x\\x = 2\end{array}\]
Therefore, x coordinate is 2.





















FAQs on Intercepts of a Line
1. What are the Y Intercept Formula Applications?
To determine a function's y-intercept, use the y-intercept formula. The procedure of graphing a function is when the y-intercept is most frequently employed.
2. Is 0 a possible x-Intercept?
For the line\[y = mx\], where m is the line's slope and the value of the x-intercept is equal to 0, 0 can indeed serve as the x-intercept.
3. What shape does the value angle between the line's Y and X intercepts take if they are equal?
When a line's x-intercept and y-intercept are equal, the line intersects the x-axis at a 45° angle if we are taking in first quadrant.
4. How many intercepts are permitted on a line?
X-intercept and Y-intercept are the two main intercepts. The line's x-intercept and y-intercept are located where the line crosses the x and y axes, respectively.



