What are the Common Terms in Geometry?
A branch of mathematics that studies the sizes, shapes, positions, angles and dimensions of 2D and 3D figures is called Geometry. Shapes like squares, circles, and triangles are a part of flat geometry and they are 2-D. However, shapes like spheres, cylinders, cubes, cuboids, and so on are 3-D. Each shape that we study in geometry has specific properties and these properties.
So, let us learn about the various geometric shapes and their appearance. Following this, we will go through the meaning of each property of a geometric shape with a specific definition.
What are 2-D Shapes in Geometry?
Shapes that have only two dimensions, i.e., length and width are 2-D shapes. Below, you can find 2-D shapes:
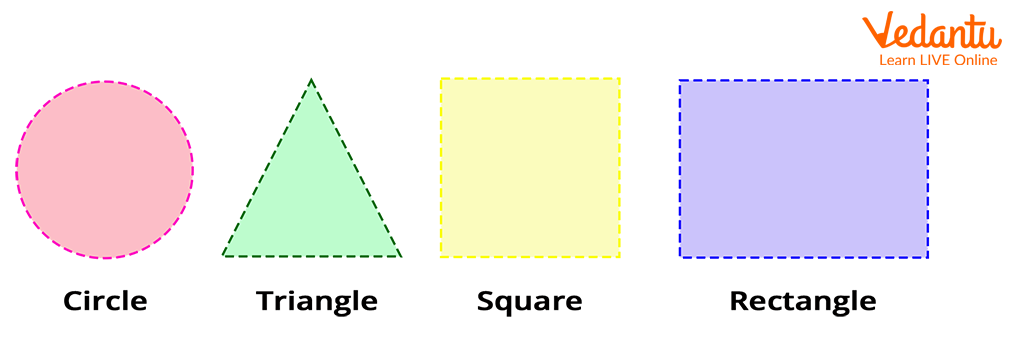
2-D Shapes
What are 3-D Shapes in Geometry?
Shapes that have three dimensions, i.e., length, width, and height are 3-D shapes. Below, you can find 3-D shapes:
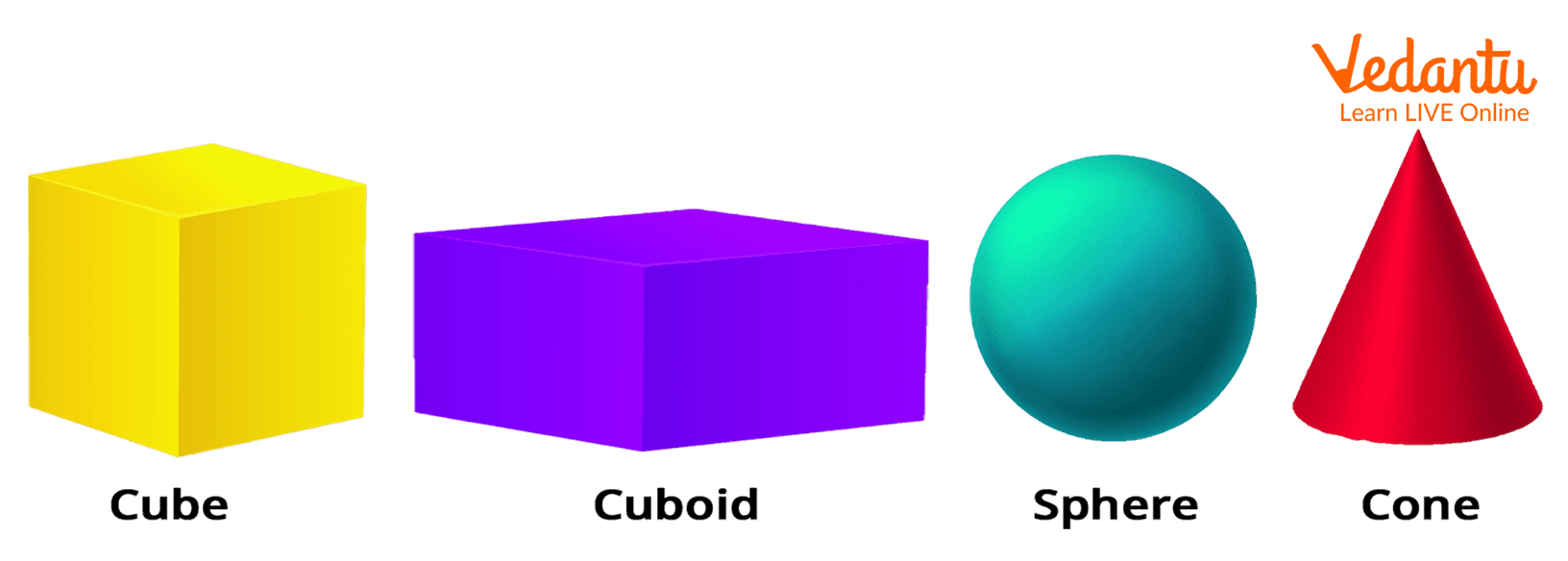
3-D Shapes
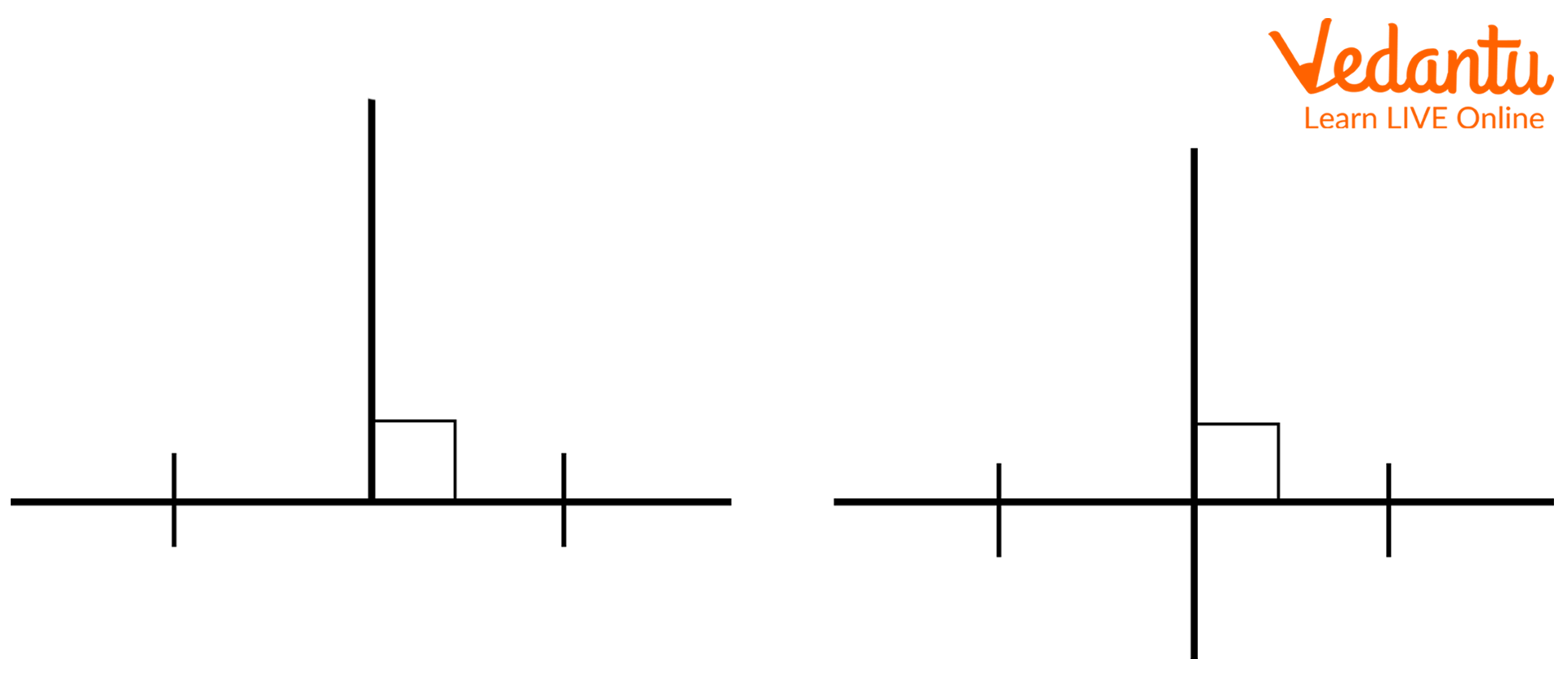
All these shapes have respective parameters like a triangle has three edges, vertices, and angles. A cuboid has length, width, and height. Now, a question arises: what are these parameters? Well, all these terms come under Geometry Vocabulary and what does this vocabulary mean? Let us understand.
Various Terms in Geometry
So, this was all about the geometry vocabulary. Going through this will help you understand what parameters does a geometrical shape has.
FAQs on Geometry Vocabulary
1. List the polygons having more than 4 sides.
Following are the polygons having more than four sides.
Pentagon
A shape that has five sides.
The number of vertices - 5
Internal angle measure - 108-degrees, therefore, the sum of internal angles - 5 x 108 = 540-degrees.
Hexagon
A shape that has six sides and vertices.
Having six angles, where the sum of internal angles measure 720-degrees. However, the sum of external angles measures 360-degrees.
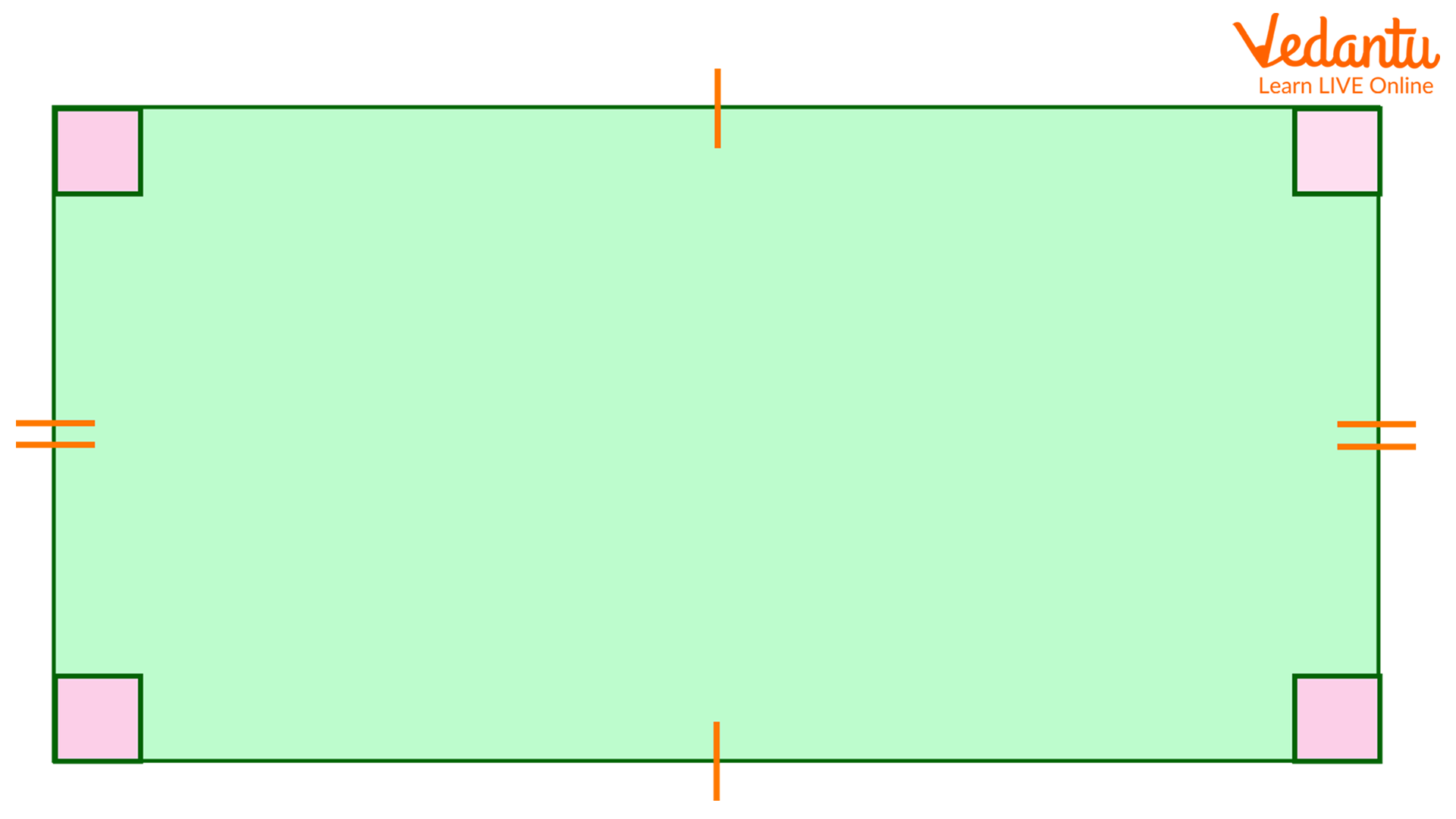
2. Define a trapezium and a parallelogram.
Trapezium- A quadrilateral that has two parallel and two nonparallel sides.
Parallelogram- It is a quadrilateral that has both pairs of opposite sides parallel to each other.