Number Power

A base number being raised to an exponent is referred to as a "power" in mathematics. It signifies that "base number" and "exponent" are the two fundamental components of powers.

Number Power:

How to find the power of a number? How many times to multiply a given number depends on its power (or exponent).

It appears as a small number above and to the right of the basic number.

The small "2" in this illustration tells us to multiply 8 twice:

\[{8^2} = 8 \times 8 = 64\]

Hence the Number Power Formula for 8 times 8 will be \[{8^2}\].

However, the term "power" can also refer to the outcome of an exponent, so in the previous example, "64" is also referred to as the power.

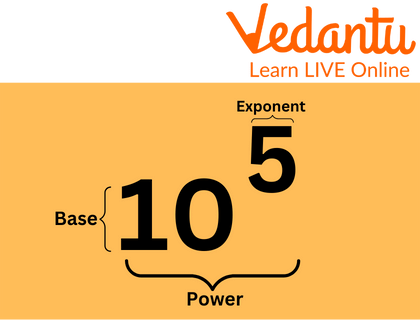
Different Terms of Exponent

Exponent of a Number:

A number's exponent indicates how many times the number has been multiplied by itself. Example: Since 2 is multiplied by itself 4 times, \[2 \times 2 \times 2 \times 2\] can be expressed as \[{2^n}\]. Here, 4 is referred to as the "exponent" or "power," while 2 is referred to as the "base." Generally speaking, \[{x^n}\] denotes that x has been multiplied by itself n times.

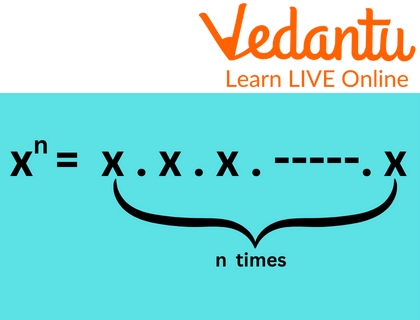
Exponent of a Number

Here, Here, in the term xⁿ

x is called the "base"

n is called the "exponent"

And is read as "x to the power of n" or "x raised to n".

Power of Exponents:

We may easily express and represent extremely big and small numbers using exponential notation or the exponential form of numbers. For instance, 10000000000000 is equal to \[1 \times {10^{13}}\] whereas 0.0000000000000007 is equal to\[7 \times {10^{ - 16}}\]. This makes numbers easier to read, helps in ensuring their accuracy, and saves us time.

Properties of Exponents:

These characteristics are regarded as major exponents rules that must be followed when solving exponents. The following list includes exponent qualities.

Product law: \[{a^m} \times {a^n} = {a^{m + n}}\]

Quotient law: \[{a^m} \times {a^n} = {a^{m -n}}\]

Zero Exponent law: \[{a^0} = 1\]

Negative Exponent law: \[{a^{ - m}} = 1 \div {a^m}\]

Power of a Power law: \[{({a^m})^n} = {a^{mn}}\]

Power of a Product law: \[{\left( {ab} \right)^m} = {a^m}{b^m}\]

Important Points for Power:

• When a fraction's exponent is negative, we take the fraction's reciprocal to make it positive. Consequently, \[{\left( {\frac{a}{b}} \right)^{ - m}} = {\left( {\frac{b}{a}} \right)^m}\]

• We can set the bases to equal when the exponents in an equation are the same on both sides, and vice versa.

Solved Examples:

1: Each tree in a garden has roughly \[{5^7}\] leaves, and there are about \[{5^3}\] trees overall. Calculate the total number of leaves using exponents.

Ans: The number of trees in the garden is \[{5^3}\] , and each tree has \[{5^7}\] leaves. \[{5^3}\]\[ \times \] \[{5^7}\] = \[{5^{10}}\] leaves total, according to the exponents law.

Therefore, there are \[{5^{10}}\] leaves in all.

2: What is 2 when it has a 7 exponent?

Ans: When 2 has an exponent of 7 then the answer will be \[{2^7} = 128\].

As \[2 \times 2 \times 2 \times 2 \times 2 \times 2 \times 2\] \[ = 128\].

3: Solve \[{25^3}/{5^3}\].

Ans: Using quotient Law: \[{a^m} \div {a^n} = {a^{\frac{m}{n}}}\]

It is possible to write \[{25^3}/{5^3}\] as \[{(25/5)^3}\]

\[ = {5^3}\]

Conclusion:

An expression known as "power" denotes the process of repeatedly multiplying a value or integer. \[{a^n}\] is often a power where n is the exponent and \[{a^n}\] is the base.