How to Find the LCM of Decimal Numbers with Formula and Examples
The least Common Multiple is the meaning of the abbreviation LCM. The lowest number that may be divided by both numbers is the least common multiple (LCM) of two numbers. It can also be computed using two or more numbers. Finding the LCM of a given set of numbers can be done in various ways. Utilizing the prime factorization of each number and then calculating the product of the highest powers of the shared prime factors is one of the quickest techniques to determine the LCM of two numbers. In this article, we will learn how the lcm of decimal numbers can be found and see LCM of fractions formula.
How to Find the LCM of Decimal Numbers?
To find the LCM of decimal numbers, we have two different methods. Anyone willing to use any of these can use it anywhere to solve LCM of decimal numbers. The two different methods to determine the LCM of a set of numbers are the following:
LCM by Using the Listing MethodLCM of 1.50 and 5.00 by Listing Method: For 1.50 and 5.00, move the decimal 2 places to the right. Now, they are whole numbers. We will find the LCM of 150 and 500. In the end, we will move the decimal point back to 2 places to the left.
Multiples of 150 are 150, 300, 450, 600, 750, 900, 1050, 1200, 1350, 1500, 1650, and 1800, ….
Multiples of 500 are 500, 1000, 1500, 2000, 2500, …
The lowest common multiple of 150 and 500 is 1500
So, LCM(150, 500) = 1500.
Now, we move the decimal towards 2 places to the left.Therefore LCM(1.50,5.00) = 15.00.
LCM by Using the Division MethodLet’s understand the LCM by using the division method through an example:
LCM of 1.50 and 5.00 by Common Division Method
For each of the numbers, we move the decimal to two places to the right and calculate the LCM of whole numbers 150 and 500. Then, in the end, we move the decimal to two places back.
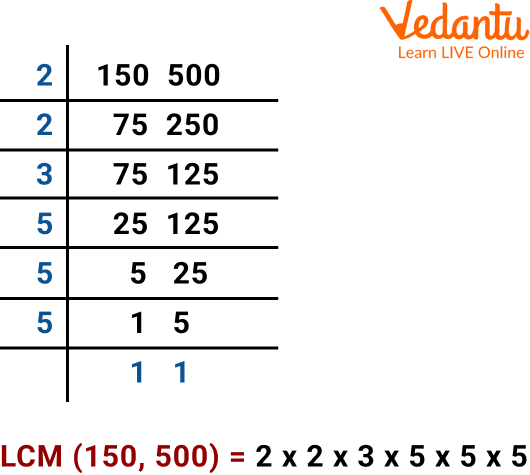
LCM of 150 and 500 is1500
We see that LCM of 150 and 500 is 1500. Since we multiplied by 100 to remove the decimal point, we will divide by 100, moving the decimal point two places back. So, we get the LCM of 1.5 and 5 as 15.
Hence, LCM of 1.50 and 5.00 is 15.
LCM of Fractions Formula
To solve any problem efficiently, one must know its formula. In the same way, finding out the LCM of fractions requires some formula. To find L.C.M of $\dfrac{a}{b}$ and $\dfrac{c}{d}$ the generalized formula will be:
L.C.M $=\dfrac{\text { L.C.M of numerators }}{\text { H.C.F of denominators }}$.
Now L.C.M of two numbers is the smallest number (not zero), a multiple of both.
Let's take the example of $\dfrac{2}{5} \text { and } \dfrac{3}{7}$
$\text { L.C.M }=\dfrac{\text { L.C.M of }(2,3)}{\text { H.C.F of }(5,7)}$ ....(1)
So H.C.F of 5,7:
The factors of 5 are: 1,5
The factors of 7 are: 1,7
1 is the only common factor as it is the only number common to both 5 and 7.
Therefore, H.C.F of $(5,7)=1$. .....(2)
Now L.C.M of 2, 3:
The multiples of 2 are $2,4,6,8, \ldots$.
The multiples of 3 are $3,6,9,12, \ldots$
6 is the lowest common multiple as it is a multiple common to both 2 and 3.
Therefore, L.C.M of $(2,3)=6$ .......(3)
Putting the value of H.C.F from equation (2) and the value of L.C.M from equation (3) in equation (1), we get L.C.M $=\dfrac{6}{1}=6$
LCM calculator with work can be done by using the online calculator, which is available on the internet. Through this calculator, we can easily find the LCM online.
Solved Examples
Q 1. Find the least common multiple (LCM) of 6 and 15 using the division method.
Ans: Let us find the least common multiple (LCM) of 6 and 15 using the division method using the steps given below.
Step 1: 2 is the smallest prime number, a factor of 6. Write 2 on the left of the two numbers. For each number in the right column, continue finding prime numbers and their factors.
Step 2: 2 divides 6, but it is not a factor of 15, so we write the number 15 in the row below as it is. Continue the steps until 1 is left in the last row. Then, we divide 3 and 15 by 3. This gives us 1 and 3. We write 5 on the left side and finally get 1, 1 as the quotient in the last row.
Step 3: Then, we multiply these numbers on the left. The LCM is the product of all these prime numbers. LCM of 6 and 15 is 2 × 3 × 5 = 30.
LCM of 6 and 15
Q 2. Find the LCM of 25, 15, and 30 by listing method.
Ans: Let us use the following steps to find the LCM of the 3 numbers.
Step 1: List the first few multiples of all three numbers, This will be:
Multiples of 25 = 25, 50, 75, 100, 125, 150, 175, ....,
Multiples of 15 = 15, 30, 45, 60, 75, 90, 105, 120, 135, 150, 175,...
Multiples of 30 = 30, 60, 90, 120, 150, 180, 210, ...
Step 2: Among the common multiples of 25, 15 and 30, we can see that 150 is the least multiple that is common in all three numbers.
Therefore, the LCM of 25, 15 and 30 = 150.
Practice Questions
Q 1. Find the LCM of 0.48, 0.72, and 0.108.
Ans: 4.32
Q 2. Find the LCM of $\dfrac{1}{3}$, $\dfrac{1}{6}$, and $\dfrac{1}{9}$.
Ans: $\dfrac{1}{18}$
Q 3. Calculate the LCM of 0.8, 0.2, and 4.8.
Ans: 4.8
Summary
Finding out LCM is quite easy to learn and remember. Interestingly, the division method is highly used to find the LCM of any two or three numbers. We are sure the kids have learned in depth about the LCM of decimal numbers, how to find the LCM of decimal numbers, the LCM of fractions, the LCM calculator with work, and many more. Other than this, solving various types of practice questions will help the kids master the particular topic in a better way.
FAQs on LCM of Decimal Numbers Explained with Steps
1. What is the LCM of decimal numbers?
The LCM of decimal numbers is the smallest positive number that is exactly divisible by all the given decimal numbers. To find it, we usually convert decimals into whole numbers by multiplying by powers of 10, calculate the LCM of those whole numbers, and then adjust the decimal place in the final answer. This method ensures accurate calculation while following the standard Least Common Multiple (LCM) rules.
2. How do you find the LCM of decimal numbers step by step?
To find the LCM of decimal numbers, first convert them into whole numbers, find their LCM, and then adjust the decimal place. Follow these steps:
- Step 1: Identify the maximum number of decimal places.
- Step 2: Multiply each decimal by 10, 100, 1000, etc., to make them whole numbers.
- Step 3: Find the LCM of the whole numbers.
- Step 4: Divide the LCM by the same power of 10 used earlier.
Example: LCM of 0.4 and 0.6 → Multiply by 10 → 4 and 6 → LCM = 12 → Divide by 10 → 1.2.
3. What is the formula for finding the LCM of decimals?
The formula for LCM of decimals is: LCM (decimals) = LCM (converted whole numbers) ÷ 10ⁿ, where n is the highest number of decimal places. First convert all decimals into integers by multiplying by 10ⁿ, then calculate their LCM, and finally divide the result by 10ⁿ to restore the decimal value.
4. Can you give an example of LCM of decimal numbers?
Yes, the LCM of 1.5 and 2.5 is 7.5. Here’s how:
- Step 1: Multiply both numbers by 10 → 15 and 25.
- Step 2: Find LCM of 15 and 25 → 75.
- Step 3: Divide by 10 → 75 ÷ 10 = 7.5.
Thus, 7.5 is the smallest decimal number divisible by both 1.5 and 2.5.
5. Why do we convert decimals to whole numbers when finding LCM?
We convert decimals to whole numbers because the standard LCM method works directly with integers. Decimal values make division and factorization complex, so multiplying by powers of 10 simplifies the numbers. After finding the LCM of integers, we adjust the decimal place to get the correct final result.
6. Is the LCM of decimal numbers always a decimal?
Yes, the LCM of decimal numbers is usually a decimal unless the result simplifies to a whole number. Since the original numbers contain decimal places, the final LCM typically includes decimals after adjusting back from the integer form.
7. What is the LCM of 0.2 and 0.8?
The LCM of 0.2 and 0.8 is 0.8. Steps:
- Multiply both by 10 → 2 and 8.
- LCM of 2 and 8 = 8.
- Divide by 10 → 8 ÷ 10 = 0.8.
Therefore, 0.8 is the smallest decimal divisible by both 0.2 and 0.8.
8. What is the difference between LCM of integers and LCM of decimals?
The difference is that LCM of integers is found directly, while LCM of decimals requires conversion to whole numbers first. For decimals:
- Multiply to remove decimal places.
- Find LCM of integers.
- Adjust the decimal place in the result.
The core concept remains the same: finding the smallest common multiple.
9. Can we use prime factorization to find the LCM of decimals?
Yes, you can use prime factorization after converting decimals into whole numbers. First multiply to eliminate decimals, then factorize the integers into primes, take the highest powers of each prime, and finally adjust the decimal place in the result.
10. What are common mistakes when finding the LCM of decimal numbers?
Common mistakes when calculating the LCM of decimal numbers include incorrect decimal adjustment and incomplete conversion to whole numbers. Avoid these errors:
- Not multiplying all numbers by the same power of 10.
- Forgetting to divide the final LCM by 10ⁿ.
- Using different decimal place conversions.
- Making errors in integer LCM calculation.
Always check decimal places carefully before and after computing the LCM.
