Exam - Focused Revision Notes for CBSE Class 10 Maths Chapter 11 - Areas Related to Circles
Areas Related to Circles Class 10 Notes CBSE Maths Chapter 11 (Free PDF Download)
FAQs on Areas Related to Circles Class 10 Notes CBSE Maths Chapter 11 (Free PDF Download)
1. Why Should I Refer to Vedantu for CBSE Class 10 Maths Notes Chapter 11 Areas Related to Circles?
Ans: Vedantu has a strong faculty with vast experience in teaching Math subjects. Until offering solutions to major issues, these experts conduct extensive research. Subject matter experts developed these solutions and principles to simultaneously answer your concerns and doubts. This technique would also help you research more efficiently during your self-study hours. As a result, when students use the free PDF, they can learn and enjoy the subject.
2. What are the Topics that are Covered in the Introduction of the Area Related to Circles?
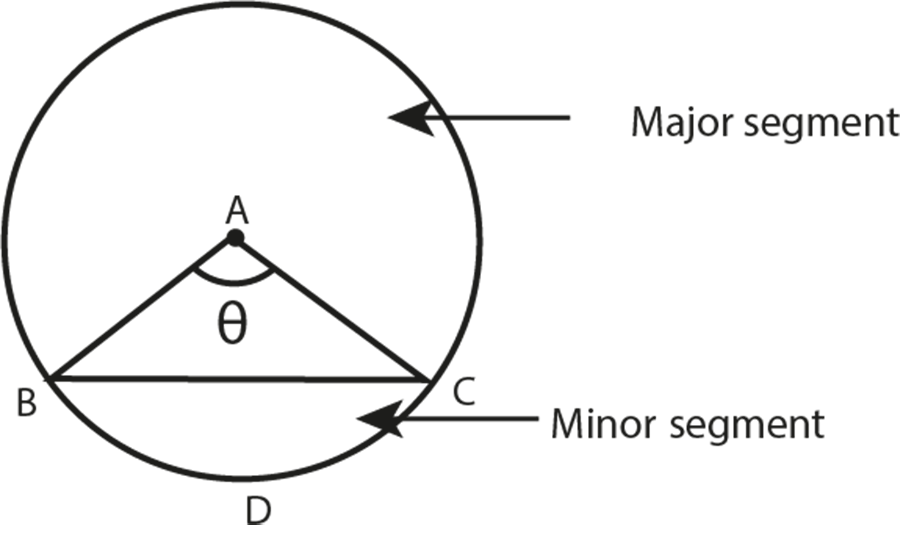
Ans: Circles chapter is one of the important chapters which has more weightage in CBSE exams. Around 5% of weightage is given to this chapter in the board exam and also 2-3% weightage is given JEE exams. The chapter covers Introduction, Area of a Circle, Circumference of a Circle, Segment of a Circle, Minor arc and Major Arc, Sector of a Circle, Angle of a Sector, Length of an arc of a sector, Area of a Sector of a Circle, Area of a Triangle, Area of a Segment of a Circle, Visualizations, Areas of different plane figures, Areas of Combination of Plane figures.
3. How can I Access a Free PDF of Areas Related to Circles Class 10 Notes?
Ans: Vedantu provides free PDF solutions to Chapter 11 Areas Related to Circles. If you solve these exercises extensively using Vedantu platforms as a reference source, you get full conceptual clarity in question and answer format. This free PDF also includes additional problems for students to practise alongside the exercise problems.
4. What are some of the important points to remember for Areas Related to Circles Class 10 Notes CBSE Maths Chapter 11?
Here are some of the important points to remember for Areas Related to Circles Class 10 Notes CBSE Maths Chapter 11:
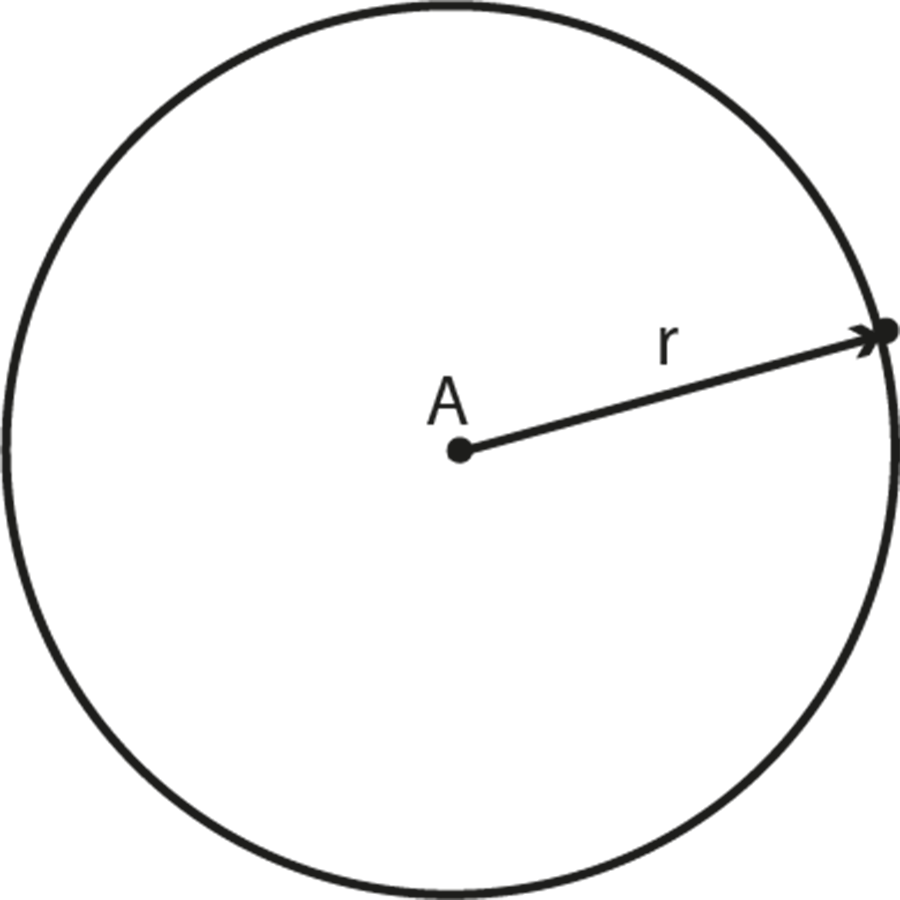
The radius of a circle is the distance from the center of the circle to any point on its circumference.
The diameter of a circle is twice the radius.
The circumference of a circle is the distance around the circle.
The area of a circle is the amount of space enclosed by the circle.
The formula for the circumference of a circle is 2πr.
The formula for the area of a circle is πr².
5. What are some of the properties and applications of circles?
Properties:
All radii of a circle are equal in length.
All chords of a circle that pass through its center are equal in length.
The angle in a semicircle is a right angle.
The sum of the angles in a triangle inscribed in a circle is 180°.
Applications:
Circles have many applications in the real world, such as:
Wheels
Clocks
Sports equipment
Architecture
Engineering























 Watch Video
Watch Video