Question

In the given figure $ \angle PQR = {100^o} $ , $ P,Q $ and $ R $ are points on the circle with center at $ O $ .Find $ \angle OPR $ .

Answer 1

Hint: The sum of opposite angles in a cyclic quadrilateral is $ {180^o} $ . Also, the angle subtended by an arc at the center is double the angle subtended by it at any another point on the circle.

Complete step-by-step answer:
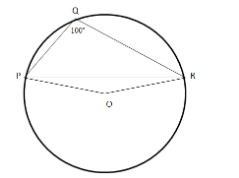
 $ P,Q $ and $ R $ points lying on the circle such that $ \angle PQR = {100^o} $ .
There is a construction required in the above diagram. Take a point $ S $ on the major arc of the circle and join $ PS $ and $ RQ $ .

Now from the figure it is clear that, $ PQRS $ is acyclic quadrilateral as all the points are lying on the circle.
The sum of opposite angles in a cyclic quadrilateral is $ {180^o} $
Therefore,
 $ \angle PQR + \angle PSQ = {180^o} \cdots \left( 1 \right) $
But $ \angle PQR = {100^o} $ (given in the question), substitute it in equation (1),
 $ {100^o} + \angle PSR = {180^o} $
Now, solving for $ \angle PSR $ .
 $
  \angle PSR = {180^o} - {100^o} \\
  \angle PSR = {80^o} \cdots \left( 2 \right) \\
  $
Now, PQ subtends an angle at the center, $ \angle POR $ and on the point $ S $ , $ \angle PSR $ as well.
There is property that angle subtended by an arc at the center is double the angle subtended by it at any point on the circle.
Now according to the property it can be concluded that,
 $ \angle POR = 2\left( {\angle PSR} \right) \cdots \left( 3 \right) $
Substitute the value of $ \angle PSR = {80^o} $ from equation (2) in equation (3),
 $
  \angle POR = 2\left( {{{80}^o}} \right) \\
  \angle POR = {160^o} \\
  $
Now, in $ \Delta OPR $
 $ OP = OR $ (Both are radius of the circle).
 $ \angle OPR = \angle ORP $
(The two sides of a $ \Delta OPR $ are equal, the triangle is an isosceles triangle. Therefore, the angles are equal)
In $ \Delta OPR $
Sum of all angles of the triangle is $ {180^o} $ .
 $ \angle OPR + \angle ORP + \angle POR = {180^o} \cdots \left( 4 \right) $
Since, $ \angle OPR = \angle ORP $ and substitute $ \angle POR = {160^o} $ , equation (4) can be written as,
 $
  2\angle OPR + {160^o} = {180^o} \\
  2\angle OPR = {180^o} - {160^o} \\
  \angle OPR = {10^o} \\
  $
Hence, the value of $ \angle OPR = {10^o} $

Note: The cyclic quadrilateral is a quadrilateral whose all points lie on the circle.
The important points are two properties which are used in the question. They should be understood and remembered.
The sum of opposite angles in a cyclic quadrilateral is $ {180^o} $ .
The angle subtended by an arc at the center is double the angle subtended by it any point on the circle.