Question

Each side of a cube is 5 inches long. How do you find the lengths of a diagonal of the cube?

Answer 1

Hint: Here we are asked to find the lengths of a diagonal of the cube where the side of a cube is given. To find this we need the length of the diagonal of the face. Consider any one of the faces of the cube. We find the length of the diagonal of this face by using Pythagoras theorem. Then after getting the value for the length of the diagonal of the face, we construct a triangle inside the cube and find the overall longer diagonal of the cube by Pythagoras theorem.

Complete step by step solution:
Let us denote the side of the cube by `s’.
Given the side of a cube $s = 5$ inches.
We are asked to find the lengths of a diagonal `d’ of the cube.
For this we must know the length of the diagonal of any one of the face.
Consider the cube given in the diagram.

We need to find the length of the diagonal of the face from E to F.
We find the length EF using the Pythagoras theorem, which states that in a right angled triangle, the square of the length of the hypotenuse is equal to the sum of the squares of the other two sides.
So consider the one of the faces of the cube.

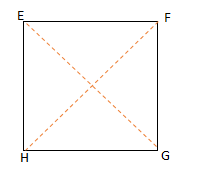
Now we need to find the length of EF which is the shorter diagonal of the cube.
By Pythagoras theorem we have,
${a^2} + {b^2} = {c^2}$
Here a, b are the sides of the cube and c is the length of EF.
Hence we have, $a = b = s$.
$ \Rightarrow {s^2} + {s^2} = E{F^2}$
$ \Rightarrow 2{s^2} = E{F^2}$
We know that the length of the side, $s = 5$inches.
$ \Rightarrow 2{(5)^2} = E{F^2}$
$ \Rightarrow 2 \times 25 = E{F^2}$
Taking square root on both the sides we get,
$ \Rightarrow \sqrt {2 \times 25} = EF$
We know that $\sqrt {25} = 5$.
$ \Rightarrow \sqrt 2 \times 5 = EF$
$\therefore EF = 5\sqrt 2 $
Hence the length of the diagonal of the face is $EF = 5\sqrt 2 $ inches.
Now we can construct the triangle and find the overall longer diagonal `d’ of the cube using Pythagoras theorem.
Consider the triangle $EFG$. We need to find the length of $GE$.

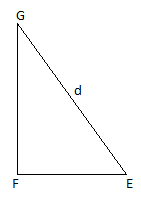
From the triangle we have,
$E{F^2} + F{G^2} = {\text{G}}{{\text{E}}^2}$
Where $EF = 5\sqrt 2 $, $BC = 5$ which is the edge of the cube and $GE = d$
$ \Rightarrow {(5\sqrt 2 )^2} + {5^2} = {d^2}$
$ \Rightarrow (25 \times 2) + 25 = {d^2}$
$ \Rightarrow 50 + 25 = {d^2}$
$ \Rightarrow 75 = {d^2}$
Taking square root on both the sides we get,
$ \Rightarrow \sqrt {75} = d$
We can write,
$\sqrt {75} = \sqrt {25 \times 3} $
$ \Rightarrow 5\sqrt 3 $
Therefore $d = 5\sqrt 3 $
Hence the lengths of the diagonal of the cube is $5\sqrt 3 $ inches.

Note: Alternative method :
Given the side of the cube $s = 5$ inches.
We need to find the lengths of the diagonal `d’ of the cube.
The formula to find the length of the diagonal `d’ of the cube is given by,
$d = s \times \sqrt 3 $
$ \Rightarrow d = 5 \times \sqrt 3 $
$ \Rightarrow d = 5\sqrt 3 $.
Hence the lengths of the diagonal of the cube is $5\sqrt 3 $ inches.
Students must remember the Pythagoras theorem and how to apply it to a given problem.
Pythagoras theorem states that,
‘’In a right angled triangle, the square of the length of the hypotenuse is equal to the sum of the squares of the other two sides.’’