Physics JEE Main 2025: Work, Energy and Power

The Work, Energy, and Power chapter is an important part of JEE Main 2025 Physics. This chapter will teach us about concepts like work, energy and power. Work, energy and power are essential concepts in physics. Work is the displacement of an object caused by a force (push or pull). Energy is defined as the ability to perform work. We also get to know about the work done formula and energy formula. Understanding these concepts is essential for solving problems in mechanics and preparing for the JEE Main exam.

JEE Main Physics Syllabus JEE Main Physics Revision Notes JEE Main Physics Important Questions JEE Main Physics Difference Between JEE Main Physics Question Papers

The chapter also includes the following concepts:

• Kinetic Energy: It is the energy that a body possesses as a result of its motion.

• Potential Energy: It is the energy possessed by a body by virtue of its position or configuration in some field. 

• Work Energy Theorem: This concept states that the work done by net force in displacing a body is equal to the change in kinetic energy of the body. 

• Principle of Conservation of Energy: According to this principle, if we account for all forms of energy, the total energy of an isolated system does not change.

• Collision: It is an isolated event in which two or more colliding bodies exert relatively strong force on each other for a relatively short time.

Now, let's move onto the important concepts and formulae related to IIT JEE exams along with a few solved examples.

Important Topics of Work Energy and Power Chapter

• Work done by variable force.

• Conservative and non-conservative forces.

• Power definition and relation between work and power.

• Kinetic energy and linear momentum relation.

• Work energy theorem.

• Potential energy of a spring.

• Mechanical energy and its conservation.

• Collision and its types.

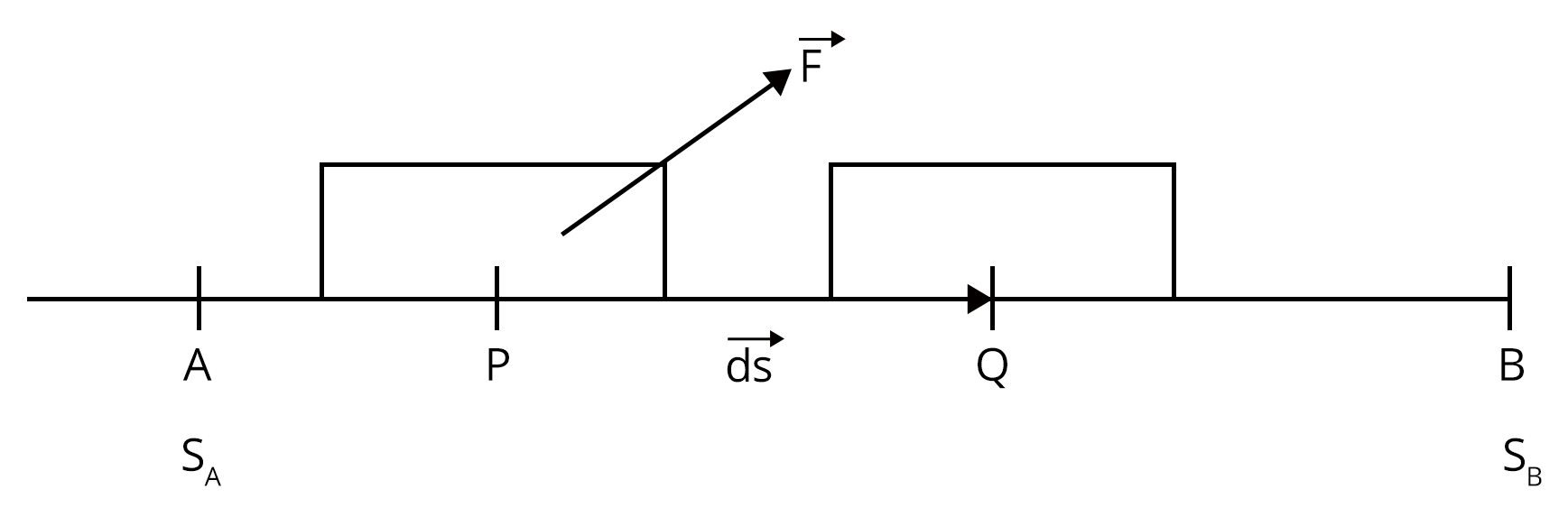
What is Work?

Work happens when a force makes something move. For example, when a person climbs a mountain, work is being done because they are going against gravity to go up. So, two things matter for work:

• Magnitude of force

• The direction in which the body moves due to the force applied.

To calculate work, you multiply how far something moves by the force acting on it. Work is just a number, and the unit we use to measure it is called the Joule. The formula for work is: Work = Force × Distance or W = F $\times$ S

But there's a little twist: if the force isn't pushing in the exact same direction as the movement, you have to use the cosine of the angle between them in the formula: W = F $\times$ S $\times$ Cos $\theta$

It's important to note that work only happens when a force causes something to move. If you push a wall and it doesn't move, you're not doing any work on it. However, you're still using energy because your muscles are working, and you might get tired.

Formula on Work

• Work Done by Constant Force:
  $W = F \times d \times \cos\theta$
  Where:
  W = Work done (Joules)
  F = Force applied (Newtons)
  d = Displacement (Metres)
  $\theta$ = Angle between force and displacement

• Work Done by Variable Force:
  $W = \int \vec{F} \cdot \vec{ds}$
  Where:
  $\vec{F}$ = Variable force
  $\vec{ds}$ = Infinitesimal displacement

• Work Done in Terms of Kinetic Energy (Work-Energy Theorem):
  $W = \Delta K.E. = \frac{1}{2}m(v_f^2 - v_i^2)$
  Where:
  m = Mass of the object (kg)
  $v_f$​ = Final velocity (m/s)
  $v_i$​ = Initial velocity (m/s)

• Work Done Against Gravity:
  $W = m \cdot g \cdot h$
  Where:
  m = Mass (kg)
  g = Acceleration due to gravity ($9.8 \, \text{m/s}^2$)
  h = Height (m)

Unit of Work

• Joule (J)

• Definition: One Joule is the work done when a force of 1 Newton moves an object by 1 metre in the direction of the force.

• Formula: $1 \, \text{Joule} = 1 \, \text{Newton} \cdot 1 \, \text{metre}$ $(1 \, \text{J} = 1 \, \text{N} \cdot \text{m})$

Example on Work

Lifting an Object: When you lift a book weighing 2 kg to a shelf 1.5 metres high, work is done against gravity.

• Formula: $W = m \cdot g \cdot h$

• Calculation: $W = 2 \, \text{kg} \cdot 9.8 \, \text{m/s}^2 \cdot 1.5 \, \text{m} = 29.4 \, \text{J}$

Pushing a Cart: A person pushes a shopping cart with a force of 50 N over a distance of 10 metres in the direction of the force.

• Formula: $W = F \cdot d$

• Calculation: $W = 50 \, \text{N} \cdot 10 \, \text{m} = 500 \, \text{J}$

What is Energy?

The capacity of a man that allows him to do work is called energy. In simple words, energy is the ability to work. It is in scalar quantity and has only magnitude and no direction. energy can neither be created nor destroyed, it can only change in its form. Energy can be found in many things, and so there are many forms of energy. The most important types of energy are kinetic energy and potential energy.

Kinetic energy refers to the energy of a body due to its movement or motion. It refers to the work that is required to accelerate a body of the given mass differentiating it from its velocity. While acceleration after the body gains energy, it maintains kinetic energy until its speed changes.

The formula of kinetic energy is –\[ \frac{1}{2}mv^{2}\] 

Hence from the formula given above, we can state:

1. The kinetic energy of a body gets doubled whenever its mass gets doubled.

2. If the mass of a body is halved, then the kinetic energy is also halved.

3. The kinetic energy of a mass increases by four times when the velocity doubles. 

Types of Energy:

1. Kinetic Energy

• Energy possessed by a body due to its motion.

• Formula: $K.E. = \frac{1}{2}mv^2$, where m is mass and v is velocity.

• Example: A moving car, flowing water, or a rolling ball.

2. Potential Energy

• Energy stored in a body due to its position or configuration.

• Formula: $P.E. = mgh$, where mmm is mass, g is acceleration due to gravity, and h is height.

• Example: A stretched spring, water stored in a dam.

3. Mechanical Energy

• Sum of kinetic and potential energy in a system.

• Formula:$E = K.E. + P.E$

• Example: Energy in a swinging pendulum.

4. Thermal Energy

• Energy due to the movement of particles in a substance, also called heat energy.

• Example: Boiling water, the heat from a stove.

5. Chemical Energy

• Energy stored in chemical bonds, released during chemical reactions.

• Example: Energy in food, batteries, or fuels.

6. Electrical Energy

• Energy due to the flow of electric charges (current).

• Example: Power from batteries or electric circuits.

7. Magnetic Energy

• Energy stored in magnetic fields.

• Example: Energy in magnets or electromagnets.

8. Nuclear Energy

• Energy released during nuclear reactions (fission or fusion).

• Example: Energy in nuclear reactors or the Sun.

9. Light (Radiant) Energy

• Energy carried by electromagnetic waves, such as visible light or X-rays.

• Example: Energy from the Sun, a light bulb.

10. Sound Energy

• Energy carried by sound waves.

• Example: Energy from musical instruments or a speaker.

Unit of Energy

The SI unit of energy, Joule (J), is named after James Prescott Joule, recognising his work in energy and thermodynamics.

What is Power?

Power is a way to talk about how quickly work is being done. Work power or simply power is referred to as a physical concept that includes several meanings, depending on the context and the details available. Power can be defined as the rate of doing work. Power is the energy that is consumed per unit of time. It is present in scalar quantity as it does not have any direction. The SI unit of power is Joules/second and is termed as Watt. Watt refers to the power needed to do one Joule of work in a second. 

Example of Power

Here are a few examples of power in everyday situations:

1. Electric Bulb: A 60-watt light bulb uses 60 joules of energy every second to produce light and heat.

2. Car Engine: A car engine with 100 horsepower ($1 \, \text{HP} = 746 \, \text{W}$) delivers $74,600 \, \text{W}$ of power to accelerate the vehicle.

3. Weightlifting: If a person lifts a 50 kg weight to a height of 2 metres in 5 seconds, the power is: $P = \frac{W}{t} = \frac{mgh}{t} = \frac{50 \cdot 9.8 \cdot 2}{5} = 196 \, \text{W}$.

4. Electric Appliances: A refrigerator rated at 200 watts consumes energy at the rate of 200 joules per second.

Work, Energy and Power Important Concept for JEE Main

Name of the Concept	Key Points of the Concepts
1. Work done by variable force	Suppose we have to calculate work done in moving a body from a point A($S_A$) to a point B($S_B$) under the action of a variable force.  So the work done by this variable force in displacing a body from point $A$ to $B$ is, $W=\int_{S_B}^{S_A}\overrightarrow{F}.\overrightarrow{ds}$
2. Conservative and non-conservative forces	A force is said to be conservative if work done by or against the force in moving a body depends only on the initial and final position of the body, and not on the natural path followed between the initial and final positions. If the work done by or against a force in moving a body from one position to another relies on the path taken between these two places, the force is said to be non-conservative.
3. Power definition and relation between work and power	Power is defined as the rate at which work is completed in a given amount of time. The relation between work ($W$) and power ($P$) is expressed as  $P=\dfrac{W}{t}$
4. Kinetic energy and linear momentum relation	The energy of a body is defined as the capability or ability of the body to do the work. The relation between kinetic energy ($K.E.$) and linear momentum($P$) shows that a body cannot have kinetic energy without having linear momentum and vice-versa. $K.E.=\dfrac{p^2}{2m}$
5. Work energy theorem	This theorem states that if some work ($W$) is done by the body then the kinetic energy($K.E.$) of the body also increases by the same amount. Work done = increase in K.E. of the body
6. Potential energy of a spring	The potential energy ($P.E.$) of the spring is the energy associated with the state of compression or expansion of an elastic spring. It is given as $P.E.=\dfrac{1}{2}kx^2$ Here, $k$ = spring constant and $x$ = stretch or compression in the string
7. Mechanical energy and its conservation	The total mechanical energy of the system is conserved if the forces doing work on the system are conservative i.e, when net work done by external non-conservative force is zero. The mechanical energy ($E$) of a body is the sum of kinetic energy ($K$) and potential energy ($V$) of the body. Mathematically, we can write it as $E=K+V=\text{constant}$
8. Collision and its types	When two things bump or strike against each other, it is referred to as a collision. There are two types of collision, Elastic collision: A collision in which there is no kinetic energy loss at all. Inelastic collision: A collision that results in some loss of kinetic energy. The basic characteristics of elastic collision are: Linear momentum is conserved Total energy of the system is conserved. The kinetic energy is conserved. The basic characteristics of inelastic collision are: Linear momentum is conserved. Total energy is conserved. Kinetic energy is not conserved.

List of Important Formulas for Work Energy and Power Chapter

What are the Differences Between Work and Energy?

Work and energy are two fundamental concepts in physics that are often used interchangeably, but they have distinct meanings and applications.

The key difference between work and energy lies in their role in physical processes. Work is the process of transferring energy from one object to another, while energy is the inherent ability of an object to do work.

To illustrate this difference, consider the following example: A person lifts a book from the floor to a shelf. The person applies force to the book, causing it to move upwards. This process involves work, as the person's force transfers energy from their muscles to the book. The book now possesses potential energy due to its elevated position. If the book were to fall off the shelf, its potential energy would be converted into kinetic energy as it falls, eventually doing work on the floor by impact.

If you want to know more differences between Work and Energy, check Vedantu’s page on the Difference Between Work and Energy.

You can also check Meaning, Differences, and FAQs on the Difference between Work, Energy, and Power on Vedantu’s page.

Is Formula for Power Important for JEE Main?

Yes, the formula for power is important for JEE Main. Power is a fundamental concept in physics, and it is used in a variety of problems on the JEE Main exam. The formula of power is P = $\frac{W}{t}$ , where P is power, W is work, and t is time.

Here are some examples of how the Power formula in Physics is used on the JEE Main exam:

1. A light bulb consumes 60 watts of power. How much work does it do in 1 hour?

2. A car accelerates from 0 to 60 mph in 10 seconds. What is the power of the car's engine?

3. A pump lifts 100 liters of water to a height of 10 meters in 5 minutes. What is the power of the pump?

As you can see, the formula for power is a versatile tool that can be used to solve a variety of problems on the JEE Main exam. Therefore, it is important to understand the formula and how to use it.

Power Formula for Electricity

In electricity, power is the rate at which electrical energy is transferred. It is measured in watts (W). The power equation is:

P = VI

where:

P is power in watts (W)

V is voltage in volts (V)

I is current in amperes (A).

This formula tells us that the power consumed by an electrical device is directly proportional to the voltage applied to it and the current flowing through it.

Examples of Power Calculations:

Here are some examples of how to use the power formula:

1. A light bulb with a resistance of 10 ohms is connected to a 12-volt battery. What is the power consumed by the light bulb?

P = VI = (12V) (1A) = 1W

2. A motor draws 5 amperes of current from a 24-volt power supply. What is the power consumed by the motor?

P = VI = (24V) (5A) = 120W

3. A household appliance has a power rating of 1,000 watts. How much current does it draw from a 120-volt outlet?

I = $\frac{\text{P}}{\text{V}} = \frac{1,000 \, \text{W}}{120 \, \text{V}} = 8.33 \, \text{A}$

Real-World Applications of Power Formula

The power formula is used in a variety of real-world applications, such as:

• Designing Electrical Circuits: Engineers use the power formula to design electrical circuits that can deliver the required amount of power to various devices.

• Calculating Electrical Bills: Electricity companies use the power formula to calculate the amount of electricity consumed by their customers.

• Sizing Fuses and Circuit Breakers: Fuses and circuit breakers are designed to protect electrical circuits from overloading. The power formula is used to determine the appropriate size of fuse or circuit breaker for a given circuit.

How is Work Different from Power?

You can explore further elaboration on the distinctions between work and power to gain a clearer understanding.

How is Work Different from Energy?

To gain a better comprehension, delve deeper into the distinguish between work and power.

How is Power Different from Energy?

You can delve into a more comprehensive explanation of distinguishing between work and power, To gain a better grasp. You can also go through the Difference Between work energy and power provided in detail along with their characteristics.

All Formulas of Electricity Class 10 For JEE Main 

In the realm of physics, understanding the fundamentals of electricity is crucial, and this holds particularly true for students preparing for competitive exams like JEE Main. This introduction serves as a gateway to comprehending all the essential electricity formulas from Class 10. From Ohm's Law to power calculations, formulas of electricity class 10 lay the foundation for solving intricate problems. As we delve into this comprehensive compilation, students will gain a holistic perspective on the principles governing electrical phenomena, providing them with the tools needed to excel in their JEE Main examinations. 

Here are the essential electricity class 10 formulas for electricity for the JEE Main exam:

• Ohm's Law

Ohm's law is a fundamental principle in electricity that states that the current through a conductor is directly proportional to the voltage across it and inversely proportional to its resistance.

Formula:

I = $\frac{\text{V}}{\text{R}}$

where:

(I) is the current in amperes (A),

(V) is the voltage in volts (V),

(R) is the resistance in ohms $(\Omega)$

• Resistance

Resistance is a measure of a material's opposition to the flow of electric charge. It is measured in ohms $(Omega)$ .

Formula:

R = $\frac{\rho l}{A}$

where:

(R) is the resistance in ohms $(\Omega)$ ,

$(\rho)$ is the resistivity of the material in ohm-meters $(\Omega \cdot \text{m})$ ,

(l) is the length of the conductor in meters $(\text{m})$ ,

(A) is the cross-sectional area of the conductor in square meters $(\text{m}^2)$

• Resistivity

Resistivity is a property of a material that describes its resistance to the flow of electric charge. It is measured in ohm-meters $(\Omega \cdot \text{m})$ .

Formula:

$\rho = \frac{1}{\sigma}$

where:

$(\rho)$ is the resistivity of the material in ohm-meters $(\Omega \cdot \text{m})$ ,

$(\sigma)$ is the conductivity of the material in siemens per meter (S/m).

• Current

Current is the rate of flow of electric charge through a conductor. It is measured in amperes (A).

Formula:

I = $\frac{Q}{t}$

where:

I is the current in amperes (A)

Q is the charge in coulombs (C)

t is the time in seconds (s)

• Voltage

Voltage is the electrical potential difference between two points in a circuit. It is measured in volts (V).

Formula:

V = $\frac{W}{Q}$

where:

V is the voltage in volts (V)

W is the work done in joules (J)

Q is the charge in coulombs (C)

• Power

Power is the rate at which work is done. It is measured in watts (W).

Formula:

P = $\frac{W}{t}$

where:

P is the power in watts (W)

W is the work done in joules (J)

t is the time in seconds (s)

Kirchhoff's Laws

Kirchhoff's laws are two fundamental principles in electricity that describe the behavior of currents and voltages in electrical circuits.

• Kirchhoff's First Law (Junction Law)

The algebraic sum of the currents entering a junction is zero.

• Kirchhoff's Second Law (Loop Law)

The algebraic sum of the voltages around a closed loop is zero.

These formulas provide a solid foundation for understanding and solving problems related to electricity in JEE Main. By mastering these concepts, students can effectively analyze electrical circuits, calculate currents and voltages, and design electrical systems.

JEE Main Work, Energy and Power Solved Examples 

1. A particle travels in a straight path, with retardation proportional to displacement. Calculate the kinetic energy loss for every displacement $x$.

Sol:

It is given that retardation($a$) is proportional to displacement ($x$). So in order to solve this problem, we first write retardation in terms of velocity and after separating and integrating the quantities in the given relation, we will be able to reach the answer.

According to the question,

$-a\varpropto x$

$-a=kx$...........(1)

Here, $k$ is the proportionality constant.

Now putting $a=\dfrac{\text{d}v}{\text{d}t}$ in the eq.(1), we get:

$-a=-\dfrac{\text{d}v}{\text{d}t} = kx$

Now after multiplying and dividing on L.H.S by $\text{d}x$, we get:

$\dfrac{\text{d}v}{\text{d}t} \dfrac{\text{d}x}{\text{d}x} =-kx$

As $\dfrac{\text{d}x}{\text{d}t}=v$, therefore, $v\text{d}v=-kx\text{d}x$

$\int_{v}^{0}vdv=-k\int_{x}^{0}xdx$

$[\dfrac{v^2}{2}]^v_u=-k[\dfrac{x^2}{2}]^x_0$

$\dfrac{1}{2}mv^2-\dfrac{1}{2}mu^2=\dfrac{-kx^2m}{2}$

Thus, the loss in kinetic energy is $\Delta K=\dfrac{-kmx^2}{2}$.

Trick: Use the given condition of the question and put the retardation formula in terms of velocity then separate the similar quantities and integrate them.

2. A body falls to the earth from a height of $8,m$ and then bounces to a height of $2,m$. Calculate the ratio of the body's velocities right before and after the contact. Calculate the body's kinetic energy loss as a percentage during the contact with the ground.

Sol:

It is given that heights $h_1=8\,m$ and $h_2=2\,m$. Let $v_1$ be the velocity of the body just before collision with the ground and $v_2$ be the velocity of the body just after collision.

Now, according to the conservation of mechanical energy, we can write:

$\dfrac{1}{2}mv_1^2=mgh_1$ and $\dfrac{1}{2}mv_2^2=mgh_2$

After dividing the above equation, we get:

$\dfrac{v^2_1}{v^2_2}=\dfrac{h_1}{h_2}$

Putting the values of $h_1$ and $h_2$, we get:

$\dfrac{v^2_1}{v^2_2}=\dfrac{8}{2}=4$

$\dfrac{v_1}{v_2}=2$

Therefore, the ratio of the velocities is 2:1.

Now, the percentage loss in kinetic energy is given as:

$\% \text{ age loss in K.E}=(\dfrac{K_1-K_2}{K_1}\times 100$ 

$\% \text{ age loss in K.E}=\dfrac{mg(h_1-h_2)}{mgh_1}\times 100$

$\% \text{ age loss in K.E}=\dfrac{(8-2)}{8}\times 100$

$\% \text{ age loss in K.E}= 75\%$

Hence, the percentage loss in kinetic energy is 75 %.

Key point: The laws of conservation of mechanical energy is an important concept and so is the loss of kinetic energy expression.

Previous Years’ Questions from JEE Paper

1. A block moving horizontally on a smooth surface with a speed of $40\,m/s$ splits into two equal parts. If one of the parts moves at $60\,m/s$ in the same direction, then the fractional change in the kinetic energy will be $x : 4$ where $x$ = ___________. (JEE Main 2021)

Sol: 

Given:

Initial velocity of the block before splitting into two equal parts, $V=40\,m/s$

As the block is split into two equal masses, so if we consider the initial mass of the block $m$ then after splitting, it will be $\dfrac{m}{2}$. Let the velocity of 1st part be $v$ and the velocity of the second part according to the question is $v’=60\,m/s$.

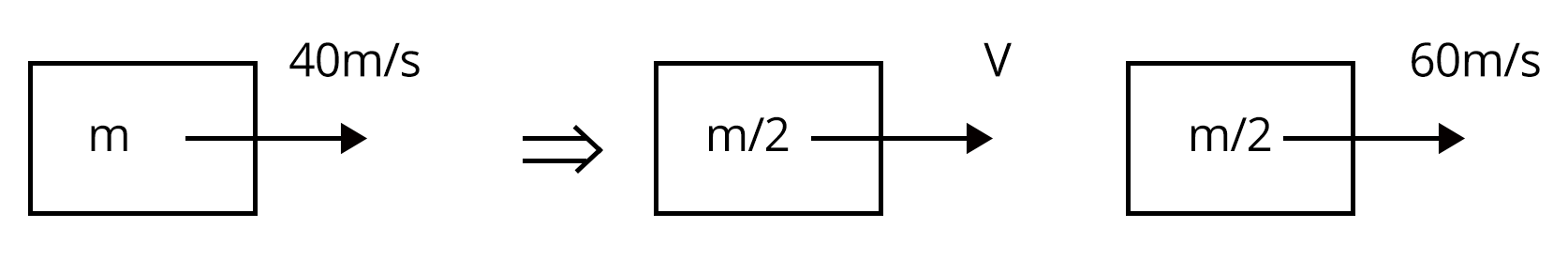
Now, using the momentum conservation principle, we get:

$P_i=P_f$

$mV=\dfrac{m}{2} v+\dfrac{m}{2} v’$

$V=\dfrac{v}{2} +\dfrac{v’}{2}=\dfrac{v}{2}+\dfrac{60}{2}$

$40=\dfrac{v}{2}+30$

$v=10\times 2=20\,m/s$

Now, the initial kinetic energy ($(K.E.)_i$) is

$(K.E.)_i = \dfrac{1}{2}mV^2=\dfrac{1}{2}m(40)^2=800m$

The final kinetic energy ($(K.E.)_f$) is

$(K.E.)_f = \dfrac{1}{2}\dfrac{m}{2}(20)^2+\dfrac{1}{2}\dfrac{m}{2}(60)^2=1000m$

The change in kinetic energy ($\Delta K.E.$) is

$\lvert\Delta K.E.\rvert=\lvert 1000m-800m\rvert=200m$

The fractional change in kinetic energy is

$\text{fractional change in K.E.}=\dfrac{\Delta K.E.}{(K.E.)_i}=\dfrac{200 m}{800m}$

$\text{fractional change in K.E.}= \dfrac{1}{4}=\dfrac{x}{4}$

Hence, the value of $x$ is $1$.

Trick: Just apply the concept of conservation of momentum then fraction change in the kinetic energy.

1. A force of $F = (5y + 20) \widehat{j}\,N$ acts on a particle. The work done by this force when the particle is moved from $y = 0\,m$ to $y = 10\,m$ is ___________ J. (JEE Main 2021)

Sol:

Given:

A variable force expression, $F = (5y + 20) \widehat{j}\,N$

Moved from $y = 0\,m$ to $y = 10\,m$

The work done under the variable force is given by

$W=\int Fdy=\int_{0}^{10} Fdy$

Now, putting the value of $F$ in the above expression and integrating it, we get:

$W=\int_{0}^{10} (5y + 20)dy$

$W=(\dfrac{5y^2}{2}+2y)^{10}_0$

$W=\dfrac{5}{2}\times 100+2\times 10$

$W=250+200=450\,J$

Therefore, the value of work done is 450 J.

Key point: The expression of work done for variable forces is the important key to solve this problem.

Practice Questions

1. A 10 kg ball and a 20 kg ball collide at velocities 20 m/s and 10 m/s, respectively. What are their post-collision velocities if the collision is completely elastic? 

Ans: -20 m/s; 10 m/s

2. A spring gun has a spring constant of 80 N/cm. A ball of mass 15g compresses the spring by 12 cm. How much is the potential energy of the spring? What is the velocity of the ball if the trigger is pulled? 

Ans: 57.6 J; 87.6 m/s

JEE Main Physics Work, Energy and Power Study Materials

Here, you'll find a comprehensive collection of study resources for Work, Energy and Power designed to help you excel in your JEE Main preparation. These materials cover various topics, providing you with a range of valuable content to support your studies. Simply click on the links below to access the study materials of Work, Energy and Power and enhance your preparation for this challenging exam.

JEE Main Physics Study and Practice Materials

Explore an array of resources in the JEE Main Physics Study and Practice Materials section. Our practice materials offer a wide variety of questions, comprehensive solutions, and a realistic test experience to elevate your preparation for the JEE Main exam. These tools are indispensable for self-assessment, boosting confidence, and refining problem-solving abilities, guaranteeing your readiness for the test. Explore the links below to enrich your Physics preparation.

Conclusion

The "Work, Energy, and Power" chapter in the JEE Main syllabus is a cornerstone of physics that unveils the principles governing motion, transformation of energy, and the concept of power. It equips aspiring engineers and scientists with the tools to understand and solve intricate problems related to kinetic and potential energy, work done by forces, and the rate at which work is performed. Beyond exam success, the knowledge gained here is instrumental in comprehending real-world phenomena, from machinery to the cosmos. It empowers students to analyze and optimize systems, making it an indispensable component of their scientific journey.

JEE Main 2025 Subject-Wise Important Chapters

The JEE Main 2025 subject-wise important chapters provide a focused strategy for Chemistry, Physics, and Maths. These chapters help students prioritise their preparation, ensuring they cover high-weightage topics for better performance in the exam.

Important Study Materials Links for JEE Exams 2025