What is the Fractional of a Hundred?

In Maths, a fraction is used to symbolise the element or part of the entirety. It represents the equal elements of the entire. A fraction has two parts, namely numerator and denominator. The number on the top is called the numerator, and the number on the bottom is called the denominator. The numerator defines the number of identical parts taken, whereas the denominator defines the entire quantity of the same components in a whole.

For instance, $\dfrac{5}{10}$ is a fraction. Here, 5 is a numerator, and 10 is a denominator. So what is the fractional of a hundred? In this article, you'll learn how to find the fractional of 100.

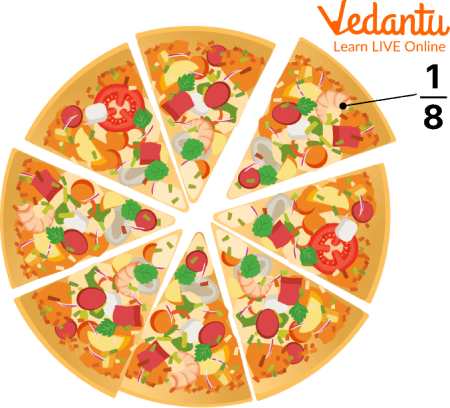
Fraction

Types of Fractions

There are four different varieties of fractions. They are:

• Unit Fraction: In a fraction, the numerator with 1 is known as a unit fraction. For instance, $\dfrac{1}{2}$,$\dfrac{1}{4}$

• Proper Fraction: If a numerator is less than the denominator, it is known as a proper fraction. $\dfrac{7}{9}$,$\dfrac{8}{10}$

• Improper Fraction: If a numerator is greater than the denominator, it is called an improper fraction. Example: $\dfrac{11}{2}$,$\dfrac{6}{4}$

• Mixed Fraction: If a fraction includes a whole number with a proper fraction, it is called a mixed fraction. Example five $5\dfrac{1}{2}$,$10\dfrac{1}{4}$

Other Types of Fractions

• Like Fractions: The fractions with the identical denominator are called like fractions.

Example: $\dfrac{4}{2}, \dfrac{7}{2}, \dfrac{9}{2}$

Here, the denominators of all of the fractions are 2. Hence, they're referred to as like fractions.

• Unlike Fractions: The fractions with distinct denominators are known as unlike fractions.

Example: $\dfrac{5}{2}, \dfrac{4}{6}, \dfrac{9}{4}$

Here, the denominator values are one-of-a-kind in all of the fractions. Hence, they're referred to as in-contrast-to fractions.

• Equivalent Fractions: If two fractions bring about identical values after simplification, they're equal to every different.

Example: $\dfrac{2}{3}$ and $\dfrac{4}{6}$ are equal fractions, given that $\dfrac{4}{6} = \dfrac{(2 \times 2)}{(2 \times 3)}=\dfrac{2}{3}$

Fraction on a Number Line

Representing fractions on a number line implies that we can plot fractions on a number line in the same way that we can plot whole numbers and integers. Parts of a whole are represented by fractions. On the number line, fractions are represented by making equal portions of a whole, i.e. 0 to 1, and the number of those equal parts is the same as the number given in the fraction's denominator.

For example, if we need to represent $\dfrac{1}{8}$ on the number line, we need to mark 0 and 1 on the 2 ends and divide the number line into eight identical parts. And then mark the point which we have to represent.

Fraction on Number Line

Solved Examples

Q1. Write five hundredths in decimal form.

Ans: Since we know that one-tenth is $\dfrac{1}{10}.$ and one-hundredths is $\dfrac{1}{100}$ and so on.

So, when we are expanding any number which is having a decimal point.

After the decimal point, the place values of digits from left to right are tenth, hundredths, thousandth, and so on.

In digits these values are $\dfrac{1}{10}, \dfrac{1}{100}, \dfrac{1}{1000} \ldots$

So,

5 hundredths $=\dfrac{5}{100}$

In decimal form, it is $0.05$.

Therefore, five hundredths in the decimal form will be $0.05$.

Q2. What will be the fractional of 100?

Ans: A fraction is made up of two parts. The number on the top of the line is called the numerator. The number below the line is called the denominator.

In this case, we need to express 100 as a fraction.

Since 20 is a whole number, its denominator will predominantly be 1, and the numerator will remain $100 .$

Since a fraction $=$ numerator $/$ denominator

Thus, the fractional of 100 will be $\dfrac{100}{1}.$

Practice Problems

Q1. What will be the decimal form of $\dfrac{5}{10}$?

Ans: 0.5

Q2. What will be the fractional form of 0.44?

Ans: $\dfrac{44}{100}$

Summary

In this article, we learned about fractions and different types of fractions. Fractions are represented as numerical value, which defines a part of a whole. A fraction can be a portion or section of any quantity out of a whole, whereas the whole can be any number, a specific value, or a thing. Then we learned how to write five hundredths in decimal form and fractional of 100.