
Answer
479.4k+ views
Hint: To draw a line segment of length 7.6 cm, we simply have to plot two points (say A and B) which are 7.6 cm apart and then draw a line through them. To divide them in a particular ratio, we make use of properties of ratio and proportions such that if ratio is a:b, one portion of line segment is $\dfrac{b}{a}$times the other segment.
Complete step-by-step solution:
Line segment is a part of a line that is bounded by two distinct end points. Thus, to draw them we are going to take two points A and B. Then we will mark B at a distance of 7.6 cm apart from A and then join them by a line. Thus, to show this,

Thus, above shown is a line segment AB which is of 7.6 cm (Since, A and B are 7.6 units (here, units are in cm) apart as shown).
Now to divide the line segment in the ratio of 5:8, we proceed as follows-
Let, there be an internal point C (that is, C is in between A and B), which divides AB in ratio of 5:8. Thus, we would have,
$\dfrac{AC}{CB}=\dfrac{5}{8}$
AC = $\dfrac{5}{8}BC$
Now, AB = AC+CB (apparent from the figure below)
7.6 = $\dfrac{5}{8}BC$+BC
7.6 = $\dfrac{13}{8}BC$
BC = $\dfrac{8\times 7.6}{13}$
BC = $\dfrac{60.8}{13}$
Since, AC = $\dfrac{5}{8}BC$, we have,
AC = $\dfrac{5}{8}\times \dfrac{60.8}{13}$
AC = $\dfrac{304}{104}$
AC = $\dfrac{38}{13}$
Thus, now we can mark point C that divides AB in a ratio of 5:8. We have,
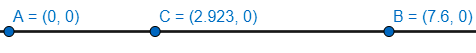
Here, AC = $\dfrac{38}{13}$=2.923 cm
Thus, we have point C, which divides AB in the ratio of 5:8.
Note: Another simpler way to proceed with questions involving ratio and proportions (say ratio of a: b) is to assume the proportions to be ak and bk (where k is a constant). So, in this case, the proportions would be 5k and 8k (since, the ratio is 5:8). Now, since, length is 7.6 cm, we have,
5k+8k = 7.6
13k =7.6
k = $\dfrac{7.6}{13}$
Thus, to divide AB by a point C (where C divides AB in ratio 5:8). Thus,
AC = 5k and CB=8k
We have,
AC = $\dfrac{38}{13}$
BC = $\dfrac{60.8}{13}$
Which is the same as the solution above.
Complete step-by-step solution:
Line segment is a part of a line that is bounded by two distinct end points. Thus, to draw them we are going to take two points A and B. Then we will mark B at a distance of 7.6 cm apart from A and then join them by a line. Thus, to show this,

Thus, above shown is a line segment AB which is of 7.6 cm (Since, A and B are 7.6 units (here, units are in cm) apart as shown).
Now to divide the line segment in the ratio of 5:8, we proceed as follows-
Let, there be an internal point C (that is, C is in between A and B), which divides AB in ratio of 5:8. Thus, we would have,
$\dfrac{AC}{CB}=\dfrac{5}{8}$
AC = $\dfrac{5}{8}BC$
Now, AB = AC+CB (apparent from the figure below)
7.6 = $\dfrac{5}{8}BC$+BC
7.6 = $\dfrac{13}{8}BC$
BC = $\dfrac{8\times 7.6}{13}$
BC = $\dfrac{60.8}{13}$
Since, AC = $\dfrac{5}{8}BC$, we have,
AC = $\dfrac{5}{8}\times \dfrac{60.8}{13}$
AC = $\dfrac{304}{104}$
AC = $\dfrac{38}{13}$
Thus, now we can mark point C that divides AB in a ratio of 5:8. We have,
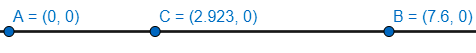
Here, AC = $\dfrac{38}{13}$=2.923 cm
Thus, we have point C, which divides AB in the ratio of 5:8.
Note: Another simpler way to proceed with questions involving ratio and proportions (say ratio of a: b) is to assume the proportions to be ak and bk (where k is a constant). So, in this case, the proportions would be 5k and 8k (since, the ratio is 5:8). Now, since, length is 7.6 cm, we have,
5k+8k = 7.6
13k =7.6
k = $\dfrac{7.6}{13}$
Thus, to divide AB by a point C (where C divides AB in ratio 5:8). Thus,
AC = 5k and CB=8k
We have,
AC = $\dfrac{38}{13}$
BC = $\dfrac{60.8}{13}$
Which is the same as the solution above.
Recently Updated Pages
How many sigma and pi bonds are present in HCequiv class 11 chemistry CBSE

Mark and label the given geoinformation on the outline class 11 social science CBSE

When people say No pun intended what does that mea class 8 english CBSE

Name the states which share their boundary with Indias class 9 social science CBSE

Give an account of the Northern Plains of India class 9 social science CBSE

Change the following sentences into negative and interrogative class 10 english CBSE

Trending doubts
Fill the blanks with the suitable prepositions 1 The class 9 english CBSE

The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

In Indian rupees 1 trillion is equal to how many c class 8 maths CBSE

Which are the Top 10 Largest Countries of the World?

How do you graph the function fx 4x class 9 maths CBSE

Give 10 examples for herbs , shrubs , climbers , creepers

Difference Between Plant Cell and Animal Cell

Difference between Prokaryotic cell and Eukaryotic class 11 biology CBSE

Why is there a time difference of about 5 hours between class 10 social science CBSE



