Answer
375.6k+ views
Hint: While solving the problem apply the sign convention rule for spherical mirrors. The object is placed to the left of the mirror at a distance ‘u’. This implies that the light from the object falls on the mirror from the left side. According to the sign convention rule, the distances measured from the right of the mirror (+ve x-axis direction) are taken to be positive whereas all distances measured from the left side of the mirror (-ve x-axis direction) are taken to be negative.
Complete step-by-step solution:
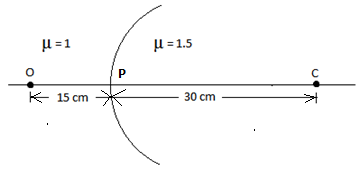
Given: \[{\mu _1} = {\text{ }}1;{\text{ }}{\mu _2} = {\text{ }}1.5\]
Radius of curvature,\[R = 30{\text{ }}cm\] ;
Object distance \[u = {\text{ }} - 15{\text{ }}cm\;\;\;\;\] (-ve because it is in the direction opposite to the direction of the incident light)
To find: Image distance, v
Substitute the given values into equation (1)
$\dfrac{{{{{\mu }}_2}}}{{{v}}} - \dfrac{{{{{\mu }}_1}}}{u} = \dfrac{{{{{\mu }}_2} - {{{\mu }}_1}}}{{{R}}}$
$\Rightarrow$$\dfrac{{1.5}}{{\text{V}}} - \dfrac{1}{{ - 15}} = \dfrac{{1.5 - 1}}{{30}}$
Simplifying and rearranging,
$\Rightarrow$$\dfrac{{1.5}}{{\text{V}}} + \dfrac{1}{{15}} = \dfrac{{0.5}}{{30}}$
$\Rightarrow$$\dfrac{{1.5}}{{\text{V}}} = \dfrac{{0.5}}{{30}} - \dfrac{1}{{15}}$
$\Rightarrow$$\dfrac{{1.5}}{{\text{V}}} = \dfrac{{0.5}}{{30}} - \dfrac{2}{{30}}$
$\Rightarrow$$\dfrac{{1.5}}{{\text{V}}} = \dfrac{{2 - 0.5}}{{30}} = \dfrac{{ - 1.5}}{{30}}$
$\Rightarrow$$\dfrac{1}{{\text{V}}} = \dfrac{{ - 1}}{{30}}$
Reciprocal, \[v = - 30{\text{ }}cm\]
The image is formed \[30\] cm to the left of the mirror and is virtual.
Note: The reflecting surface of a concave mirror is curved inwards. On the other hand, for a convex mirror, the reflective surface is curved outwards. A convex mirror is also referred to as a diverging mirror. The image formed by a convex mirror is always virtual, erect, and diminished whereas for a concave mirror the image formed can be real and inverted or virtual and erect. These spherical mirrors have a wide range of applications. In torches, vehicle headlights, and searchlights concave mirrors are utilized while convex mirrors are used as rear-view mirrors in vehicles.
Complete step-by-step solution:

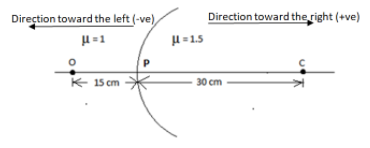
Given: \[{\mu _1} = {\text{ }}1;{\text{ }}{\mu _2} = {\text{ }}1.5\]
Radius of curvature,\[R = 30{\text{ }}cm\] ;
Object distance \[u = {\text{ }} - 15{\text{ }}cm\;\;\;\;\] (-ve because it is in the direction opposite to the direction of the incident light)
To find: Image distance, v
Substitute the given values into equation (1)
$\dfrac{{{{{\mu }}_2}}}{{{v}}} - \dfrac{{{{{\mu }}_1}}}{u} = \dfrac{{{{{\mu }}_2} - {{{\mu }}_1}}}{{{R}}}$
$\Rightarrow$$\dfrac{{1.5}}{{\text{V}}} - \dfrac{1}{{ - 15}} = \dfrac{{1.5 - 1}}{{30}}$
Simplifying and rearranging,
$\Rightarrow$$\dfrac{{1.5}}{{\text{V}}} + \dfrac{1}{{15}} = \dfrac{{0.5}}{{30}}$
$\Rightarrow$$\dfrac{{1.5}}{{\text{V}}} = \dfrac{{0.5}}{{30}} - \dfrac{1}{{15}}$
$\Rightarrow$$\dfrac{{1.5}}{{\text{V}}} = \dfrac{{0.5}}{{30}} - \dfrac{2}{{30}}$
$\Rightarrow$$\dfrac{{1.5}}{{\text{V}}} = \dfrac{{2 - 0.5}}{{30}} = \dfrac{{ - 1.5}}{{30}}$
$\Rightarrow$$\dfrac{1}{{\text{V}}} = \dfrac{{ - 1}}{{30}}$
Reciprocal, \[v = - 30{\text{ }}cm\]
The image is formed \[30\] cm to the left of the mirror and is virtual.
Note: The reflecting surface of a concave mirror is curved inwards. On the other hand, for a convex mirror, the reflective surface is curved outwards. A convex mirror is also referred to as a diverging mirror. The image formed by a convex mirror is always virtual, erect, and diminished whereas for a concave mirror the image formed can be real and inverted or virtual and erect. These spherical mirrors have a wide range of applications. In torches, vehicle headlights, and searchlights concave mirrors are utilized while convex mirrors are used as rear-view mirrors in vehicles.
Recently Updated Pages
How many sigma and pi bonds are present in HCequiv class 11 chemistry CBSE

Mark and label the given geoinformation on the outline class 11 social science CBSE

When people say No pun intended what does that mea class 8 english CBSE

Name the states which share their boundary with Indias class 9 social science CBSE

Give an account of the Northern Plains of India class 9 social science CBSE

Change the following sentences into negative and interrogative class 10 english CBSE

Trending doubts
Fill the blanks with the suitable prepositions 1 The class 9 english CBSE

The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

In Indian rupees 1 trillion is equal to how many c class 8 maths CBSE

Which are the Top 10 Largest Countries of the World?

How do you graph the function fx 4x class 9 maths CBSE

Give 10 examples for herbs , shrubs , climbers , creepers

Difference Between Plant Cell and Animal Cell

Difference between Prokaryotic cell and Eukaryotic class 11 biology CBSE

Why is there a time difference of about 5 hours between class 10 social science CBSE