Question

In the below figure, $PR>PQ$ and $PS$ bisects $\angle QPR$ . Prove that $\angle PSR>\angle PSQ$ .

Answer 1

Hint: We use the property that the angle opposite the greater side is greater, which gives $\angle PQR>\angle PRQ$ . We now compare the two triangles $\Delta PQS$ and $\Delta PSR$ . One of the angles of the two triangles being the same and one angle being greater than the other $\left( \angle PQR>\angle PRQ \right)$ , we must then have the remaining angle smaller than the other of the second triangle.

Complete step by step answer:
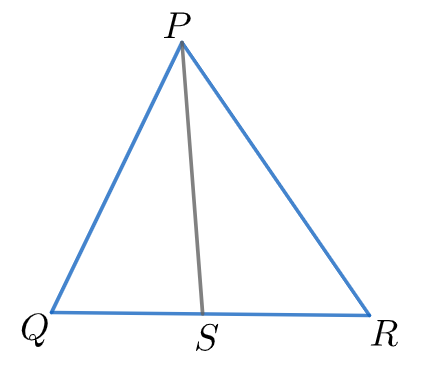
Let us start off the solution by saying that $PS$ is an angular bisector and it bisects $\angle QPR$ . This means that
$\angle QPS=\angle SPR....\left( 1 \right)$
Also, we are given that $PR>PQ$ . We know that in a triangle, the side opposite the greater angle is greater. In reverse, it can be said that the angle opposite the greater side is greater in a triangle. In this triangle $\Delta PQR$ , outside of sides $PQ$ and $PR$ , $PR$ is the greater side. The angle opposite to side $PR$ is $\angle PQR$ . This means that
$\angle PQR>\angle PRQ....\left( 2 \right)$
We know the property of a triangle that the sum of all the internal angles of a triangle is equal to ${{180}^{\circ }}$ . This means that the sum of internal angles of $\Delta PQS$ and $\Delta PRS$ separately are ${{180}^{\circ }}$ . This means,
$\angle PQS+\angle PSQ+\angle QPS={{180}^{\circ }}....\left( 3 \right)$
And
$\angle PRS+\angle PSR+\angle SPR={{180}^{\circ }}....\left( 4 \right)$
By $\left( 3 \right)$ and $\left( 4 \right)$ , we can say that
$\Rightarrow \angle PQS+\angle PSQ+\angle QPS=\angle PRS+\angle PSR+\angle SPR$
By $\left( 1 \right)$ , we can cancel the last terms from both the sides of the equation. The equation thus becomes,
$\Rightarrow \angle PQS+\angle PSQ=\angle PRS+\angle PSR$
We now subtract $\angle PRS$ from both sides of the above equation, We get,
$\Rightarrow \angle PQS-\angle PRS+\angle PSQ=\angle PSR$
We now subtract $\angle PSQ$ from both sides of the above equation, We get,
$\Rightarrow \angle PQS-\angle PRS=\angle PSR-\angle PSQ$
By $\left( 2 \right)$ we can say that the left hand side of the above equation is positive. This means that the right hand side of the above equation is also positive. For the right hand side to be positive, $\angle PSR>\angle PSQ$ .
Therefore, it has been proved that $\angle PSR>\angle PSQ$ .

Note: In these problems, the most common problem that students face is that they cannot find out how to start the problem. An easy way for it is to observe the given conditions and find out, which property is related to these conditions. We also make mistakes while naming the angles and so, we should be careful here.