Question

If the sides of a triangle are proportional to the cosines of the opposite angles then the triangle is \[\]
A. Right Angled\[\]
B. Equilateral \[\]
C. Obtuse Angled\[\]
D. None of these \[\]

Answer 1

Hint: We use the sine law of triangle $\dfrac{a}{\sin A}=\dfrac{b}{\sin B}=\dfrac{c}{\sin C}$ and given condition from the question to make the equation $\dfrac{\sin A}{\cos A}=\dfrac{\sin B}{\cos B}=\dfrac{\sin C}{\cos C}$. We take first two terms of the equation and use the angle difference e formula of sine $\sin \left( \alpha -\beta \right)=\sin \alpha \cos \beta -\cos \alpha \sin \beta $ to find relation between $A,B$. We follow the same procedure for the second two terms and find a relation between $B,C$. We find a relation between $A,B,C$ to choose the correct option. \[\]

Complete step by step answer:
We know from sine difference of formula that for two angles with measures $\alpha ,\beta $ we have,
\[\sin \left( \alpha -\beta \right)=\sin \alpha \cos \beta -\cos \alpha \sin \beta \]
We draw the figure of the triangle ABC and denote the measure of the angle subtended at vertices A,B,C are given as $A,B,C$ respectively and length of the sides opposite to vertices A,B,C as $a,b,c$. We have the rough figure as,\[\]
 

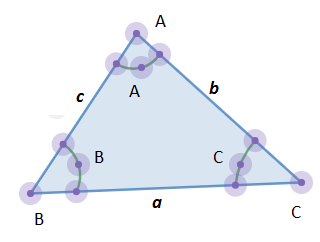
We know from sine law which states that sides of a triangle are proportional to sin of the angle opposite to them which means
\[\dfrac{a}{\sin A}=\dfrac{b}{\sin B}=\dfrac{c}{\sin C}\]
Let us assume for some constant $k$ ,
\[\begin{align}
  & \dfrac{a}{\sin A}=\dfrac{b}{\sin B}=\dfrac{c}{\sin C}=k \\
 & \Rightarrow a=k\sin A,b=k\sin B,c=k\sin C.......\left( 1 \right) \\
\end{align}\]
We are given in the question that the sides of a triangle are proportional to the cosines of the opposite angles. So we have
\[\dfrac{a}{\cos A}=\dfrac{b}{\cos B}=\dfrac{c}{\cos C}\]
We put the values of $a,b,c$ obtain din equation (1) in the above proportion to have,
\[\begin{align}
  & \dfrac{k\sin A}{\cos A}=\dfrac{k\sin B}{\cos B}=\dfrac{k\sin C}{\cos C} \\
 & \Rightarrow \dfrac{\sin A}{\cos A}=\dfrac{\sin B}{\cos B}=\dfrac{\sin C}{\cos C}.......\left( 2 \right) \\
\end{align}\]
We take first two terms in the above proportion (2) and cross-multiply to have,
\[\begin{align}
  & \dfrac{\sin A}{\cos A}=\dfrac{\sin B}{\cos B} \\
 & \Rightarrow \sin A\cos B=\cos A\sin B \\
 & \Rightarrow \sin A\cos B-\cos A\sin B=0 \\
\end{align}\]
We use sine difference of angle formula for $\alpha =A,\beta =B$ in the above step to have,
\[\begin{align}
  & \Rightarrow \sin \left( A-B \right)=0 \\
 & \Rightarrow A-B=0 \\
 & \Rightarrow A=B......\left( 3 \right) \\
\end{align}\]
We take first second terms in the above proportion (2) and cross-multiply to have,
\[\begin{align}
  & \dfrac{\sin B}{\cos B}=\dfrac{\sin C}{\cos C} \\
 & \Rightarrow \sin B\cos C=\cos B\sin C \\
 & \Rightarrow \sin B\cos C-\cos B\sin C=0 \\
\end{align}\]
We use sine difference of angle formula for $\alpha =B,\beta =C$ in the above step to have,
\[\begin{align}
  & \Rightarrow \sin \left( B-C \right)=0 \\
 & \Rightarrow B-C=0 \\
 & \Rightarrow B=C.......\left( 4 \right) \\
\end{align}\]
We have from equation (3) and (4)
\[A=B=C\]
We know that only equilateral triangles have all three angles equal.

So, the correct answer is “Option B”.

Note: We note that the general solution of the equation $\sin x=0$ with arbitrary integer $n$ are given by $x=n\pi $ but when we calculated $A-B=0,B-C=0$ we have taken $n=0$ and rejected all other values of $n$ because the difference of two angles cannot be greater than or equal to $\pi $ in a triangle. We must be careful of the confusion between the angle difference formula of sine and cosine which is $\cos \left( \alpha +\beta \right)=\cos \alpha \cos \beta -\sin \alpha \sin \beta $.