Answer
384k+ views
Hint: First, find the x-intercepts of the curve by putting $y = 0$. After that, find the y-intercept by putting $x = 0$. Then take a minimum of 5 points and plot the points. After plotting the points, join the points with a smooth freehand curve and identify the curve that we have obtained.
Complete step by step solution:
We know that the graph of a function is the locus of points $\left( {x,y} \right)$ such that $y = f\left( x \right)$ where x, y are real numbers. We are given the following quadratic polynomial function,
$ \Rightarrow y = {\left( {x + 5} \right)^2} - 3$
So, let us put $y = 0$ and find the x-intercept. We have,
$ \Rightarrow 0 = {\left( {x + 5} \right)^2} - 3$
Move 3 on the other side,
$ \Rightarrow {\left( {x + 5} \right)^2} = 3$
Take the square root on both sides,
$ \Rightarrow x + 5 = \pm \sqrt 3 $
Subtract 5 on both sides,
$ \Rightarrow x + 5 - 5 = \pm \sqrt 3 - 5$
Simplify the terms,
$ \Rightarrow x = - 5 \pm \sqrt 3 $
It means the curve cuts the x-axis at $\left( { - 5 + \sqrt 3 ,0} \right)$ and $\left( { - 5 - \sqrt 3 ,0} \right)$.
Let us put $x = 0$ and find the y-intercept. We have,
$ \Rightarrow y = {\left( {0 + 5} \right)^2} - 3$
Simplify the terms,
$ \Rightarrow y = {\left( 5 \right)^2} - 3$
Square the term on the right side,
$ \Rightarrow y = 25 - 3$
Simplify the terms,
$ \Rightarrow y = 22$
It means the curve cuts the y-axis at $\left( {0,22} \right)$.
We know that all quadratic functions of the type $y = a{x^2} + bc + c$ have minimum values but not maximum.
Since the square is always non-negative, we have ${\left( {x + 5} \right)^2} \ge 0$, then we have
$ \Rightarrow y = {\left( {x + 5} \right)^2} - 3 \ge 0$
So, the minimum value of $y = - 3$ and the minimum value occurs when ${\left( {x + 5} \right)^2} = 0$ or $x = - 5$.
We have already three points for the curve $\left( { - 5 - \sqrt 3 ,0} \right)$, $\left( { - 5 + \sqrt 3 ,0} \right)$ and $\left( {0,22} \right)$. We find y for two more points.
At $x = - 5$ we have,
$ \Rightarrow y = {\left( { - 5 + 5} \right)^2} - 3$
Simplify the terms,
$ \Rightarrow y = {\left( 0 \right)^2} - 3$
Simplify the terms,
$ \Rightarrow y = - 3$
At $x = - 2$ we have,
$ \Rightarrow y = {\left( { - 2 + 5} \right)^2} - 3$
Simplify the terms,
$ \Rightarrow y = {\left( 3 \right)^2} - 3$
Square the term on the right side,
$ \Rightarrow y = 9 - 3$
Simplify the terms,
$ \Rightarrow y = 6$
So, we draw the table for x and y.
We plot the above points and join them to have the graph as
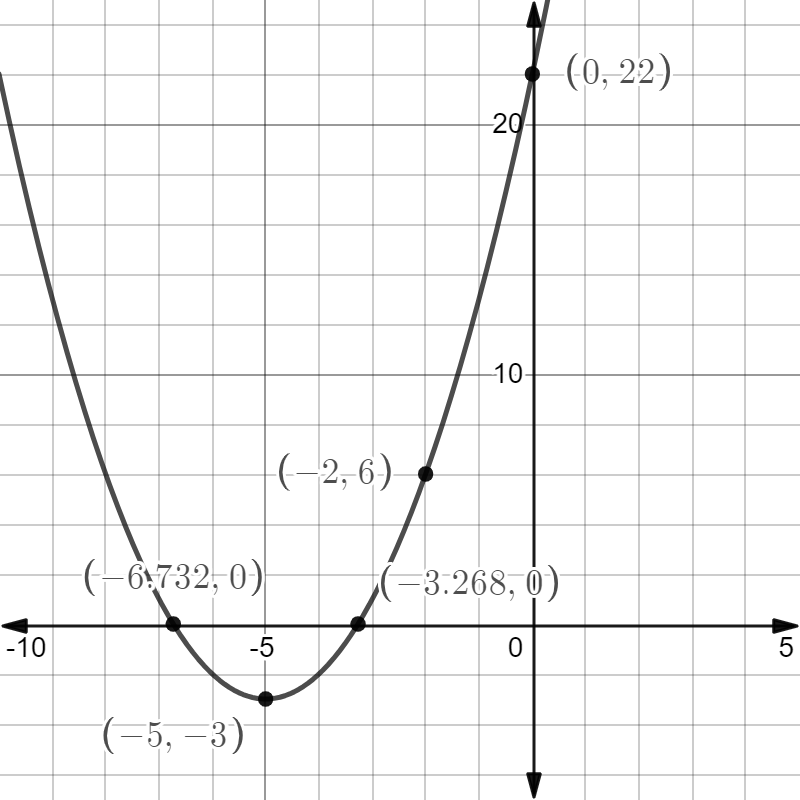
Note: We note that the obtained graph is the graph of the upward parabola whose general equation is given by $y = a{x^2} + bx + c$ with the condition $a > 0$ whose vertex here is $\left( { - 5, - 3} \right)$ . We can directly find the minimum value of $y = {\left( {x + 5} \right)^2} - 3$ by finding $x = - \dfrac{b}{{2a}}$. If $a < 0$ the equation $y = a{x^2} + bx + c$ represents a downward parabola. We also note that the obtained curve is symmetric about the line $x = - 5$.
Complete step by step solution:
We know that the graph of a function is the locus of points $\left( {x,y} \right)$ such that $y = f\left( x \right)$ where x, y are real numbers. We are given the following quadratic polynomial function,
$ \Rightarrow y = {\left( {x + 5} \right)^2} - 3$
So, let us put $y = 0$ and find the x-intercept. We have,
$ \Rightarrow 0 = {\left( {x + 5} \right)^2} - 3$
Move 3 on the other side,
$ \Rightarrow {\left( {x + 5} \right)^2} = 3$
Take the square root on both sides,
$ \Rightarrow x + 5 = \pm \sqrt 3 $
Subtract 5 on both sides,
$ \Rightarrow x + 5 - 5 = \pm \sqrt 3 - 5$
Simplify the terms,
$ \Rightarrow x = - 5 \pm \sqrt 3 $
It means the curve cuts the x-axis at $\left( { - 5 + \sqrt 3 ,0} \right)$ and $\left( { - 5 - \sqrt 3 ,0} \right)$.
Let us put $x = 0$ and find the y-intercept. We have,
$ \Rightarrow y = {\left( {0 + 5} \right)^2} - 3$
Simplify the terms,
$ \Rightarrow y = {\left( 5 \right)^2} - 3$
Square the term on the right side,
$ \Rightarrow y = 25 - 3$
Simplify the terms,
$ \Rightarrow y = 22$
It means the curve cuts the y-axis at $\left( {0,22} \right)$.
We know that all quadratic functions of the type $y = a{x^2} + bc + c$ have minimum values but not maximum.
Since the square is always non-negative, we have ${\left( {x + 5} \right)^2} \ge 0$, then we have
$ \Rightarrow y = {\left( {x + 5} \right)^2} - 3 \ge 0$
So, the minimum value of $y = - 3$ and the minimum value occurs when ${\left( {x + 5} \right)^2} = 0$ or $x = - 5$.
We have already three points for the curve $\left( { - 5 - \sqrt 3 ,0} \right)$, $\left( { - 5 + \sqrt 3 ,0} \right)$ and $\left( {0,22} \right)$. We find y for two more points.
At $x = - 5$ we have,
$ \Rightarrow y = {\left( { - 5 + 5} \right)^2} - 3$
Simplify the terms,
$ \Rightarrow y = {\left( 0 \right)^2} - 3$
Simplify the terms,
$ \Rightarrow y = - 3$
At $x = - 2$ we have,
$ \Rightarrow y = {\left( { - 2 + 5} \right)^2} - 3$
Simplify the terms,
$ \Rightarrow y = {\left( 3 \right)^2} - 3$
Square the term on the right side,
$ \Rightarrow y = 9 - 3$
Simplify the terms,
$ \Rightarrow y = 6$
So, we draw the table for x and y.
| $X$ | $ - 5 - \sqrt 3 $ | $ - 5 + \sqrt 3 $ | $0$ | $-5$ | $-2$ |
| $Y$ | $0$ | $0$ | $22$ | $-3$ | $6$ |
We plot the above points and join them to have the graph as

Note: We note that the obtained graph is the graph of the upward parabola whose general equation is given by $y = a{x^2} + bx + c$ with the condition $a > 0$ whose vertex here is $\left( { - 5, - 3} \right)$ . We can directly find the minimum value of $y = {\left( {x + 5} \right)^2} - 3$ by finding $x = - \dfrac{b}{{2a}}$. If $a < 0$ the equation $y = a{x^2} + bx + c$ represents a downward parabola. We also note that the obtained curve is symmetric about the line $x = - 5$.
Recently Updated Pages
How many sigma and pi bonds are present in HCequiv class 11 chemistry CBSE

Why Are Noble Gases NonReactive class 11 chemistry CBSE

Let X and Y be the sets of all positive divisors of class 11 maths CBSE

Let x and y be 2 real numbers which satisfy the equations class 11 maths CBSE

Let x 4log 2sqrt 9k 1 + 7 and y dfrac132log 2sqrt5 class 11 maths CBSE

Let x22ax+b20 and x22bx+a20 be two equations Then the class 11 maths CBSE

Trending doubts
Fill the blanks with the suitable prepositions 1 The class 9 english CBSE

At which age domestication of animals started A Neolithic class 11 social science CBSE

Which are the Top 10 Largest Countries of the World?

Give 10 examples for herbs , shrubs , climbers , creepers

Difference between Prokaryotic cell and Eukaryotic class 11 biology CBSE

Difference Between Plant Cell and Animal Cell

Write a letter to the principal requesting him to grant class 10 english CBSE

Change the following sentences into negative and interrogative class 10 english CBSE

Fill in the blanks A 1 lakh ten thousand B 1 million class 9 maths CBSE



